A Dynamic Network Model of Societal Complexity and Resilience Inspired by Tainter’s Theory of Collapse
Abstract
1. Introduction
- The basic currency of any society is energy, since labor and material goods can be viewed as driven by or derived from energy.
- Problems need to be solved when energy availability is deficient as a consequence of stochastic events or shocks. According to the self-reinforcing process denoted as energy–complexity spiral (see above), this process always increases societal complexity.
- Increases in complexity can be modeled as increases in administrative capacity because they encapsulate increases in specialization of social roles, hierarchies and control, and information flow. Moreover, the size of an administrative body relative to the overall size of the system is a very tangible example of complexity.
2. Model Description
2.1. Tainter Inspired Network Model of a Hierarchical Society Steering into Diminishing Marginal Returns and Collapse
- (a)
- If currently, no administrators exist in the society (i.e., if ), the laborer L with the most network connections becomes the sole administrator.
- (b)
- If at least one administrator currently exists (i.e., if ), the coordinated laborer who has the most network connections becomes an additional administrator.
2.2. Social Mobility as a Possible Countermeasure to Collapse
3. Results
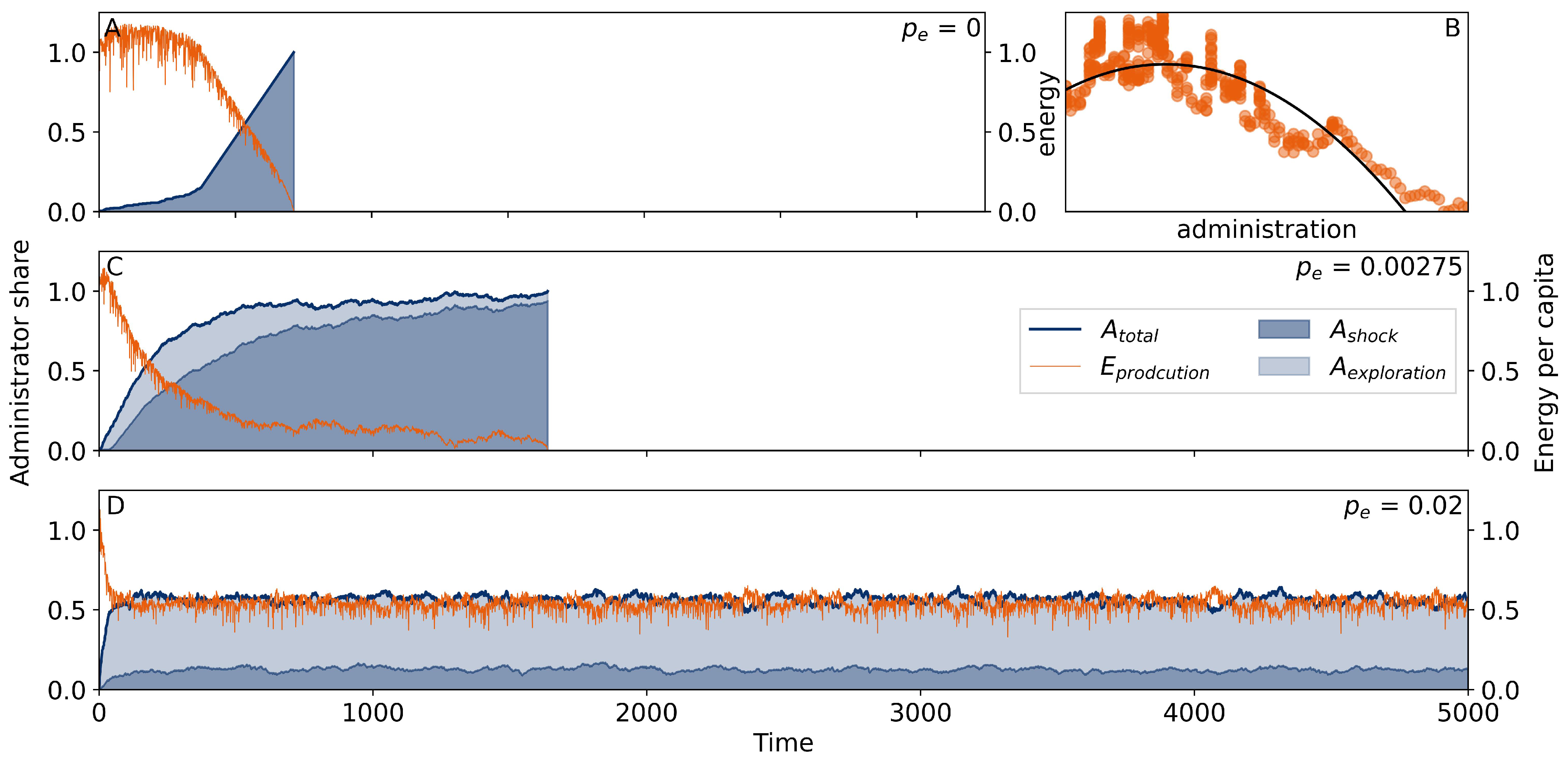
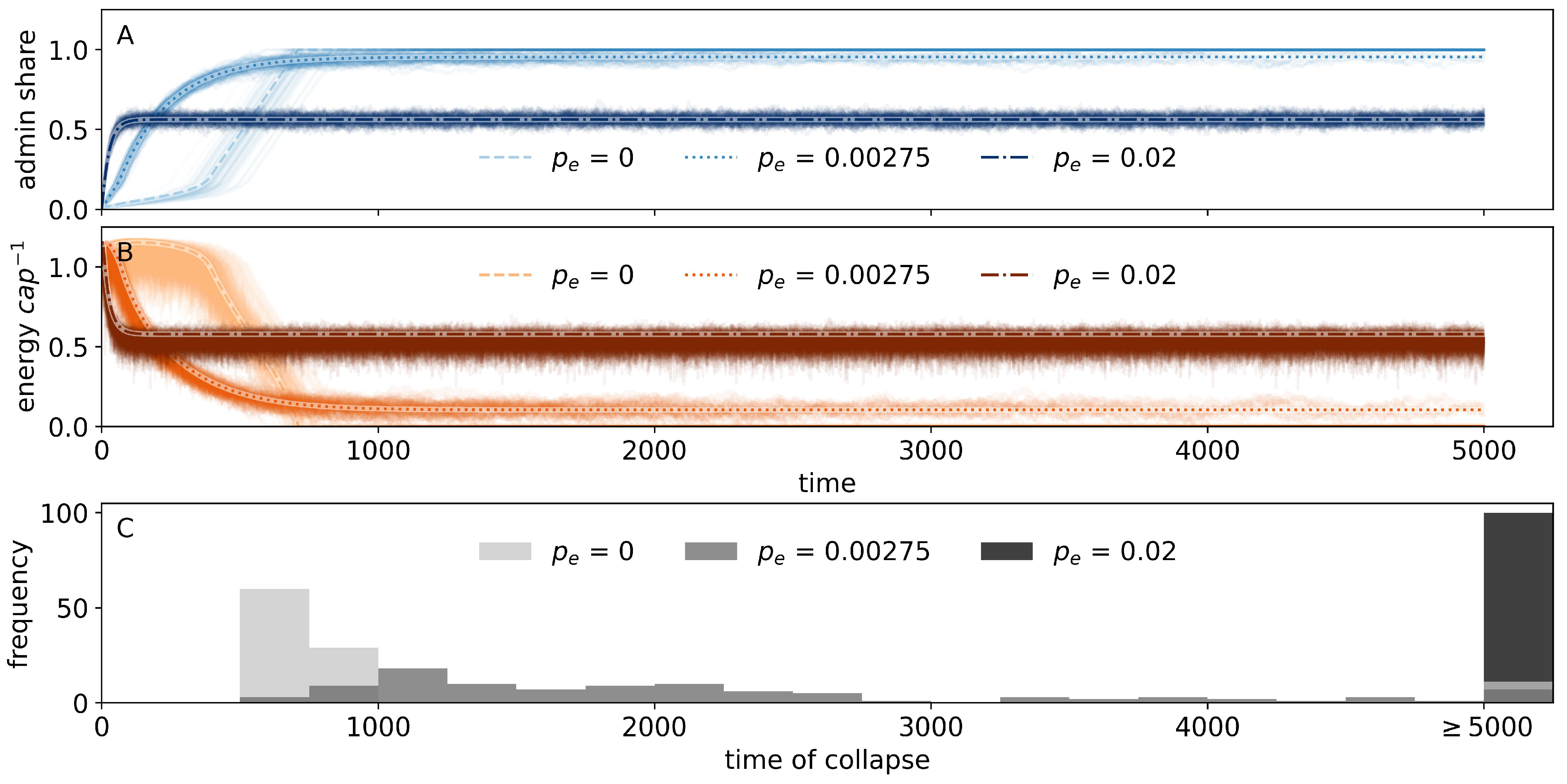
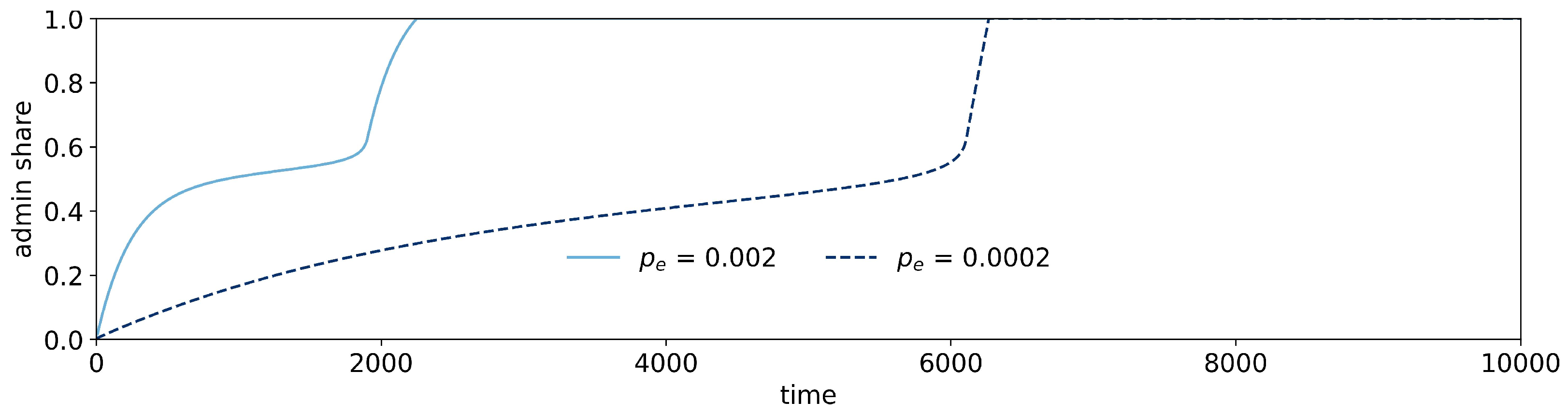
3.1. Example Trajectories
3.2. Deterministic Macroscopic Approximation of the Stochastic Micromodel
3.3. Influence of Model Parameters on Survival Time
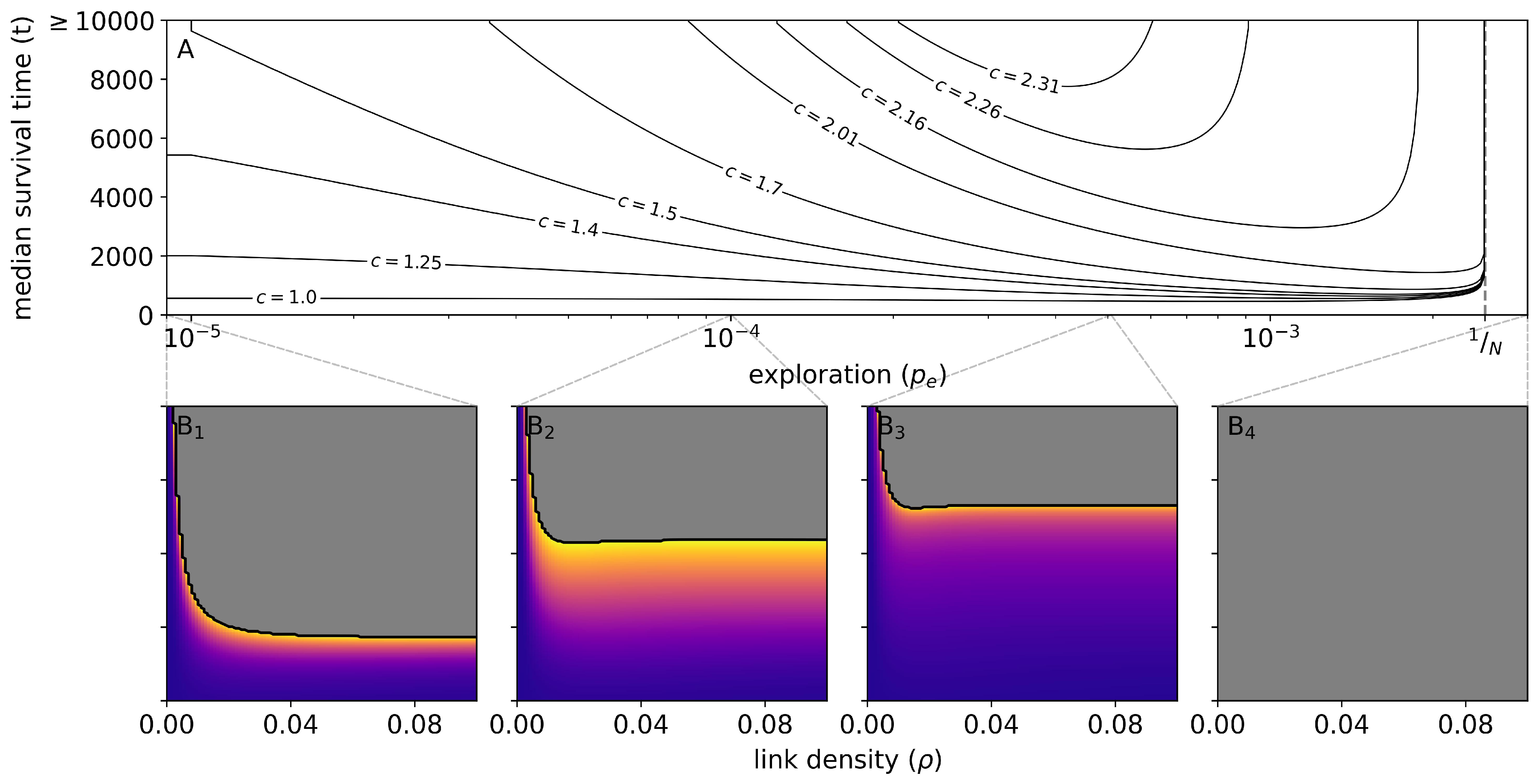
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| ROC | Returns on complexity |
| EROI | Energy return on investment |
Appendix A. Optimal Number of Administrators
References
- Scheffer, M.; Van Nes, E.H.; Kemp, L.; Kohler, T.A.; Lenton, T.M.; Xu, C. The Vulnerability of Aging States: A Survival Analysis across Premodern Societies. Proc. Natl. Acad. Sci. USA 2023, 120, e2218834120. [Google Scholar] [CrossRef] [PubMed]
- Weiss, H.; Courty, M.A.; Wetterstrom, W.; Guichard, F.; Senior, L.; Meadow, R.; Curnow, A. The genesis and collapse of third millennium north mesopotamian civilization. Science 1993, 261, 995–1004. [Google Scholar] [CrossRef] [PubMed]
- Butzer, K.W. Collapse, environment, and society. Proc. Natl. Acad. Sci. USA 2012, 109, 3632–3639. [Google Scholar] [CrossRef] [PubMed]
- Tainter, J.A. The Collapse of Complex Societies; New Studies in Archaeology; Cambridge University Press: Cambridge, UK, 1988. [Google Scholar]
- Middleton, G.D. Nothing Lasts Forever: Environmental Discourses on the Collapse of Past Societies. J. Archaeol. Res. 2012, 20, 257–307. [Google Scholar] [CrossRef]
- Turchin, P. Historical Dynamics: Why States Rise and Fall, 1st ed.; Princeton University Press: Princeton, NJ, USA, 2003. [Google Scholar] [CrossRef]
- Tol, R.S.J. The Economic Impacts of Climate Change. Rev. Environ. Econ. Policy 2018, 12, 4–25. [Google Scholar] [CrossRef]
- World Bank. Central Government Debt, Total (Current LCU); International Monetary Fund, Government Finance Statistics Yearbook and Data Files; World Bank: Washington, DC, USA, 2020. [Google Scholar]
- Trump, B.D.; Linkov, I.; Hynes, W. Combine resilience and efficiency in post-COVID societies. Nature 2020, 588, 220. [Google Scholar] [CrossRef]
- Cumming, G.S.; Peterson, G.D. Unifying Research on Social-Ecological Resilience and Collapse. Trends Ecol. Evol. 2017, 32, 695–713. [Google Scholar] [CrossRef]
- Malthus, T.R. An Essay on the Principle of Population, as It Affects the Future Improvement of Society with Remarks on the Speculations of Mr. Godwin, M. Condorcet and Other Writers; J. Johnson: London, UK, 1798. [Google Scholar]
- Meadows, D.H.; Meadows, D.L.; Jorgen, R.; Behrens, W.W. The Limits to Growth: A Report for the Club of Rome’s Project on the Predicament of Mankind; Universe Books: New York, NY, USA, 1972. [Google Scholar]
- Rockström, J.; Steffen, W.; Noone, K.; Persson, A.; Chapin, F.S.; Lambin, E.F.; Lenton, T.M.; Scheffer, M.; Folke, C.; Schellnhuber, H.J.; et al. A safe operating space for humanity. Nature 2009, 461, 472–475. [Google Scholar] [CrossRef]
- Diamond, J.M. Collapse: How Societies Choose to Fail or Survive/Jared Diamond; Penguin: New York, NY, USA, 2011. [Google Scholar]
- Dixson-Declève, S.; Gaffney, O.; Ghosh, J.; Randers, J.; Rockstrom, J.; Stoknes, P.E. Earth for All: A Survival Guide for Humanity; New Society Publishers: Gabriola Island, BC, Canada, 2022. [Google Scholar]
- Kennett, D.J.; Breitenbach, S.F.M.; Aquino, V.V.; Asmerom, Y.; Awe, J.; Baldini, J.U.; Bartlein, P.; Culleton, B.J.; Ebert, C.; Jazwa, C.; et al. Development and Disintegration of Maya Political Systems in Response to Climate Change. Science 2012, 338, 788–791. [Google Scholar] [CrossRef]
- Acemoglu, D.; Naidu, S.; Restrepo, P.; Robinson, J.A. (Eds.) Democracy, Redistribution, and Inequality. In Handbook of Income Distribution; Elsevier: Amsterdam, The Netherlands, 2015; Volume 2, pp. 1885–1966. [Google Scholar] [CrossRef]
- Tainter, J.A. Social complexity and sustainability. Ecol. Complex. 2006, 3, 91–103. [Google Scholar] [CrossRef]
- Acemoglu, D.; Robinson, J.A. Persistence of Power, Elites, and Institutions. Am. Econ. Rev. 2008, 98, 267–293. [Google Scholar] [CrossRef]
- Feinstein, C. Structural change in the developed countries during the twentieth century. Oxf. Rev. Econ. Policy 1999, 15, 35–55. [Google Scholar] [CrossRef]
- Coglianese, C. Empirical Analysis and Administrative Law. Univ. Ill. Law Rev. 2002, 2002, 1111–1138. [Google Scholar] [CrossRef]
- Steensma, D.P.; Kantarjian, H.M. Impact of cancer research bureaucracy on innovation, costs, and patient care. J. Clin. Oncol. Off. J. Am. Soc. Clin. Oncol. 2014, 32, 376–378. [Google Scholar] [CrossRef] [PubMed]
- Hall, C.; Balogh, S.; Murphy, D. What is the Minimum EROI that a Sustainable Society Must Have? Energies 2009, 2, 25–47. [Google Scholar] [CrossRef]
- Barton, C.M.; Ullah, I.I.; Bergin, S. Land use, water and Mediterranean landscapes: Modelling long-term dynamics of complex socio-ecological systems. Philos. Trans. Ser. A Math. Phys. Eng. Sci. 2010, 368, 5275–5297. [Google Scholar] [CrossRef]
- Barton, C.M.; Riel-Salvatore, J. Agents of Change: Modelling Biocultural Evolution in Upper Pleistocene Western Eurasia. Adv. Complex Syst. 2012, 15, 1150003. [Google Scholar] [CrossRef]
- Kohler, T.A.; van der Leeuw, S.E. The Model-Based Archaeology of Socionatural Systems, 1st ed.; School for Advanced Research Press: Santa Fe, NM, USA, 2007. [Google Scholar]
- Ostrom, E. A general framework for analyzing sustainability of social-ecological systems. Science 2009, 325, 419–422. [Google Scholar] [CrossRef]
- Schlueter, M.; Mcallister, R.R.; Arlinghaus, R.; Bunnefeld, N.; Eisenack, K.; Hoelker, F.; MILNER-GULLAND, E.J.; Müller, B.; Nicholson, E.; Quaas, M.; et al. New horizons for managing the environment: A review of coupled social-ecological systems modeling. Nat. Resour. Model. 2012, 25, 219–272. [Google Scholar] [CrossRef]
- Brander, J.A.; Taylor, S.M. The Simple Economics of Easter Island: A Ricardo-Malthus Model of Renewable Resource Use. Am. Econ. Rev. 1998, 88, 119–138. [Google Scholar]
- Freeman, J.; Anderies, J.M. Intensification, tipping points, and social change in a coupled forager-resource system. Hum. Nat. 2012, 23, 419–446. [Google Scholar] [CrossRef] [PubMed]
- Motesharrei, S.; Rivas, J.; Kalnay, E. Human and nature dynamics (HANDY): Modeling inequality and use of resources in the collapse or sustainability of societies. Ecol. Econ. 2014, 101, 90–102. [Google Scholar] [CrossRef]
- Nitzbon, J.; Heitzig, J.; Parlitz, U. Sustainability, collapse and oscillations in a simple World-Earth model. Environ. Res. Lett. 2017, 12, 074020. [Google Scholar] [CrossRef]
- Anderies, J.M.; Barfuss, W.; Donges, J.F.; Fetzer, I.; Heitzig, J.; Rockström, J. A modeling framework for World-Earth system resilience: Exploring social inequality and Earth system tipping points. Environ. Res. Lett. 2023, 18, 095001. [Google Scholar] [CrossRef]
- Rounsevell, M.D.; Arneth, A. Representing human behaviour and decisional processes in land system models as an integral component of the earth system. Glob. Environ. Chang. 2011, 21, 840–843. [Google Scholar] [CrossRef]
- Arneth, A.; Brown, C.; Rounsevell, M.D.A. Global models of human decision-making for land-based mitigation and adaptation assessment. Nat. Clim. Chang. 2014, 4, 550–557. [Google Scholar] [CrossRef]
- Brown, C.; Seo, B.; Rounsevell, M. Societal breakdown as an emergent property of large-scale behavioural models of land use change. Earth Syst. Dyn. 2019, 10, 809–845. [Google Scholar] [CrossRef]
- Schlüter, M.; Baeza, A.; Dressler, G.; Frank, K.; Groeneveld, J.; Jager, W.; Janssen, M.A.; McAllister, R.R.; Müller, B.; Orach, K.; et al. A framework for mapping and comparing behavioural theories in models of social-ecological systems. Ecol. Econ. 2017, 131, 21–35. [Google Scholar] [CrossRef]
- Schill, C.; Anderies, J.M.; Lindahl, T.; Folke, C.; Polasky, S.; Cárdenas, J.C.; Crépin, A.S.; Janssen, M.A.; Norberg, J.; Schlüter, M. A more dynamic understanding of human behaviour for the Anthropocene. Nat. Sustain. 2019, 2, 1075–1082. [Google Scholar] [CrossRef]
- Beckage, B.; Moore, F.C.; Lacasse, K. Incorporating human behaviour into Earth system modelling. Nat. Hum. Behav. 2022, 6, 1493–1502. [Google Scholar] [CrossRef]
- Moore, F.C.; Lacasse, K.; Mach, K.J.; Shin, Y.A.; Gross, L.J.; Beckage, B. Determinants of emissions pathways in the coupled climate–social system. Nature 2022, 603, 103–111. [Google Scholar] [CrossRef]
- Wiedermann, M.; Smith, E.K.; Heitzig, J.; Donges, J.F. A network-based microfoundation of Granovetter’s threshold model for social tipping. Sci. Rep. 2020, 10, 11202. [Google Scholar] [CrossRef] [PubMed]
- Donges, J.F.; Heitzig, J.; Barfuss, W.; Wiedermann, M.; Kassel, J.A.; Kittel, T.; Kolb, J.J.; Kolster, T.; Müller-Hansen, F.; Otto, I.M.; et al. Earth system modeling with endogenous and dynamic human societies: The copan:CORE open World–Earth modeling framework. Earth Syst. Dyn. 2020, 11, 395–413. [Google Scholar] [CrossRef]
- Bardi, U.; Falsini, S.; Perissi, I. Toward a General Theory of Societal Collapse: A Biophysical Examination of Tainter’s Model of the Diminishing Returns of Complexity. Biophys. Econ. Resour. Qual. 2019, 4, 3. [Google Scholar] [CrossRef]
- Newman, M. The Structure and Function of Complex Networks. SIAM Rev. 2003, 45, 167–256. [Google Scholar] [CrossRef]
- Castellano, C.; Fortunato, S.; Loreto, V. Statistical physics of social dynamics. Rev. Mod. Phys. 2009, 81, 591. [Google Scholar] [CrossRef]
- Heckbert, S. MayaSim: An Agent-Based Model of the Ancient Maya Social-Ecological System. J. Artif. Soc. Soc. Simul. 2013, 16, 11. [Google Scholar] [CrossRef]
- Wiedermann, M.; Donges, J.F.; Heitzig, J.; Lucht, W.; Kurths, J. Macroscopic description of complex adaptive networks coevolving with dynamic node states. Phys. Rev. E 2015, 91, 052801. [Google Scholar] [CrossRef]
- Schleussner, C.F.; Donges, J.F.; Engemann, D.A.; Levermann, A. Clustered marginalization of minorities during social transitions induced by co-evolution of behaviour and network structure. Sci. Rep. 2016, 6, 30790. [Google Scholar] [CrossRef]
- Erdos, P.; Rényi, A. On the Evolution of Random Graphs. Proc. Publ. Math. Inst. Hung. Acad. Sci. 1960, 5, 17–61. [Google Scholar]
- Stawarz, N. Patterns of intragenerational social mobility: An analysis of heterogeneity of occupational careers. Adv. Life Course Res. 2018, 38, 1–11. [Google Scholar] [CrossRef]
- Adermon, A.; Lindahl, M.; Waldenström, D. Intergenerational Wealth Mobility and the Role of Inheritance: Evidence from Multiple Generations. Econ. J. 2018, 128, F482–F513. [Google Scholar] [CrossRef]
- Guzmán, R.A.; Drobny, S.; Rodríguez-Sickert, C. The Ecosystems of Simple and Complex Societies: Social and Geographical Dynamics. J. Artif. Soc. Soc. Simul. 2018, 21. [Google Scholar] [CrossRef]
- Laurence, P.J. The Peter Principle: Why Things Always Go Wrong; William Morrow: New York, NY, USA, 1969. [Google Scholar]
- Pluchino, A.; Rapisarda, A.; Garofalo, C. The Peter Principle Revisited: A Computational Study. Phys. A Stat. Mech. Its Appl. 2010, 389, 467–472. [Google Scholar] [CrossRef]
- Watson, A.J.; Lovelock, J.E. Biological homeostasis of the global environment: The parable of Daisyworld. Tellus B Chem. Phys. Meteorol. 1983, 35, 284–289. [Google Scholar] [CrossRef]
- Granovetter, M. Threshold Models of Collective Behavior. Am. J. Sociol. 1978, 83, 1420–1443. [Google Scholar] [CrossRef]
- Parkinson, C.N.; Lancaster, O. Parkinson’s Law or The Pursuit of Progress; Sidgwick & Jackson: London, UK, 1986. [Google Scholar]
- Weber, M. Wirtschaft und Gesellschaft; Mohr: Tübingen, Germany, 1922. [Google Scholar]
- Turchin, P.; Whitehouse, H.; François, P.; Hoyer, D.; Alves, A.; Baines, J.; Baker, D.; Bartkowiak, M.; Bates, J.; Bennett, J.; et al. An Introduction to Seshat: Global History Databank. J. Cogn. Hist. 2020, 5, 115–123. [Google Scholar] [CrossRef]
- Lenton, T.M.; Pichler, P.P.; Weisz, H. Revolutions in energy input and material cycling in Earth history and human history. Earth Syst. Dyn. 2016, 7, 353–370. [Google Scholar] [CrossRef]
- Tamberg, L.A.; Heitzig, J.; Donges, J.F. A Modeler’s Guide to Studying the Resilience of Social-Technical-Environmental Systems. Environ. Res. Lett. 2022, 17, 055005. [Google Scholar] [CrossRef]
- Helbing, D. Globally networked risks and how to respond. Nature 2013, 497, 51–59. [Google Scholar] [CrossRef]
- Rocha, J.C.; Peterson, G.; Bodin, Ö.; Levin, S. Cascading regime shifts within and across scales. Science 2018, 362, 1379–1383. [Google Scholar] [CrossRef] [PubMed]

| Parameter | Function | Value |
|---|---|---|
| N | Network size (number of nodes) | 400 |
| Maximal runtime of the simulation (time steps) | 10,000 | |
| Parameter of shock regulating the Beta distribution | 1 | |
| Parameter of shock regulating the Beta distribution | 15 | |
| Energy threshold for coordinating a new A | 1.0 | |
| a | Output elasticity to scale of uncoordinated workers L | 0.75 |
| b | Output elasticity to scale of coordinated workers C | 0.75 |
| c | Productivity of coordinated workers C | 1.05 |
| Link probability between nodes | 0.02 | |
| Exploration probability between node states | 0.00 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Schunck, F.; Wiedermann, M.; Heitzig, J.; Donges, J.F. A Dynamic Network Model of Societal Complexity and Resilience Inspired by Tainter’s Theory of Collapse. Entropy 2024, 26, 98. https://doi.org/10.3390/e26020098
Schunck F, Wiedermann M, Heitzig J, Donges JF. A Dynamic Network Model of Societal Complexity and Resilience Inspired by Tainter’s Theory of Collapse. Entropy. 2024; 26(2):98. https://doi.org/10.3390/e26020098
Chicago/Turabian StyleSchunck, Florian, Marc Wiedermann, Jobst Heitzig, and Jonathan F. Donges. 2024. "A Dynamic Network Model of Societal Complexity and Resilience Inspired by Tainter’s Theory of Collapse" Entropy 26, no. 2: 98. https://doi.org/10.3390/e26020098
APA StyleSchunck, F., Wiedermann, M., Heitzig, J., & Donges, J. F. (2024). A Dynamic Network Model of Societal Complexity and Resilience Inspired by Tainter’s Theory of Collapse. Entropy, 26(2), 98. https://doi.org/10.3390/e26020098







