Response Analysis of the Three-Degree-of-Freedom Vibroimpact System with an Uncertain Parameter
Abstract
1. Introduction
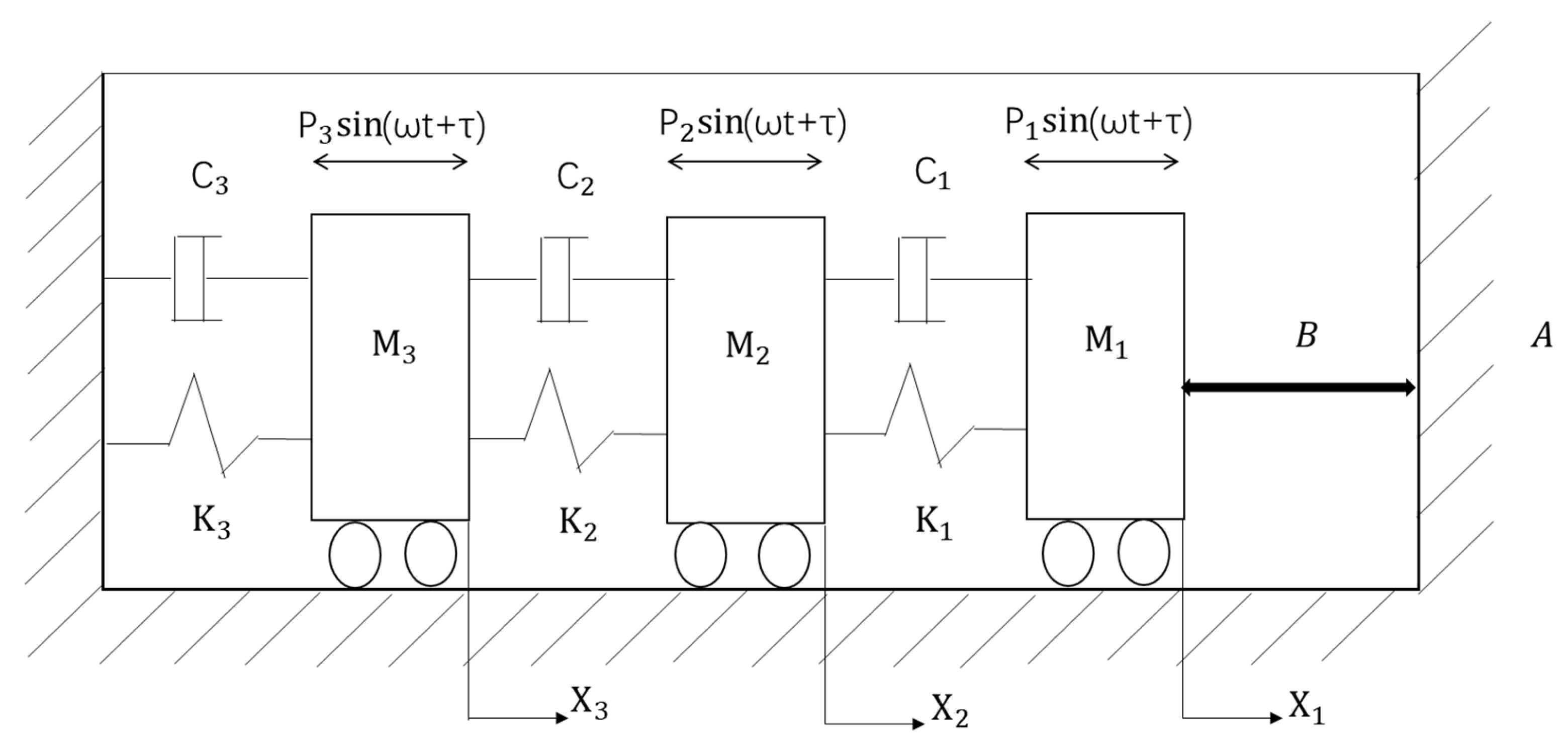
2. Model of the Three-Degree-of-Freedom Vibroimpact System with an Uncertain Parameter
3. The Approximation of the Vibroimpact System with an Uncertain Parameter
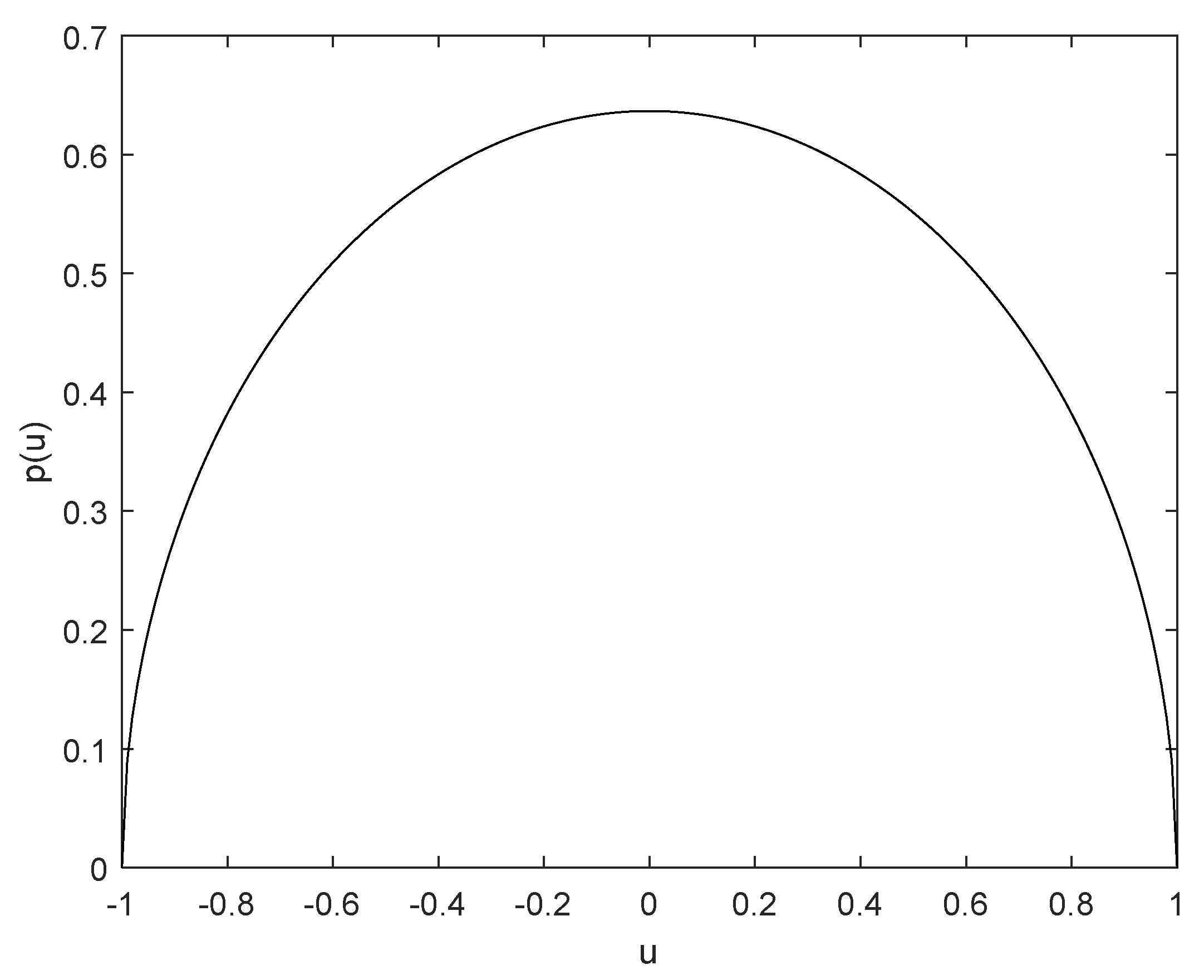
3.1. Chebyshev Polynomial Approximation
3.2. Equivalent Deterministic System
4. Reponses of the Three-Degree-of-Freedom Vibroimpact System
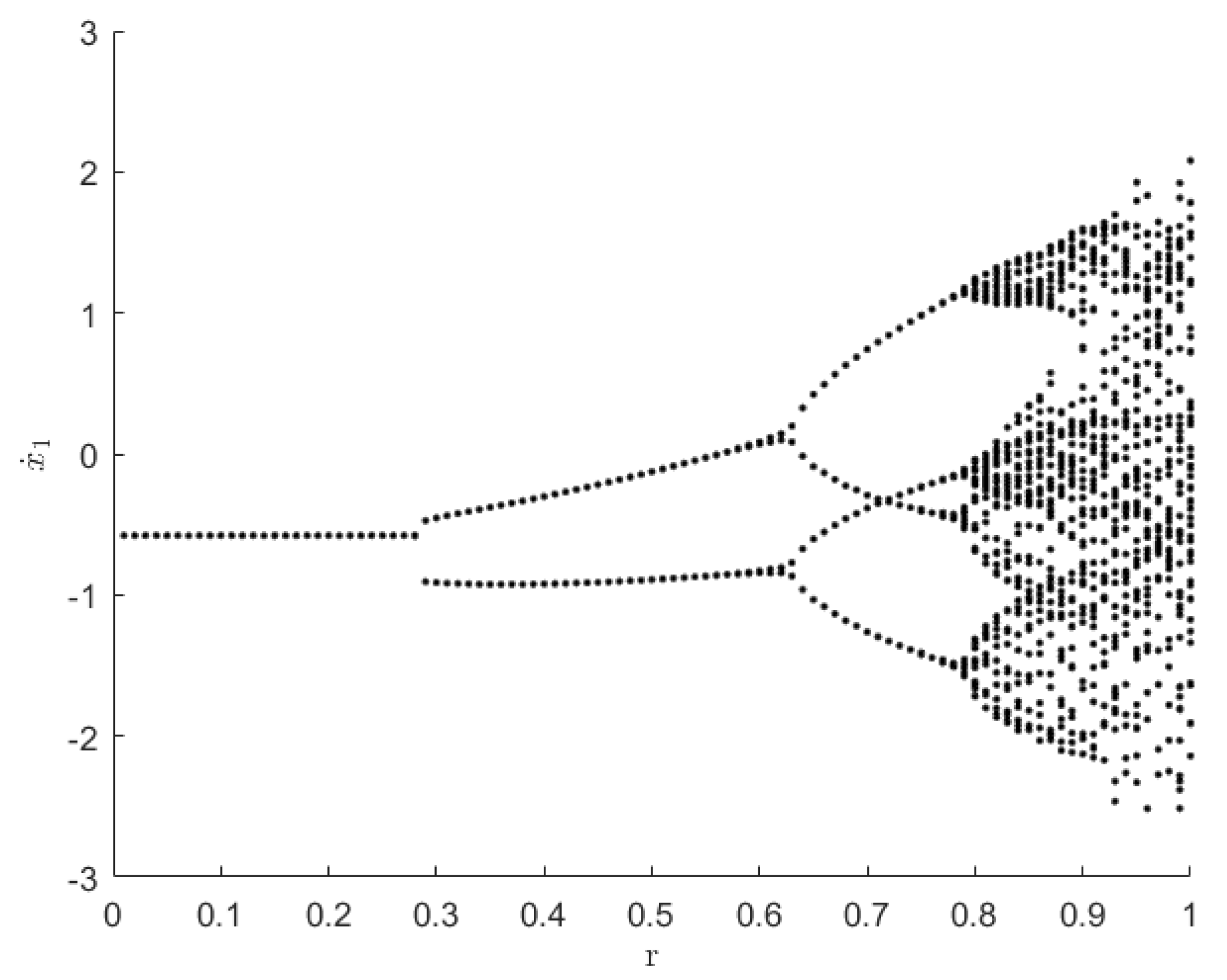
4.1. Period-Doubling Bifurcation
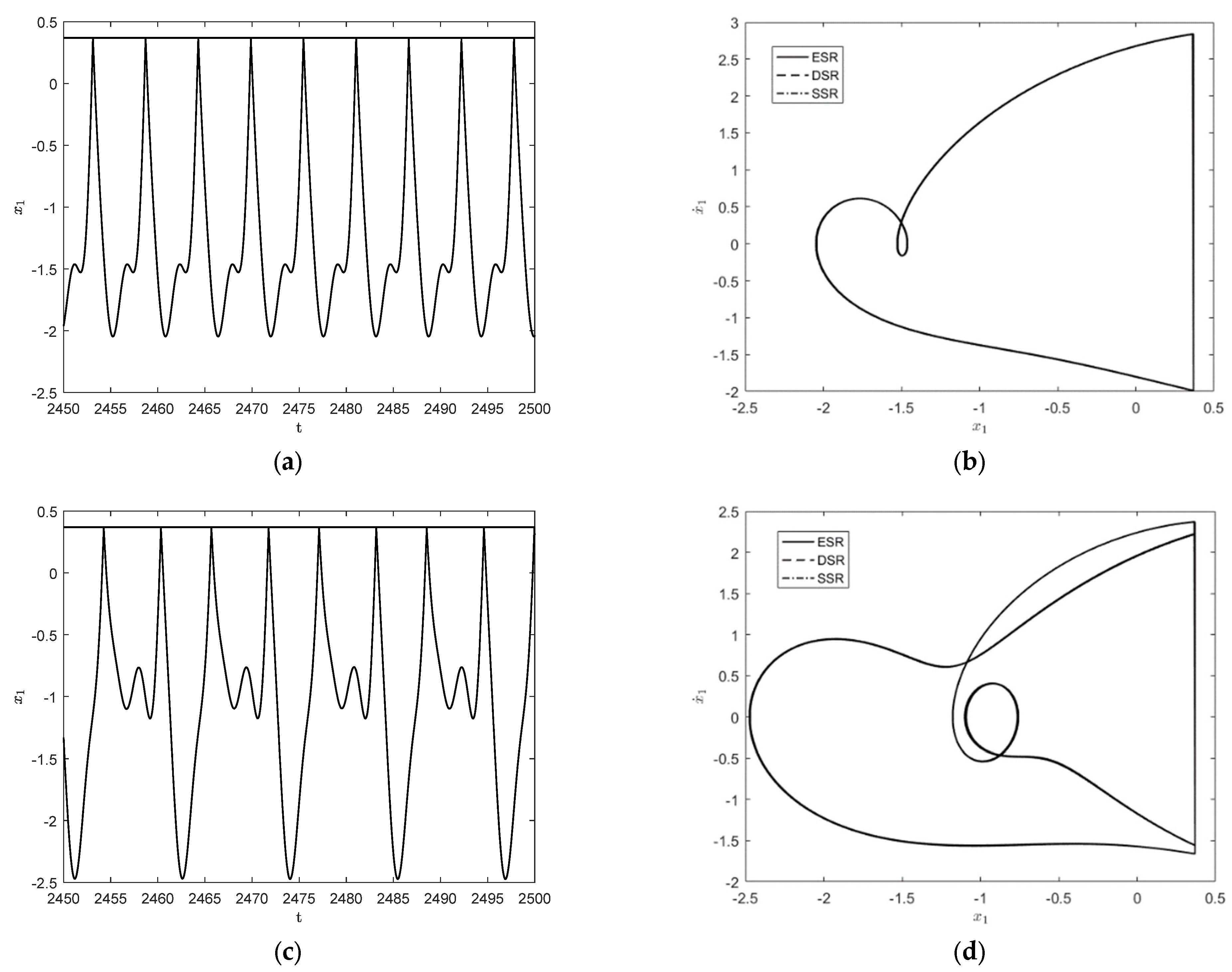
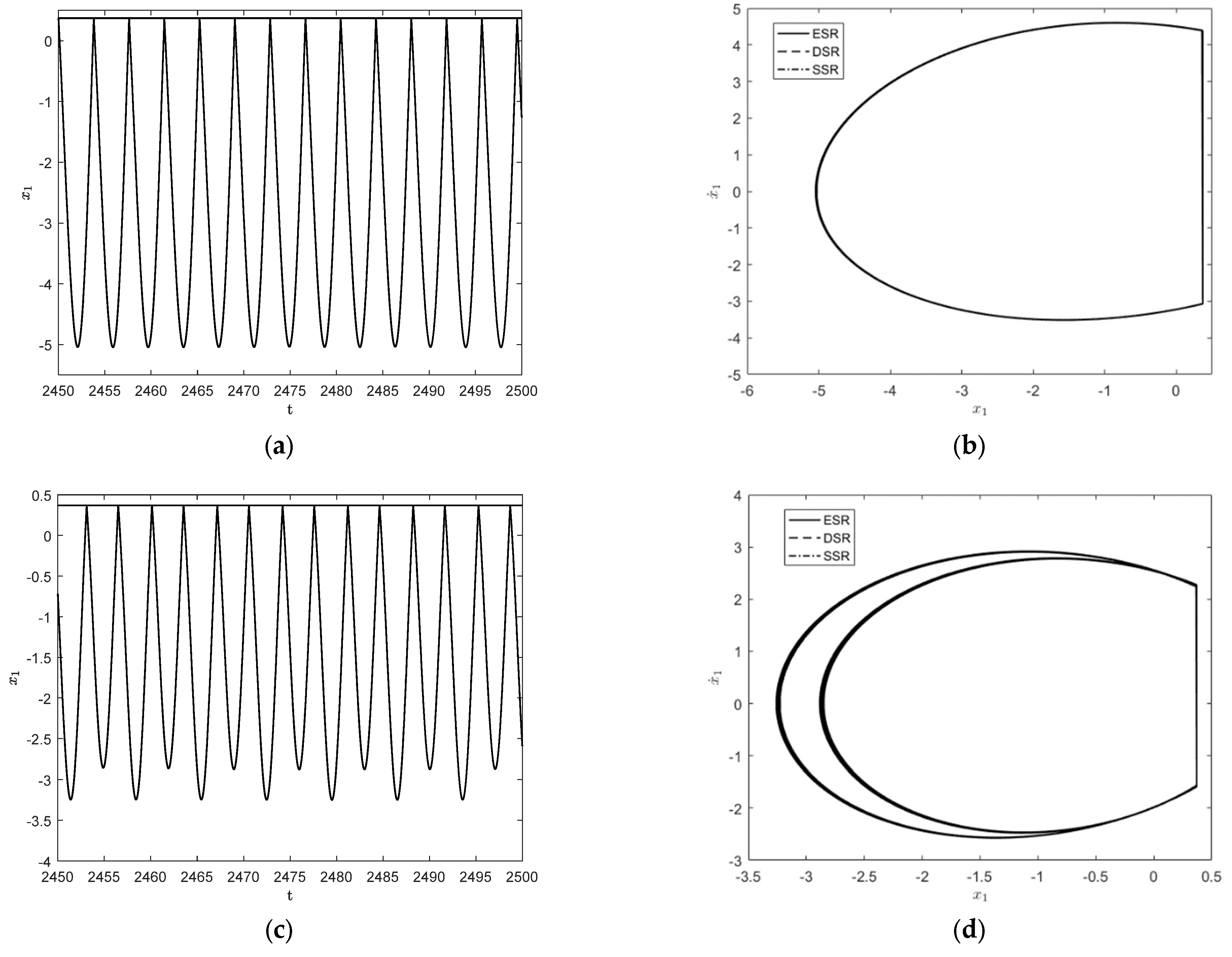
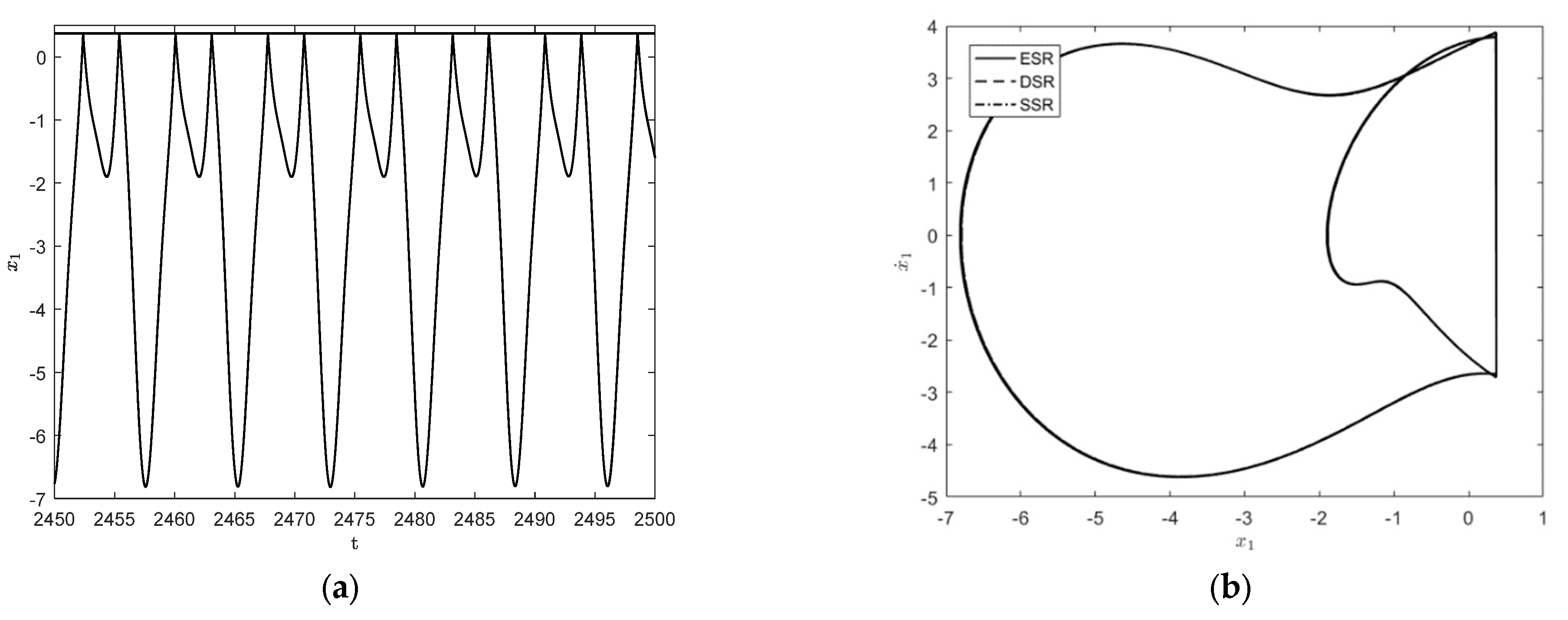
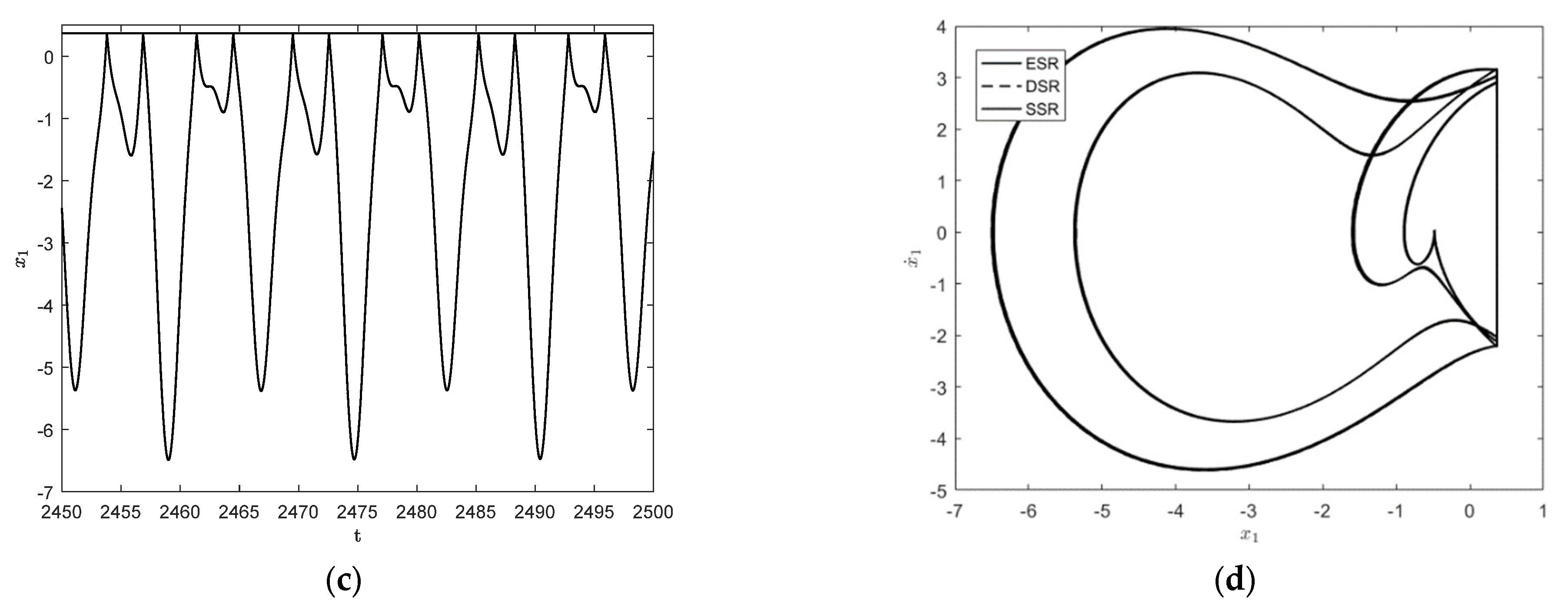
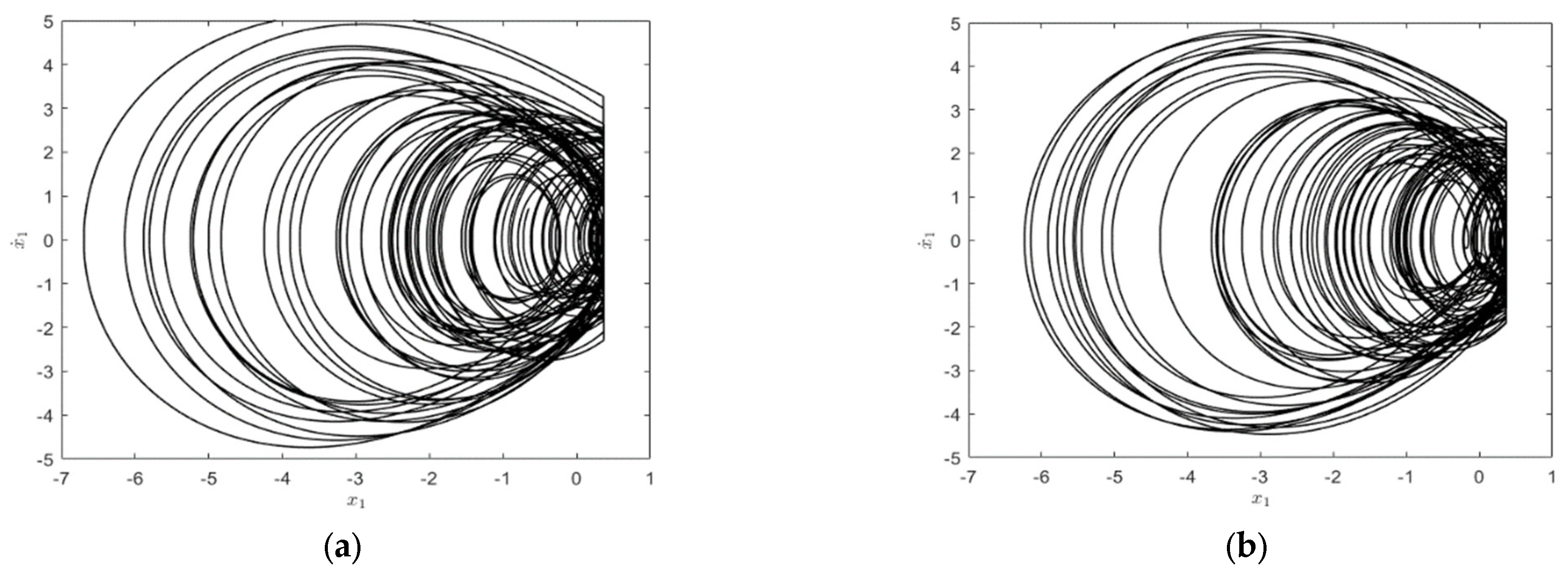
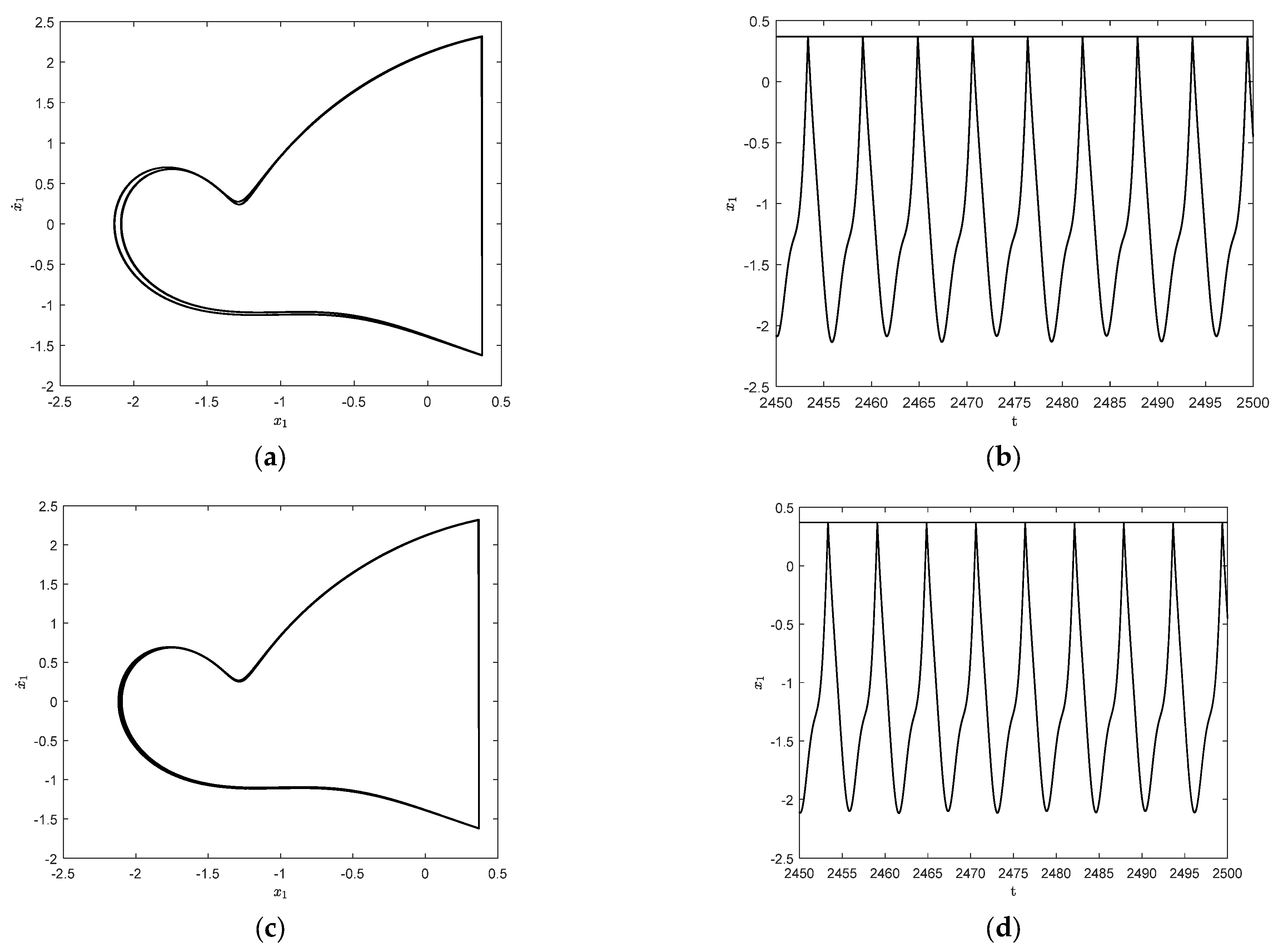
4.2. From Period-Doubling Bifurcation to Chaos
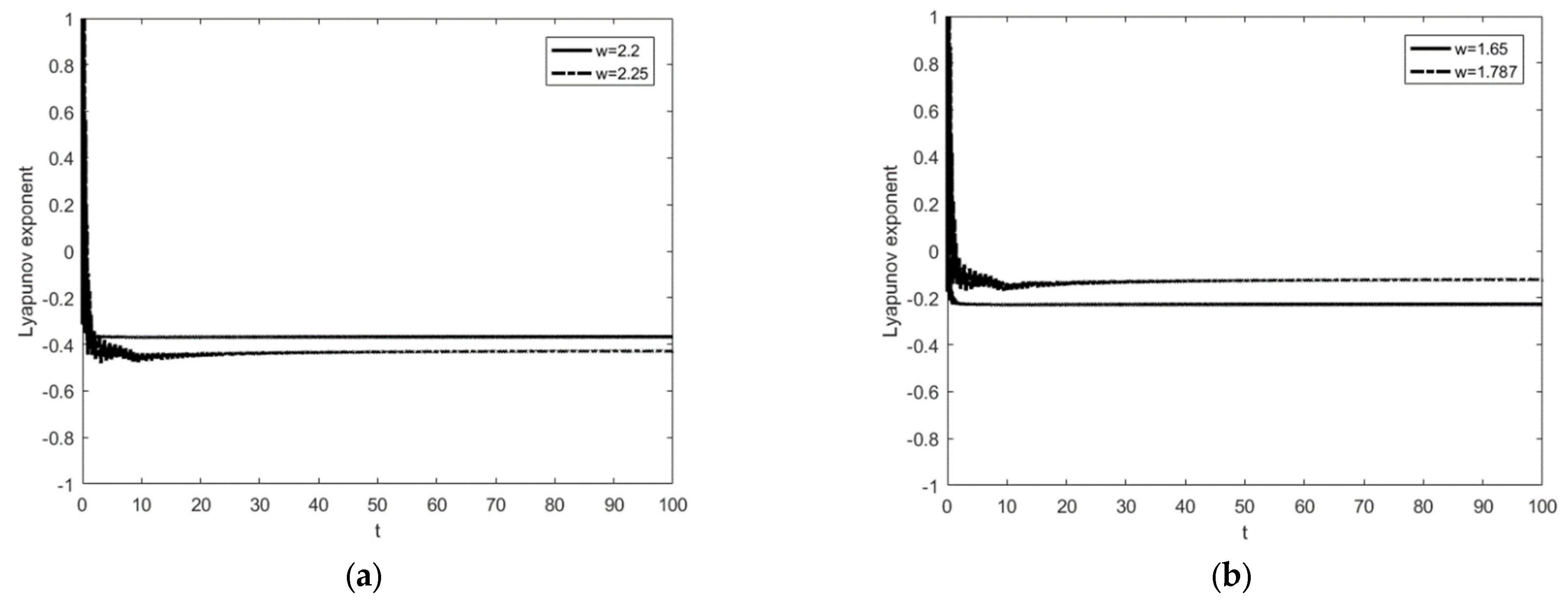
4.3. Influence of the Restitution Coefficient
4.4. Influence of the Uncertain Parameter
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Dimentberg, M.F.; Iourtchenko, D.V. Random vibrations with impacts: A review. Nonlinear Dyn. 2004, 36, 229–254. [Google Scholar] [CrossRef]
- Guo, Y.; Yin, X.; Yu, B.; Hao, Q.; Xiao, X.; Jiang, L.; Wang, H.; Chen, C.; Xie, W.; Ding, H.; et al. Experimental analysis of dynamic behavior of elastic visco-plastic beam under repeated mass impacts. Int. J. Impact. Eng. 2023, 171, 104371. [Google Scholar] [CrossRef]
- Wang, L.; Wang, B.; Peng, J.; Yue, X.; Xu, W. Stochastic response of a vibro-impact system via a new impact-to-impact mapping. Int. J. Bifurcat. Chaos 2021, 31, 2150139. [Google Scholar] [CrossRef]
- Chen, L.; Zhu, H.; Sun, J.Q. Novel method for random vibration analysis of single-degree-of-freedom vibroimpact systems with bilateral barriers. Appl. Math. Mech. 2019, 40, 1759–1776. [Google Scholar] [CrossRef]
- Yue, Y.; Xie, J.H. Symmetry and bifurcations of a two-degree-of-freedom vibro-impact system. J. Sound. Vib. 2008, 314, 228–245. [Google Scholar] [CrossRef]
- Xu, Y.; Liu, Q.; Guo, G.; Xu, C.; Liu, D. Dynamical responses of airfoil models with harmonic excitation under uncertain disturbance. Nonlinear Dyn. 2017, 89, 1579–1590. [Google Scholar] [CrossRef]
- Huang, D.M.; Han, J.; Zhou, S.X.; Han, Q.; Yang, G.D.; Yurchenko, D. Stochastic and deterministic responses of an asymmetric quad-stable energy harvester. Mech. Syst. Signal Process. 2022, 168, 108672. [Google Scholar] [CrossRef]
- Hou, T.; Liu, S.; Ling, J.; Tian, Y.; Li, P.; Zhang, J. Vibration Response Law of Aircraft Taxiing under Random Roughness Excitation. Appl. Sci. 2023, 13, 7386. [Google Scholar] [CrossRef]
- Zhu, W.Q.; Cai, G.Q. Nonlinear stochastic dynamics: A survey of recent developments. Acta Mech. Sin. 2002, 18, 551–566. [Google Scholar]
- Ibrahim, R.A. Vibro-Impact Dynamics: Modeling, Mapping and Applications; Springer: Berlin/Heidelberg, Germany, 2009; Volume 43. [Google Scholar]
- Whiston, G.S. Singularities in vibro-impact dynamics. J. Sound Vib. 1992, 152, 427–460. [Google Scholar] [CrossRef]
- Knudsen, J.; Massih, A.R. Vibro-impact dynamics of a periodically forced beam. J. Pressure Vessel Technol. 2000, 122, 210–221. [Google Scholar] [CrossRef]
- Iourtchenko, D.V.; Song, L. Numerical investigation of a response probability density function of stochastic vibroimpact systems with inelastic impacts. Int. J. Non-Linear Mech. 2006, 41, 447–455. [Google Scholar] [CrossRef]
- Shaw, S.W.; Holmes, P.J. A periodically forced impact oscillator with large dissipation. J. Appl. Mech. 1983, 50, 849–857. [Google Scholar] [CrossRef]
- Shaw, S.W. The dynamics of a harmonically excited system having rigid amplitude constraints, Part 1: Subharmonic motions and local bifurcations. J. Appl. Mech. 1985, 52, 453–458. [Google Scholar] [CrossRef]
- Shaw, S.W. The dynamics of a harmonically excited system having rigid amplitude constraints, Part 2: Chaotic motions and Glocal bifurcations. J. Appl. Mech. 1985, 52, 459–464. [Google Scholar] [CrossRef]
- Mason, J.F.; Humphries, N.; Piiroinen, P.T. Numerical analysis of codimension-one,-two and-three bifurcations in a periodically-forced impact oscillator with two discontinuity surfaces. Math. Comput. Simul. 2014, 95, 98–110. [Google Scholar] [CrossRef]
- Li, C.; Xu, W.; Yue, X.L. Stochastic response of a vibro-impact system by path integration based on generalized cell mapping method. Int. J. Bifurcat. Chaos 2014, 24, 1450129. [Google Scholar] [CrossRef]
- Liu, L.; Xu, W.; Yue, X.L.; Han, Q. Global analysis of crises in a Duffing vibro-impact oscillator with non-viscously damping. Acta Phys. Sin. 2013, 62, 200501. [Google Scholar] [CrossRef]
- Qian, J.; Chen, L.; Sun, J.Q. Random vibration analysis of vibro-impact systems: RBF neural network method. Int. J. Non-Linear Mech. 2023, 148, 104261. [Google Scholar] [CrossRef]
- Ding, W.C.; Li, G.W.; Luo, G.W.; Xie, J.H. Torus T2 and its locking, doubling, chaos of a vibro-impact system. J. Franklin Inst. 2012, 349, 337–348. [Google Scholar] [CrossRef]
- Sun, Y.J.; Xu, J.Q.; Qiang, H.Y.; Wang, W.J.; Lin, G.B. Hopf bifurcation analysis of maglev vehicle–guideway interaction vibration system and stability control based on fuzzy adaptive theory. Comput. Ind. 2019, 108, 197–209. [Google Scholar] [CrossRef]
- Luo, G.W.; Xie, J.H. Hopf bifurcation of a two-degree-of-freedom vibro-impact system. J. Sound Vib. 1998, 213, 391–408. [Google Scholar] [CrossRef]
- Luo, G.W.; Xie, J.H. Hopf bifurcations and chaos of a two-degree-of-freedom vibro-impact system in two strong resonance cases. Int. J. Non-Linear Mech. 2002, 37, 19–34. [Google Scholar] [CrossRef]
- Chin, W.; Ott, E.; Nusse, H.E.; Grebogi, C. Grazing bifurcations in impact oscillators. Phys. Rev. E 1994, 50, 4427. [Google Scholar] [CrossRef] [PubMed]
- Nordmark, A.B. Existence of periodic orbits in grazing bifurcations of impacting mechanical oscillators. Nonlinearity 2001, 14, 1517. [Google Scholar] [CrossRef]
- Budd, C.J. Non-smooth dynamical systems and the grazing bifurcation. Nonlinear Math. Its Appl. 1996, 219–235. [Google Scholar]
- Wagg, D.J. Periodic sticking motion in a two-degree-of-freedom impact oscillator. Int. J. Non-Linear Mech. 2005, 40, 1076–1087. [Google Scholar] [CrossRef]
- Li, X.; Yao, Z.; Wu, R. Modeling and sticking motion analysis of a vibro-impact system in linear ultrasonic motors. Int. J. Mech Sci. 2015, 100, 23–31. [Google Scholar] [CrossRef]
- Dankowicz, H.; Fotsch, E. On the analysis of chatter in mechanical systems with impacts. Procedia IUTAM 2017, 20, 18–25. [Google Scholar] [CrossRef]
- Wagg, D.J.; Bishop, S.R. Chatter, sticking and chaotic impacting motion in a two-degree of freedom impact oscillator. Int. J. Bifurcat. Chaos 2001, 11, 57–71. [Google Scholar] [CrossRef]
- Budd, C.J.; Piiroinen, P.T. Corner bifurcations in non-smoothly forced impact oscillators. Phys. D 2006, 220, 127–145. [Google Scholar] [CrossRef][Green Version]
- Di Bernardo, M.; Budd, C.J.; Champneys, A.R. Corner collision implies border-collision bifurcation. Phys. D 2001, 154, 171–194. [Google Scholar] [CrossRef]
- Xu, W.; Yang, G.D.; Yue, X.L. P-bifurcations of a Duffing-Rayleigh vibroimpact system under stochastic parametric excitation. Acta Phys. Sin. 2016, 65, 210501. [Google Scholar] [CrossRef]
- Zhu, H.T. Stochastic response of vibro-impact Duffing oscillators under external and parametric Gaussian white noises. J. Sound Vib. 2014, 333, 954–961. [Google Scholar] [CrossRef]
- Yang, G.D.; Xu, W.; Feng, J.Q.; Gu, X.D. Response analysis of Rayleigh–Van der Pol vibroimpact system with inelastic impact under two parametric white-noise excitations. Nonlinear Dyn. 2015, 82, 1797–1810. [Google Scholar] [CrossRef]
- Liu, L.; Xu, W.; Yang, G.D.; Huang, D.M. Reliability and control of strongly nonlinear vibro-impact system under external and parametric Gaussian noises. Sci. China Technol. Sci. 2020, 63, 1837–1845. [Google Scholar] [CrossRef]
- Sampaio, R.; Soize, C. On measures of nonlinearity effects for uncertain dynamical systems—Application to a vibro-impact system. J. Sound Vib. 2007, 303, 659–674. [Google Scholar] [CrossRef]
- Lima, R.; Sampaio, R. Energy behavior of an electromechanical system with internal impacts and uncertainties. J. Sound Vib. 2016, 373, 180–191. [Google Scholar] [CrossRef]
- Feng, J.Q.; Xu, W.; Wang, R. Period-doubling bifurcation of stochastic Duffing one-sided constraint system. Acta Phys. Sin. 2006, 55, 5733–5739. [Google Scholar] [CrossRef]
- Sun, X.J.; Xu, W.; Ma, S.J. Period-doubling bifurcation of a double-well Duffing-van der Pol system with bounded random parameters. Acta Phys. Sin. 2006, 55, 610. [Google Scholar]
- Ma, S.J.; Xu, W.; Li, W.; Jin, Y.F. Period-doubling bifurcation analysis of stochastic van der Pol system via Chebyshev polynomial approximation. Acta Phys. Sin. 2005, 54, 3508–3515. [Google Scholar]
- Zhang, Y.; Zhang, J.; Gu, S.H. Periodic motions and bifurcations of a three-degree-of-freedom vibration system with a rigid constrain. J. Lanzhou Jiaotong Univ. 2006, 25, 39–43. [Google Scholar]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, G.; Deng, Z.; Du, L.; Lin, Z. Response Analysis of the Three-Degree-of-Freedom Vibroimpact System with an Uncertain Parameter. Entropy 2023, 25, 1365. https://doi.org/10.3390/e25091365
Yang G, Deng Z, Du L, Lin Z. Response Analysis of the Three-Degree-of-Freedom Vibroimpact System with an Uncertain Parameter. Entropy. 2023; 25(9):1365. https://doi.org/10.3390/e25091365
Chicago/Turabian StyleYang, Guidong, Zichen Deng, Lin Du, and Zicheng Lin. 2023. "Response Analysis of the Three-Degree-of-Freedom Vibroimpact System with an Uncertain Parameter" Entropy 25, no. 9: 1365. https://doi.org/10.3390/e25091365
APA StyleYang, G., Deng, Z., Du, L., & Lin, Z. (2023). Response Analysis of the Three-Degree-of-Freedom Vibroimpact System with an Uncertain Parameter. Entropy, 25(9), 1365. https://doi.org/10.3390/e25091365





