Non-Equilibrium Thermodynamics of Heat Transport in Superlattices, Graded Systems, and Thermal Metamaterials with Defects
Abstract
1. Introduction
2. Current Developments and Frontiers in Heat Transport
2.1. Simplified Illustrative Expression for the Thermal Conductivity
2.2. Three Current Frontiers in Functional Materials for Controlled Heat Transport
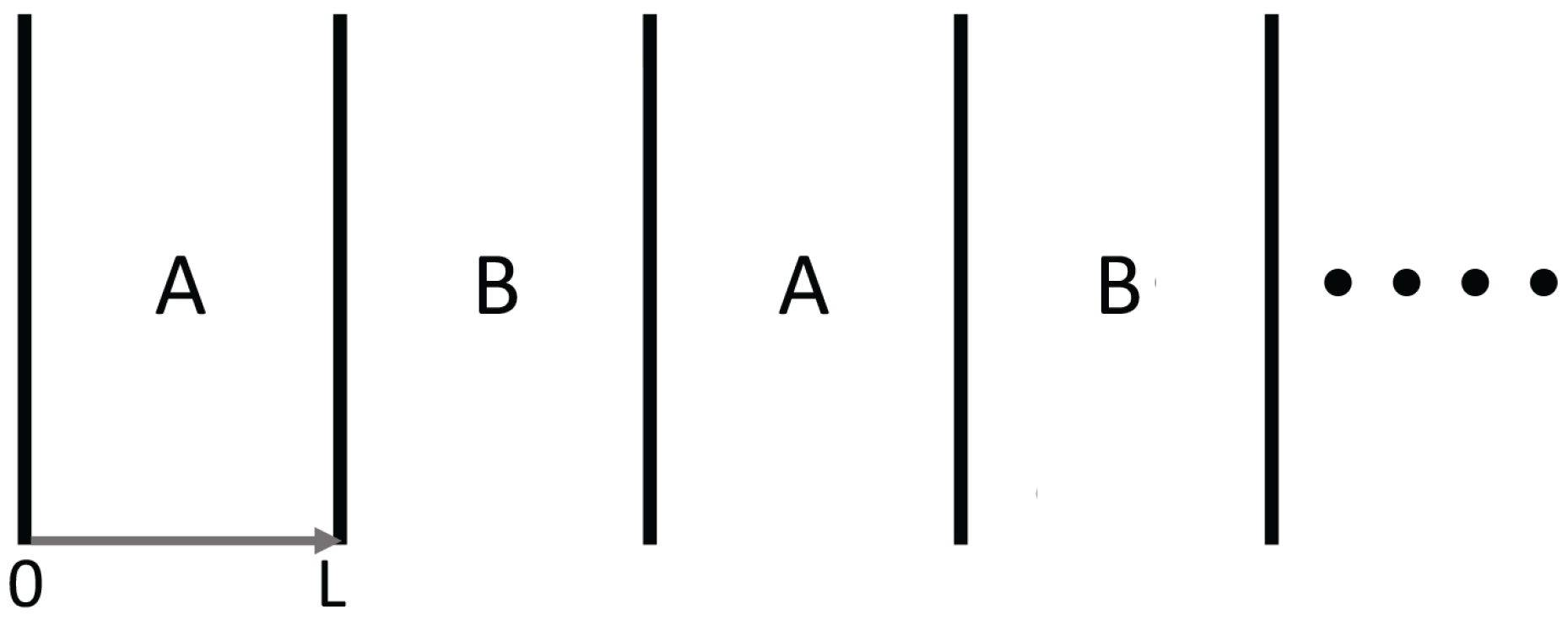
2.2.1. Superlattices
2.2.2. Functionally Graded Materials
2.2.3. Thermal Metamaterials
2.3. Some Engineering Strategies for the Control of Transport Coefficients
2.3.1. Defect Engineering
2.3.2. Dislocation Engineering
2.3.3. Stress Engineering
2.3.4. Phonon Engineering
3. Some Illustrations of New Functional Aims in Heat Transport
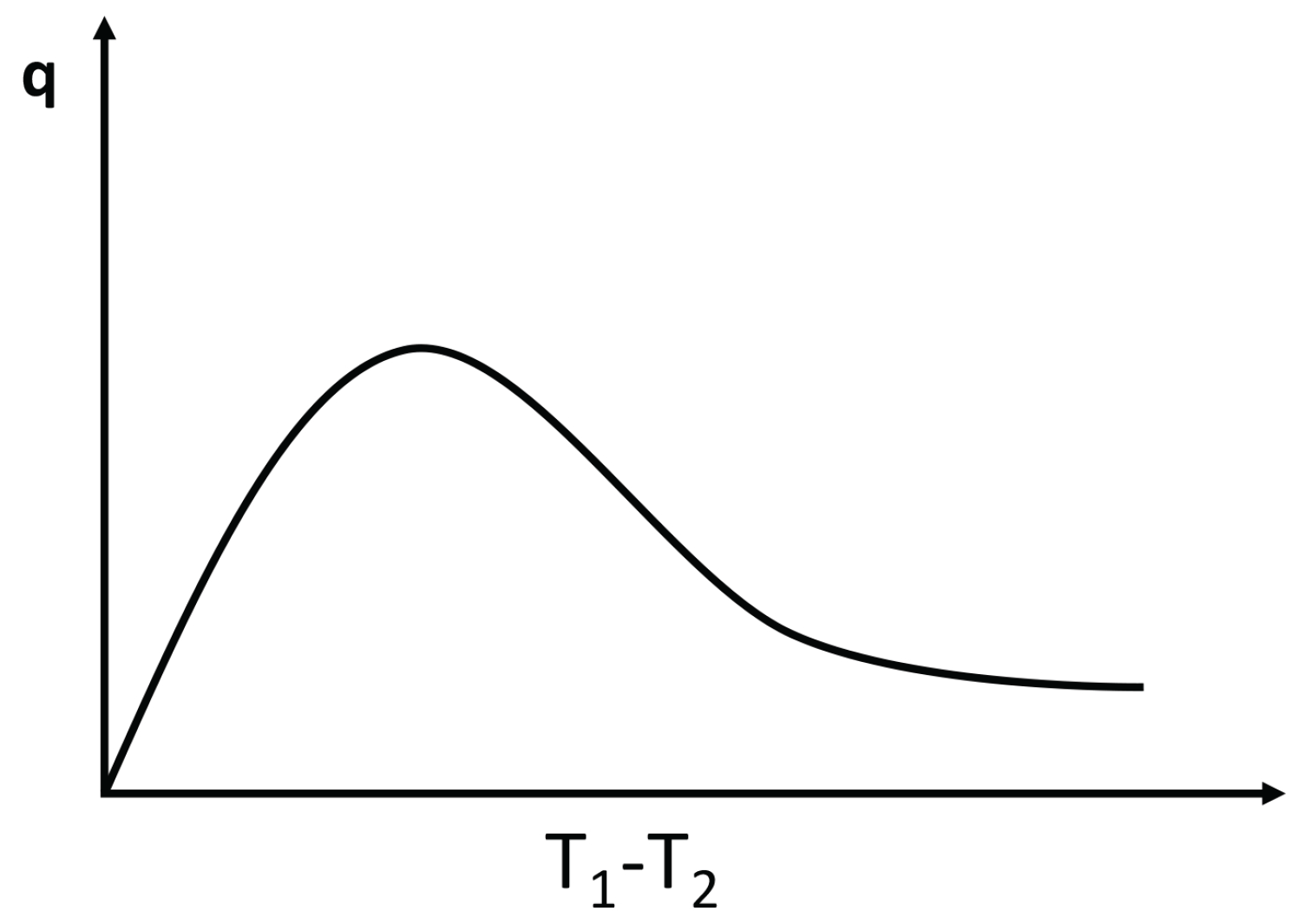
3.1. Heat Rectification and Thermal Diodes
- Stress-Induced Heat Rectification
- Defect-induced heat rectification
3.2. Negative Differential Thermal Conductivity, Thermal Transistors
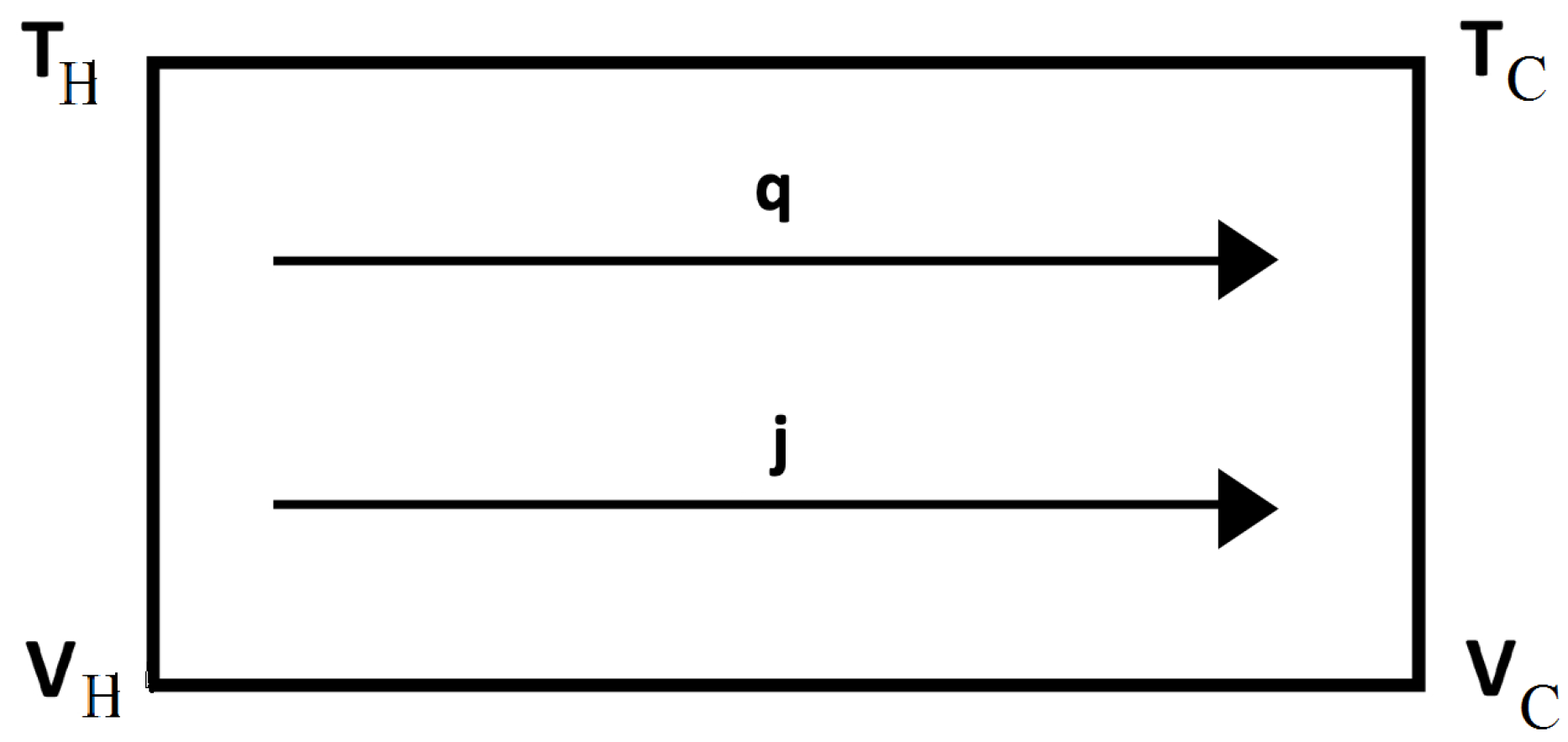
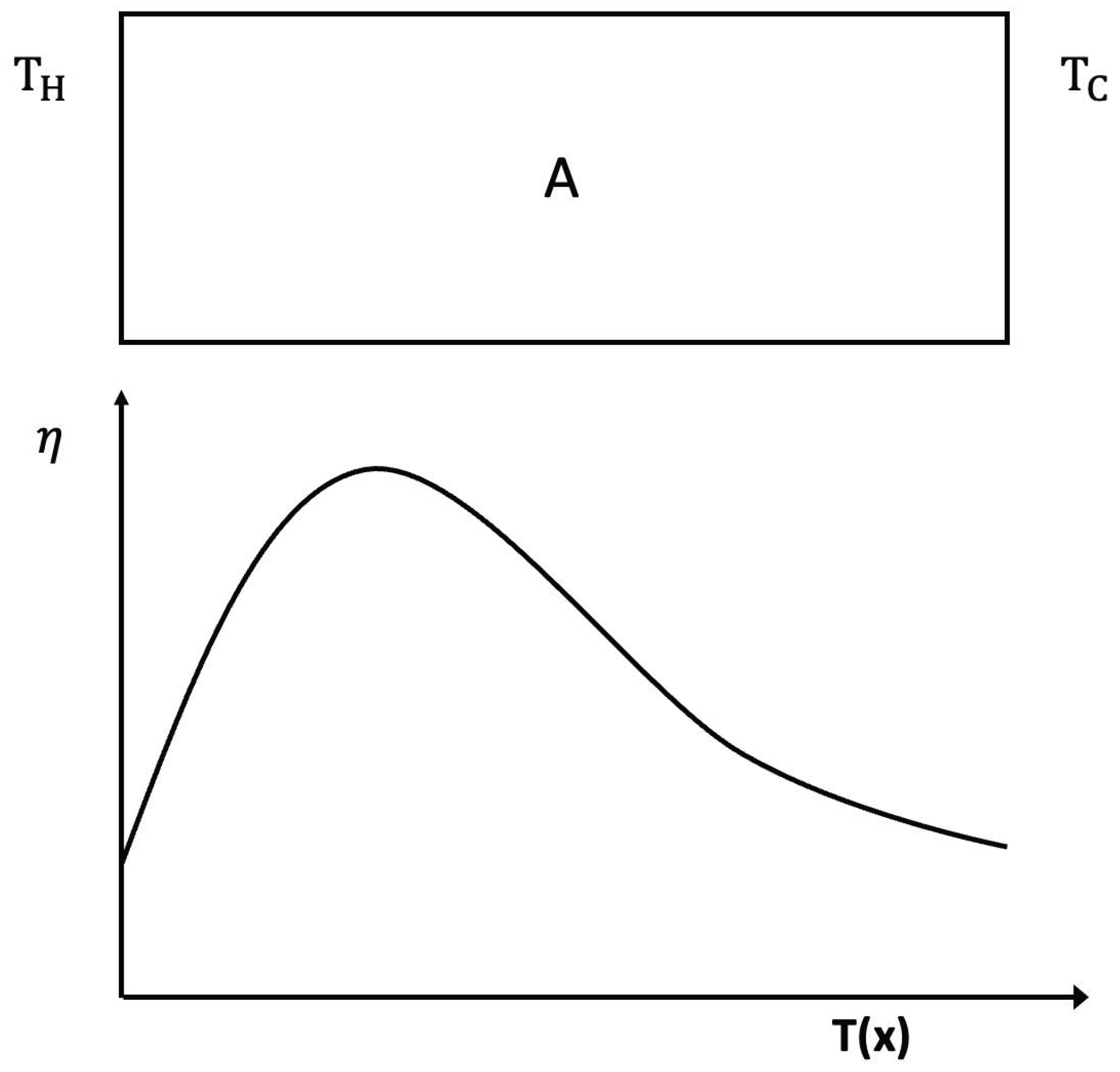
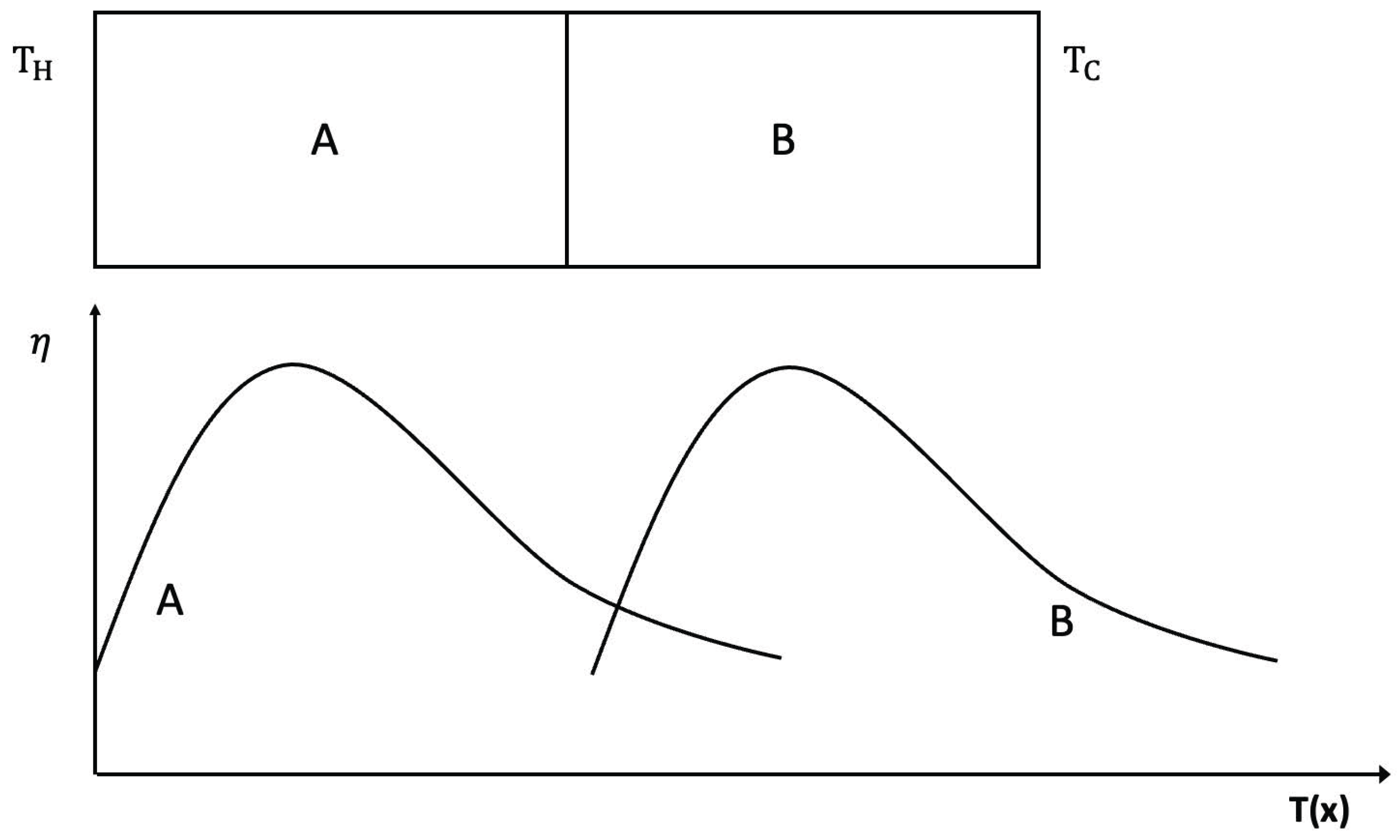
3.3. Efficiency of Thermoelectric Energy Conversion
- A Simplified Illustration
- Thermoelectric Refrigeration
- Effects of Applied Stresses to Enlarge the Optimal Behavior
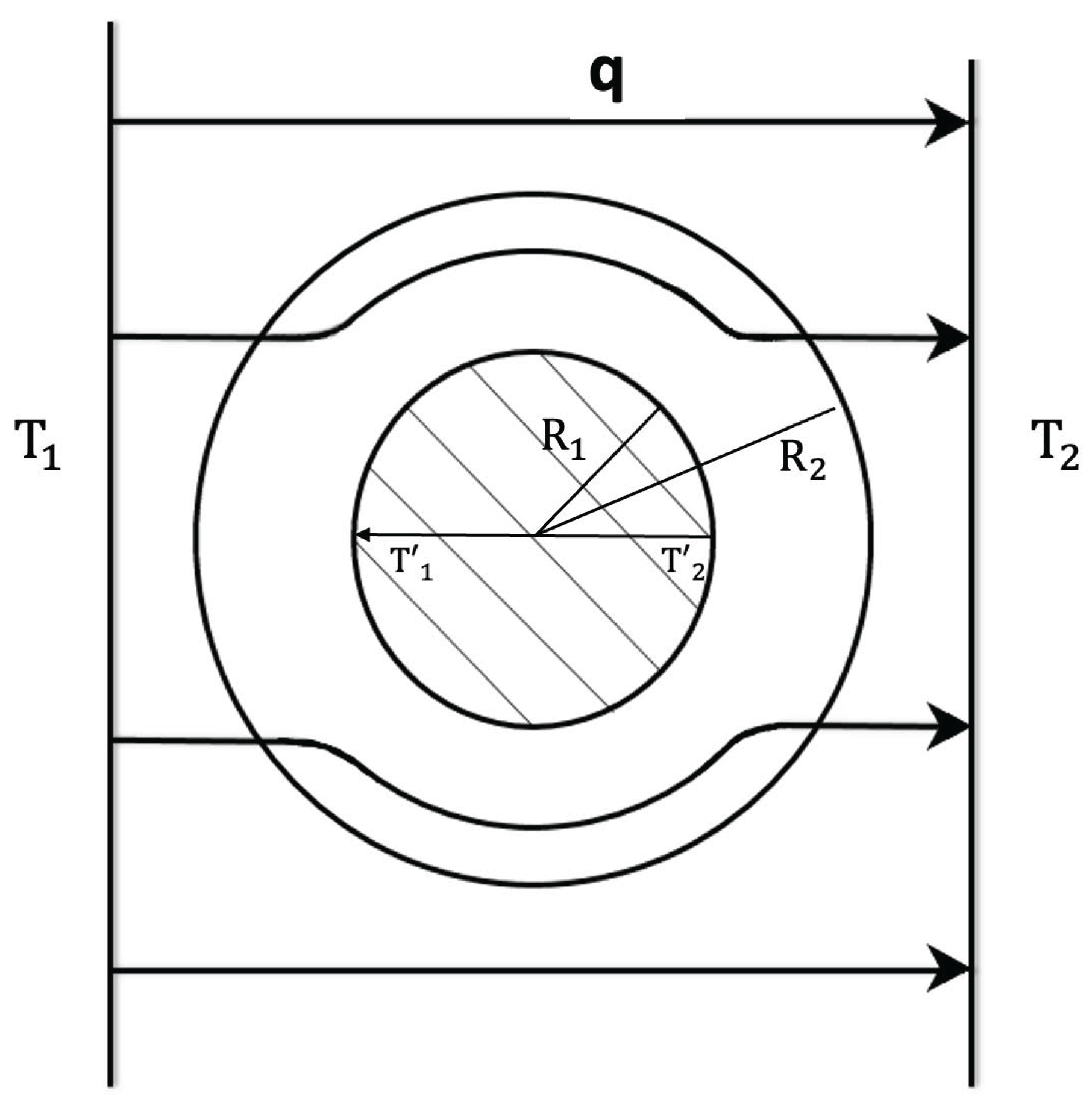
3.4. Thermal Cloak and Thermal Concentration
3.5. Oscillations and Wave Propagation
4. Main Equations
4.1. Selection of Variables
4.2. Governing Equations
4.3. Second-Law Restrictions
5. Constitutive Theory and Rate Equations for Charges, Heat, and Defect Field
5.1. Constitutive Relations: Equations of State
5.2. Rate Equations for the Fluxes and the Defects
5.3. Conditions at the Interfaces
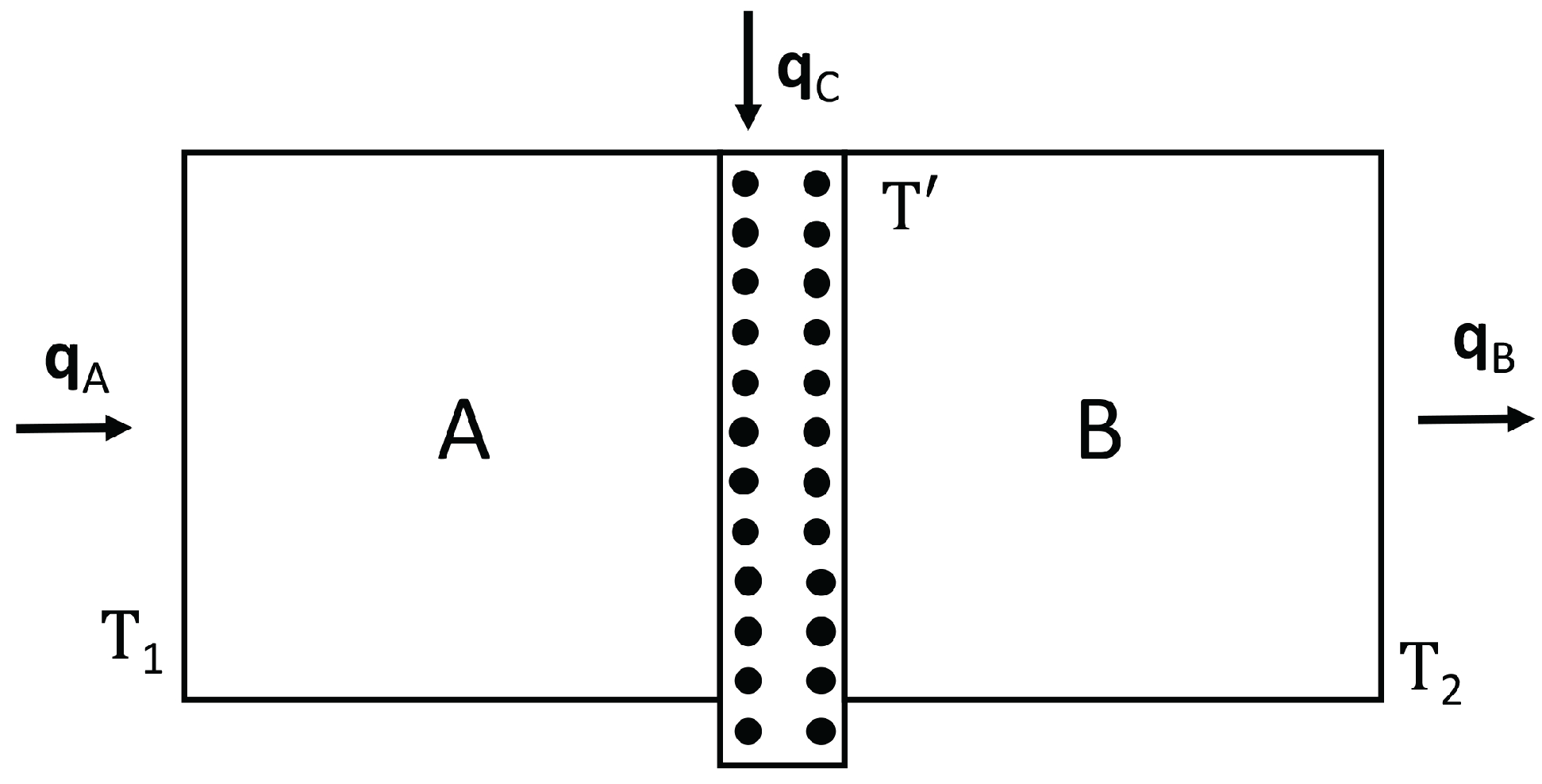
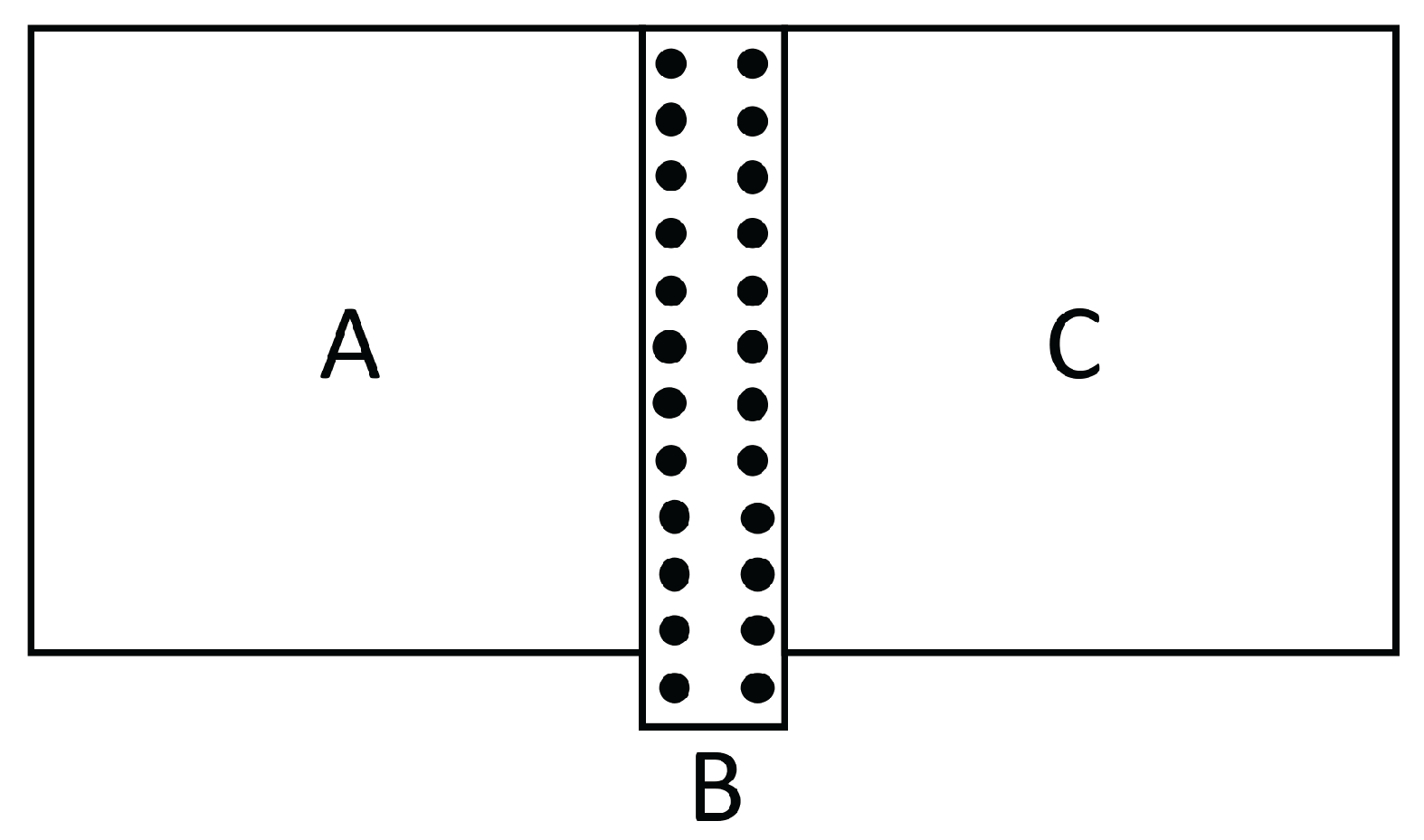
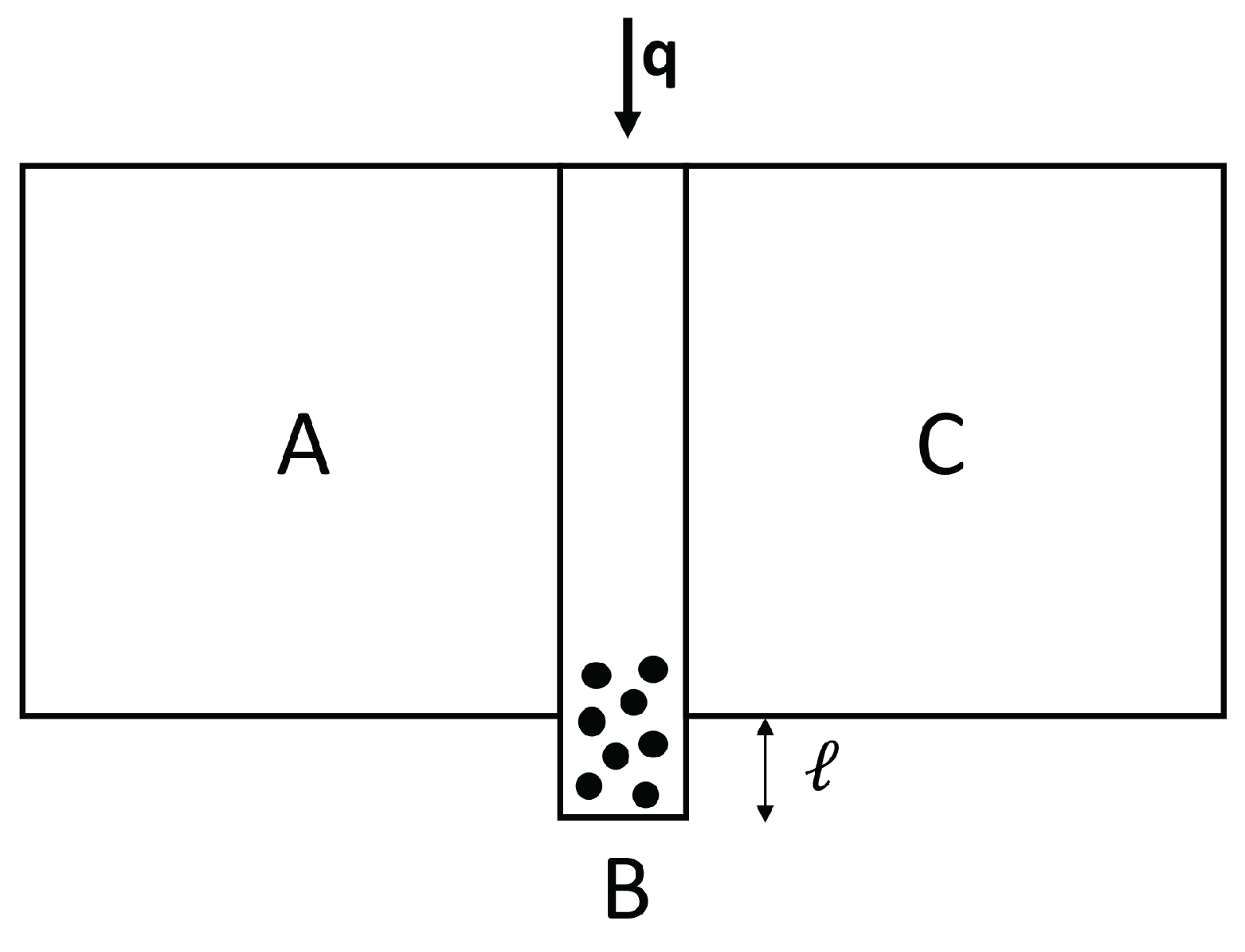
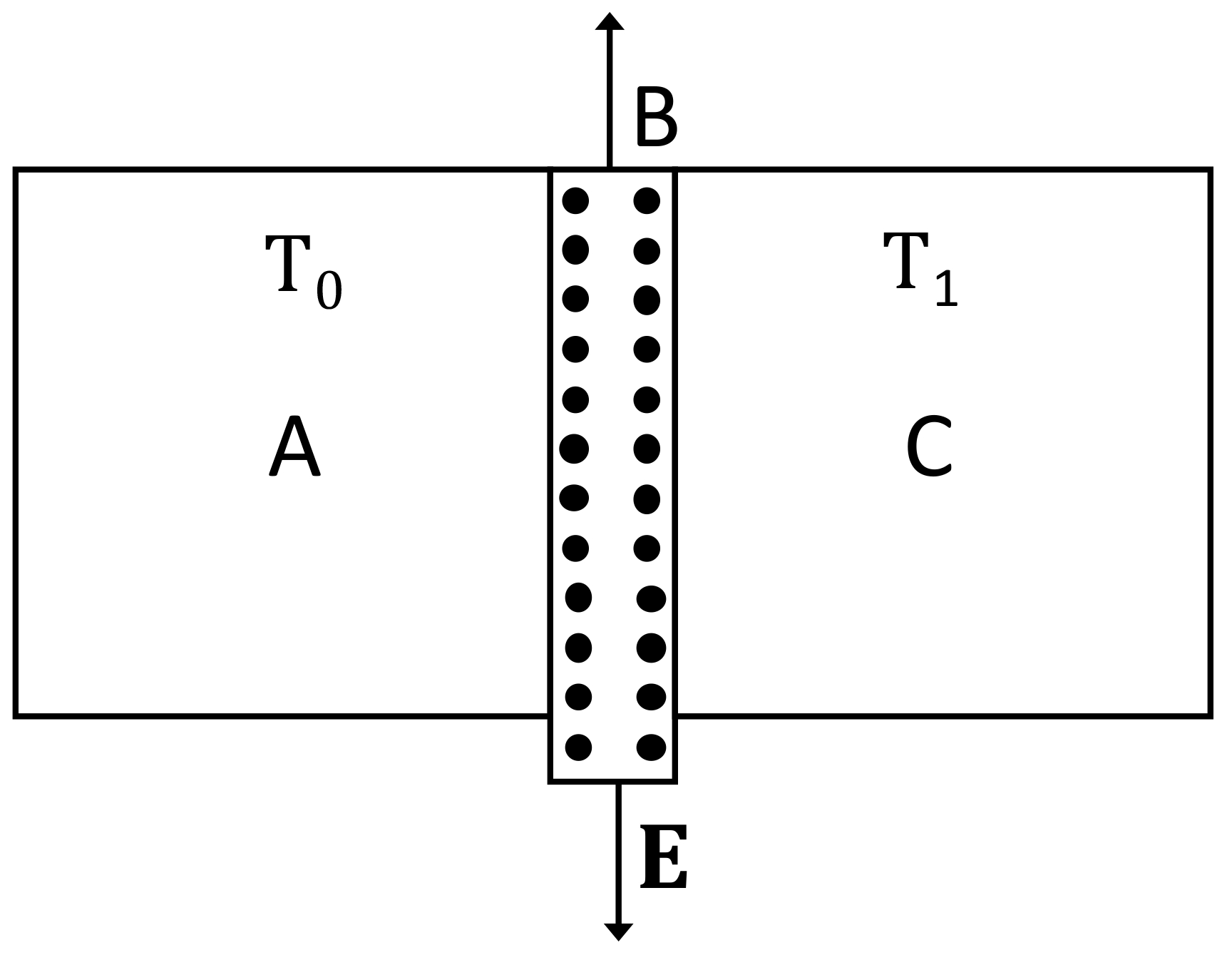
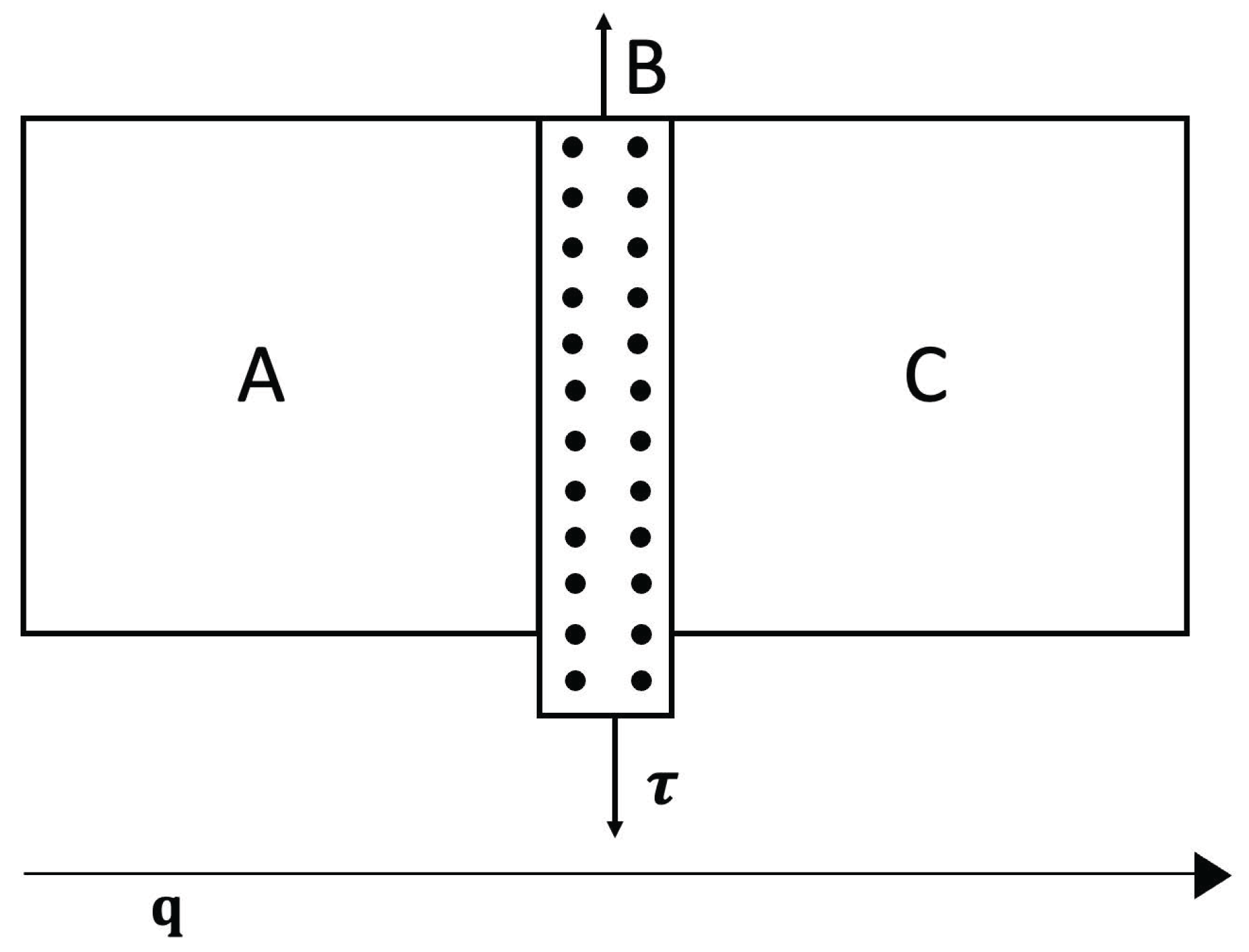
6. Systems with Mobile Defects—Some New Results and Possible Applications
6.1. A Mathematical Model for a Thermal Transistor Based on Mobile Defects
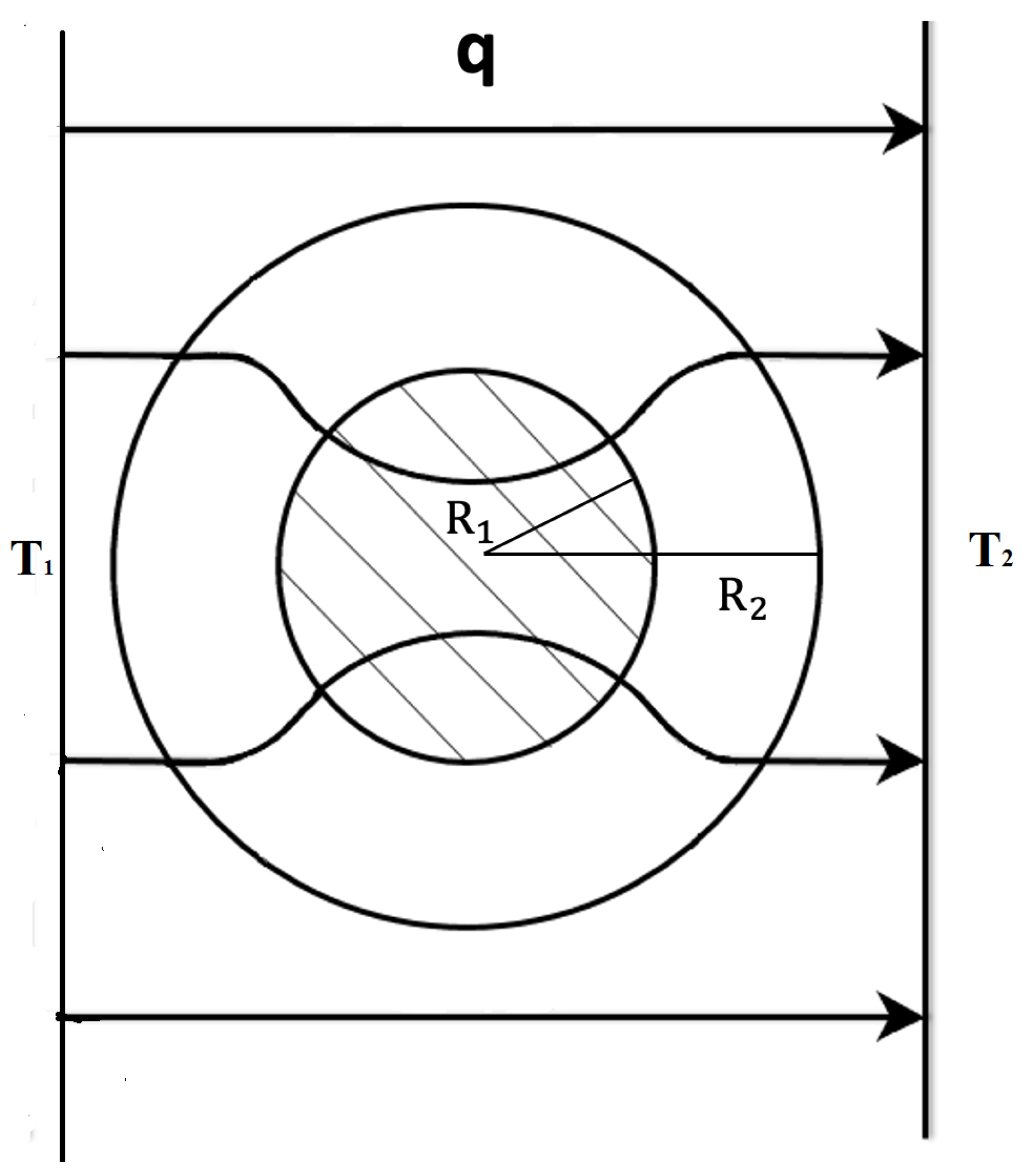
6.2. Steady-State Nonlinear Heat Transport in Superlattices with Mobile Defects
6.3. Charged Mobile Defects: Electrical Production of Heat Waves
6.4. Coupled Defect Waves and Temperature Waves
6.5. Coupled Electronic Waves and Dislocation Waves
6.6. Temperature Waves
7. Summary and Outlook
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Marcinkowski, M.J.; Brown, N.; Fisher, R.M. Dislocation configurations in AuCu3 and AuCu type superlattices. Acta Metall. 2016, 9, 129–137. [Google Scholar] [CrossRef]
- Gowley, P.L.; Drummond, T.J.; Doyle, B.L. Dislocation filtering in semiconductor superlattices with lattice-matched and lattice- mismatched layer materials. Appl. Phys. Lett. 1986, 49, 100. [Google Scholar]
- Blakeslee, A.E. The use of superlattices to block the propagation of dislocations in semiconductors. MRS Online Proc. Libr. 1989, 148, 217. [Google Scholar] [CrossRef]
- Nandwana, D.; Ertkin, E. Ripples, Strain and misfit dislocations: Structure of graphene-boro nitride superlattice interfaces. Nano Lett. 2015, 15, 1468–1475. [Google Scholar] [CrossRef] [PubMed]
- Sugawara, Y.; Ishikawa, Y.; Watanabe, D.; Miyoshi, M.; Egawa, T. Characterization of dislocations in GaN layer grown on 4-inch Si (111) with AlGaN/AlN strained layer superlattices. Jpn. J. Appl. Phys. 2016, 55, 05FB08. [Google Scholar] [CrossRef]
- He, M.; Liu, L.; Tao, H.; Qiu, M.; Zou, B.; Zhang, Z. Threading dislocations in La0.67Sr0.33MnO3/SrTiO3 superlattices. Micro Nano Lett. 2013, 8–9, 512–514. [Google Scholar] [CrossRef]
- Kim, H.-S.; Kang, S.D.; Tang, Y.; Hanus, R.; Snyder, G.J. Dislocation strain as the mechanism of phonon scattering at grain boundaries. Mater. Horiz. 2016, 3, 234–240. [Google Scholar] [CrossRef]
- Klimin, S.N.; Tempere, J.; Lombardi, G.; Devreese, J.T. Finite temperature effective field theory and two-band superfluidity in Fermi gases. Eur. Phys. J. B 2015, 88, 122. [Google Scholar] [CrossRef]
- Chen, G. Thermal conductivity and ballistic-phonon transport in the cross-plane direction of superlattices. Phys. Rev. B 1998, 57, 14958. [Google Scholar] [CrossRef]
- Lin, K.; Strachan, A. Thermal transport in SiGe superlattice thin films and nanowires: Effects of specimen and periodic lengths. Phys. Rev. B 2013, 87, 115302. [Google Scholar] [CrossRef]
- Koh, Y.K.; Cao, Y.; Cahill, D.G. Heat-Transport Mechanisms in Superlattices. Adv. Funct. Mater. 2009, 19, 610. [Google Scholar] [CrossRef]
- Emery, A.F.; Cochran, R.J.; Pepper, D.W. Current trends in heat transfer computations. J. Thermophys. Heat Transf. 1993, 7, 193–212. [Google Scholar] [CrossRef]
- Chen, Z.; Yang, J.; Zhuang, P.; Chen, M.; Zhu, J.; Chen, Y. Thermal conductivity measurement of InGaAs/InGaAsP superlattice thin films. Chin. Sci. Bull. 2006, 51, 2931–2936. [Google Scholar] [CrossRef]
- Chen, Y.; Li, D.; Yang, J.; Wu, Y.; Jennifer, R.; Lukes, J.R.; Majumdar, A. Molecular dynamics study of the lattice thermal conductivity of Kr/Ar superlattice nanowires. Phys. B Condens. Matter 2004, 349, 270–280. [Google Scholar] [CrossRef]
- Chen, Y.; Li, D.; Lukes, J.R.; Ni, Z.; Chen, M. Minimum superlattice thermal conductivity from molecular dynamics. Phys. Rev. B 2005, 72, 174302. [Google Scholar] [CrossRef]
- Savić, I.; Donadio, D.; Gygi, F.; Galli, G. Dimensionality and heat transport in Si-Ge superlattices. Appl. Phys. Lett. 2013, 102, 073113. [Google Scholar] [CrossRef]
- Sutradhar, A.; Paulino, G.H. The simple boundary element method for transient heat conduction in functionally graded materials. Comput. Methods Appl. Mech. Eng. 2004, 193, 4511–4539. [Google Scholar] [CrossRef]
- Burlayenko, V.N.; Altenbach, H.; Sadowski, T.; Dimitrova, S.D.; Bhaskar, A. Modelling functionally graded materials in heat transfer and thermal stress analysis by means of graded finite elements. Appl. Math. Model. 2017, 45, 422. [Google Scholar] [CrossRef]
- Jou, D.; Carlomagno, I.; Cimmelli, V.A. A thermodynamic model for heat transport and thermal wave propagation in graded systems. Phys. E Low-Dimens. Syst. Nanostruct. 2015, 73, 242–249. [Google Scholar] [CrossRef]
- Jou, D.; Carlomagno, I.; Cimmelli, V.A. Rectification of low-frequency thermal waves in graded SSicGe1c. Phys. Lett. A 2016, 380, 1824–1829. [Google Scholar] [CrossRef]
- Carlomagno, I.; Cimmelli, V.A.; Jou, D. Enhanced thermal rectification in graded SicGe1c alloys. Mech. Res. Commun. 2020, 103, 103472. [Google Scholar] [CrossRef]
- Han, D.; Fan, H.; Yan, C.; Wang, T.; Yang, Y.; Ali, S.; Wang, G. Heat conduction and cracking of functionally graded materials using an FDEM-based Thermo-Mechanical coupling model. Appl. Sci. 2022, 12, 12279. [Google Scholar] [CrossRef]
- Tian, J.H.; Jiang, K. Heat conduction investigation of the functionally graded materials plates with variable gradient parameters under exponential heat source load. Int. J. Heat Mass Transf. 2018, 122, 22. [Google Scholar] [CrossRef]
- Wang, F.; Bai, S.; Tress, W.; Hagfeldt, A.; Gao, F. Defects engineering for high-performance perovskite solar cells. NPJ Flex. Electron. 2018, 2, 22. [Google Scholar] [CrossRef]
- Liu, D.P.; Chen, P.J.; Huang, H.H. Realization of a thermal cloak-concentrator using a metamaterial transformer. Sci. Rep. 2018, 8, 2493. [Google Scholar] [CrossRef] [PubMed]
- Chen, F.; Lei, D.Y. Experimental realization of extreme heat flux concentration with easy-to-make thermal metamaterials. Sci. Rep. 2015, 5, 11552. [Google Scholar] [CrossRef]
- Fan, C.; Gao, Y.; Huang, A.J. Shaped graded materials with an apparent negative thermal conductivity. Appl. Phys. Lett. 2008, 92, 251907. [Google Scholar] [CrossRef]
- Guenneau, S.; Amra, C.; Veynante, D. Transformation thermodynamics: Cloaking and concentrating heat flux. Opt. Express 2012, 20, 8207–8218. [Google Scholar] [CrossRef]
- Guenneau, S.; Amra, C. Anisotropic conductivity rotates heat fluxes in transient regimes. Opt. Express 2013, 21, 6578–6583. [Google Scholar] [CrossRef]
- Han, T.; Yuan, T.; Li, B.; Qiu, C.W. Homogeneous thermal cloak with constant conductivity and tunable heat localization. Sci. Rep. 2013, 3, 1593. [Google Scholar] [CrossRef]
- Han, T.; Bai, X.; Gao, D.; Thong, J.T.; Li, B.; Qiu, C.W. Experimental demonstration of a bilayer thermal cloak. Phys. Rev. Lett. 2014, 112, 054302. [Google Scholar] [CrossRef] [PubMed]
- Han, T.; Bai, X.; Liu, D.; Gao, D.; Li, B.; Thong, J.T.; Qiu, C.W. Manipulating steady heat conduction by sensu-shaped thermal metamaterials. Sci. Rep. 2015, 5, 10242. [Google Scholar] [CrossRef] [PubMed]
- Keidar, M.; Shashurin, A.; Delaire, S.; Fang, X.; Beilis, I. Inverse heat flux in double layer thermal metamaterial. J. Phys. D Appl. Phys. 2015, 48, 485104. [Google Scholar] [CrossRef]
- Narayana, S.; Sato, Y. Heat flux manipulation with engineered thermal materials. Phys. Rev. Lett. 2012, 108, 214303. [Google Scholar] [CrossRef]
- Narayana, S.; Savo, S.; Sato, Y. Transient heat flux shielding using thermal metamaterials. Appl. Phys. Lett. 2013, 102, 201904. [Google Scholar] [CrossRef]
- Li, T.H.; Zhu, D.L.; Mao, F.C.; Huang, M.; Yang, J.J.; Li, S.B. Design of diamond-shaped transient thermal cloaks with homogeneous isotropic materials. Front. Phys. 2016, 11, 1–7. [Google Scholar] [CrossRef]
- Yang, T.; Huang, L.; Chen, F.; Xu, W. Heat flux and temperature field cloaks for arbitrarily shaped objects. J. Phys. D Appl. Phys. 2013, 46, 305102. [Google Scholar] [CrossRef]
- Peralta, I.; Fachinotti, V.D.; Ciarbonetti, A. Optimization-based design of a heat flux concentrator. Sci. Rep. 2017, 7, 40591. [Google Scholar] [CrossRef]
- Schittny, R.; Kadic, M.; Guenneau, S.; Wegener, M. Experiments on transformation thermodynamics: Molding the flow of heat. Phys. Rev. Lett. 2013, 110, 195901. [Google Scholar] [CrossRef]
- Sun, L.; Yu, Z.; Huang, J. Design of plate directional heat transmission structure based on layered thermal metamaterials. AIP Adv. 2016, 6, 025101. [Google Scholar] [CrossRef]
- Jou, D.; Restuccia, L. Non-equilibrium thermodynamics framework for dislocations in semiconductor crystals and superlattices. Ann. Acad. Rom. Sci. Ser. Math. Its Appl. 2018, 10, 90–109. [Google Scholar]
- Jou, D.; Restuccia, L. Non-Equilibrium dislocation dynamics in semiconductor crystals and superlattices. J. Non-Equilib. Thermodyn. 2018, 43, 163–170. [Google Scholar] [CrossRef]
- Jou, D.; Restuccia, L. Temperature, heat transport, and dislocations. Atti della Accademia Peloritana dei Pericolanti 2019, 97, A11. [Google Scholar] [CrossRef]
- Jou, D.; Restuccia, L. Nonlinear heat transport in superlattices with mobile defects. Entropy 2019, 21, 1200. [Google Scholar] [CrossRef]
- Jou, D.; Restuccia, L. Non-linear heat transport effects in systems with defects. J. Non-Equilib. Thermodyn. 2022, 47, 179–186. [Google Scholar] [CrossRef]
- Saluto, L.; Restuccia, L.; Jou, D. Electric field dependence of thermal conductivty in bulk systems and nanosystems with charged mobile defects. J. Math Phys. 2022, 63, 063302. [Google Scholar] [CrossRef]
- Restuccia, L. A model for extrinsic semiconductors with dislocations in the framework of non-equilibrium thermodynamics. Atti Accad. Peloritana Pericolanti 2023. to be published. [Google Scholar]
- Chen, H.; McGaughey, A.J.H. Thermal conductivity of carbon nanotubes with defects. In Proceedings of the ASME/JSME 2011 Thermal Engineering Joint Conference, Honolulu, HI, USA, 13–17 March 2011. [Google Scholar] [CrossRef]
- Fiks, V.B. Dragging and deceleration of mobile defects in metals by conduction electrons. Role of electron dispersion law. Sov. Phys. Solid State 1981, 80, 1539–1542. [Google Scholar]
- Granato, A. Thermal properties of mobile defects. Phys. Rev. 1958, 111, 740. [Google Scholar] [CrossRef]
- Li, H.-P.; Zhang, R.Q. Vancancy-defect-induced diminution of thermal conductivity in silicone. EPL Europhys. Lett. 2012, 99, 36001. [Google Scholar] [CrossRef]
- Li, Z.; Xiao, C.; Zhu, H.; Xie, Y. Defect chemistry for thermoelectric materials. J. Am. Chem Soc. 2016, 138, 14810–14819. [Google Scholar] [CrossRef] [PubMed]
- Morosov, A.I.; Sigov, A.S. Electron scattering by mobile defects. J. Phys. Condens. Matter 1991, 3, 2867. [Google Scholar] [CrossRef]
- Ok, K.M.; Ohishi, Y.; Muta, H.; Kurosaki, K.; Yamanaka, S. Effect of point and planar defects on termal conductivity of TiO2-x. J. Am. Ceram. Soc. 2018, 101, 334–346. [Google Scholar] [CrossRef]
- Jou, D.; Casas-Vázquez, J.; Lebon, G. Extended Irreversible Thermodynamics, 4th ed.; Springer: Berlin, German, 2010. [Google Scholar] [CrossRef]
- Jou, D.; Restuccia, L. Mesoscopic transport equations and contemporary thermodynamics: An introduction. Contemp. Phys. 2011, 52, 465–474. [Google Scholar] [CrossRef]
- Volz, S.; Ordonez-Miranda, J.; Shchepetov, A.; Prunnila, M.; Ahopelto, J.; Pezeril, T.; Vaudel, G.; Gusev, V.; Ruello, P.; Weig, E.M.; et al. Nanophononics: State of the art and perspectives. Eur. Phys. J. B 2016, 89, 15. [Google Scholar] [CrossRef]
- Maldovan, M. Sound and heat revolutions in phononics. Nature 2013, 503, 209–217. [Google Scholar] [CrossRef]
- Balandin, A.A. Phononics of graphene and related materials. ACS Nano 2020, 14, 5170–5178. [Google Scholar] [CrossRef]
- Jansen, M.; Tisdale, W.A.; Wood, V. Nanocrystal phononics. Nat. Mater 2023, 22, 161–169. [Google Scholar] [CrossRef]
- Benenti, G.; Donadio, D.; Lepri, S.; Livi, R. Non- Fourier heat transport in nanosystems. La Rivista del Nuovo Cimento 2023, 46, 105–161. [Google Scholar] [CrossRef]
- Alvarez, F.X.; Jou, D. Size and frequency dependence of the effective thermal conductivity in nanosystems. J. Appl. Phys. 2008, 103, 094321. [Google Scholar] [CrossRef]
- Cimmelli, V.A. Different thermodynamic theories and different heat conduction laws. J. Non-Equilib. Thermodyn. 2009, 34, 299–333. [Google Scholar] [CrossRef]
- Lebon, G. Heat conduction at micro and nanoscales: A review through the prism of extended irreversible thermodynamics. J. Non-Equilib. Thermodyn. 2014, 39, 35–59. [Google Scholar] [CrossRef]
- Guo, Y.; Wang, M. Phonon hydrodynamics and its applications in nanoscale heat transport. Phys. Rep. 2015, 595, 1–44. [Google Scholar] [CrossRef]
- Sellitto, A.; Cimmelli, V.; Jou, D. Mesoscopic Theories of Heat Transport on Nanosystems; SEMA-SIMAI Springer Series; Springer: Berlin, Germeny, 2016. [Google Scholar]
- Machrafi, H. Extended Non-Equilibrium Thermodynamics. From Principles to Applications in Nanosystems; CRC Press: Boca Raton, FL, USA, 2019. [Google Scholar]
- Liao, B. (Ed.) Nanoscale Energy Transport. Emerging Phenomena, Methods and Applications; IOP Publishing: Bristol, UK, 2020. [Google Scholar]
- Chen, G. Non-Fourier phonon heat conduction at the microscale and nanoscale. Nat. Rev. Phys. 2021, 3, 555–569. [Google Scholar] [CrossRef]
- Muschik, W.; Restuccia, L. Terminology and classification of special versions of continuum thermodynamics. Commun. SIMAI Congr. 2006, 1, 1–4. [Google Scholar] [CrossRef]
- Muschik, W. Aspects of Non-Equilibrium Thermodynamics; World Scientific: Singapore, 1990. [Google Scholar]
- Maugin, G. The Thermodynamics of Non-Linear Irreversible Behaviour: An Introduction; World Scientific: Singapore, 1999. [Google Scholar]
- Mu¨ller, I. Thermodynamics; Pitman Advanced Publishing Program: Boston, MA, USA, 1985. [Google Scholar]
- Muschik, W. Fundamentals of Non-Equilibrium Thermodynamics. In Non-Equilibrium Thermodynamics with Applications to Solids; Muschik, W., Ed.; Springer: Wien, NY, USA, 1993; Volume 336, pp. 1–63. [Google Scholar] [CrossRef]
- Berezovski, A.; Van, P. Internal Variables in Thermoelasticity; Springer: Berlin, Germany, 2017. [Google Scholar]
- Restuccia, L.; Maruszewski, B. Interactions between electronic field and dislocations in a deformable semiconductor. Int. J. Appl. Electromagn. Mech. 1995, 6, 139. [Google Scholar]
- Sellan, D.P.; Landry, E.S.; Turney, J.E.; McGaughey, A.J.; Amon, C.H. Size effects in molecular dynamics thermal conductivity predictions. Phys. Rev. B 2010, 81, 214305. [Google Scholar] [CrossRef]
- Lorenzi, B.; Dettori, R.; Dunham, M.T.; Melis, C.; Tonini, R.; Colombo, L.; Sood, A.; Goodson, K.E.; Narducci, D. Phonon scattering in Silicon by multiple morphological defects: A multiscale analysis. J. Electron. Mater. 2018, 47, 9. [Google Scholar] [CrossRef]
- Chen, J.; Xu, X.; Zhou, J.; Li, B. Interfacial thermal resistance: Past, present, and future. Rev. Mod. Phys. 2022, 94, 025002. [Google Scholar] [CrossRef]
- Anno, Y.; Imakita, Y.; Takei, K.; Akita, S.; Arie, T. Enhancement of graphene thermoelectric performance through defect engineering. 2D Mater. 2017, 4, 025019. [Google Scholar] [CrossRef]
- Arifutzzaman, A.; Khan, A.A.; Ismail, A.F.; Alam, M.Z.; Yaacob, I.I. Effect of exfoliated graphene defects on thermal conductivity of water-based graphene nanofluids. Int. J. Appl. Eng. Res. 2018, 13, 4871–4877. [Google Scholar]
- Park, J.S.; Kim, S.; Xie, Z.; Walsh, A. Point defect engineering in thin-film solar cells. Nat. Rev. Mater. 2018, 3, 194–221. [Google Scholar] [CrossRef]
- Ramesh, R. Defect engineering using crystal symmetry. Proc. Natl. Acad. Sci. USA 2018, 115, 9344–9346. [Google Scholar] [CrossRef] [PubMed]
- Scott, E.A.; Hattar, K.; Rost, C.M.; Gaskins, J.T.; Fazli, M.; Ganski, C.; Li, C.; Bai, T.; Wang, Y.; Esfarjani, K.; et al. Phonon scattering effects from point and extended defects of thermal conductivity studied via ion irradiation of crystals with self-impurities. Phys. Rev. Mater. 2018, 2, 095001. [Google Scholar] [CrossRef]
- Wight, N.; Bennet, N. Reduced thermal conductivity in silicon thin fils via vacancies. Solid State Phenom. 2015, 242, 344–349. [Google Scholar] [CrossRef]
- Zhang, N.; Gao, C.; Xiong, Y. Defect engineering: A versatile tool for tuning the activation of key molecules in photocatalytic reactions. J. Energy Chem. 2019, 37, 43–57. [Google Scholar] [CrossRef]
- Zheng, Y.; Slade, T.J.; Hu, L.; Tan, X.Y.; Luo, Y.; Luo, Z.Z.; Xu, J.; Yan, Q.; Kanatzidis, M.G. Defect engineering in thermoelectric materials: What have we learned? Chem. Soc. Rev. 2021, 50, 9022–9054. [Google Scholar] [CrossRef]
- Hu, L.; Zhu, T.; Liu, X.; Zhao, X. Point Defect Engineering of High-Performance Bismuth-Telluride-Based Thermoelectric Materials. Adv. Funct. Mater. 2014, 24, 5211–5218. [Google Scholar] [CrossRef]
- Zhao, M.; Pan, W.; Wan, C.; Qu, Z.; Li, Z.; Yang, J. Defect engineering in development of low thermal conducitivy materials: A review. J. Eur. Ceram. Soc. 2017, 37, 1–13. [Google Scholar] [CrossRef]
- Zhao, W.; Wang, Y.; Wu, Z.; Wang, W.; Bi, K.; Liang, Z.; Yang, J.; Chen, Y.; Xu, Z.; Ni, Z. Defect-engineered heat transport in Graphene: A route to high efficient thermal rectification. Sci. Rep. 2015, 5, 11962. [Google Scholar] [CrossRef]
- Zhao, Y.; Liu, D.; Chen, J.; Zhu, L.; Belianinov, A.; Ouchinnikouq, O.S.; Unocic, R.R.; Thong, J.T.L. Engineering the thermal conductivity along an individual silicon nanowire by selective helium ion irradiation. Nat. Commun. 2017, 8, 15919. [Google Scholar] [CrossRef]
- Lanzillo, N.A.; Thomas, J.B.; Watson, B.; Nayak, S.K. Pressure-enabled phonon engineering in metals. Proc. Natl. Acad. Sci. USA 2014, 111, 8712–8716. [Google Scholar] [CrossRef]
- Bhowmick, S.; Shenoy, V.B. Effect of strain on the thermal conductivity of solids. J. Chem. Phys. 2006, 125, 164513. [Google Scholar] [CrossRef] [PubMed]
- Li, X.; Maute, K.; Dunn, M.L.; Yang, R. Strain effects on the thermal conductivity of nanostructures. Phys. Rev. B. 2010, 81, 245318. [Google Scholar] [CrossRef]
- Wei, N.; Xu, L.; Wang, H.Q.; Zheng, J.C. Strain engineering of thermal conductivity in graphene sheets and nanoribbons: A demonstration of magic flexibility. Nanotechnology 2011, 22, 105705. [Google Scholar] [CrossRef] [PubMed]
- Alam, M.T.; Manoharan, M.; Haque, A.; Muratore, C.; Voevodin, A.A. Influence of strain on thermal conductivity of silicon nitride thin films. J. Micromech. Microeng. 2012, 22, 045001. [Google Scholar] [CrossRef]
- Fan, D.; Sigg, H.; Spolenak, R.; Ekinci, Y. Strain and thermal conductivity in ultrathin suspended silicon nanowires. Phys. Rev. B 2017, 96, 115307. [Google Scholar] [CrossRef]
- Vakulov, D.; Gireesan, S.; Swinkels, M.Y.; Chavez, R.; Vogelaar, T.; Torres, P.; Campo, A.; De Luca, M.; Verheijen, M.A.; Koelling, S.; et al. Ballistic phonons in ultrathin nanowires. Nano Lett. 2020, 20, 2703–2709. [Google Scholar] [CrossRef]
- Nomura, M.; Shiomi, J.; Shiga, T.; Anufriev, R. Thermal phonon engineering by tailored nanostructures. Jpn. J. Appl. Phys. 2018, 57, 080101. [Google Scholar] [CrossRef]
- Toberer, E.S.; Zevalnika, A.; Snyder, G.J. Phonon engineering through crystal chemistry. J. Mater. Chem. 2011, 21, 15843. [Google Scholar] [CrossRef]
- Ortíz, O.; Esmann, M.; Lanzillotti-Kimura, N.D. Phonon engineering with superlattices: Generalized nanomechanical potentials. Phys. Rev. B 2019, 100, 085430. [Google Scholar] [CrossRef]
- Xiang, H.; Zhou, Y. Phonon engineering in tuning the thermal conductivity of alkaline-earth hexaborides. J. Eur. Ceram. Soc. 2020, 40, 1352–1360. [Google Scholar] [CrossRef]
- Pirc, R.; Blinc, R. Vogel-Fulcher freezing in relaxor ferroelectrics. Phys. Rev. B 2007, 76, 020101(R). [Google Scholar] [CrossRef]
- Dettori, R.; Melis, C.; Rurali, R. Thermal rectification in silicon by a graded distribution of defects. J. Appl. Phys. 2016, 119, 215102. [Google Scholar] [CrossRef]
- Hu, M.; Keblinski, P.; Baowen Li, B. Thermal rectification at silicon-amorphous polyethylene interface. Appl. Phys. Lett. 2008, 92, 211908. [Google Scholar] [CrossRef]
- Rurali, R.; Cartoixá, X.; Colombo, L. Heat transport across a SiGe nanowire axial junction: Interface thermal resistance and thermal rectification. Phys. Rev. B 2014, 90, 041408(R). [Google Scholar] [CrossRef]
- Cartoixá, X.; Colombo, L.; Rurali, R. Thermal rectification by design in telescopic Si nanowires. Nano Lett. 2015, 15, 8255–8259. [Google Scholar] [CrossRef] [PubMed]
- Chang, C.W.; Okawa, D.; Majumdar, A.; Zettl, A. Solid-state thermal rectifier. Science 2006, 314, 1121–1124. [Google Scholar] [CrossRef]
- Wu, G.; Li, B. Thermal rectifiers from deformed carbon nanohorns. J. Phys. Condens. Matter 2008, 20, 175211. [Google Scholar] [CrossRef]
- Yang, N.; Zhang, G.; Li, B. Carbon nanocone: A promising thermal rectifier. Appl. Phys. Lett. 2008, 93, 243111. [Google Scholar] [CrossRef]
- Hu, J.; Ruan, X.; Chen, Y.P. Thermal conductivity and thermal rectification in graphene nanoribbons: A molecular dynamics study. Nano Lett. 2009, 9, 2730–2735. [Google Scholar] [CrossRef]
- Tian, H.; Xie, D.; Yang, Y.; Ren, T.L.; Zhang, G.; Wang, Y.F. A novel solid-state thermal rectifier based on reduced graphene oxide. Sci. Rep. 2012, 2, 523. [Google Scholar] [CrossRef]
- Sandonas, L.M.; Gutierrez, R.; Arezoo Dianat, A.; Cuniberti, G. Engineering thermal rectification in MoS2 nanoribbons: A non-equilibrium molecular dynamics study. RRSC Adv. 2015, 5, 54345–54351. [Google Scholar] [CrossRef]
- Melis, C.; Barbarino, G.; Colombo, L. Exploiting hydrogenation for thermal rectification in graphene nanoribbons. Phys. Rev. B 2015, 92, 245408. [Google Scholar] [CrossRef]
- Wang, Y.; Chen, S.; Ruan, X. Tunable thermal rectification in graphene nanoribbons through defect engineering: A molecular dynamics study. Appl. Phys. Lett. 2012, 100, 163101. [Google Scholar] [CrossRef]
- Peyrard, M. The design of a thermal rectifier. Europhys. Lett. 2006, 76, 49. [Google Scholar] [CrossRef]
- Gunawardana, K.G.S.H.; Mullen, K.; Hu, J.; Chen, Y.P.; Ruan, X. Tunable thermal transport and thermal rectification in strained graphene nanoribbons. Phys. Rev. B 2012, 85, 245417. [Google Scholar] [CrossRef]
- Carlomagno, I.; Cimmelli, V.A.; Jou, D. Gradient-dependent heat rectification in thermoelastic solids. J. Therm. Stress. 2021, 44, 919–934. [Google Scholar] [CrossRef]
- Zhao, J.; We, D.; Gao, A.; Dong, H.; Bao, Y.; Jiang, Y.; Liu, D. Thermal rectification enhancement of bi-segment thermal rectifier based on stress induced interface thermal contact resistance. Appl. Therm. Eng. 2020, 176, 115410. [Google Scholar] [CrossRef]
- Zhao, J.; Wei, D.; Dong, Y.; Zhang, D.; Liu, D. Thermal rectification mechanism of composite cylinders with temperature and stress-dependent interface thermal resistance. Int. J. Heat Mass Transf. 2022, 194, 123024. [Google Scholar] [CrossRef]
- Malik, F.K.; Fobelets, K. A review of thermal rectification in solid-state devices. J. Semicond. 2022, 43, 103101. [Google Scholar] [CrossRef]
- Machrafi, H.; Lebon, G.; Jou, D. Thermal rectifier efficiency of various bulk—Nanoporous silicon devices. Int. J. Heat Mass Transf. 2016, 97, 603–610. [Google Scholar] [CrossRef]
- Go, D.B.; Crossmark, M.S. On the Condition for Thermal Rectification Using Bulk Materials. J. Heat Transf. 2010, 132, 124502. [Google Scholar] [CrossRef]
- Alidoust, N.; Lessio, M.; Emily, A. Carter, E.A. Cobalt (II) oxide and nickel (II) oxide alloys as potential intermediate-band semiconductors: A theoretical study. J. Appl. Phys. 2016, 119, 025102. [Google Scholar] [CrossRef]
- Hayashi, H.; Ito, Y.; Takahashi, K. Thermal rectification of asymmetrically-defective materials. J. Mech. Sci. Technol. 2011, 25, 27–32. [Google Scholar] [CrossRef]
- Hayashi, H.; Takahashi, K. Defect-Induced Thermal Rectification: Numerical Study on Carbon Nanotube and FPU-Beta Lattice. In Proceedings of the ASME/JSME 2011 8th Thermal Engineering Joint Conference, Honolulu, HI, USA, 13–17 March 2011. [Google Scholar] [CrossRef]
- Wang, H.; Hu, S.; Takahashi, K.; Zhang, X.; Takamatsu, H.; Chen, J. Experimental study of thermal rectification in suspended monolayer graphene. Nat. Commun. 2017, 8, 15843. [Google Scholar] [CrossRef]
- Gandomi, Y.A.; Wang, J.; Bowman, M.H.; Marable, D.C.; Kim, D.; Shin, S. Thermal rectification via asymmetric structural defects in graphene. Carbon 2018, 132, 565–572. [Google Scholar] [CrossRef]
- Carlomagno, I.; Cimmelli, V.A.; Jou, D. Tunable heat rectification by applied mechanical stress. Phys. Lett. A 2020, 384, 126905. [Google Scholar] [CrossRef]
- Li, B.; Wang, L.; Casati, G. Negative differential thermal resistance and thermal transistor. Appl. Phys. Lett. 2006, 88, 143501. [Google Scholar] [CrossRef]
- Wang, L.; Li, B. Heat switch and modulator: A model of thermal transistor. Int. J. Mod. Phys. B 2007, 21, 4017–4020. [Google Scholar] [CrossRef]
- Shafranjuk, S.E. Graphene thermal flux transistor. Nanoscale 2016, 8, 19314–19325. [Google Scholar] [CrossRef] [PubMed]
- Sood, A.; Xiong, F.; Chen, S.; Wang, H.; Selli, D.; Zhang, J.; McClellan, C.J.; Sun, J.; Donadio, D.; Cui, Y.; et al. An electrochemical thermal transistor. Nat. Commun. 2018, 9, 4510. [Google Scholar] [CrossRef] [PubMed]
- Ghosh, R.; Ghoshal, A.; Sen, U. Quantum thermal transistors: Operation characteristics in steady state versus transient regimes. Phys. Rev. A 2021, 103, 052613. [Google Scholar] [CrossRef]
- Gupt, N.; Bhattacharyya, S.; Das, B.; Datta, S.; Mukherjee, V.; Ghosh, A. Floquet quantum thermal transistor. Phys. Rev. E 2022, 106, 024110. [Google Scholar] [CrossRef]
- Castelli, L.; Zhu, Q.; Shimokusu, T.J.; Wehmeyer, G. A three-terminal magnetic thermal transistor. Nat. Commun. 2023, 14, 393. [Google Scholar] [CrossRef]
- Yang, Q.; Cho, H.J.; Bian, Z.; Yoshimura, M.; Lee, J.; Jeen, H.; Lin, J.; Wei, J.; Feng, B.; Ikuhara, Y.; et al. Solid-State Electrochemical Thermal Transistors. Adv. Funct. Mater. 2023, 33, 2214939. [Google Scholar] [CrossRef]
- Benenti, G.; Casati, G.; Mejía-Monasterio, C.; Peyrard, M. From thermal rectifiers to thermoelectric devices. In Thermal Transport in Low Dimensions: From Statistical Physics to Nanoscale Heat Transfer; Lecture Notes in Physics; Springer: Berlin, Germany, 2016; Volume 921, pp. 365–407. [Google Scholar]
- Nishio, Y.; Hirano, T. Improvement of the efficiency of thermoelectric energy conversion by utilizing potential barriers. Jpn. J. Appl. Phys. 1997, 36, 170. [Google Scholar] [CrossRef]
- Chen, G. Thermoelectric energy conversion: Materials, devices, and systems. J. Phys. Conf. Ser. 2015, 660, 012066. [Google Scholar] [CrossRef]
- Kim, H.S.; Liu, W.; Chen, G.; Ren, Z. Relationship between thermoelectric figure of merit and energy conversion efficiency. Proc. Natl. Acad. Sci. USA 2015, 112, 8205–8210. [Google Scholar] [CrossRef]
- Bu, Z.; Zhang, X.; Hu, Y.; Chen, Z.; Lin, S.; Li, W.; Xiao, C.; Pei, Y. A record thermoelectric efficiency in tellurium-free modules for low-grade waste heat recovery. Nat. Commun. 2022, 13, 237. [Google Scholar] [CrossRef]
- Ishida, A. Formula for energy conversion efficiency of thermoelectric generator taking temperature dependent thermoelectric parameters into account. J. Appl. Phys. 2020, 128, 135105. [Google Scholar] [CrossRef]
- Zhou, C.; Lee, Y.K.; Yu, Y.; Byun, S.; Luo, Z.Z.; Lee, H.; Ge, B.; Lee, Y.L.; Chen, X.; Lee, J.Y.; et al. Polycrystalline SnSe with a thermoelectric figure of merit greater than the single crystal. Nat. Mater. 2021, 20, 1378–1384. [Google Scholar] [CrossRef]
- Zabrocki, K.; Müller, E.; Seifert, W.; Trimper, S. Performance optimization of a thermoelectric generator element with linear, spatial material profiles in a one-dimensional setup. J. Mater. Res. 2011, 26, 1963–1974. [Google Scholar] [CrossRef]
- Rogolino, P.; Cimmelli, V.A. Thermoelectric efficiency of graded SicGe1–c alloys. J. Appl. Phys. 2018, 124, 094301. [Google Scholar] [CrossRef]
- Cimmelli, V.A.; Rogolino, P. New and recent results for thermoelectric energy conversion in graded alloys at nanoscale. Nanomaterials 2022, 12, 2378. [Google Scholar] [CrossRef]
- Cramer, C.L.; Wang, H.; Ma, K. Performance of functionally graded thermoelectric materials and devices: A review. J. Electron. Mater. 2018, 47, 5122–5132. [Google Scholar] [CrossRef]
- Jin, Z.H.; Wallace, T.T.; Lad, R.J.; Su, J. Energy conversion efficiency of an exponentially graded thermoelectric material. J. Electron. Mater. 2014, 43, 308–313. [Google Scholar] [CrossRef]
- Onsager, L. Reciprocal relations of irreversible processes I. Phys. Rev. 1931, 37, 405–426. [Google Scholar] [CrossRef]
- Onsager, L. Reciprocal relations of irreversible processes II. Phys. Rev. 1931, 38, 2265–2279. [Google Scholar] [CrossRef]
- Prigogine, I. Introduction to Thermodynamics of Irreversible Processes; Interscience Publishers: New York, NY, USA; John Wiley and Sons: London, UK, 1961. [Google Scholar]
- De Groot, S.R.; Mazur, P. Non-Equilibrium Thermodynamics; North-Holland Publishing Company: Amsterdam; Interscience Publishers Inc.: New York, NY, USA, 1962. [Google Scholar]
- Joseph, D.D.; Preziosi, L. Heat waves. Rev. Mod. Phys. 1989, 61, 41. [Google Scholar] [CrossRef]
- Müller, I.; Ruggeri, T. Rational Extended Thermodynamics; Springer: New York, NY, USA, 1985. [Google Scholar]
- Busse, G. Thermal Waves for Material Inspection. In Physical Acoustics; Leroy, O., Breazeale, M.A., Eds.; Springer: Boston, MA, USA, 1991. [Google Scholar] [CrossRef]
- Straughan, B. Heat Waves; Springer: Berlin, Germany, 2011. [Google Scholar]
- Marín, E. Thermal Wave Physics and Related Photothermal Techniques: Basic Principles and Recent Developments; Marín, E., Ed.; Transworld Research Network: Kerala, India, 2009. [Google Scholar]
- Beardo, A.; López–Suárez, M.; Pérez, L.A.; Sendra, L.; Alonso, M.I.; Melis, C.; Bafaluy, J.; Camacho, J.; Colombo, L.; Rurali, R.; et al. Observation of second sound in a rapidly varying temperature field in Ge. Sci. Adv. 2021, 7, eabg4677. [Google Scholar] [CrossRef] [PubMed]
- Nabarro, F.R.N. Theory of Crystal Dislocations; Clarendon Press: Oxford, UK, 1967. [Google Scholar]
- Mataré, H.F. Defects Electronics in Semiconductors; Wiley–Interscience: New York, NY, USA, 1971. [Google Scholar]
- Mazzeo, M.P.; Restuccia, L. On the heat equation for n-type semiconductors defective by dislocations. Commun. SIMAI Congr. 2009, 3, 308. [Google Scholar] [CrossRef]
- Mazzeo, M.P.; Restuccia, L. Material element model for extrinsic semiconductors with defects of dislocations. Ann. Acad. Rom. Sci. Ser. Math. Its Appl. 2011, 3, 188–206. [Google Scholar]
- Restuccia, L. Non-equilibrium temperatures and heat transport in nanosystems with defects, described by a tensorial internal variable. Commun. Appl. Ind. Math. 2016, 7, 81–97. [Google Scholar] [CrossRef]
- Maruszewski, B. On a dislocation core tensor. Phys. Status Solidi (b) 1991, 168, 59. [Google Scholar] [CrossRef]
- Jou, D.; Mongiovì, M.S. Description and evolution of anisotropy in superfluid vortex tangles with conterflow and rotation. Phys. Rev. B 2006, 74, 054509. [Google Scholar] [CrossRef]
- Mazzeo, M.P.; Restuccia, L. Thermodynamics of n-type extrinsic semiconductors. Energy 2011, 36, 4577–4584. [Google Scholar] [CrossRef]
- Eu, B.C.; Wagh, A.S. Nonlinear field dependence of carrier mobilities and irreversible thermodynamics in semiconductors. Phys. Rev. B 1983, 27, 1037. [Google Scholar] [CrossRef]
- Estreicher, S.K.; Sanati, M.; West, D.; Ruymgaart, F. Thermodynamics of impurities in semiconductors. Phys. Rev. B 2004, 70, 125209. [Google Scholar] [CrossRef]
- Dettori, R.; Melis, C.; Cartoixà, X.; Rurali, R.; Colombo, L. Thermal boundary resistance in semiconductors by non-equilibrium thermodynamics. Adv. Phys. X 2016, 1, 246–261. [Google Scholar] [CrossRef]
- Wolfe, J.P. Thermodynamics of excitons in semiconductors. Phys. Today 1982, 35, 46. [Google Scholar] [CrossRef]
- Albinus, G.; Gajewski, H.; Hu¨nlich, R. Thermodynamic design of energy models of semiconductor devices. Nonlinearity 2002, 15, 367–384. [Google Scholar] [CrossRef]
- Luzzi, R.; Vasconcellos, A.R.; Galvao Ramos, J. Predictive Statistical Mechanics. In A Non-Equilibrium Ensemble Formalism; Kluwer: Dordrecht, The Netherlands, 2002. [Google Scholar]
- Luzzi, R.; Vasconcellos, A.R.; Galvao Ramos, J. Statistical Foundations of Non-Equilibrium Thermodynamics; Teubner: Leipzig, Germany, 2000. [Google Scholar]
- Muschik, W. Remarks on thermodynamical terminology. J. Non-Equilib. Thermodyn. 2004, 29, 199–203. [Google Scholar] [CrossRef]
- Muschik, W.; Papenfuss, C.; Ehrentraut, H. A sketch of continuum Thermodynamics. J. Non–Newton. Fluid Mech. 2001, 96, 255–299. [Google Scholar] [CrossRef]
- Muschik, W. Second Law and its Amendment: The Axiom of No-reversible Directions-Revisited. arXiv 2023, arXiv:2306.09794. [Google Scholar] [CrossRef]
- Luca, L.; Romano, V. Comparing linear and nonlinear hydrodynamical models for charge transport in graphene based on the Maximum Entropy Principle. Int. J. Non-Linear Mech. 2018, 104, 39–58. [Google Scholar] [CrossRef]
- Drago, C.R.; Romano, V. Optimal control for semiconductor diode design based on the MEP energy-transport model. J. Theor. Comput. Transp. 2018, 46, 459–479. [Google Scholar] [CrossRef]
- Mascali, G.; Romano, V. Exploitation of the maximum entropy principle in mathematical modeling of charge transport in semiconductors. Entropy 2017, 19, 36. [Google Scholar] [CrossRef]
- Camiola, V.D.; Romano, V. 2 DEG-3DEG charge transport model for MOSFET based on the maximum entropy principle. SIAM J. Appl. Math. 2013, 73, 1439–1459. [Google Scholar] [CrossRef]
- Camiola, D.; Mascali, G.; Romano, V. Simulation of a double-gate MOSFET by a non-parabolic energy-transport model for semiconductors based on the maximum entropy principle. Math. Comput. Model. 2013, 58, 321–343. [Google Scholar] [CrossRef]
- Mascali, G.; Romano, V. A non parabolic hydrodynamical subband model for semiconductors based on the maximum entropy principle. Math. Comput. Model. 2012, 55, 1003–1020. [Google Scholar] [CrossRef]
- Mascali, G.; Romano, V. Hydrodynamic subband model for semiconductors based on the maximum entropy principle. Il Nuovo Cimento 2010, 33, 155–163. [Google Scholar]
- La Rosa, S.; Mascali, G.; Romano, V. Exact maximum entropy closure of the hydrodynamical model for Si semiconductors: The 8-moment case. SIAM J. Appl. Math. 2009, 70, 710–734. [Google Scholar] [CrossRef]
- Romano, V. Quantum corrections to the semiclassical hydrodynamical model of semiconductors based on the maximum entropy principle. J. Math. Phys. 2007, 48, 123504. [Google Scholar] [CrossRef]
- Junk, M.; Romano, V. Maximum entropy moment system of the semiconductor Boltzmann equation using Kane’s dispersion relation. Cont. Mech. Thermodyn. 2005, 17, 247–267. [Google Scholar] [CrossRef]
- Mascali, G.; Romano, V. Hydrodynamical model of charge transport in GaAs based on the maximum entropy principle. Contin. Mech. Thermodyn. 2002, 14, 405–423. [Google Scholar] [CrossRef]
- Anile, A.M.; Muscato, O.; Romano, V. Moment equations with maximum entropy closure for carrier transport in semiconductor devices: Validation in bulk silicon. VLSI Des. 2000, 10, 335–354. [Google Scholar] [CrossRef]
- Anile, A.M.; Romano, V.; Russo, G. Extended hydrodynamical model of carrier transport in semiconductors. SIAM J. Appl. Math. 2000, 61, 74–101. [Google Scholar] [CrossRef]
- Anile, A.M.; Romano, V. Non parabolic band transport in semiconductors: Closure of the moment equations. Cont. Mechan. Thermodyn. 1999, 11, 307–325. [Google Scholar] [CrossRef]
- Liu, I.-S. Method of Lagrange multipliers for exploitation of the entropy principle. Arch. Ration. Mech. Anal. 1972, 46, 131–148. [Google Scholar] [CrossRef]
- Landolt-Bo¨rnstein. Numerical Data and Functional Relationships in Science of Technology; NS III/17a; Springer: Berlin/Heidelberg, 1982. [Google Scholar]
- Swinburne, T.D.; Dudarev, S.L. Phonon drag force acting on a mobile crystal defect: Full treatment of discreteness and nonlinearity. Phys. Rev. B 2015, 92, 134302. [Google Scholar] [CrossRef]
- Lu¨cke, K.; Granato, A.V. Simplified theory of dislocation damping including point-defect drag. I. Theory of drag by equidistant point defects. Phys. Rev. B 1981, 24, 6991. [Google Scholar] [CrossRef]
- Malashenko, V.V. Dynamic drag of dislocation by point defects in near-surface crystal layer. Modern Phys. Lett. B 2009, 23, 2041–2047. [Google Scholar] [CrossRef]
- Masharov, S.I. Effect of phonon drag on the kinetic properties of alloys. Phys. Status Solidi (b) 1968, 27, 455–461. [Google Scholar] [CrossRef]
- Maruszewski, B. Thermodiffusive surface waves in semiconductors. J. Acoust. Soc. Am. 1989, 85, 1967–1977. [Google Scholar] [CrossRef]
- Kireev, P.S. Semiconductors Physics; MIR Publishers: Moscow, Russia, 1978. [Google Scholar]
- Lebon, G.; Jou, D.; Casas-Vázquez, J. Understanding Non-Equilibrium Thermodynamics. Foundations, Applications, Frontiers; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Huber, W.H.; Hernandez, L.M.; Goldman, A.M. Electric field dependence of the thermal conductivity of quantum paraelectrics. Phys. Rev. B 2000, 62, 8588–8591. [Google Scholar]
- Aggarwal, G.K.; Ashok, K.; Naithani, U.C. Field dependent thermal conductivity of SrTiO3, BaTiO3 and KTaO3 ferroelectric perovskites. Int. J. Eng. Res. Dev. 2012, 4, 61–67. [Google Scholar]
- Seijas-Bellido, J.A.; Aramberri, H.; Íñiguez, J.; Rurali, R. Electric control of the heat flux through electrophononic effects. Phys. Rev. B 2018, 97, 184306. [Google Scholar] [CrossRef]
- Colomer, A.M.; Massaguer, E.; Pujol, T.; Comamala, M.; Montoro, L.; González, J.R. Electrically tunable thermal conductivity in thermoelectric materials: Active and passive control. Appl. Energy 2015, 154, 709–717. [Google Scholar]
- Liu, C.; Mishra, V.; Chen, Y.; Dames, C. Large thermal conductivity switch ratio in barium titanate under electric field through first-principles calculation. Adv. Theory Simul. 2018, 1, 1800098. [Google Scholar] [CrossRef]


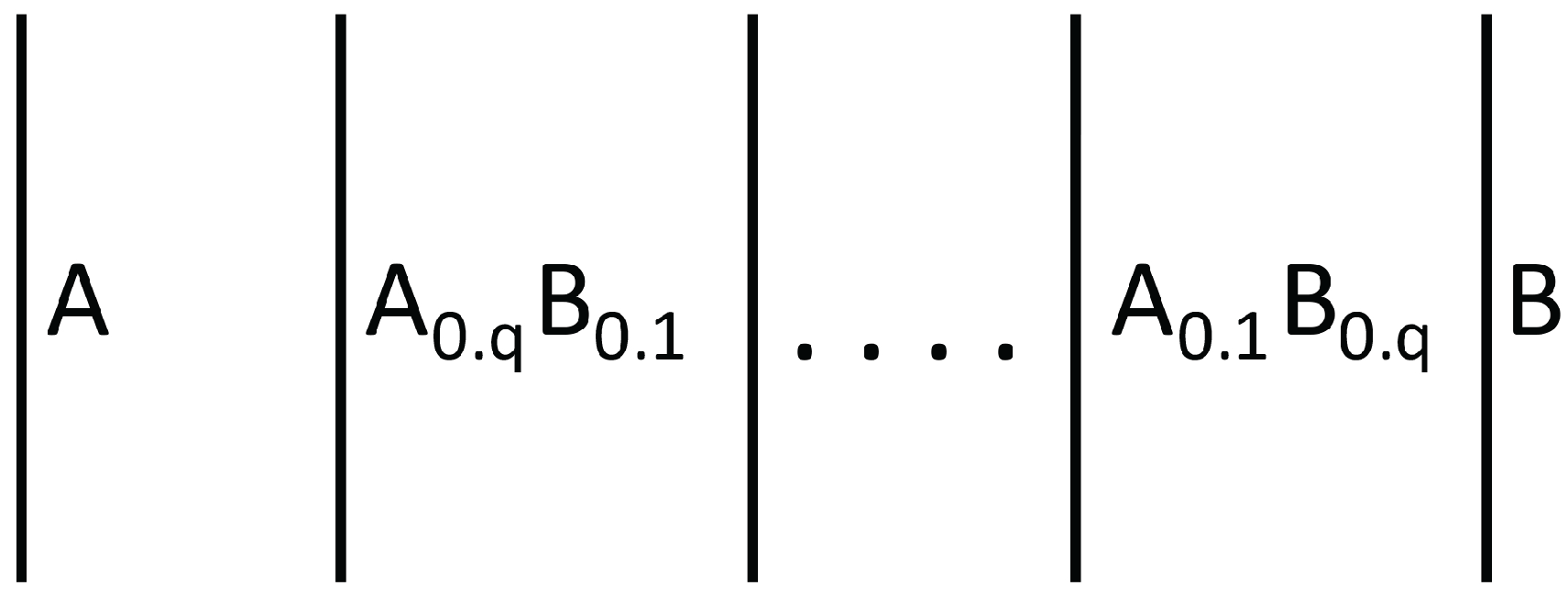




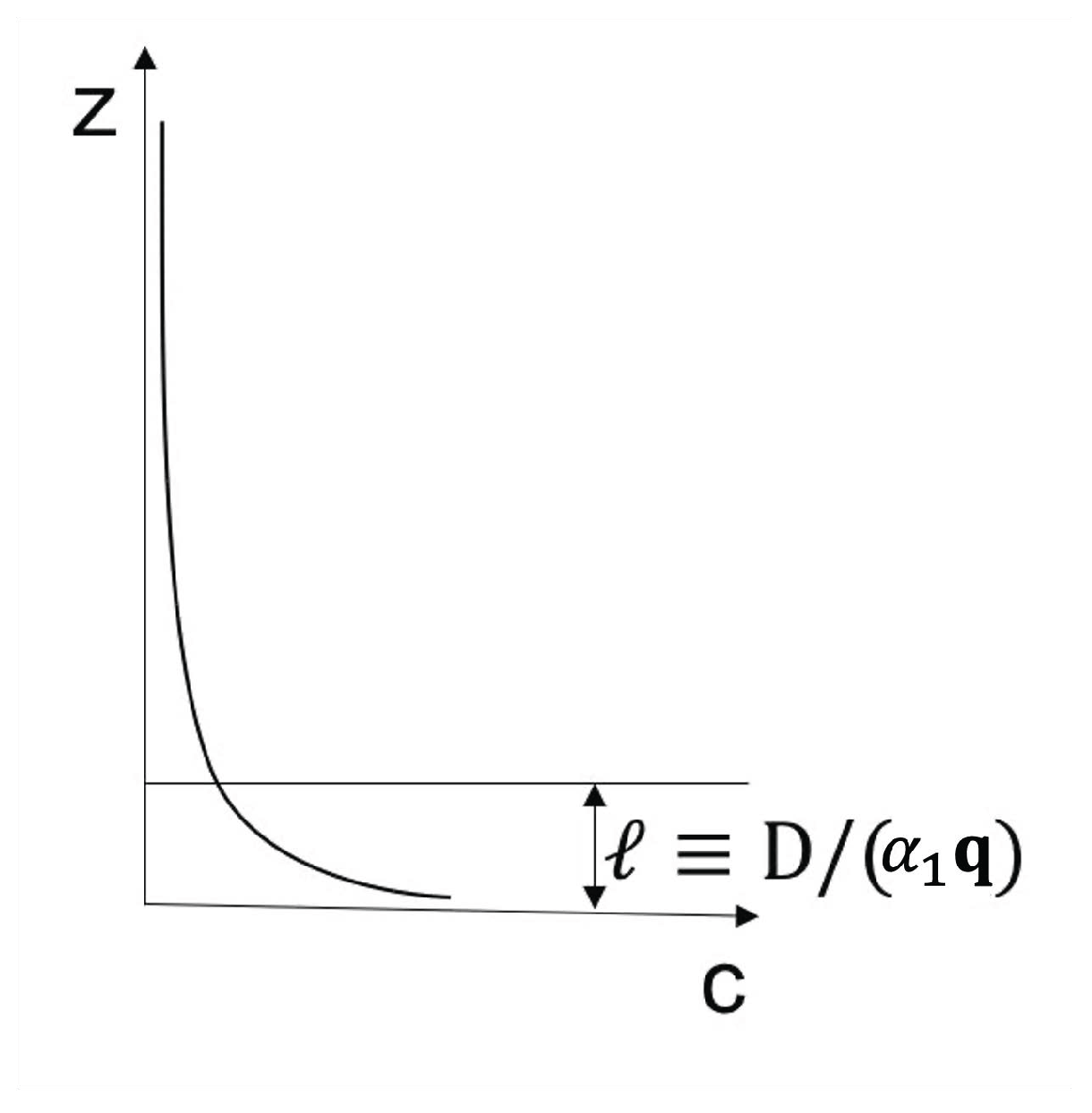
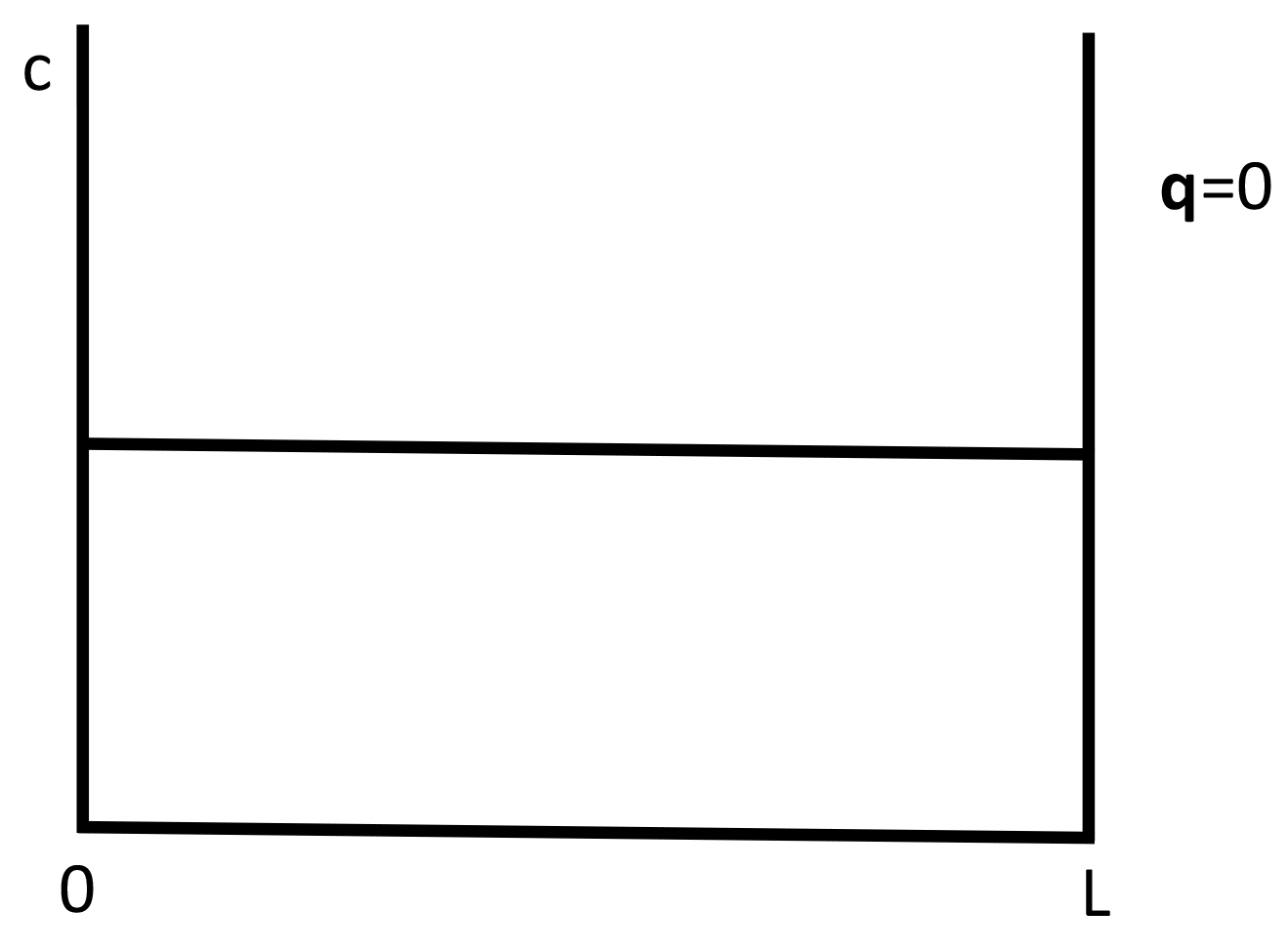
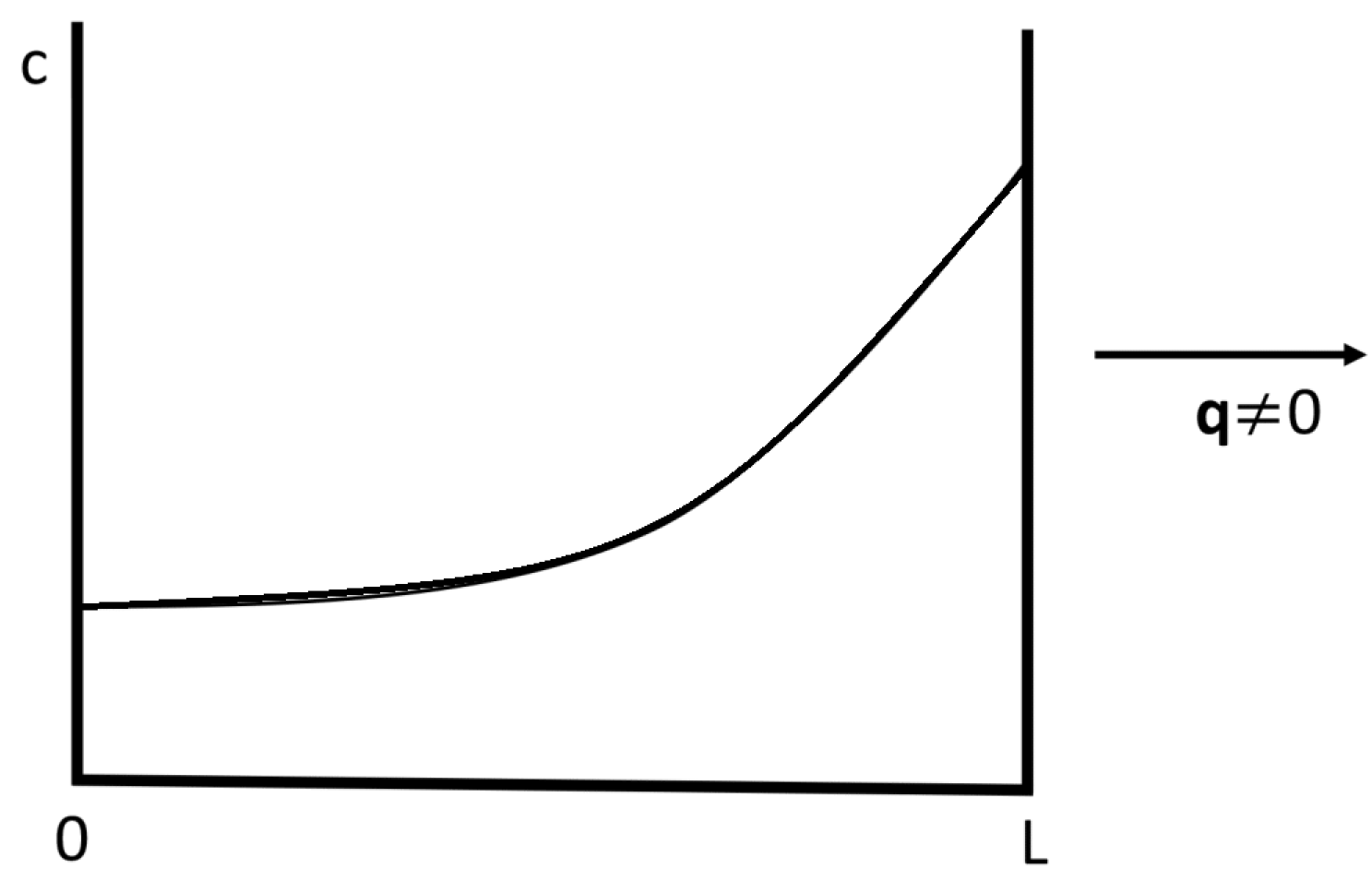
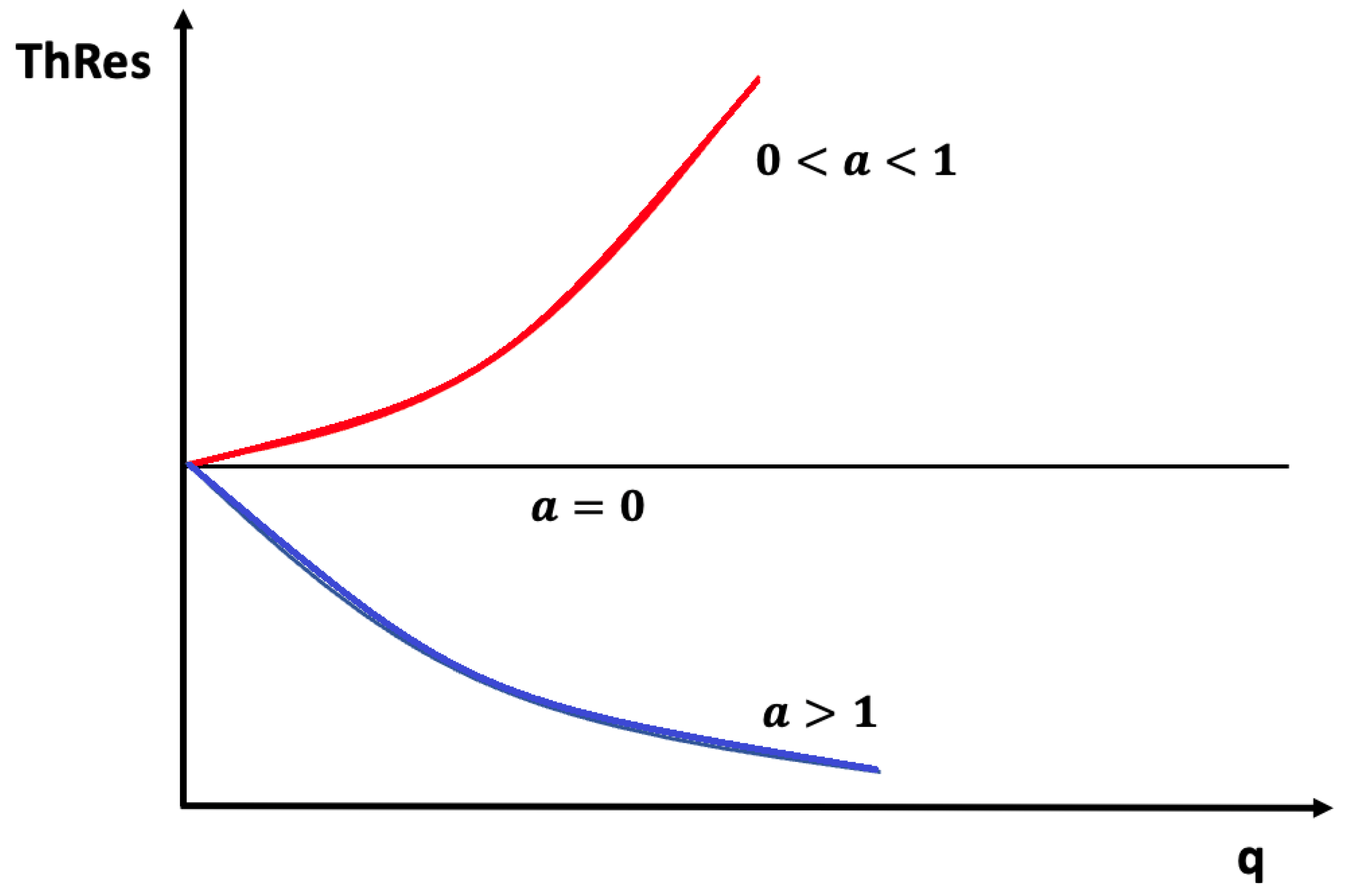
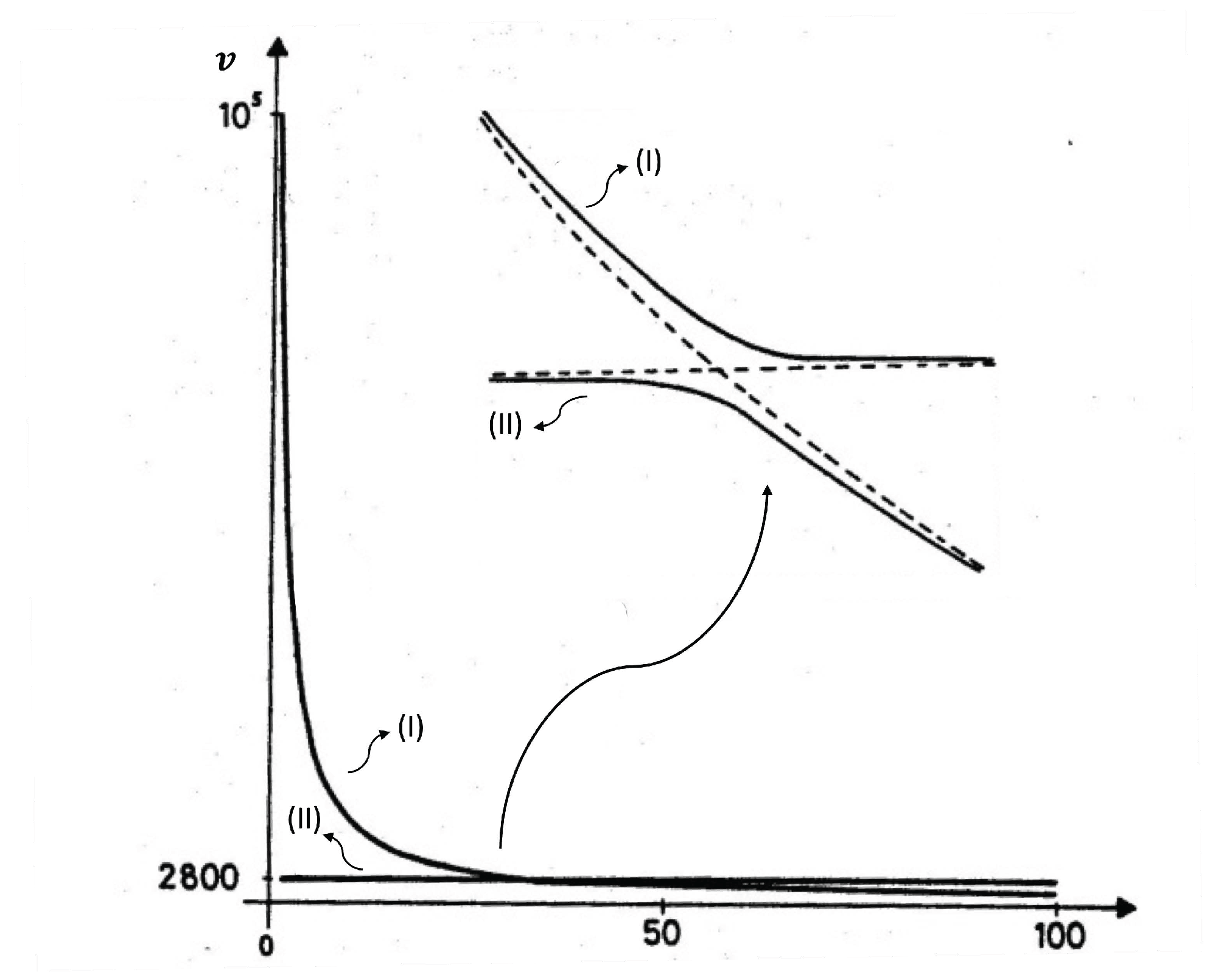
| Coefficient | Measure Unit | Value | Name |
|---|---|---|---|
| Kg m | mass density [193,198] | ||
| m s | electron diffusion coefficient [193,198] | ||
| m s | dislocation diffusion coefficient (119) | ||
| s | < | electron relaxation time [198] | |
| s | dislocation relaxation time [160] | ||
| C m s | < | cross-effects function, estimated [161,199] | |
| Kg C s | <70 | cross-effects function, estimated [161,199] | |
| C m s | < | recombination constant, estimated [161] | |
| m s | (119) | ||
| m s | 2846 | [160] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jou, D.; Restuccia, L. Non-Equilibrium Thermodynamics of Heat Transport in Superlattices, Graded Systems, and Thermal Metamaterials with Defects. Entropy 2023, 25, 1091. https://doi.org/10.3390/e25071091
Jou D, Restuccia L. Non-Equilibrium Thermodynamics of Heat Transport in Superlattices, Graded Systems, and Thermal Metamaterials with Defects. Entropy. 2023; 25(7):1091. https://doi.org/10.3390/e25071091
Chicago/Turabian StyleJou, David, and Liliana Restuccia. 2023. "Non-Equilibrium Thermodynamics of Heat Transport in Superlattices, Graded Systems, and Thermal Metamaterials with Defects" Entropy 25, no. 7: 1091. https://doi.org/10.3390/e25071091
APA StyleJou, D., & Restuccia, L. (2023). Non-Equilibrium Thermodynamics of Heat Transport in Superlattices, Graded Systems, and Thermal Metamaterials with Defects. Entropy, 25(7), 1091. https://doi.org/10.3390/e25071091







