Lossy Micromaser Battery: Almost Pure States in the Jaynes–Cummings Regime
Abstract
:1. Introduction
2. The Model
3. Incoherent Charging Protocol
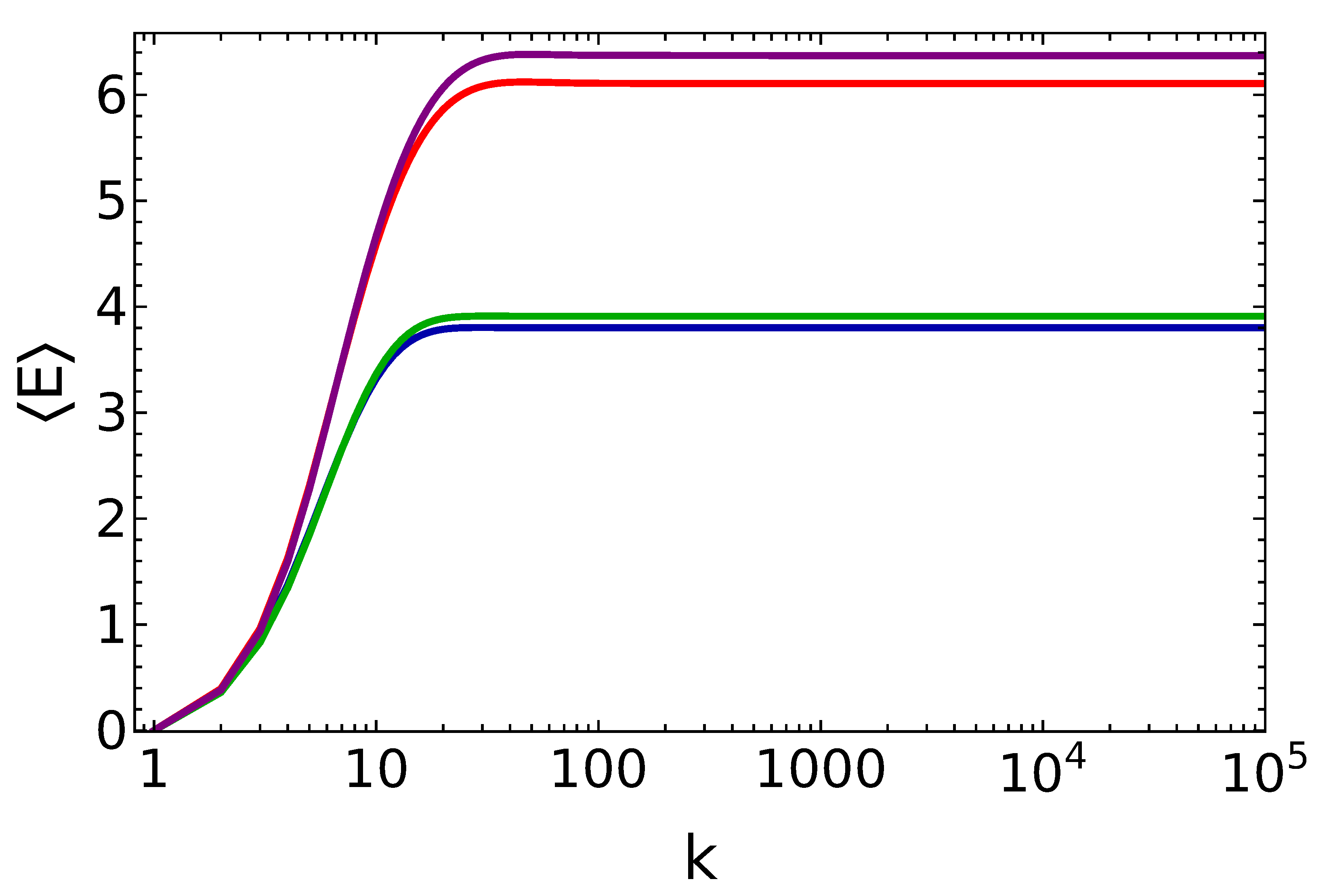
4. Coherent Charging Protocol
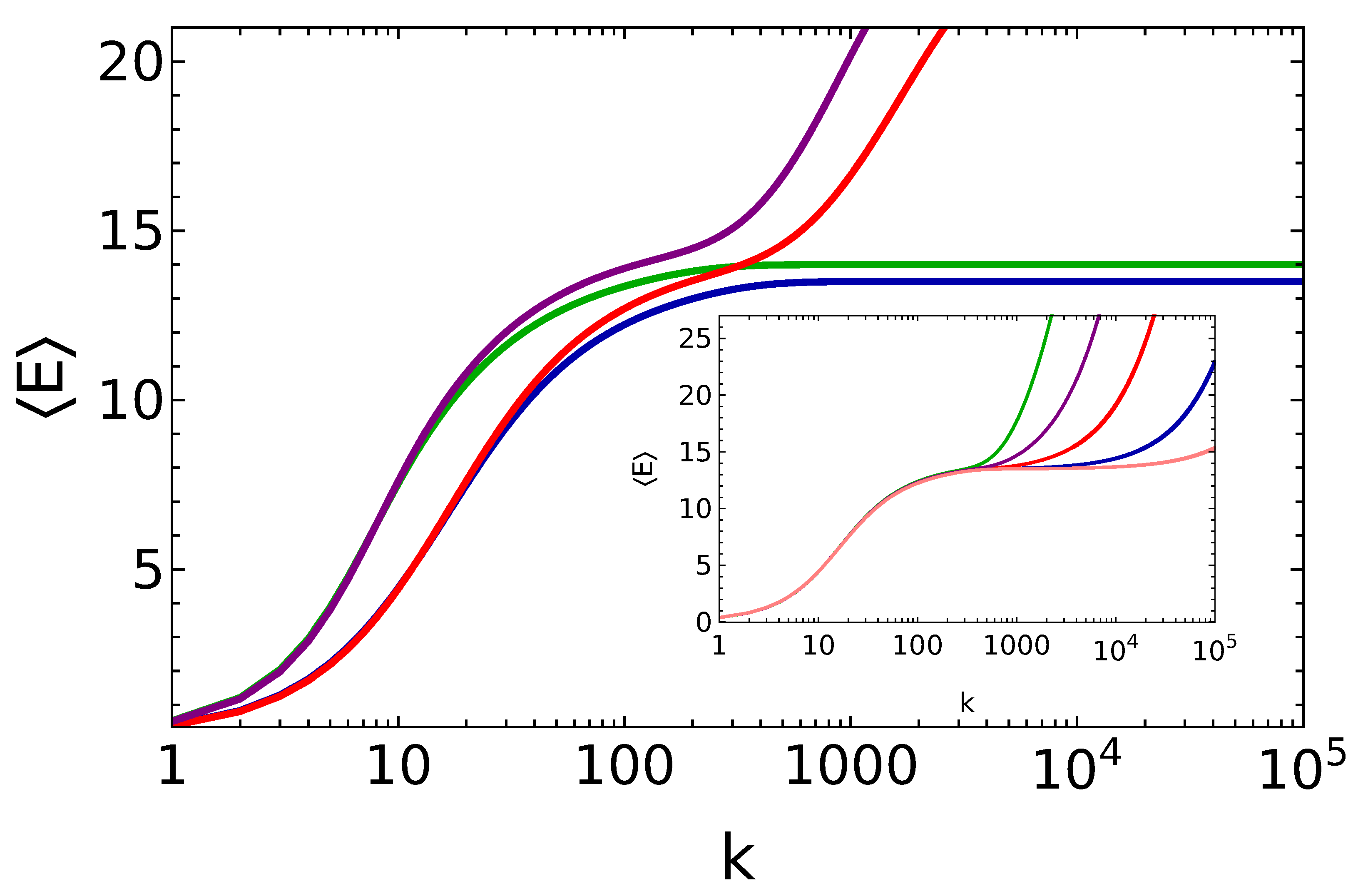
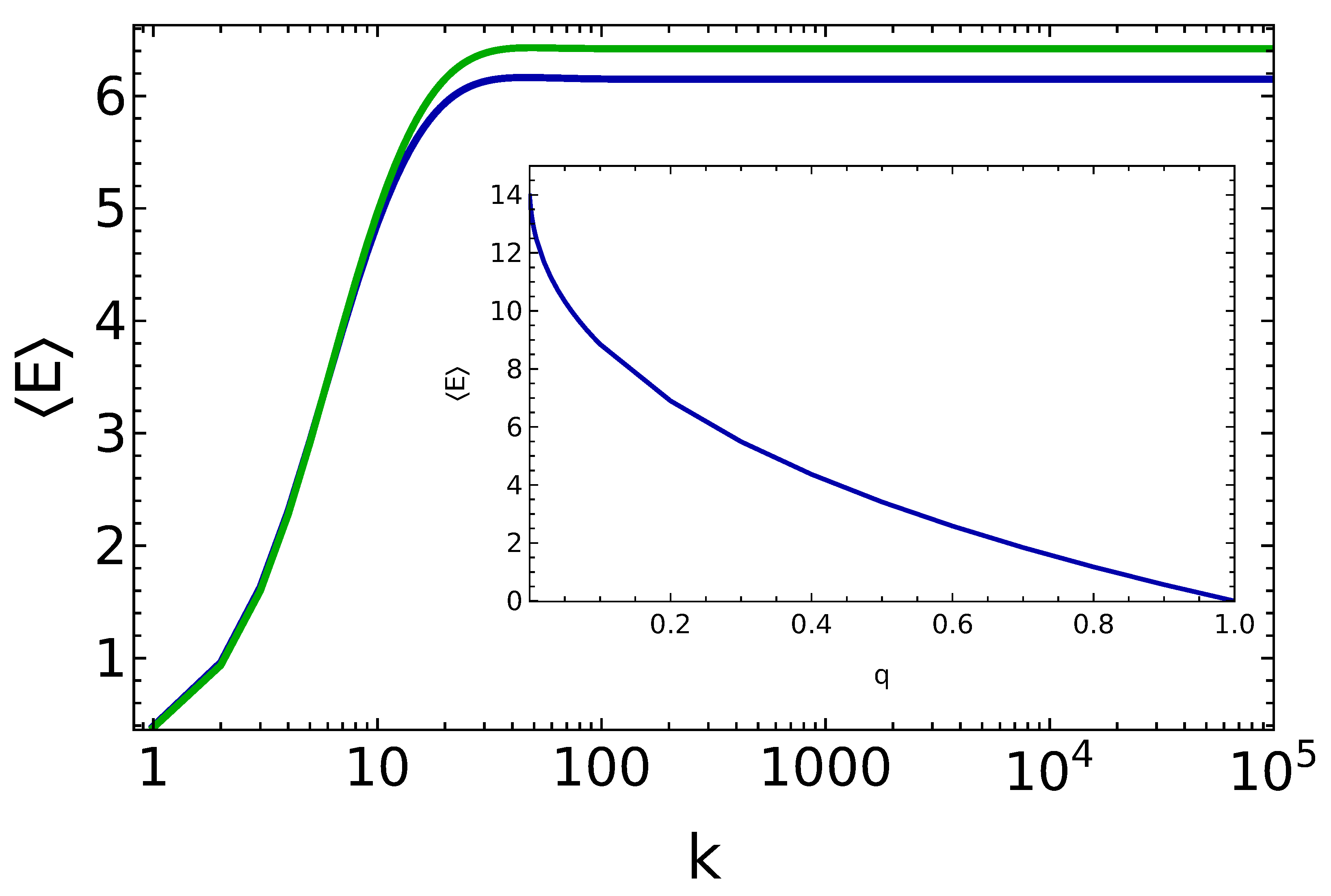
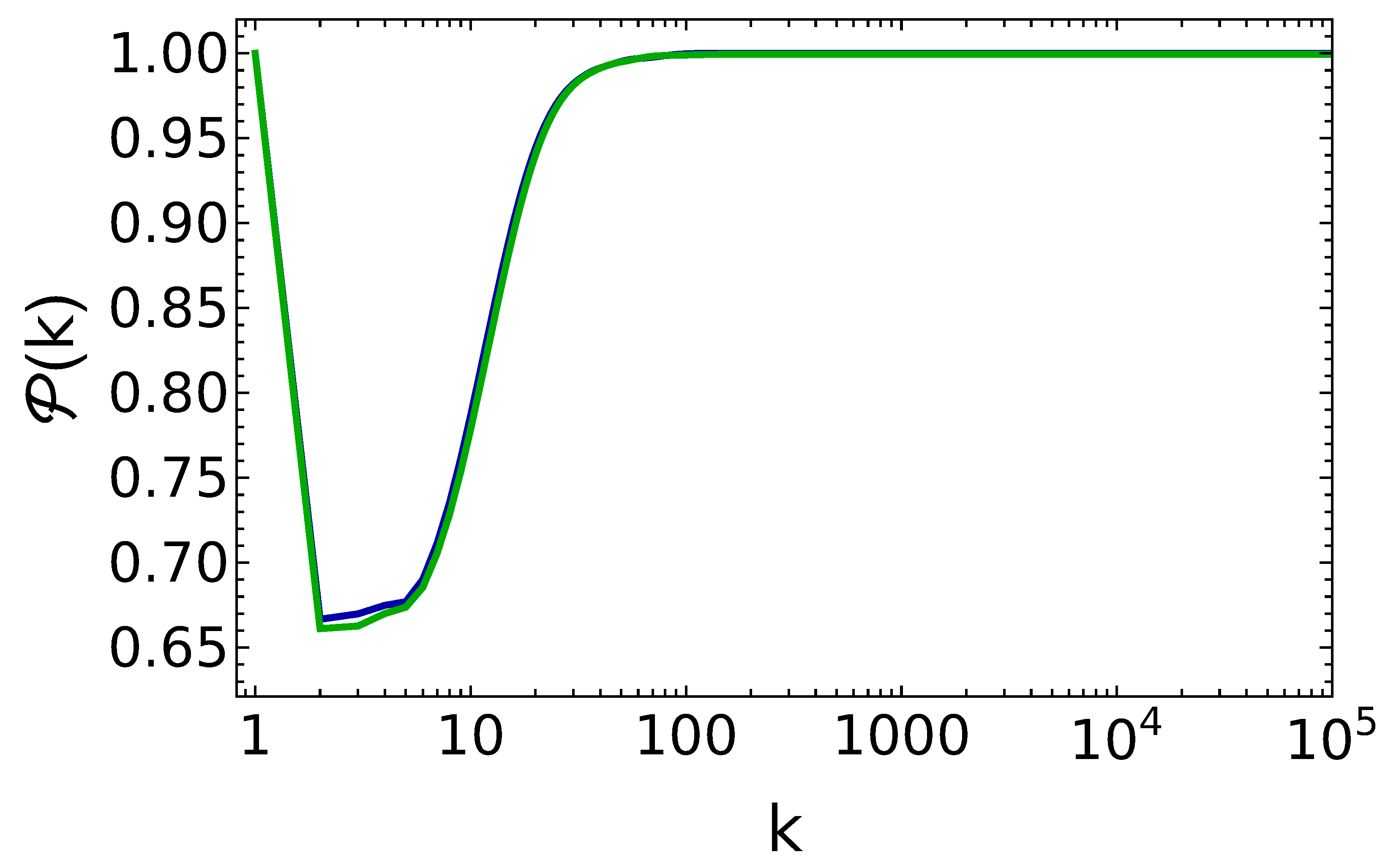
5. Lossy Cavity
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Rau, J. Relaxation Phenomena in Spin and Harmonic Oscillator Systems. Phys. Rev. 1963, 129, 1880–1888. [Google Scholar] [CrossRef]
- Alicki, R.; Lendi, K. Quantum Dynamical Semigroups and Applications; Springer: Berlin/Heidelberg, Germany, 1987. [Google Scholar]
- Scarani, V.; Ziman, M.; Štelmachovič, P.; Gisin, N.; Bužek, V. Thermalizing Quantum Machines: Dissipation and Entanglement. Phys. Rev. Lett. 2002, 88, 097905. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ziman, M.; Štelmachovič, P.; Bužek, V.; Hillery, M.; Scarani, V.; Gisin, N. Diluting quantum information: An analysis of information transfer in system-reservoir interactions. Phys. Rev. A 2002, 65, 042105. [Google Scholar] [CrossRef] [Green Version]
- Ziman, M.; Bužek, V. All (qubit) decoherences: Complete characterization and physical implementation. Phys. Rev. A 2005, 72, 022110. [Google Scholar] [CrossRef] [Green Version]
- Benenti, G.; Palma, G.M. Reversible and irreversible dynamics of a qubit interacting with a small environment. Phys. Rev. A 2007, 75, 052110. [Google Scholar] [CrossRef] [Green Version]
- Giovannetti, V.; Palma, G.M. Master Equations for Correlated Quantum Channels. Phys. Rev. Lett. 2012, 108, 040401. [Google Scholar] [CrossRef] [Green Version]
- Uzdin, R.; Kosloff, R. The multilevel four-stroke swap engine and its environment. New J. Phys. 2014, 16, 095003. [Google Scholar] [CrossRef]
- Lorenzo, S.; McCloskey, R.; Ciccarello, F.; Paternostro, M.; Palma, G.M. Landauer’s Principle in Multipartite Open Quantum System Dynamics. Phys. Rev. Lett. 2015, 115, 120403. [Google Scholar] [CrossRef] [Green Version]
- Strasberg, P.; Schaller, G.; Brandes, T.; Esposito, M. Quantum and Information Thermodynamics: A Unifying Framework Based on Repeated Interactions. Phys. Rev. X 2017, 7, 021003. [Google Scholar] [CrossRef] [Green Version]
- Chiara, G.D.; Landi, G.; Hewgill, A.; Reid, B.; Ferraro, A.; Roncaglia, A.J.; Antezza, M. Reconciliation of quantum local master equations with thermodynamics. New J. Phys. 2018, 20, 113024. [Google Scholar] [CrossRef]
- Pezzutto, M.; Paternostro, M.; Omar, Y. An out-of-equilibrium non-Markovian quantum heat engine. Quantum Sci. Technol. 2019, 4, 025002. [Google Scholar] [CrossRef] [Green Version]
- Landi, G.T. Battery Charging in Collision Models with Bayesian Risk Strategies. Entropy 2021, 23, 1627. [Google Scholar] [CrossRef]
- Shaghaghi, V.; Palma, G.M.; Benenti, G. Extracting work from random collisions: A model of a quantum heat engine. Phys. Rev. E 2022, 105, 034101. [Google Scholar] [CrossRef] [PubMed]
- Seah, S.; Perarnau-Llobet, M.; Haack, G.; Brunner, N.; Nimmrichter, S. Quantum Speed-Up in Collisional Battery Charging. Phys. Rev. Lett. 2021, 127, 100601. [Google Scholar] [CrossRef]
- Salvia, R.; Perarnau-Llobet, M.; Haack, G.; Brunner, N.; Nimmrichter, S. Quantum advantage in charging cavity and spin batteries by repeated interactions. arXiv 2022, arXiv:2205.00026. [Google Scholar] [CrossRef]
- Campbell, S.; Vacchini, B. Collision models in open system dynamics: A versatile tool for deeper insights? Europhys. Lett. 2021, 133, 60001. [Google Scholar] [CrossRef]
- Ciccarello, F.; Lorenzo, S.; Giovannetti, V.; Palma, G.M. Quantum collision models: Open system dynamics from repeated interactions. Phys. Rep. 2022, 954, 1–70. [Google Scholar] [CrossRef]
- Esposito, M.; Harbola, U.; Mukamel, S. Nonequilibrium fluctuations, fluctuation theorems, and counting statistics in quantum systems. Rev. Mod. Phys. 2009, 81, 1665–1702. [Google Scholar] [CrossRef] [Green Version]
- Campisi, M.; Hänggi, P.; Talkner, P. Colloquium: Quantum fluctuation relations: Foundations and applications. Rev. Mod. Phys. 2011, 83, 771–791. [Google Scholar] [CrossRef] [Green Version]
- Vinjanampathy, S.; Anders, J. Quantum thermodynamics. Contemp. Phys. 2016, 57, 545–579. [Google Scholar] [CrossRef] [Green Version]
- Pekola, J.P.; Karimi, B. Colloquium: Quantum heat transport in condensed matter systems. Rev. Mod. Phys. 2021, 93, 041001. [Google Scholar] [CrossRef]
- Carrega, M.; Cangemi, L.M.; De Filippis, G.; Cataudella, V.; Benenti, G.; Sassetti, M. Engineering Dynamical Couplings for Quantum Thermodynamic Tasks. PRX Quantum 2022, 3, 010323. [Google Scholar] [CrossRef]
- Barra, F. Dissipative Charging of a Quantum Battery. Phys. Rev. Lett. 2019, 122, 210601. [Google Scholar] [CrossRef] [Green Version]
- Shaghaghi, V.; Singh, V.; Benenti, G.; Rosa, D. Micromasers as quantum batteries. Quantum Sci. Technol. 2022, 7, 04LT01. [Google Scholar] [CrossRef]
- Alicki, R.; Fannes, M. Entanglement boost for extractable work from ensembles of quantum batteries. Phys. Rev. E 2013, 87, 042123. [Google Scholar] [CrossRef] [Green Version]
- Hovhannisyan, K.V.; Perarnau-Llobet, M.; Huber, M.; Acín, A. Entanglement Generation is Not Necessary for Optimal Work Extraction. Phys. Rev. Lett. 2013, 111, 240401. [Google Scholar] [CrossRef] [Green Version]
- Andolina, G.M.; Farina, D.; Mari, A.; Pellegrini, V.; Giovannetti, V.; Polini, M. Charger-mediated energy transfer in exactly solvable models for quantum batteries. Phys. Rev. B 2018, 98, 205423. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Y.Y.; Yang, T.R.; Fu, L.; Wang, X. Powerful harmonic charging in a quantum battery. Phys. Rev. E 2019, 99, 052106. [Google Scholar] [CrossRef] [Green Version]
- Caravelli, F.; Coulter-De Wit, G.; García-Pintos, L.P.; Hamma, A. Random quantum batteries. Phys. Rev. Res. 2020, 2, 023095. [Google Scholar] [CrossRef]
- Quach, J.Q.; Munro, W.J. Using Dark States to Charge and Stabilize Open Quantum Batteries. Phys. Rev. Appl. 2020, 14, 024092. [Google Scholar] [CrossRef]
- Crescente, A.; Carrega, M.; Sassetti, M.; Ferraro, D. Charging and energy fluctuations of a driven quantum battery. New J. Phys. 2020, 22, 063057. [Google Scholar] [CrossRef]
- Friis, N.; Huber, M. Precision and Work Fluctuations in Gaussian Battery Charging. Quantum 2018, 2, 61. [Google Scholar] [CrossRef] [Green Version]
- Rossini, D.; Andolina, G.M.; Polini, M. Many-body localized quantum batteries. Phys. Rev. B 2019, 100, 115142. [Google Scholar] [CrossRef] [Green Version]
- Santos, A.C.; Çakmak, B.; Campbell, S.; Zinner, N.T. Stable adiabatic quantum batteries. Phys. Rev. E 2019, 100, 032107. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Rosa, D.; Rossini, D.; Andolina, G.M.; Polini, M.; Carrega, M. Ultra-stable charging of fast-scrambling SYK quantum batteries. J. High Energy Phys. 2020, 2020, 67. [Google Scholar] [CrossRef]
- Binder, F.C.; Vinjanampathy, S.; Modi, K.; Goold, J. Quantacell: Powerful charging of quantum batteries. New J. Phys. 2015, 17, 075015. [Google Scholar] [CrossRef] [Green Version]
- Campaioli, F.; Pollock, F.A.; Binder, F.C.; Céleri, L.; Goold, J.; Vinjanampathy, S.; Modi, K. Enhancing the Charging Power of Quantum Batteries. Phys. Rev. Lett. 2017, 118, 150601. [Google Scholar] [CrossRef] [Green Version]
- Le, T.P.; Levinsen, J.; Modi, K.; Parish, M.M.; Pollock, F.A. Spin-chain model of a many-body quantum battery. Phys. Rev. A 2018, 97, 022106. [Google Scholar] [CrossRef] [Green Version]
- Ferraro, D.; Campisi, M.; Andolina, G.M.; Pellegrini, V.; Polini, M. High-Power Collective Charging of a Solid-State Quantum Battery. Phys. Rev. Lett. 2018, 120, 117702. [Google Scholar] [CrossRef] [Green Version]
- Andolina, G.M.; Keck, M.; Mari, A.; Giovannetti, V.; Polini, M. Quantum versus classical many-body batteries. Phys. Rev. B 2019, 99, 205437. [Google Scholar] [CrossRef] [Green Version]
- Crescente, A.; Carrega, M.; Sassetti, M.; Ferraro, D. Ultrafast charging in a two-photon Dicke quantum battery. Phys. Rev. B 2020, 102, 245407. [Google Scholar] [CrossRef]
- Ghosh, S.; Chanda, T.; Sen (De), A. Enhancement in the performance of a quantum battery by ordered and disordered interactions. Phys. Rev. A 2020, 101, 032115. [Google Scholar] [CrossRef] [Green Version]
- Rossini, D.; Andolina, G.M.; Rosa, D.; Carrega, M.; Polini, M. Quantum Advantage in the Charging Process of Sachdev-Ye-Kitaev Batteries. Phys. Rev. Lett. 2020, 125, 236402. [Google Scholar] [CrossRef]
- García-Pintos, L.P.; Hamma, A.; del Campo, A. Fluctuations in Extractable Work Bound the Charging Power of Quantum Batteries. Phys. Rev. Lett. 2020, 125, 040601. [Google Scholar] [CrossRef] [PubMed]
- Cusumano, S.; Rudnicki, L. Comment on “Fluctuations in Extractable Work Bound the Charging Power of Quantum Batteries”. Phys. Rev. Lett. 2021, 127, 028901. [Google Scholar] [CrossRef] [PubMed]
- García-Pintos, L.P.; Hamma, A.; del Campo, A. García-Pintos, Hamma, and del Campo Reply. Phys. Rev. Lett. 2021, 127, 028902. [Google Scholar] [CrossRef] [PubMed]
- Zakavati, S.; Tabesh, F.T.; Salimi, S. Bounds on charging power of open quantum batteries. Phys. Rev. E 2021, 104, 054117. [Google Scholar] [CrossRef]
- Ghosh, S.; Chanda, T.; Mal, S.; Sen (De), A. Fast charging of a quantum battery assisted by noise. Phys. Rev. A 2021, 104, 032207. [Google Scholar] [CrossRef]
- Mondal, S.; Bhattacharjee, S. Periodically driven many-body quantum battery. Phys. Rev. E 2022, 105, 044125. [Google Scholar] [CrossRef]
- Kanti Konar, T.; Lakkaraju, L.G.C.; De, A.S. Quantum Battery with Non-Hermitian Charging. arXiv 2022, arXiv:2203.09497. [Google Scholar]
- Gyhm, J.Y.; Šafránek, D.; Rosa, D. Quantum Charging Advantage Cannot Be Extensive without Global Operations. Phys. Rev. Lett. 2022, 128, 140501. [Google Scholar] [CrossRef]
- Mazzoncini, F.; Cavina, V.; Andolina, G.M.; Erdman, P.A.; Giovannetti, V. Optimal Control Methods for Quantum Batteries. arXiv 2022, arXiv:2210.04028. [Google Scholar] [CrossRef]
- Erdman, P.A.; Andolina, G.M.; Giovannetti, V.; Noé, F. Reinforcement learning optimization of the charging of a Dicke quantum battery. arXiv 2022, arXiv:2212.12397. [Google Scholar] [CrossRef]
- Allahverdyan, A.E.; Balian, R.; Nieuwenhuizen, T.M. Maximal work extraction from finite quantum systems. Europhys. Lett. (EPL) 2004, 67, 565–571. [Google Scholar] [CrossRef] [Green Version]
- Delmonte, A.; Crescente, A.; Carrega, M.; Ferraro, D.; Sassetti, M. Characterization of a Two-Photon Quantum Battery: Initial Conditions, Stability and Work Extraction. Entropy 2021, 23, 612. [Google Scholar] [CrossRef] [PubMed]
- Šafránek, D.; Rosa, D.; Binder, F. Work extraction from unknown quantum sources. arXiv 2022, arXiv:2209.11076. [Google Scholar] [CrossRef]
- Carrega, M.; Crescente, A.; Ferraro, D.; Sassetti, M. Dissipative dynamics of an open quantum battery. New J. Phys. 2020, 22, 083085. [Google Scholar] [CrossRef]
- Shnirman, A.; Makhlin, Y.; Schön, G. Noise and Decoherence in Quantum Two-Level Systems. Phys. Scr. 2002, 2002, 147. [Google Scholar] [CrossRef] [Green Version]
- Devoret, M.H.; Schoelkopf, R.J. Superconducting Circuits for Quantum Information: An Outlook. Science 2013, 339, 1169–1174. [Google Scholar] [CrossRef] [Green Version]
- Calzona, A.; Carrega, M. Multi-mode architectures for noise-resilient superconducting qubits. Supercond. Sci. Technol. 2022, 36, 023001. [Google Scholar] [CrossRef]
- Meystre, P.; Sargent, M. Elements of Quantum Optics; Springer: Berlin/Heidelberg, Germany, 1998. [Google Scholar]
- Huang, J.F.; Liao, J.Q.; Kuang, L.M. Ultrastrong Jaynes-Cummings model. Phys. Rev. A 2020, 101, 043835. [Google Scholar] [CrossRef]
- Lindblad, G. On the generators of quantum dynamical semigroups. Commun. Math. Phys. 1976, 48, 119–130. [Google Scholar] [CrossRef]
- Gorini, V.; Kossakowski, A.; Sudarshan, E.C.G. Completely positive dynamical semigroups of N-level systems. J. Math. Phys. 1976, 17, 821–825. [Google Scholar] [CrossRef]
- Benenti, G.; Casati, G.; Rossini, D.; Strini, G. Principles of Quantum Computation and Information (A Comprehensive Textbook); World Scientific: Singapore, 2019. [Google Scholar]
- Filipowicz, P.; Javanainen, J.; Meystre, P. Quantum and semiclassical steady states of a kicked cavity mode. J. Opt. Soc. Am. B 1986, 3, 906–910. [Google Scholar] [CrossRef]
- Slosser, J.J.; Meystre, P.; Braunstein, S.L. Harmonic oscillator driven by a quantum current. Phys. Rev. Lett. 1989, 63, 934–937. [Google Scholar] [CrossRef] [PubMed]
- Slosser, J.J.; Meystre, P. Tangent and cotangent states of the electromagnetic field. Phys. Rev. A 1990, 41, 3867–3874. [Google Scholar] [CrossRef]
- Gardiner, C.; Zoller, P. Quantum Noise: A Handbook of Markovian and Non-markovian Quantum Stochastic Methods With Applications to Quantum Optics; Springer: Berlin/Heidelberg, Germany, 2004. [Google Scholar]
- Slosser, J.J.; Meystre, P.; Wright, E.M. Generation of macroscopic superpositions in a micromaser. Opt. Lett. 1990, 15, 233–235. [Google Scholar] [CrossRef] [PubMed]
- Forn-Díaz, P.; Lamata, L.; Rico, E.; Kono, J.; Solano, E. Ultrastrong coupling regimes of light-matter interaction. Rev. Mod. Phys. 2019, 91, 025005. [Google Scholar] [CrossRef] [Green Version]
- Frisk Kockum, A.; Miranowicz, A.; De Liberato, S.; Savasta, S.; Nori, F. Ultrastrong coupling between light and matter. Nat. Rev. Phys. 2019, 1, 19–40. [Google Scholar] [CrossRef] [Green Version]
- Liu, Y.Y.; Stehlik, J.; Eichler, C.; Gullans, M.J.; Taylor, J.M.; Petta, J.R. Semiconductor double quantum dot micromaser. Science 2015, 347, 285–287. [Google Scholar] [CrossRef] [Green Version]
- You, J.Q.; Liu, Y.X.; Sun, C.P.; Nori, F. Persistent single-photon production by tunable on-chip micromaser with a superconducting quantum circuit. Phys. Rev. B 2007, 75, 104516. [Google Scholar] [CrossRef] [Green Version]
- Quach, J.Q.; McGhee, K.E.; Ganzer, L.; Rouse, D.M.; Lovett, B.W.; Gauger, E.M.; Keeling, J.; Cerullo, G.; Lidzey, D.G.; Virgili, T. Superabsorption in an organic microcavity: Toward a quantum battery. Sci. Adv. 2022, 8, eabk3160. [Google Scholar] [CrossRef]
- Gemme, G.; Grossi, M.; Ferraro, D.; Vallecorsa, S.; Sassetti, M. IBM quantum platforms: A quantum battery perspective. Batteries 2022, 8, 43. [Google Scholar] [CrossRef]
- Joshi, J.; Mahesh, T.S. Experimental investigation of a quantum battery using star-topology NMR spin systems. Phys. Rev. A 2022, 106, 042601. [Google Scholar] [CrossRef]
- Hu, C.K.; Qiu, J.; Souza, P.J.P.; Yuan, J.; Zhou, Y.; Zhang, L.; Chu, J.; Pan, X.; Hu, L.; Li, J.; et al. Optimal charging of a superconducting quantum battery. Quantum Sci. Technol. 2022, 7, 045018. [Google Scholar] [CrossRef]
- Wenniger, I.M.d.B.; Thomas, S.E.; Maffei, M.; Wein, S.C.; Pont, M.; Harouri, A.; Lemaître, A.; Sagnes, I.; Somaschi, N.; Auffèves, A.; et al. Coherence-powered work exchanges between a solid-state qubit and light fields. arXiv 2022, arXiv:2202.01109. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shaghaghi, V.; Singh, V.; Carrega, M.; Rosa, D.; Benenti, G. Lossy Micromaser Battery: Almost Pure States in the Jaynes–Cummings Regime. Entropy 2023, 25, 430. https://doi.org/10.3390/e25030430
Shaghaghi V, Singh V, Carrega M, Rosa D, Benenti G. Lossy Micromaser Battery: Almost Pure States in the Jaynes–Cummings Regime. Entropy. 2023; 25(3):430. https://doi.org/10.3390/e25030430
Chicago/Turabian StyleShaghaghi, Vahid, Varinder Singh, Matteo Carrega, Dario Rosa, and Giuliano Benenti. 2023. "Lossy Micromaser Battery: Almost Pure States in the Jaynes–Cummings Regime" Entropy 25, no. 3: 430. https://doi.org/10.3390/e25030430
APA StyleShaghaghi, V., Singh, V., Carrega, M., Rosa, D., & Benenti, G. (2023). Lossy Micromaser Battery: Almost Pure States in the Jaynes–Cummings Regime. Entropy, 25(3), 430. https://doi.org/10.3390/e25030430








