Aggregated Power Indices for Measuring Indirect Control in Complex Corporate Networks with Float Shareholders
Abstract
1. Introduction
- The size of shareholding does not reflect the degree of shareholder control power over corporate matters. In other words, a shareholder can have substantially more or less corporate control than the shareholding percentage may suggest. Consider the example of a company with three shareholders: the first shareholder owns 70% of the shares, the second shareholder owns 20%, and the third shareholder owns the remaining 10% of the shares. If only shareholding size is examined, it would appear that the degree of control for each of the three investors is not in proportion to the percentage of their shareholdings, as the shareholder with 70% of shares controls this company fully. The situation may be even more complicated when the so-called right to block a majority coalition exists (the right of veto, which is established for other than economic reasons mostly). For example, in Poland, there is the concept of the so-called “golden share”, the possession of which allows you to block some decisions of the majority coalition. In the Volkswagen AG company, which is the basis of the example analyzed in [1], this role is played by a “4/5 rule”, which gives the State of Lower Saxony a blocking majority, as it controls more than 20% of the shares.
- There exists indirect control over companies when, for example, one fully controlled company is a majority shareholder of another company.
2. Notation and Definitions
2.1. Simple and Weighted Games
- value of an empty coalition is equal to zero: ;
- value of a grand coalition is equal to 1: v(N) = 1;
- (monotonicity) for all coalitions S and T, such that .
- , such that and , .
- , such that and , and .
2.2. Power Indices
2.2.1. Some Desirable Properties of Power Indices
2.2.2. Power Indices Considered in This Paper
- A group of power indices that satisfy the transfer property—also called the additivity property. To this group belong indices which follow Shapley and Shubik [2], absolute Banzhaf [21,49], Rae [50], Nevison [51], and Solidarity [45,52] indices. From this group of indices, we have chosen only one—the Solidarity index—for developing a group of power indices meant to represent the real power of the firms in a mutually complex shareholding network.
- A group of power indices that are based on minimal winning coalitions. These kinds of power indices were introduced by Deegan and Packel [53], Holler [54,55], Alonso-Meijide and Freixas [56], Alonso-Meijide, Freixas, and Molinero [57], and Felsenthal [58]. We take all indices from this group for further consideration, although this group is the most sensitive considering the postulate of local monotonicity. Namely, in this group of indices, only the PI index satisfies this property; see Felsenthal (2016) [58].
- A group of power indices that satisfy the null player removable property. In this group, we have all power indices from the previous group that relate to the minimal winning coalitions. The indices that are based on null player-free winning coalitions proposed by Álvarez-Mozos et al. [29], and the indices proposed by Banzhaf [49], Johnston [59], and Shapley and Shubik [2]. We take all these indices into consideration as well. Note that all indices in this group satisfy the null player property as well.
- The Shapley and Shubik [2] power index is defined as follows:
- The Deegan and Packel [53] power index is given as follows:
- The Shift index [56] is defined as follows:
- The Shift Deegan–Packel index [57] is defined as follows:
- The Felsenthal [58] power index PI of the winning coalitions of least size—proposed by Felsenthal (2016)—can be seen as a slight modification of the Deegan and Packel [53] index by replacing in its underlying assumptions of the minimal winning coalitions by the winning coalitions of least size (WCLS). The Felsenthal power index of WCLS for any player i, originally denoted by PI, is obtained as follows:
- The fn− power index [29] is defined as follows:
- The Álvarez-Mozos et al. [29] gn− power index is defined as follows:
- The Johnston [59] index is defined as follows:where for each vulnerable coalition, and critical player , and for non-critical players, .
2.3. The Karos and Peters Approach
3. A General Framework of Aggregated Power Indices for Indirect Control
3.1. Modelling of Corporate Shareholding Networks
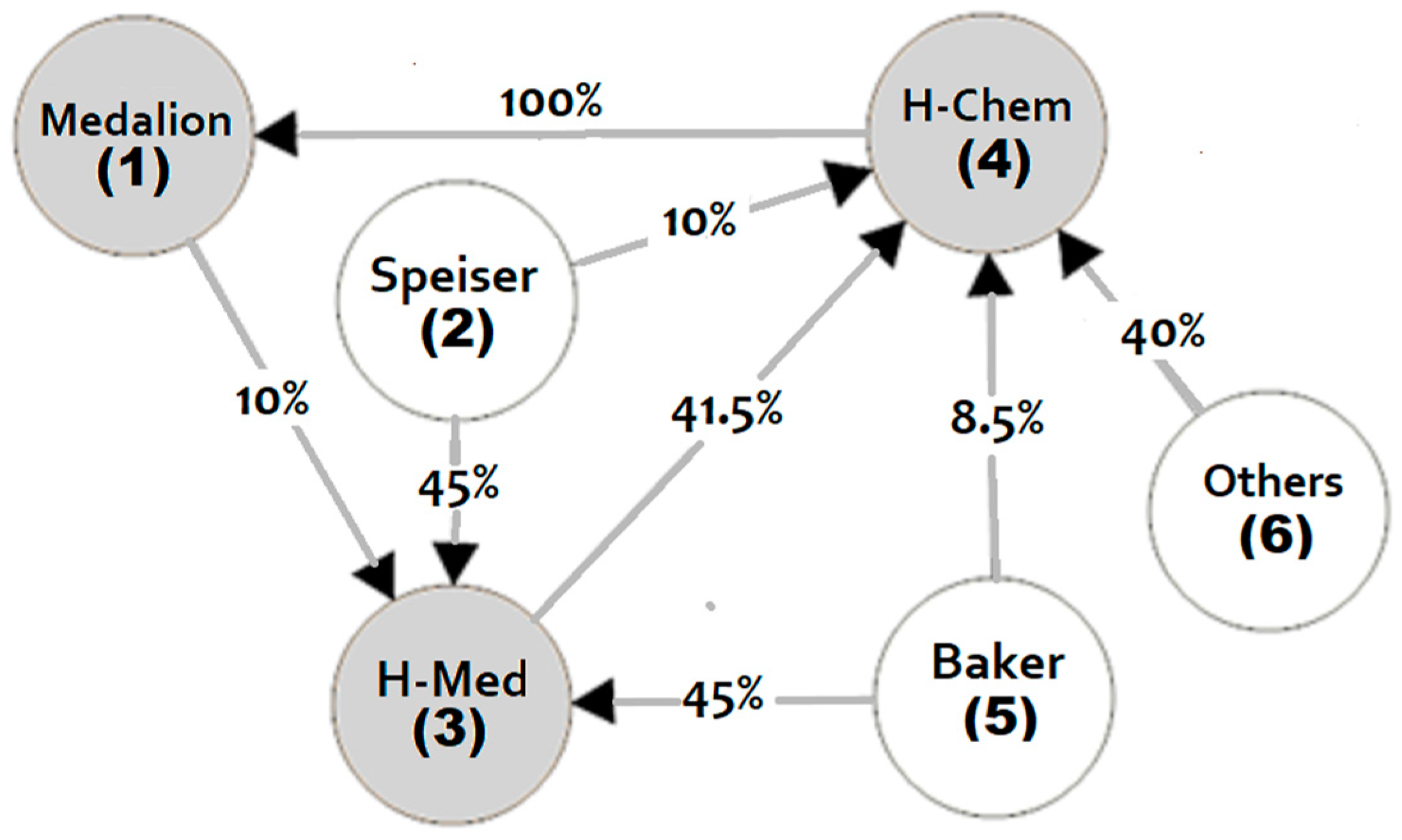
3.2. Motivation and Illustration
3.3. The Karos and Peters Index in the Example
3.4. Aggregated Power Indices a Generalization of the Karos and Peters Approach
3.4.1. The Aggregated Banzhaf Index
3.4.2. The Aggregated Solidarity Index
3.4.3. The Aggregated Deegan and Packel Index
3.4.4. The Aggregated Holler Index
3.4.5. The Aggregated Shift Index
3.4.6. The Aggregated Shift Deegan–Packel Index
3.4.7. The Aggregated PI Index
3.4.8. The Aggregated fn− Power Index
3.4.9. The Aggregated gn− Power Index
3.4.10. The Aggregated Johnston Power Index
3.5. The Float in Aggregated Power Indices
4. The Karos and Peters Approach with a Fuzzy Float
4.1. Basic Notions on Fuzzy Set Theory
4.2. The Karos and Peters Approach to a Fuzzy Float
| Algorithm 1 Defuzzification fuzzy weights |
| Step 1. Transform float shareholders’ weights expressed by the Z-fuzzy number Z = (A, B) into classical fuzzy numbers Z′ using the dictionary for the values of the second component B and Formulas (18)–(20). Step 2. Find a crisp equivalent of float shareholders’ weights, i.e., expected value E(Z′), using Formula (21). |
4.3. Karos and Peters Index for the Fuzzy Float for the Corporate Shareholding Network Which Deals with the Speiser and Baker Case
5. Discussion, Comparison, and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Karos, D.; Peters, H. Indirect control and power in mutual control structures. Games Econ. Behav. 2015, 92, 150–165. [Google Scholar] [CrossRef]
- Shapley, L.S.; Shubik, M. A method for evaluating the distribution of power in a committee system. Am. Polit. Sci. Rev. 1954, 48, 787–792. [Google Scholar] [CrossRef]
- Crama, Y.; Leruth, L. Power indices and the measurement of control in corporate structures. Int. Game Theory Rev. 2013, 15, 1340017-1–1340017-15. [Google Scholar] [CrossRef]
- Gambarelli, G.; Owen, G. Indirect control of corporations. Int. J. Game Theory 1994, 23, 287–302. [Google Scholar] [CrossRef]
- Turnovec, F. Privatization, ownership structure and transparency: How to measure the true involvement of the state. Eur. J. Political Econ. 1999, 15, 605–618. [Google Scholar] [CrossRef]
- Hu, X.; Shapley, L.S. On authority distributions in organizations: Controls. Games Econ. Behav. 2003, 45, 153–170. [Google Scholar] [CrossRef]
- Hu, X.; Shapley, L.S. On authority distributions in organizations: Equilibrium. Games Econ. Behav. 2003, 45, 132–152. [Google Scholar] [CrossRef]
- Leech, D. Shareholder voting power and ownership control of companies. In Power, Voting, and Voting Power: 30 Years After; Springer: Berlin/Heidelberg, Germany, 2013; Volume 19, pp. 345–371. [Google Scholar] [CrossRef]
- Crama, Y.; Leruth, L. Control and voting power in corporate networks: Concepts and computational aspects. Eur. J Oper. Res. 2007, 178, 879–893. [Google Scholar] [CrossRef]
- Mercik, J.; Łobos, K. Index of implicit power as a measure of reciprocal ownership. In Transactions on Computational Collective Intelligence XXIII. Lecture Notes in Computer Science; Nguyen, N.T., Kowalczyk, R., Mercik, J., Eds.; Springer: Berlin/Heidelberg, Germany, 2016; Volume 9760, pp. 128–140. [Google Scholar] [CrossRef]
- Levy, M.; Szafarz, A. Cross-ownership: A device for management entrenchment? Rev. Financ. 2017, 21, 1675–1699. [Google Scholar] [CrossRef]
- Mercik, J.; Stach, I. On measurement of control in corporate structures. In Transactions on Computational Collective Intelligence XXXI, Lecture Notes in Computer Science; Nguyen, N.T., Kowalczyk, R., Mercik, J., Motylska-Kuźma, A., Eds.; Springer: Berlin/Heidelberg, Germany, 2018; Volume 11290, pp. 64–79. [Google Scholar] [CrossRef]
- Stach, I.; Mercik, J.; Bertini, C. Some propositions of approaches for measuring indirect control power of firms and mutual connections in corporate shareholding structures. In Transactions on Computational Collective Intelligence XXXV, LNCS; Nguyen, N.T., Kowalczyk, R., Mercik, J., Motylska-Kuźma, A., Eds.; Springer: Berlin/Heidelberg, Germany, 2020; Volume 12330, pp. 116–132. [Google Scholar] [CrossRef]
- Staudacher, J.; Olsson, L.; Stach, I. Implicit power indices and software for measuring indirect control in corporate structures. In Transactions on Computational Collective Intelligence XXXVI, Lecture Notes in Computer Science; Nguyen, N.T., Kowalczyk, R., Motylska-Kuźma, A., Mercik, J., Eds.; Springer: Berlin/Heidelberg, Germany, 2021; Volume 13010, pp. 73–93. [Google Scholar] [CrossRef]
- Stach, I.; Mercik, J. Measurement of control power in corporate networks. Oper. Res. Decis. 2021, 31, 97–121. [Google Scholar] [CrossRef]
- Staudacher, J.; Olsson, L.; Stach, I. Algorithms for measuring indirect control in corporate networks and effects of divestment. In Transactions on Computational Collective Intelligence XXXVII, Lecture Notes in Computer Science; Nguyen, N.T., Kowalczyk, R., Mercik, J., Motylska-Kuźma, A., Eds.; Springer: Berlin/Heidelberg, Germany, 2022; Volume 13750, pp. 53–74. [Google Scholar] [CrossRef]
- Stach, I.; Mercik, J.; Bertini, C. Measuring voting power in complex shareholding structures: A Public Good Index approach. In Power and Responsibility: Interdisciplinary Perspectives for the 21st Century in Honor of Manfred J. Holler, 1st ed.; Leroch, M.A., Rupp, F., Eds.; Springer International Publishing: Cham, Switzerland, 2023; pp. 165–183. [Google Scholar] [CrossRef]
- Bertini, C.; Mercik, J.; Stach, I. Indirect control and power. Oper. Res. Decis. 2016, 26, 7–30. [Google Scholar] [CrossRef]
- Kołodziej, M.; Stach, I. Control Sharing Analysis and Simulation. In Conference Proceedings: ICIL 2016: 13th International Conference on Industrial Logistics, 28 September–1 October 2016, Zakopane, Poland; Sawik, T., Ed.; AGH University of Science and Technology: Krakow, Poland, 2016; pp. 101–108. [Google Scholar]
- Stach, I. Indirect control of corporations: Analysis and simulations. Decis. Mak. Manuf. Serv. 2017, 11, 31–51. [Google Scholar] [CrossRef]
- Penrose, L.S. The elementary statistics of majority voting. J. Roy. Stat. Soc. 1946, 109, 53–57. [Google Scholar] [CrossRef]
- Dubey, P.; Shapley, L.S. Mathematical properties of the Banzhaf power index. Math. Operations Res. 1979, 4, 99–131. [Google Scholar] [CrossRef]
- Mielcová, E. Weighted Voting Bodies under I-Fuzzy Settings. In Proceedings of the 2016 IEEE International Conference on Fuzzy Systems (FUZZ-IEEE), Vancouver, BC, Canada, 24–29 July 2016; pp. 1752–1759. [Google Scholar] [CrossRef]
- Mielcová, E. Shapley Value of Simple Cooperative Games with Fuzzy Coalitions Applied on the Real Voting Data. In Proceedings of the 30th International Conference Mathematical Methods in Economics, Karviná, Czech Republic, 11–13 September 2012; Ramík, J., Stavárek Karviná, D., Eds.; pp. 581–586. [Google Scholar]
- Przybyła-Kasperek, M. The power of agents in a dispersed system—The Shapley-Shubik power index. J. Parallel Distrib. Comput. 2021, 157, 105–124. [Google Scholar] [CrossRef]
- Pawlak, Z. Rought sets and fuzzy sets. Int. J. Fuzzy Sets Syst. 1985, 17, 99–102. [Google Scholar] [CrossRef]
- Stach, I. Proper simple game. In Encyclopedia of Power; Dowding, K., Ed.; SAGE Publications: Los Angeles, CA, USA, 2011; pp. 537–539. [Google Scholar]
- Stach, I.; Bertini, C. Reformulation of some indices using null player free winning coalitions. In Transactions on Computational Collective Intelligence XXXVI, Lecture Notes in Computer Science; Nguyen, N.T., Kowalczyk, R., Motylska-Kuźma, A., Mercik, J., Eds.; Springer: Berlin/Heidelberg, Germany, 2021; Volume 13010, pp. 108–115. [Google Scholar] [CrossRef]
- Álvarez-Mozos, M.; Ferreira, F.; Alonso-Meijide, J.M.; Pinto, A.A. Characterizations of power indices based on null player free winning coalitions. Optimization 2015, 64, 675–686. [Google Scholar] [CrossRef]
- Stach, I. Reformulation of Public Help Index θ using null player free winning coalitions. Group Decis. Negot. 2022, 31, 317–334. [Google Scholar] [CrossRef]
- Isbell, J.R. A class of simple games. Duke Math. J. 1958, 25, 423–439. [Google Scholar] [CrossRef]
- Felsenthal, D.; Machover, M. Voting power measurement: A story of misreinvention. Soc. Choice Welf. 2005, 25, 485–506. [Google Scholar] [CrossRef]
- Laruelle, A.; Martınez, R.; Valenciano, F. Success versus decisiveness conceptual discussion and case study. J. Theor. Politics 2006, 18, 185–205. [Google Scholar] [CrossRef]
- Gambarelli, G.; Stach, I. Power indices in politics: Some results and open problems. In Essays in Honor of Hannu Nurmi, Homo Oecon.; Holler, M.J., Widgrén, M., Eds.; Springer: Berlin/Heidelberg, Germany, 2009; Volume 26, pp. 417–441. [Google Scholar]
- Bertini, C.; Stach, I. Voting power. In Encyclopedia of Power; Dowding, K., Ed.; SAGE: Los Angeles, CA, USA, 2011; pp. 699–700. [Google Scholar]
- Bertini, C.; Freixas, J.; Gambarelli, G.; Stach, I. Comparing power indices. Int. Game Theory Rev. 2013, 15, 1340004-1–1340004-19. [Google Scholar] [CrossRef]
- Bertini, C.; Gambarelli, G.; Stach, I. Some open problems in the application of power indices to politics and finance. In Future of Power Indices, Special Issue of Homo Oecon; Holler, M., Nurmi, H., Eds.; Accedo Verlagsgesellschaft: München, Germany, 2015; Volume 32, pp. 147–156. [Google Scholar]
- Bertini, C.; Stach, I. On public values and power indices. Decis. Mak. Manuf. Serv. 2015, 9, 9–25. [Google Scholar] [CrossRef]
- Stach, I. Power measures and public goods. In Transactions on Computational Collective Intelligence XXIII, Lecture Notes in Computer Science 9760; Nguyen, N., Kowalczyk, R., Mercik, J., Eds.; Springer: Berlin/Heidelberg, Germany, 2016; pp. 99–110. [Google Scholar] [CrossRef]
- Bertini, C.; Gambarelli, G.; Stach, I. Indici di potere in politica e in finanza (Power indices in politics and finance). Boll. Docenti Mat. 2016, 72, 9–34. [Google Scholar]
- Bertini, C.; Gambarelli, G.; Stach, I.; Zola, M. Some results and open problems in applications of cooperative games. Int. J. Econ. Manag. Syst. 2017, 2, 271–276. [Google Scholar]
- Bertini, C.; Gambarelli, G.; Stach, I.; Zola, M. Power indices for finance. In Studies in Fuzziness and Soft-Computing; Soft Computing Applications for Group Decision-Making and Consensus Modeling; Collan, M., Kacprzyk, J., Eds.; Springer: Cham, Switzerland, 2018; Volume 357, pp. 45–70. [Google Scholar] [CrossRef]
- Bertini, C.; Gambarelli, G.; Stach, I.; Zola, M. The Shapley-Shubik index for finance and politics. In Handbook of the Shapley Value, 1st ed.; Algaba, E., Fragnelli, V., Sánchez-Soriano, J., Eds.; Taylor&Francis Group, Chapman and Hall/CRC: New York, NY, USA, 2020; pp. 393–417. [Google Scholar] [CrossRef]
- Staudacher, J.; Kóczy, L.A.; Stach, I.; Filipp, J.; Kramer, M.; Noffke, T.; Olsson, L.; Pichler, J.; Singer, T. Computing power indices for weighted voting games via dynamic programming. Oper. Res. Decis. 2021, 31, 123–145. [Google Scholar] [CrossRef]
- Stach, I.; Bertini, C. Solidarity Measures. In Transactions on Computational Collective Intelligence XXXVII, Lecture Notes in Computer Science; Nguyen, N.T., Kowalczyk, R., Mercik, J., Motylska-Kuźma, A., Eds.; Springer: Berlin/Heidelberg, Germany, 2022; Volume 13750, pp. 89–105. [Google Scholar] [CrossRef]
- Dubey, P. On the uniqueness of the Shapley value. Int. J. Game Theory 1975, 4, 131–139. [Google Scholar] [CrossRef]
- Shapley, L.S. A value for n-person games. In Contributions to the Theory of Games II; Kuhn, H., Tucker, A., Eds.; Princeton University Press: Princeton, NJ, USA, 1953; pp. 307–317. [Google Scholar] [CrossRef]
- Laruelle, A.; Valenciano, F. Shapley–Shubik and Banzhaf indices revisited. Math. Operations Res. 2001, 26, 89–104. [Google Scholar] [CrossRef]
- Banzhaf, J.F. Weighted voting doesn’t work: A mathematical analysis. Rutgers Law Rev. 1965, 19, 317–343. [Google Scholar]
- Rae, D. Decision rules and individual values in constitutional choice. Am. Political Sci. Rev. 1969, 63, 40–56. [Google Scholar] [CrossRef]
- Nevison, C.H. Structural power and satisfaction in simple games. In Applied Game Theory; Springer: Berlin/Heidelberg, Germany, 1979; pp. 39–57. [Google Scholar] [CrossRef]
- Nowak, A.S.; Radzik, T. A solidarity value for n-person transferable utility games. Int. J. Game Theory 1994, 23, 43–48. [Google Scholar] [CrossRef]
- Deegan, J.; Packel, E.W. A new index of power for simple n–person games. Int. J. Game Theory 1978, 7, 113–123. [Google Scholar] [CrossRef]
- Holler, M. Forming coalitions and measuring voting power. Political Stud. 1982, 30, 262–271. [Google Scholar] [CrossRef]
- Holler, M.J. The Public Good index: A brief introduction. In Finnish-German Yearbook of Political Economy; 2018; Volume 1, Available online: https://ssrn.com/abstract=3598861 (accessed on 26 January 2023).
- Alonso-Meijide, J.M.; Freixas, J. A new power index based on minimal winning coalitions without any surplus. Decis. Support Syst. 2010, 49, 70–76. [Google Scholar] [CrossRef]
- Alonso-Meijide, J.M.; Freixas, J.; Molinero, X. Computation of several power indices by generating functions. Appl. Math. Comput. 2012, 219, 3395–3402. [Google Scholar] [CrossRef]
- Felsenthal, D. A well-behaved index of a priori P-power for simple n-person games. Homo Oecon 2016, 33, 367–381. [Google Scholar] [CrossRef]
- Johnston, R.J. On the measurement of power: Some reactions to Laver. Environ. Plan. A 1978, 10, 907–914. [Google Scholar] [CrossRef]
- Bertini, C.; Gambarelli, G.; Stach, I. A Public Help Index. In Power, Freedom, and Voting; Braham, M., Steffen, F., Eds.; Springer: Berlin/Heidelberg, Germany, 2008; pp. 83–98. [Google Scholar] [CrossRef]
- Karos, D.; Peters, H. Indirect Control and Power in Mutual Control Structures; GSBE Research Memorandum 048; University of Maastricht: Maastricht, The Netherlands, 2013; pp. 1–26. [Google Scholar]
- Levy, M. The Banzhaf index in complete and incomplete shareholding structures: A new algorithm. Eur. J. Oper. Res. 2011, 215, 411–421. [Google Scholar] [CrossRef]
- Leech, D. Power indices in large voting bodies. Public Choice 2013, 155, 61–79. [Google Scholar] [CrossRef]
- Zadeh, L.A. Fuzzy sets. Inf. Control. 1965, 8, 338–353. [Google Scholar] [CrossRef]
- Dubois, D.; Prade, H. Possibility Theory: An Approach to Computerized Processing of Uncertainty; Plenum Press: New York, NY, USA, 1988. [Google Scholar] [CrossRef]
- Zadeh, L.A. Fuzzy sets as a basis of theory of possibility. Fuzzy Sets Syst. 1978, 1, 3–28. [Google Scholar] [CrossRef]
- Dubois, D.; Prade, H. Algorithmes de plus courts Chemins pour traiter des donnees floues. RAIRO Rech. Oper. /Oper. Res. 1978, 12, 213–227. [Google Scholar] [CrossRef]
- Zadeh, L.A. The concept of a linguistic variable and its application to approximate reasoning. Inf. Sci. 1975, 8, 199–249. [Google Scholar] [CrossRef]
- Carlsson, C.; Fullér, R. On possibilistic mean value and variance of fuzzy variable. Fuzzy Sets Syst. 2001, 122, 315–326. [Google Scholar] [CrossRef]
- Chanas, S.; Nowakowski, M. Single value simulation of fuzzy variable. Fuzzy Sets Syst. 1988, 5, 43–57. [Google Scholar] [CrossRef]
- Zadeh, L.A. A Note on Z-Numbers. Inf. Sci. 2011, 181, 2923–2932. [Google Scholar] [CrossRef]
- Azadeh, A.; Kokabi, R. Z-number DEA: A new possibilistic DEA in the context of Z-numbers. Adv. Eng. Inform. 2016, 30, 604–617. [Google Scholar] [CrossRef]
- Aliev, R.A.; Alizadeh, A.V.; Huseynov, O.H. An introduction to the arithmetic of Z-numbers by using horizontal membership functions. Procedia Comput. Sci. 2017, 120, 349–356. [Google Scholar] [CrossRef]
- Gündoğdu, F.K.; Kahraman, C. Spherical fuzzy sets and spherical fuzzy TOPSIS method. J. Intell. Fuzzy Syst. 2019, 36, 337–352. [Google Scholar] [CrossRef]
- Kang, B.; Wei, D.; Li, Y.; Deng, Y. A method of converting Z-number to classical fuzzy number. J. Inf. Comput. Sci. 2012, 9, 703–709. [Google Scholar]
- Mohamad, D.; Shaharani, S.A.; Kamis, N.H. A Z-Number-Based Decision Making Procedure with Ranking Fuzzy Numbers Method. In Proceedings of the 3rd International Conference on Quantitative Sciences and Its Applications, Langkawi Kedah, Malaysia, 12–14 August 2014. [Google Scholar]
- Freixas, J.; Marciniak, D.; Pons, M. On the ordinal equivalence of the Johnston, Banzhaf and Shapley-Shubik power indices. Eur. J. Oper. Res. 2012, 216, 367–375. [Google Scholar] [CrossRef]
- Freixas, J.; Kaniovski, S. The minimum sum representation as an index of voting power. Eur. J. Oper. Res. 2014, 233, 739–748. [Google Scholar] [CrossRef]
| Simple Game vi | Player 1 | Player 2 | Player 3 | Player 4 | Player 5 | Player 6 |
|---|---|---|---|---|---|---|
| i = 1 | 4/60 | 12/60 | 7/60 | 27/60 | 4/60 | 6/60 |
| i = 3 | 7/60 | 22/60 | 4/60 | 7/60 | 19/60 | 1/60 |
| i = 4 | 4/60 | 24/60 | 9/60 | 4/60 | 6/60 | 13/60 |
| i = 2, 5, 6 | 0 | 0 | 0 | 0 | 0 | 0 |
| Total | 15/60 | 58/60 | 20/60 | 38/60 | 29/60 | 20/60 |
| Game vi | Player 1 | Player 2 | Player 3 | Player 4 | Player 5 | Player 6 |
|---|---|---|---|---|---|---|
| i = 1 | 3/38 | 9/38 | 5/38 | 13/38 | 3/38 | 5/38 |
| i = 3 | 5/46 | 17/46 | 3/46 | 5/46 | 15/46 | 1/46 |
| i = 4 | 3/48 | 19/48 | 7/48 | 3/48 | 5/48 | 11/48 |
| Total | 5247/20976 | 21023/20976 | 7187/20976 | 10767/20976 | 10681/20976 | 8023/20976 |
| Simple Game vi | Player 1 | Player 2 | Player 3 | Player 4 | Player 5 | Player 6 |
|---|---|---|---|---|---|---|
| i = 1 | 465/3600 | 615/3600 | 525/3600 | 1035/3600 | 465/3600 | 495/3600 |
| i = 3 | 558/3600 | 804/3600 | 498/3600 | 558/3600 | 744/3600 | 438/3600 |
| i = 4 | 503/3600 | 839/3600 | 593/3600 | 503/3600 | 533/3600 | 629/3600 |
| Total | 1526/3600 | 2258/3600 | 1616/3600 | 2096/3600 | 1742/3600 | 1562/3600 |
| Simple Game vi | Player 1 | Player 2 | Player 3 | Player 4 | Player 5 | Player 6 |
|---|---|---|---|---|---|---|
| i = 1 | 5/36 | 9/36 | 6/36 | 6/36 | 5/36 | 5/36 |
| i = 3 | 6/42 | 12/42 | 5/42 | 6/42 | 11/42 | 2/42 |
| i = 4 | 5/42 | 12/42 | 6/42 | 5/42 | 7/42 | 7/42 |
| Total | 101/252 | 207/252 | 108/252 | 108/252 | 143/252 | 89/252 |
| Simple Game vi | Player 1 | Player 2 | Player 3 | Player 4 | Player 5 | Player 6 |
|---|---|---|---|---|---|---|
| i = 1 | 2/12 | 3/12 | 2/12 | 1/12 | 2/12 | 2/12 |
| i = 3 | 2/15 | 4/15 | 2/15 | 2/15 | 4/15 | 1/15 |
| i = 4 | 2/16 | 4/16 | 2/16 | 2/16 | 3/16 | 3/16 |
| Total | 102/240 | 184/240 | 102/240 | 82/240 | 149/240 | 101/240 |
| Simple Game vi | Player 1 | Player 2 | Player 3 | Player 4 | Player 5 | Player 6 |
|---|---|---|---|---|---|---|
| i = 1 | 2/10 | 2/10 | 1/10 | 1/10 | 2/10 | 2/10 |
| i = 3 | 1/9 | 1/9 | 2/9 | 1/9 | 3/9 | 1/9 |
| i = 4 | 2/12 | 2/12 | 1/12 | 2/12 | 2/12 | 3/12 |
| Total | 86/180 | 86/180 | 73/180 | 68/180 | 126/180 | 101/180 |
| Simple Game vi | Player 1 | Player 2 | Player 3 | Player 4 | Player 5 | Player 6 |
|---|---|---|---|---|---|---|
| i = 1 | 5/30 | 6/30 | 3/30 | 6/30 | 5/30 | 5/30 |
| i = 3 | 3/24 | 3/24 | 5/24 | 3/24 | 8/24 | 2/24 |
| i = 4 | 5/30 | 6/30 | 3/30 | 5/30 | 4/30 | 7/30 |
| Total | 55/120 | 63/120 | 49/120 | 59/120 | 76/120 | 58/120 |
| Simple Game vi | Player 1 | Player 2 | Player 3 | Player 4 | Player 5 | Player 6 |
|---|---|---|---|---|---|---|
| i = 1 | 0 | 0 | 0 | 1 | 0 | 0 |
| i = 3 | 2/12 | 4/12 | 1/12 | 2/12 | 3/12 | 0 |
| i = 4 | 1/10 | 4/10 | 2/10 | 1/10 | 1/10 | 1/10 |
| Total | 16/60 | 44/60 | 17/60 | 76/60 | 21/60 | 6/60 |
| Game vi | Player 1 | Player 2 | Player 3 | Player 4 | Player 5 | Player 6 |
|---|---|---|---|---|---|---|
| i = 1 | 46/306 | 54/306 | 49/306 | 63/306 | 46/306 | 48/306 |
| i = 3 | 27/172 | 36/172 | 25/172 | 27/172 | 34/172 | 23/172 |
| i = 4 | 71/492 | 108/492 | 81/492 | 71/492 | 75/492 | 86/492 |
| Total | 487270/D * | 653076/D * | 507232/D * | 547212/D * | 539953/D * | 502125/D * |
| Game vi | Player 1 | Player 2 | Player 3 | Player 4 | Player 5 | Player 6 |
|---|---|---|---|---|---|---|
| i = 1 | 27/172 | 30/172 | 28/172 | 32/172 | 27/172 | 28/172 |
| i = 3 | 24/152 | 30/152 | 23/152 | 24/152 | 29/152 | 22/152 |
| i = 4 | 22/147 | 30/147 | 24/147 | 22/147 | 23/147 | 26/147 |
| Total | 446318/D * | 553290/D * | 458655/D * | 474248/D * | 484459/D * | 465406/D * |
| Simple Game vi | Player 1 | Player 2 | Player 3 | Player 4 | Player 5 | Player 6 |
|---|---|---|---|---|---|---|
| i = 1 | 8/168 | 39/168 | 18/168 | 78/168 | 8/168 | 17/168 |
| i = 3 | 15/180 | 78/180 | 8/180 | 15/180 | 62/180 | 2/180 |
| i = 4 | 8/186 | 93/186 | 24/186 | 8/186 | 13/186 | 40/186 |
| Total | 13590/D * | 91047/D * | 21922/D * | 46140/D * | 36088/D * | 25573/D * |
| Simple Game vi | Player 1 | Player 2 | Player 3 | Player 4 | Player 5 |
|---|---|---|---|---|---|
| i = 1 | 1/7 | 1/7 | 2/7 | 2/7 | 1/7 |
| i = 3 | 1/11 | 4/11 | 1/11 | 1/11 | 4/11 |
| i = 4 | 1/9 | 2/9 | 3/9 | 1/9 | 2/9 |
| Total | 239/693 | 505/693 | 492/693 | 338/693 | 505/693 |
| Simple Game vi | Player 1 | Player 2 | Player 3 | Player 4 | Player 5 |
|---|---|---|---|---|---|
| i = 1 | 2/20 | 2/20 | 7/20 | 7/20 | 2/20 |
| i = 3 | 2/20 | 7/20 | 2/20 | 2/20 | 7/20 |
| i = 4 | 6/60 | 11/60 | 26/60 | 6/60 | 11/60 |
| Total | 18/60 | 38/60 | 53/60 | 33/60 | 38/60 |
| B | Fuzzy Reliability |
|---|---|
| Sure | (1, 0, 0.2) |
| Usually | (0.75, 0.1, 0.1) |
| Likely | (0.6, 0.1, 0.1) |
| Simple Fuzzy Game | 1 | 2 | 3 | 4 | 5 | 6 |
|---|---|---|---|---|---|---|
| Company 1 | 4/60 | 14/60 | 4/60 | 29/60 | 6/60 | 3/60 |
| Company 3 | 7/60 | 22/60 | 4/60 | 7/60 | 19/60 | 1/60 |
| Company 4 | 4/60 | 29/60 | 4/60 | 4/60 | 11/60 | 8/60 |
| Total | 15/60 | 65/60 | 12/60 | 40/60 | 36/60 | 12/60 |
| Coalition | Total Fuzzy Weight | Possibility That Coalition is Minimal Winning |
|---|---|---|
| {6} | (0, 0, 30.98) | 0 |
| {2, 6} | (10, 0, 30.98) | 0 |
| {3, 6} | (41.5, 0, 30.98) | 0.73 |
| {5, 6} | (8.5, 0, 30.98) | 0 |
| {2, 5, 6} | (18.5, 0, 30.98) | 0 |
| {3, 5, 6} | (50, 0, 30.98) | 0.27 |
| Index | Player 1 | Player 2 | Player 3 | Player 4 | Player 5 | Player 6 |
|---|---|---|---|---|---|---|
| Φ | −0.7500 | 0.9667 | −0.6667 | −0.3667 | 0.4833 | 0.3333 |
| Aβ | −0.7499 | 1.0022 | −0.6574 | −0.4867 | 0.5092 | 0.3825 |
| Aψ | −0.5761 | 0.6272 | −0.5511 | −0.4178 | 0.4839 | 0.4339 |
| AΔ | −0.5992 | 0.8214 | −0.5714 | −0.5714 | 0.5675 | 0.3532 |
| Ah | −0.5750 | 0.7667 | −0.5750 | −0.6583 | 0.6208 | 0.4208 |
| As | −0.5222 | 0.4778 | −0.5944 | −0.6222 | 0.7000 | 0.5611 |
| Aµ | −0.5417 | 0.5250 | −0.5917 | −0.5083 | 0.6333 | 0.4833 |
| API | −0.7333 | 0.7333 | −0.7167 | 0.2667 | 0.3500 | 0.1000 |
| Afn− | −0.5484 | 0.6053 | −0.5299 | −0.4928 | 0.5004 | 0.4654 |
| Agn− | −0.5355 | 0.5759 | −0.5226 | −0.5064 | 0.5042 | 0.4844 |
| Aγ | −0.8260 | 1.1654 | −0.7195 | −0.4094 | 0.4619 | 0.3274 |
| Φ with binary float | −0.7000 | 0.6333 | −0.1167 | −0.4500 | 0.6333 | – |
| Aβ with binary float | −0.6551 | 0.7287 | −0.2900 | −0.5123 | 0.7287 | – |
| Φ with fuzzy float | −0.7500 | 1.0833 | −0.8000 | −0.3333 | 0.6000 | 0.2000 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Stach, I.; Mercik, J.; Bertini, C.; Gładysz, B.; Staudacher, J. Aggregated Power Indices for Measuring Indirect Control in Complex Corporate Networks with Float Shareholders. Entropy 2023, 25, 429. https://doi.org/10.3390/e25030429
Stach I, Mercik J, Bertini C, Gładysz B, Staudacher J. Aggregated Power Indices for Measuring Indirect Control in Complex Corporate Networks with Float Shareholders. Entropy. 2023; 25(3):429. https://doi.org/10.3390/e25030429
Chicago/Turabian StyleStach, Izabella, Jacek Mercik, Cesarino Bertini, Barbara Gładysz, and Jochen Staudacher. 2023. "Aggregated Power Indices for Measuring Indirect Control in Complex Corporate Networks with Float Shareholders" Entropy 25, no. 3: 429. https://doi.org/10.3390/e25030429
APA StyleStach, I., Mercik, J., Bertini, C., Gładysz, B., & Staudacher, J. (2023). Aggregated Power Indices for Measuring Indirect Control in Complex Corporate Networks with Float Shareholders. Entropy, 25(3), 429. https://doi.org/10.3390/e25030429






