Parity-Time Symmetric Holographic Principle
Abstract
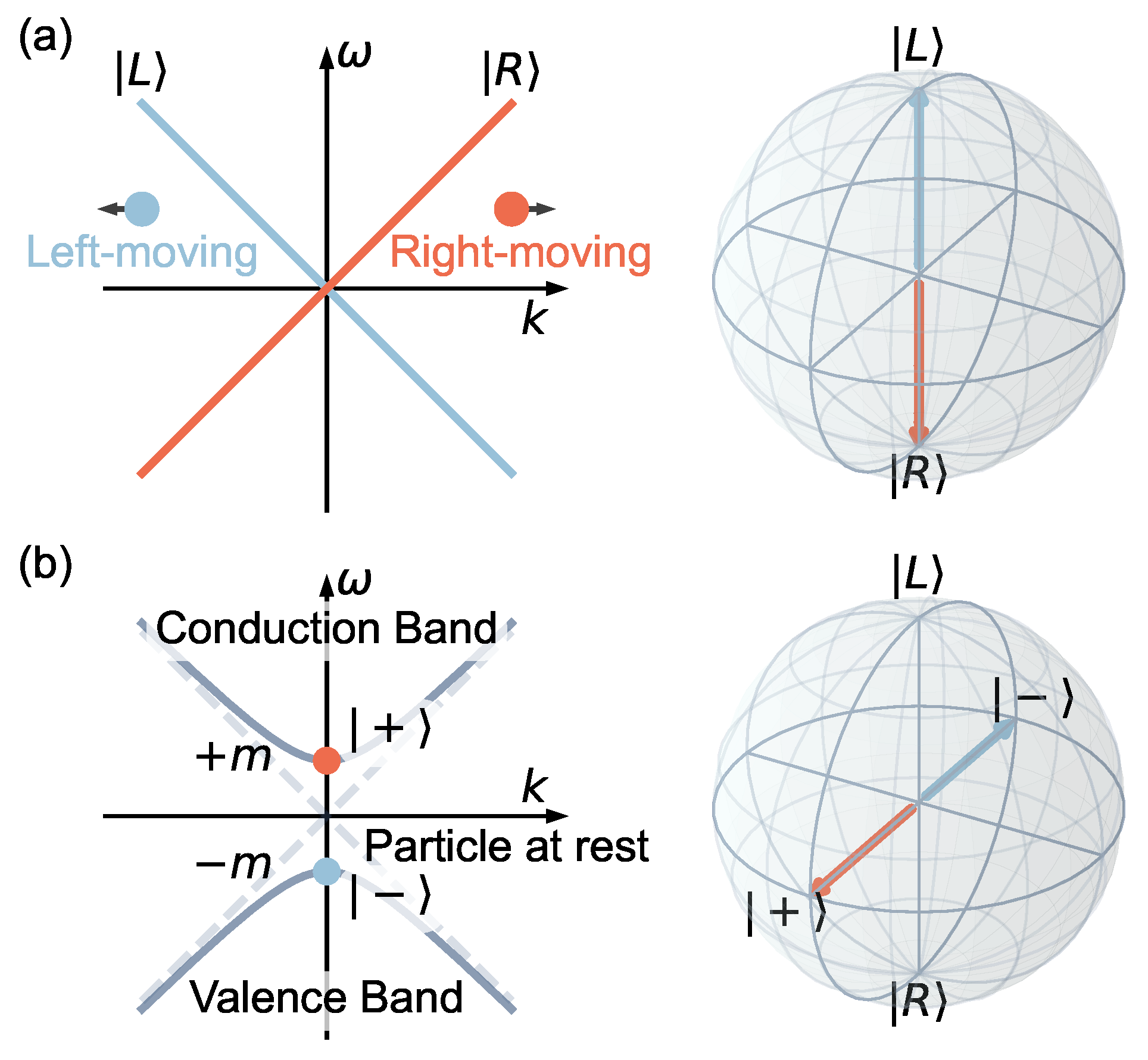
:1. Introduction
2. The Eigenvalue Problem and -Symmetric Evolution
3. Example 1: Scattering Phase Shifts
4. Example 2: Majorana Zero Mode
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Scattering Cross Section
References
- Feynman, R.P. Simulating physics with computers. Int. J. Theor. Phys. 1982, 21, 467–488. [Google Scholar] [CrossRef]
- DiVincenzo, D.P. Two-bit gates are universal for quantum computation. Phys. Rev. A 1995, 51, 1015–1022. [Google Scholar] [CrossRef] [PubMed]
- Barenco, A.; Bennett, C.H.; Cleve, R.; DiVincenzo, D.P.; Margolus, N.; Shor, P.; Sleator, T.; Smolin, J.A.; Weinfurter, H. Elementary gates for quantum computation. Phys. Rev. A 1995, 52, 3457–3467. [Google Scholar] [CrossRef] [PubMed]
- Arute, F.; Arya, K.; Babbush, R.; Bacon, D.; Bardin, J.C.; Barends, R.; Biswas, R.; Boixo, S.; Brandao, F.G.; Buell, D.A.; et al. Quantum supremacy using a programmable superconducting processor. Nature 2019, 574, 505–510. [Google Scholar] [CrossRef] [PubMed]
- Grover, L.K. Quantum mechanics helps in searching for a needle in a haystack. Phys. Rev. Lett. 1997, 79, 325–328. [Google Scholar] [CrossRef]
- Preskill, J. Quantum Computing in the NISQ era and beyond. Quantum 2018, 2, 79. [Google Scholar] [CrossRef]
- Breuer, H.P.; Petruccione, F. The Theory of Open Quantum Systems; Oxford University Press: Oxford, UK, 2007; Volume 9780199213900. [Google Scholar] [CrossRef]
- Koch, C.P. Controlling open quantum systems: Tools, achievements, and limitations. J. Phys. Condens. Matter 2016, 28, 213001. [Google Scholar] [CrossRef]
- Kapit, E. The upside of noise: Engineered dissipation as a resource in superconducting circuits. Quantum Sci. Technol. 2017, 2, 033002. [Google Scholar] [CrossRef]
- Diehl, S.; Micheli, A.; Kantian, A.; Kraus, B.; Büchler, H.P.; Zoller, P. Quantum states and phases in driven open quantum systems with cold atoms. Nat. Phys. 2008, 4, 878–883. [Google Scholar] [CrossRef]
- Shankar, S.; Hatridge, M.; Leghtas, Z.; Sliwa, K.M.; Narla, A.; Vool, U.; Girvin, S.M.; Frunzio, L.; Mirrahimi, M.; Devoret, M.H. Autonomously stabilized entanglement between two superconducting quantum bits. Nature 2013, 504, 419–422. [Google Scholar] [CrossRef]
- Harrington, P.M.; Mueller, E.; Murch, K. Engineered Dissipation for Quantum Information Science. arXiv 2022, arXiv:2202.05280. [Google Scholar] [CrossRef]
- Murch, K.W.; Vool, U.; Zhou, D.; Weber, S.J.; Girvin, S.M.; Siddiqi, I. Cavity-assisted quantum bath engineering. Phys. Rev. Lett. 2012, 109, 183602. [Google Scholar] [CrossRef] [PubMed]
- Harrington, P.M.; Naghiloo, M.; Tan, D.; Murch, K.W. Bath engineering of a fluorescing artificial atom with a photonic crystal. Phys. Rev. A 2019, 99, 052126. [Google Scholar] [CrossRef]
- Chen, W.; Özdemir, S.K.; Zhao, G.; Wiersig, J.; Yang, L. Exceptional points enhance sensing in an optical microcavity. Nature 2017, 548, 192–195. [Google Scholar] [CrossRef] [PubMed]
- Mi, X.; Ippoliti, M.; Quintana, C.; Greene, A.; Chen, Z.; Gross, J.; Arute, F.; Arya, K.; Atalaya, J.; Babbush, R.; et al. Time-crystalline eigenstate order on a quantum processor. Nature 2022, 601, 531–536. [Google Scholar] [CrossRef]
- Poyatos, J.F.; Cirac, J.I.; Zoller, P. Quantum reservoir engineering with laser cooled trapped ions. Phys. Rev. Lett. 1996, 77, 4728–4731. [Google Scholar] [CrossRef]
- Naghiloo, M.; Abbasi, M.; Joglekar, Y.N.; Murch, K.W. Quantum state tomography across the exceptional point in a single dissipative qubit. Nat. Phys. 2019, 15, 1232–1236. [Google Scholar] [CrossRef]
- Abbasi, M.; Chen, W.; Naghiloo, M.; Joglekar, Y.N.; Murch, K.W. Topological Quantum State Control through Exceptional-Point Proximity. Phys. Rev. Lett. 2022, 128, 160401. [Google Scholar] [CrossRef]
- Wu, Y.; Liu, W.; Geng, J.; Song, X.; Ye, X.; Duan, C.K.; Rong, X.; Du, J. Observation of parity-time symmetry breaking in a single-spin system. Science 2019, 364, 878–880. [Google Scholar] [CrossRef]
- Rüter, C.E.; Makris, K.G.; El-Ganainy, R.; Christodoulides, D.N.; Segev, M.; Kip, D. Observation of parity-time symmetry in optics. Nat. Phys. 2010, 6, 192–195. [Google Scholar] [CrossRef]
- Regensburger, A.; Bersch, C.; Miri, M.A.; Onishchukov, G.; Christodoulides, D.N.; Peschel, U. Parity-time synthetic photonic lattices. Nature 2012, 488, 167–171. [Google Scholar] [CrossRef] [PubMed]
- Peng, B.; Özdemir, S.K.; Rotter, S.; Yilmaz, H.; Liertzer, M.; Monifi, F.; Bender, C.M.; Nori, F.; Yang, L. Loss-induced suppression and revival of lasing. Science 2014, 346, 328–332. [Google Scholar] [CrossRef] [PubMed]
- El-Ganainy, R.; Makris, K.G.; Khajavikhan, M.; Musslimani, Z.H.; Rotter, S.; Christodoulides, D.N. Non-Hermitian physics and PT symmetry. Nat. Phys. 2018, 14, 11–19. [Google Scholar] [CrossRef]
- Makris, K.G.; El-Ganainy, R.; Christodoulides, D.N.; Musslimani, Z.H. Beam dynamics in PT symmetric optical lattices. Phys. Rev. Lett. 2008, 100, 103904. [Google Scholar] [CrossRef] [PubMed]
- Guo, A.; Salamo, G.J.; Duchesne, D.; Morandotti, R.; Volatier-Ravat, M.; Aimez, V.; Siviloglou, G.A.; Christodoulides, D.N. Observation of PT-symmetry breaking in complex optical potentials. Phys. Rev. Lett. 2009, 103, 093902. [Google Scholar] [CrossRef]
- Feng, L.; Xu, Y.L.; Fegadolli, W.S.; Lu, M.H.; Oliveira, J.E.; Almeida, V.R.; Chen, Y.F.; Scherer, A. Experimental demonstration of a unidirectional reflectionless parity-time metamaterial at optical frequencies. Nat. Mater. 2013, 12, 108–113. [Google Scholar] [CrossRef]
- Hodaei, H.; Hassan, A.U.; Wittek, S.; Garcia-Gracia, H.; El-Ganainy, R.; Christodoulides, D.N.; Khajavikhan, M. Enhanced sensitivity at higher-order exceptional points. Nature 2017, 548, 187–191. [Google Scholar] [CrossRef]
- Bender, C.M.; Boettcher, S. Real spectra in non-hermitian hamiltonians having PT symmetry. Phys. Rev. Lett. 1998, 80, 5243–5246. [Google Scholar] [CrossRef]
- Bender, C.M. Making sense of non-Hermitian Hamiltonians. Rep. Prog. Phys. 2007, 70, 947–1018. [Google Scholar] [CrossRef]
- Xiao, L.; Deng, T.; Wang, K.; Zhu, G.; Wang, Z.; Yi, W.; Xue, P. Non-Hermitian bulk–boundary correspondence in quantum dynamics. Nat. Phys. 2020, 16, 761–766. [Google Scholar] [CrossRef]
- Yao, S.; Wang, Z. Edge States and Topological Invariants of Non-Hermitian Systems. Phys. Rev. Lett. 2018, 121, 86803. [Google Scholar] [CrossRef] [PubMed]
- Kunst, F.K.; Edvardsson, E.; Budich, J.C.; Bergholtz, E.J. Biorthogonal Bulk-Boundary Correspondence in Non-Hermitian Systems. Phys. Rev. Lett. 2018, 121, 26808. [Google Scholar] [CrossRef] [PubMed]
- Weidemann, S.; Kremer, M.; Helbig, T.; Hofmann, T.; Stegmaier, A.; Greiter, M.; Thomale, R.; Szameit, A. Topological funneling of light. Science 2020, 368, 311–314. [Google Scholar] [CrossRef]
- Gong, Z.; Ashida, Y.; Kawabata, K.; Takasan, K.; Higashikawa, S.; Ueda, M. Topological Phases of Non-Hermitian Systems. Phys. Rev. X 2018, 8, 31079. [Google Scholar] [CrossRef]
- Kitaev, A.Y. Unpaired Majorana fermions in quantum wires. Physics-Uspekhi 2001, 44, 131–136. [Google Scholar] [CrossRef]
- Qi, X.L.; Zhang, S.C. Topological insulators and superconductors. Rev. Mod. Phys. 2011, 83, 1057. [Google Scholar] [CrossRef]
- Hasan, M.Z.; Kane, C.L. Colloquium: Topological insulators. Rev. Mod. Phys. 2010, 82, 3045. [Google Scholar] [CrossRef]
- Fu, L.; Kane, C.L. Superconducting proximity effect and majorana fermions at the surface of a topological insulator. Phys. Rev. Lett. 2008, 100, 096407. [Google Scholar] [CrossRef]
- Elliott, S.R.; Franz, M. Colloquium: Majorana fermions in nuclear, particle, and solid-state physics. Rev. Mod. Phys. 2015, 87, 137. [Google Scholar] [CrossRef]
- Heiss, W.D. The physics of exceptional points. J. Phys. Math. Theor. 2012, 45, 444016. [Google Scholar] [CrossRef]
- Miri, M.A.; Alù, A. Exceptional points in optics and photonics. Science 2019, 363, aar7709. [Google Scholar] [CrossRef] [PubMed]
- Lu, T.C.; Grover, T. Spacetime duality between localization transitions and measurement-induced transitions. PRX Quantum 2021, 2, 040319. [Google Scholar] [CrossRef]
- Joachain, C.J. Quantum Collision Theory; North-Holland: Amsterdam, The Netherlands, 1975; pp. 490–502. [Google Scholar]
- Varner, R.; Thompson, W.; McAbee, T.; Ludwig, E.; Clegg, T. A global nucleon optical model potential. Phys. Rep. 1991, 201, 57–119. [Google Scholar] [CrossRef]
- Dalibard, J.; Castin, Y.; Mølmer, K. Wave-function approach to dissipative processes in quantum optics. Phys. Rev. Lett. 1992, 68, 580. [Google Scholar] [CrossRef] [PubMed]
- Plenio, M.B.; Knight, P.L. The quantum-jump approach to dissipative dynamics in quantum optics. Rev. Mod. Phys. 1998, 70, 101. [Google Scholar] [CrossRef]
- Johansson, J.R.; Nation, P.D.; Nori, F. QuTiP: An open-source Python framework for the dynamics of open quantum systems. Comput. Phys. Commun. 2012, 183, 1760–1772. [Google Scholar] [CrossRef]
- Johansson, J.R.; Nation, P.D.; Nori, F. QuTiP 2: A Python framework for the dynamics of open quantum systems. Comput. Phys. Commun. 2013, 184, 1234–1240. [Google Scholar] [CrossRef]
- Mourik, V.; Zuo, K.; Frolov, S.M.; Plissard, S.R.; Bakkers, E.P.; Kouwenhoven, L.P. Signatures of Majorana Fermions in Topological Superconductor Nanowires. Science 2012, 336, 1003–1008. [Google Scholar] [CrossRef]
- Das, A.; Ronen, Y.; Most, Y.; Oreg, Y.; Heiblum, M.; Shtrikman, H. Zero-bias peaks and splitting in an Al-InAs nanowire topological superconductor as a signature of Majorana fermions. Nat. Phys. 2012, 8, 887–895. [Google Scholar] [CrossRef]
- Deng, M.T.; Vaitiekenas, S.; Hansen, E.B.; Danon, J.; Leijnse, M.; Flensberg, K.; Nygård, J.; Krogstrup, P.; Marcus, C.M. Majorana bound state in a coupled quantum-dot hybrid-nanowire system. Science 2016, 354, 1557–1562. [Google Scholar] [CrossRef]
- Lutchyn, R.M.; Bakkers, E.P.; Kouwenhoven, L.P.; Krogstrup, P.; Marcus, C.M.; Oreg, Y. Majorana zero modes in superconductor-semiconductor heterostructures. Nat. Rev. Mater. 2018, 3, 52–68. [Google Scholar] [CrossRef]
- Feynman, R.; Leighton, R.; Sands, M. The Feynman Lectures on Physics, Vol. III: The New Millennium Edition: Quantum Mechanics; The Feynman Lectures on Physics; Basic Books: New York, NY, USA, 2011. [Google Scholar]
- Srednicki, M. Quantum Field Theory; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, X.; Murch, K. Parity-Time Symmetric Holographic Principle. Entropy 2023, 25, 1523. https://doi.org/10.3390/e25111523
Song X, Murch K. Parity-Time Symmetric Holographic Principle. Entropy. 2023; 25(11):1523. https://doi.org/10.3390/e25111523
Chicago/Turabian StyleSong, Xingrui, and Kater Murch. 2023. "Parity-Time Symmetric Holographic Principle" Entropy 25, no. 11: 1523. https://doi.org/10.3390/e25111523
APA StyleSong, X., & Murch, K. (2023). Parity-Time Symmetric Holographic Principle. Entropy, 25(11), 1523. https://doi.org/10.3390/e25111523






