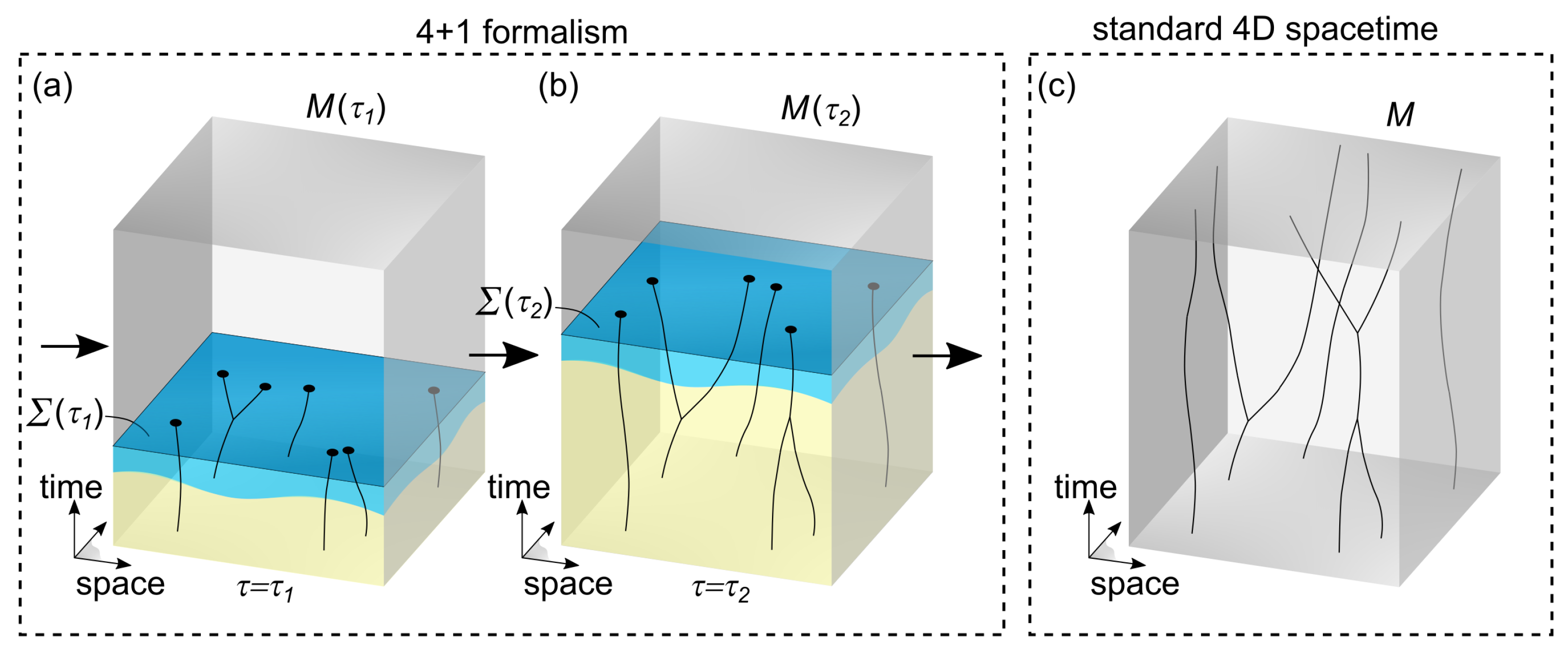
3.1. EPR Nonlocality in the 4+1 Formalism
EPR experiments demonstrate one of the most surprising features of quantum mechanics, namely, quantum nonlocality. Let us consider a typical EPR configuration with polarization-entangled photon pairs represented by the singlet state [
33,
34]:
where
and
are, respectively, the horizontal and vertical polarization and subscripts
A and
B refer to Alice’s and Bob’s photons. Measurements can be performed by Alice and Bob on their respective photons in spatially separated regions. For example, they can choose different measurement settings by rotating a polarizing beamsplitter (PBS) and can detect if a photon passes (outcome
A and
B) or is deflected (outcome
and
). If we assume that Alice measures first with PBS
set at an orientation
, then quantum theory suggests that the quantum state of Bob’s photon “instantaneously” adapts to Alice’s measurement result. If the photon passes through PBS
such that Alice observes outcome
A, the entangled system collapses into a state where both photon
A and photon
B have a polarization along
. In the opposite case that Alice observes outcome
, the polarization state of both photons collapses perpendicularly to
. After this, when Bob decides to measure his photon using his PBS
with chosen orientation
, he either observes that the photon passes (outcome
B) or is deflected (outcome
). Quantum theory predicts the following probabilities for joint detection at
A and
B [
34]:
This illustrates that the outcome of Bob’s experiment depends on the measurement setting of Alice, no matter how far they are separated (and vice versa). This is surprising since it seems to require an interaction going faster than light. These predictions of quantum theory have been confirmed in various EPR experiments [
9,
10,
15]. According to Bell’s theorem, the obtained quantum correlations cannot be explained with any hidden variable theory based on local realism [
35]. Since also a large class of nonlocal realist theories has been ruled out, it seems that realism is increasingly at risk [
8]. Furthermore, even though quantum nonlocality does not allow faster-than-light communication and does not violate relativity in this sense, there is still the difficulty of understanding the measurement process of the EPR experiment in a relativistic setting [
9,
36].
Next, we analyze whether one can make more sense of the EPR paradox in the 4+1 formalism.We follow up on the idea first proposed by Costa de Beauregard of influences traveling along worldlines, back to a common interaction event at an earlier time coordinate [
27,
28,
29]. This results in a relaxed measurement independence, which can be exploited to reproduce the nonlocal correlations of EPR experiments [
37,
38,
39]. However, like any retrocausal idea, also the idea of Costa de Beauregard conflicts with the causality of standard spacetime and is in need of a second form of time. Therefore, here, the idea of influences traveling along worldlines is embedded in the 4+1 formalism which features such a second time
.
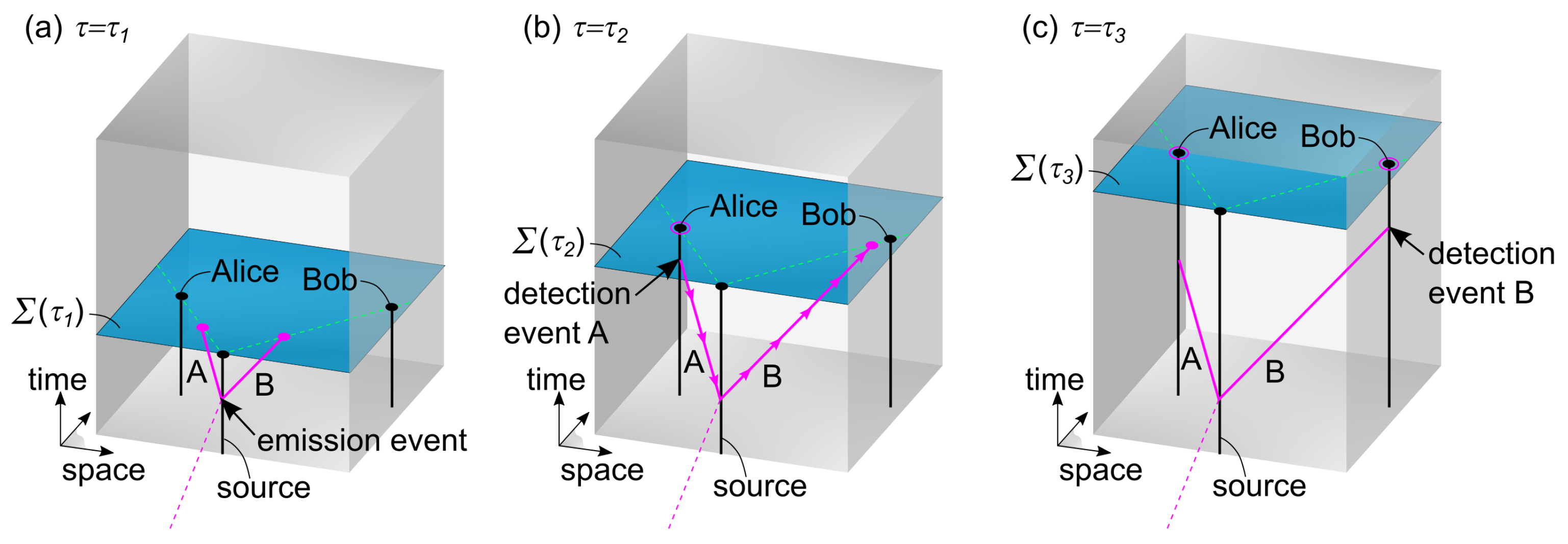
Firstly, the basic principle of the proposed EPR model in the 4+1 framework is illustrated in
Figure 2 at three values of the evolution parameter
. In essence, two entangled photons described using worldlines
A and
B are produced in an emission event and are traveling towards the measurement stations of Alice and Bob (see
Figure 2a). When Alice performs a measurement on photon
A, depending on the chosen setting for her PBS, the photon either passes or is deflected. As a consequence of Alice’s measurement, an influence travels backwards in the time dimension along worldline
A, to the emission event, and then along worldline
B as a function of
(indicated by arrows in
Figure 2b). In this way, influences can travel along worldlines to past regions of spacetime and to spatially distant regions in the present. Therefore, when Bob performs his measurement on photon
B, his outcome will depend on Alice’s outcome (see
Figure 2c).
Next, a more detailed EPR model in the 4+1 formalism is developed, and simulation results are shown in
Figure 3. As explained in
Section 2.1, a preferred frame is considered in which at each value of
the hypersurface
is characterized by a constant time
, with positive scalar
. When assigning a unit
to
, this means that
has the unit of
. For simplicity, we choose
. In this setting, each spacetime associated with a value of
may equally be identified by the time
expressed in seconds. The polarizing beamsplitters
and
are positioned, respectively, at distances
and
from the source
S. There are four detectors in total,
and
for Alice and
and
for Bob. Their positions are chosen such that the total path length
from source to each detector is the same:
. In
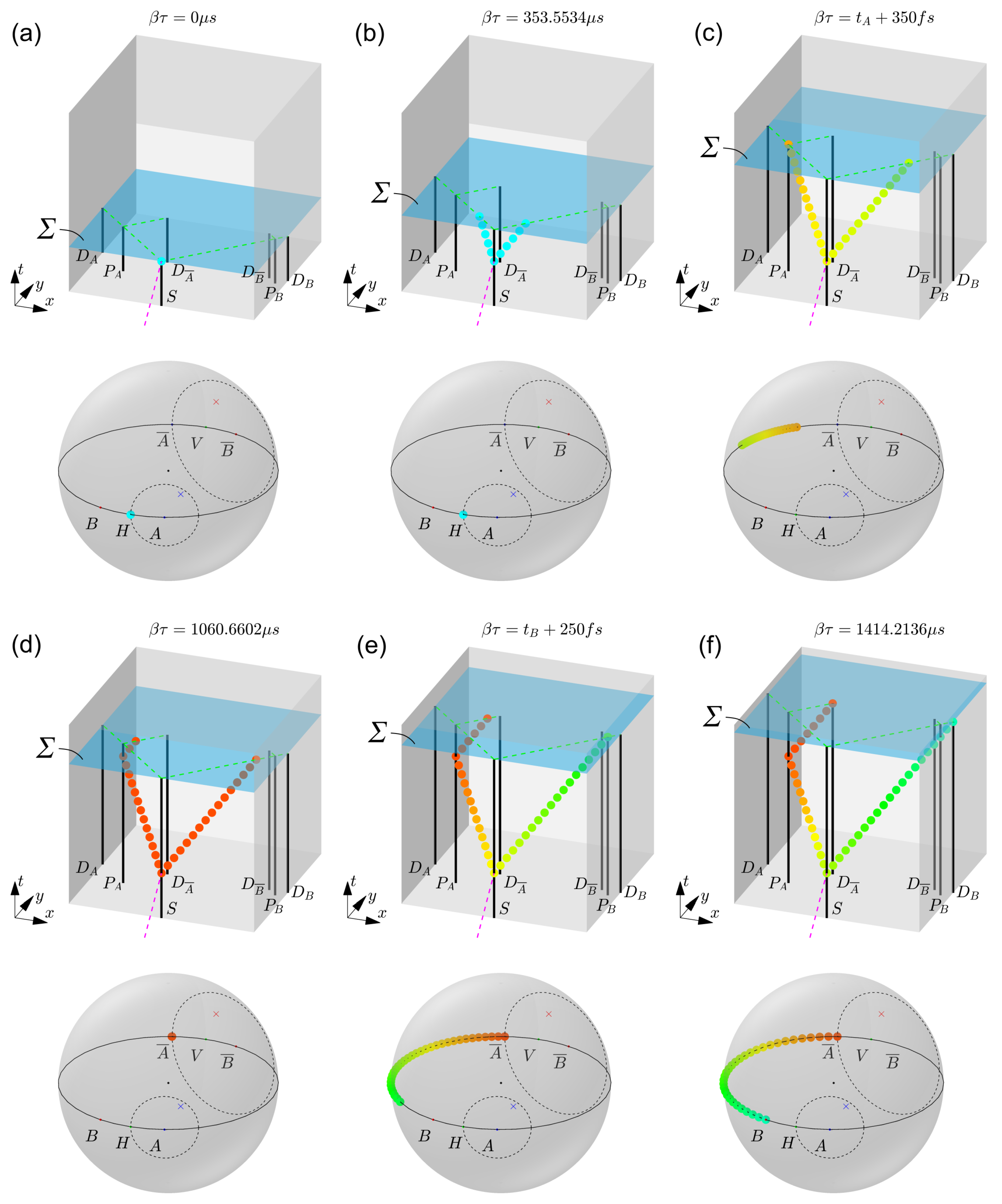
Figure 3,
=275.77 km,
=381.84 km, and
= 424.26 km, such that the time of flight for both photons is 1.414 ms. For six increasing values of
, the worldline configuration in spacetime is depicted (top) and the polarization state along the worldlines is represented on the Poincaré sphere (bottom).
We further restrict the analysis to the case in which two entangled photons are created corresponding to the state
at
s (see
Figure 3a). On the Poincaré sphere, both photons then correspond to the state
H. The other case of an initial state
can be treated in an analogous way. Two local hidden variables,
and
, which are defined as random points with a uniform distribution on the Poincaré sphere (respectively, the blue and red crosses in
Figure 3), are invoked to determine the outcome of each experiment.
At
s, the two entangled photons can be seen traveling under a 90° spatial angle towards Alice and Bob (see
Figure 3b). For each event on the photon worldlines
A and
B characterized by the affine parameter
, a polarization state
is defined by a point on the Poincaré sphere. For simplicity, the affine parameter
is chosen to be the 4D Cartesian distance
in the preferred frame along the photon worldline, starting at the interaction event near Alice and ending at the end event near Bob. The following evolution law is proposed for the polarization state:
where
K is a constant determining the strength of the fundamentally local interaction. Here, a value
is chosen. To visualize the polarization state in
Figure 3, at certain discrete events, circles are shown with hues corresponding to the azimuth angle, following the HSL color system.
At
s, the first photon reaches the PBS
of Alice. The interaction between photon
A and PBS
imposes a condition on the polarization state at this interaction event (replacing Equation (
3) at this event), governed via:
where
is chosen. Equation (
4) ensures that the polarization state at this event is attracted towards either state
or state
on the Poincaré sphere, with the physical consequence that the photon is, respectively, passing through or is deflected by the PBS. The outcome is decided by the factor
in Equation (
4). If
is situated outside of a circle centered around
passing through the state
, then this factor is +1 and the outcome of the interaction is
A. In the opposite case that
lies inside of this circle, the factor is −1 and the outcome is
. In
Figure 3c, the latter case is illustrated at an intermediate value
fs during the transition from state
H to state
. Notice how the rest of the worldline gradually adjusts to this boundary condition as a consequence of Equation (
3).
At about
fs, the complete worldline has aligned with the state
, so we can say that both photons
A and
B have collapsed to the outcome of Alice. As
increases further, photon
A is deflected by PBS
towards detector
, while photon
B continues on its path towards PBS
. This situation around
s is illustrated in
Figure 3d.
At
s, photon
B arrives at PBS
and the following evolution law is imposed at this interaction event:
Since in this example the hidden variable
is located inside the circle centered around
passing through
, the state of photon
B at the interaction event with PBS
is forced towards
. In
Figure 3e, the situation is shown at
fs, halfway through the transition of photon
B towards state
. This means that photon
B passes straight through PBS
towards detector
.
Finally,
Figure 3f shows the situation when photon
A arrives at detector
and photon
B at detector
, corresponding to an outcome
. A similar reasoning can be made for all other combinations of the initial state, polarizer settings, hidden variables, and different spatial geometries.
It can be readily verified that this scheme results in the desired quantum correlations given in Equation (
2). If the initial state of photon
A is
(in 50% of the cases), the probability for an outcome
A corresponds to the chance of finding the uniformly distributed hidden variable
outside of a circle on the Poincaré sphere with cone angle
around state
A. This chance can be calculated as
. Similarly, the chance for outcome
is
. If the initial state of photon
A is
(in the other 50% of the cases), then the chances of finding
A or
are, respectively,
and
. Together, this leads, as expected, to a 50% chance for
A and 50% chance for
. Right after Alice’s measurement, photon
B collapses to the same state as photon
A (which can have outcome
A or
). Assuming outcome
for Alice, the probability for outcome
B for Bob (i.e., passing through the PBS) corresponds to the chance of finding the uniformly distributed hidden variable
outside of a circle with opening angle
around state
B. Or similarly, this corresponds to the chance of finding
inside of the circle with cone angle
around the state
. This chance can be calculated as
. The overall probability of finding outcome
must take into account the 50% chance of getting state
to begin with, resulting in
, in agreement with the quantum prediction of Equation (
2). For all other combinations, the resulting correlations also agree with Equation (
2).
In the simulation of
Figure 3, the duration of the collapse of the polarization state of photon
B in the preferred frame is about 100 fs. This is ten orders of magnitude faster than the time (1.6 ms) needed for light to travel between PBS
and PBS
and much faster than the lower limit of around 10,000
c established using EPR tests [
40]. If needed,
K and
can be made arbitrarily large to achieve a quasi-instantaneous collapse.
Not only does this EPR model reproduce the desired quantum correlations; it also highlights some important features of the 4+1 formalism. Firstly, it is a fundamentally realist model, since it is based on really existing worldlines in a -dynamic spacetime. Secondly, it is fundamentally deterministic, since all interactions and dynamics are governed with differential equations orchestrated using the evolution parameter . And thirdly, the model is fundamentally local since each polarization state only depends on neighboring polarization states or on local interactions with a PBS. So, even though EPR correlations appear nonlocal by an observer interpreting the outcomes in ordinary spacetime, these correlations originate from fundamentally local processes at a deeper level of reality in the 4+1 formalism.
3.2. Quantum Measurement and the Arrival Time Problem in the 4+1 Formalism
To illustrate problems with quantum measurement, the arrival time, and momentum conservation in standard quantum theory, we consider the isotropic emission of a particle from a point source and its detection with two opposing hemispherical screens with different radii. For simplicity, the particle is emitted in a short pulse, resulting in a propagating wave function in the form of a thin spherically symmetrical shell. According to quantum theory, in good approximation, there is a 50% chance of detecting the particle on the closest detector D1 and 50% chance on the farthest detector D2. When the wave function pulse passes detector D1 at time , a partial collapse occurs in which it is decided if the particle is detected or not. In the case of detection, all momentum is transferred to detector D1, while an empty wave function travels further with no particular effect on detector D2. In the opposite case, there is no effect on detector D1, but then with 100% certainty, the particle will be measured by detector D2 at time .
This experiment highlights a number of problems of standard quantum theory. Firstly, the measurement of the particle by detector D1 (with 50% chance of detection) at
seems to have instantaneous consequences for the remaining, spatially separated part of the wave function, which is seemingly in conflict with relativity. This argument was first given by Einstein in his thought experiments called Einstein’s Boxes [
41]. It led Einstein to conclude that it may be useful to consider actual particle positions: “It seems to me that this difficulty cannot be overcome unless the description of the process in terms of the Schrödinger wave is supplemented by some detailed specification of the localization of the particle during its propagation” [
28]. Moreover, the collapse of the wave function at
is troubled by the violation of momentum conservation, as was already argued by Einstein in 1905: “In accordance with the assumption to be considered here, the energy of a light ray spreading out from a point source is not continuously distributed over an increasing space but consists of a finite number of energy quanta which are localized at points in space, which move without dividing, and which can only be produced and absorbed as whole units” [
42]. Hence, when assuming here that the isotropic wave function is associated with an isotropic momentum distribution, this leads to a conflict with conservation of momentum in the process of collapse when a measurement reveals the full particle momentum at detector D1 (or at D2). Bohmian mechanics has attempted to solve this problem by adding a hidden variable in the form of a particle position [
43]. In the context of the present isotropic wave function, such a strategy indeed works, but, as will be discussed in
Section 4, in other situations Bohmian mechanics does not offer a satisfying explanation for momentum conservation in free space [
44].
Secondly, by choosing the two detectors at different distances from the source, additional problems with time are highlighted. The so-called arrival time problem is a fundamental problem in quantum mechanics of defining the time that a particle is detected at a known position [
45,
46,
47]. And there are also other conceptual issues related to partial collapse at
and the resulting empty or full wave functions [
44,
48]. For example, the fact that the wave function can interact with detector D1 without setting it off, or that the continuing wave function then always leads to detection at D2, is hard to grasp in standard quantum mechanics. Again, this becomes much easier when assuming that there is a hidden property pointing to a specific particle location.
Within the 4+1 formalism, we can propose a simple model for the same experiment (see
Figure 4). Here, it is assumed that a single worldline carrying all the momentum of the particle is emitted in a random direction (see
Figure 4a). This worldline keeps its initial spatial orientation but simply grows as
shifts in the positive time dimension. Considering the simplicity of this model, no further evolution laws are needed. At
, it becomes very clear that a measurement via detector D1 only occurs if the worldline interacts directly with this detector (see
Figure 4b). If not, the worldline continues until it unavoidably interacts with detector D2 (see
Figure 4c). As a result, this simple model reproduces the expectation from quantum mechanics. In addition, it highlights the advantage of using worldlines in the 4+1 formalism for avoiding problems with quantum measurement. Namely, by relying on a single worldline that determines the outcome of the experiment, there is no measurement, collapse, or arrival time problem. And by insisting that this worldline is a geodesic between key interaction events, there is no conflict with conservation of momentum.
3.3. Interference, Wave–Particle Duality, and Superposition in the 4+1 Formalism
The quantum superposition of a particle is yet another key feature of quantum mechanics, with relevance to planned tests of quantum gravity in the laboratory. Here, a standard interferometry setup (Mach–Zehnder-type) with 50/50 beamsplitters is considered in which a photon is brought in a spatial superposition. We begin by repeating the standard quantum treatment of such interferometer. At the input of the interferometer, we consider the state
representing the polarization state of the incident photon. The two beamsplitters BS1 and BS2 are represented in the Jones matrix formalism by [
49]:
Hence, the quantum state after BS1 is given by:
where
represents the spatial state of branch A after reflection under a 90° spatial angle,
represents branch B after transmission, and
still captures the polarization state. Furthermore, in branch A, an excess path length can be introduced, which adds a phase
to this path. As a result, the state becomes:
From Equation (
8), we find, as expected, that the probability of measuring the photon in branch A or B (if a detector was placed there) is given by:
After BS2, the state can be rewritten in terms of the output ports
and
:
Detectors
and
only detect the component of the total state, respectively, in branches
C and
D. The quantum states arriving at these detectors are then given by:
Finally, this leads to the following expectation values at the detectors:
Two remarks can be made about this standard quantum result that are relevant for further analysis. Firstly, the probability of measuring a photon at one of the detectors (
or
) depends on both branches of the interferometer. This follows from the presence of
in Equation (
12). And it reveals an interference effect or wave-like behavior. Yet, each individual photon is only detected by one of both detectors, demonstrating particle-like behavior. This constitutes the well-known wave–particle duality. Secondly, quantum theory does not specify along which branch a particle actually travels but assumes instead that the particle ends up in a superposition of going either way. At present, it is not understood what the gravitational effect is of a particle in such a spatial superposition, since this requires a theory of quantum gravity. For example, it is not known from any experiment if the gravitational field also ends up in a superposition (if such a thing is possible) or if it behaves in some kind of classical way.
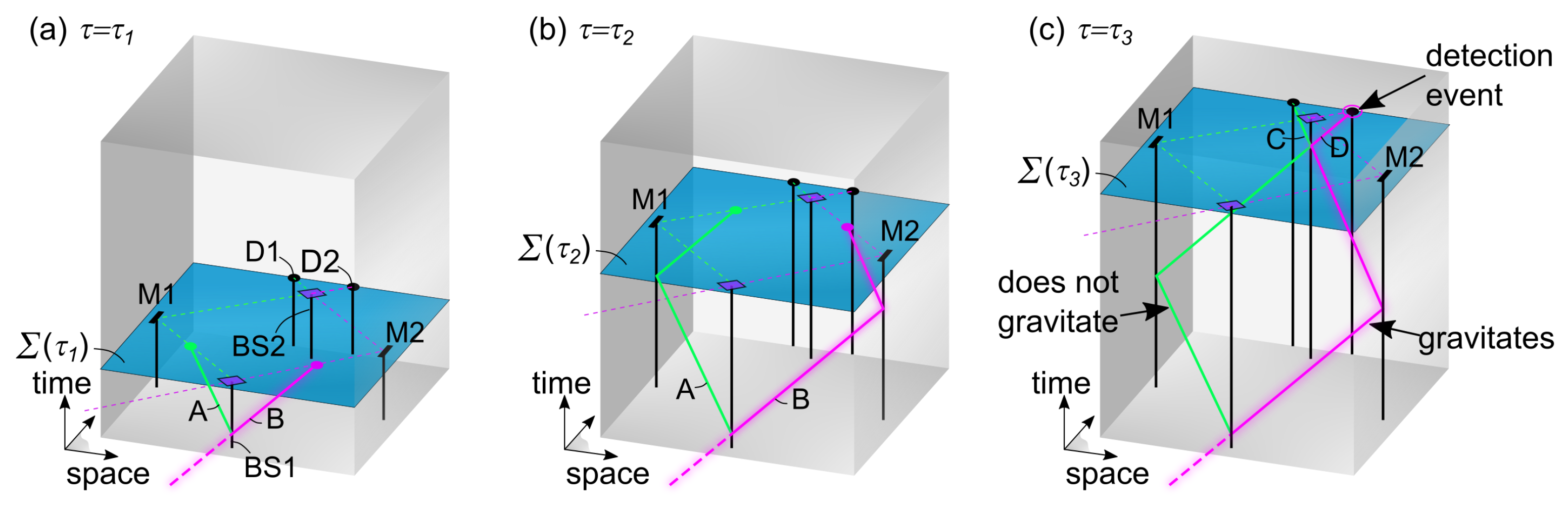
Next, a model for the Mach–Zehnder interferometer is developed within the 4+1 formalism (see
Figure 5). The interferometer setup is, for simplicity, chosen stationary in the preferred frame. Similar as for the EPR experiment,
identifies a single time coordinate
for each value of
.
Before entering the interferometer, each incident photon is modeled as a bundle of N (a large number) worldlines (null geodesics) called particle worldlines, labeled with index . Only one worldline (for which ), referred to as the momentum worldline, carries the energy and momentum of the photon. Each worldline of this incident bundle is further characterized by the same polarization and phase. As the evolution parameter increases, the hypersurface shifts forward in the time dimension. Consequently, worldlines belonging to all optical components of the interferometer and particle worldlines of the photon, which have their endpoints on the hypersurface , are growing.
Once the particle worldlines enter the interferometer, there are a number of optical elements with which these worldlines can interact, identifying key interaction events of the form . For example, represents the interaction event with BS1, and are interaction events with the mirrors, respectively, in branches A and B, and is the interaction event with BS2. Between key interaction events, worldlines are assumed to be straight (null geodesics). Each worldline can follow either branch A or B between BS1 and BS2 and either branch C or D after BS2. Next, we elaborate how the N worldlines are split up along these possible paths.
Let us first analyze the case just after interaction with beamsplitter BS1, when the endpoints of the photon worldlines are situated just after BS1 (see
Figure 5a). At each value of
, quantum mechanics provides two quantum measures
and
, respectively, at the endpoints of paths A and B on the hypersurface
, which are proportional to the probabilities
and
of finding the particle, respectively, along paths A or B (see Equation (
9)). In this simple geometry, these quantum measures can be established in a deterministic and local way along null geodesics following the path integral formalism. In the 4+1 framework, we are allowed to propagate this information backwards in time, respectively, along paths A and B in a fundamentally local way as a function of
. We further assume that this information is transferred much faster as a function of
compared to other relevant
-dynamics. As a result,
and
become available information at the past interaction event
almost immediately after the interaction with BS1 as a function of
. Hence, at the interaction event
, there is enough information to spatially separate the
N worldlines into two groups (along path A or path B) in a fundamentally local and deterministic way according to the following evolution law:
Here, for simplicity,
and
are modeled as continuous variables representing approximately the discrete numbers of worldlines aligned, respectively, along the paths A and B. The initial numbers of worldlines just after the interaction event are assumed to correspond to the numbers of incident worldlines traveling in the same direction before the interaction, i.e.,
and
. Equation (
13) implies that worldlines gradually shift between channels A and B as a function of
, until equilibrium is reached. The
-interval in which this reorganization occurs is set by the constant
, and this parameter is chosen sufficiently large that the reorganization occurs before any significant shift of
has occurred. Equation (
13) leads to an equilibrium governed by:
or equivalently, using the proportionality between
and
and between
and
(with same proportionality constant):
Therefore, the fractions of worldlines taking paths A or B become equal to the fractions of the corresponding quantum expectation values from Equation (
7).
If we assume that particle worldlines are randomly assigned to the two groups along branches A and B, then the probability that the momentum worldline labeled with index travels along branch A or B is also proportional to the number of worldlines in these respective channels and thus also proportional to the quantum expectation value of each channel. Since only the momentum worldline can interact with a detector and set it off, this means that the chance of detecting the photon in channel A or B agrees with the quantum prediction. An important difference with the standard quantum description is that here a clear decision is made along which branch of the interferometer the particle momentum travels. If the momentum worldline follows branch A, a reflection occurs and the associated recoil momentum is transferred to the beamsplitter BS1. In the other case that the momentum worldline follows branch B (transmission), no momentum is transferred to BS1.
At larger values of
but still before interacting with BS2, the two bundles along paths A and B interact with optical systems (mirrors and an optical element that introduces a phase delay) (see
Figure 5b). This produces new interaction events without affecting the number of particle worldlines in branches A and B. Only the mirror that interacts with the momentum worldline actually receives a momentum recoil.
Next, we analyze what happens after interaction with beamsplitter BS2 (see
Figure 5c). Similar reasoning as was made for branches A and B is followed here for branches C and D, leading to the following evolution law:
with quantum measures
and
being proportional, respectively, to
and
from Equation (
12) (with same proportionality constant). Hence, Equation (
16) leads to the following equilibrium:
Similar as explained above, this leads to an outcome of the Mach–Zehnder experiment in agreement with quantum mechanics. The momentum recoil with BS2 at the interaction event is determined by the chosen path of the momentum worldline. In the case that a reflection of the momentum worldline occurs, momentum is transferred to BS2. Otherwise, no momentum is transferred.
The above model for the Mach–Zehnder interferometer highlights some interesting features of the 4+1 formalism. Firstly, it shows how worldlines can be spatially arranged as a function of
in a fundamentally local and deterministic way in order to reproduce quantum interference. Secondly, it shows how momentum transfer can be concentrated at local interaction events between the momentum worldline of the photon and worldlines of the interferometer, while in between interaction events the momentum worldline is a null geodesic that preserves momentum. Thirdly, it sheds light on wave–particle duality and superposition. Even though the model relies on many particle worldlines that pass along both branches A and B and that codetermine the output of the interferometer, only one of these worldlines actually carries the energy and momentum of the photon and can set off a detector. Therefore, here, a photon is envisioned as an ensemble of many worldlines that enable its wave-like features, but with only a single momentum-carrying worldline that enables its particle-like features. Whereas the standard concept of quantum superposition states that the photon ends up in a superposition of going either via branch A or via branch B, in the 4+1 formalism, one can say that some constituent worldlines of the photon do travel both ways but that the momentum-carrying worldline only takes a single path through the interferometer. In
Section 4, further consequences for laboratory tests of quantum gravity are discussed.