On the Fidelity Robustness of CHSH–Bell Inequality via Filtered Random States
Abstract
1. Introduction
2. Random Density Matrices
3. The CHSH–Bell Inequality
4. Typicality for the Value of
5. Fidelity between a High Nonclassical State and a Set of Neighbouring States
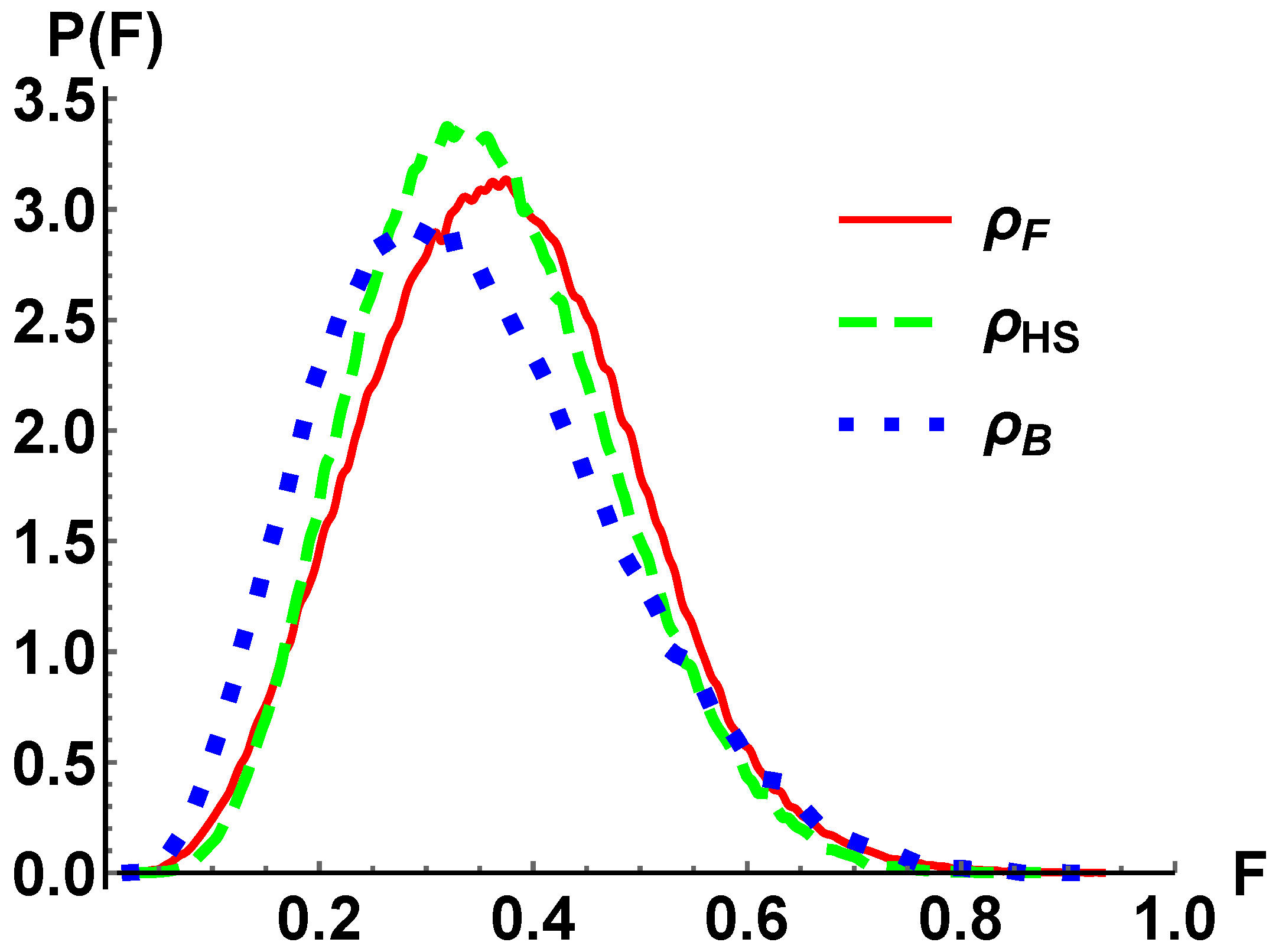
5.1. Fidelity between Two Quantum States
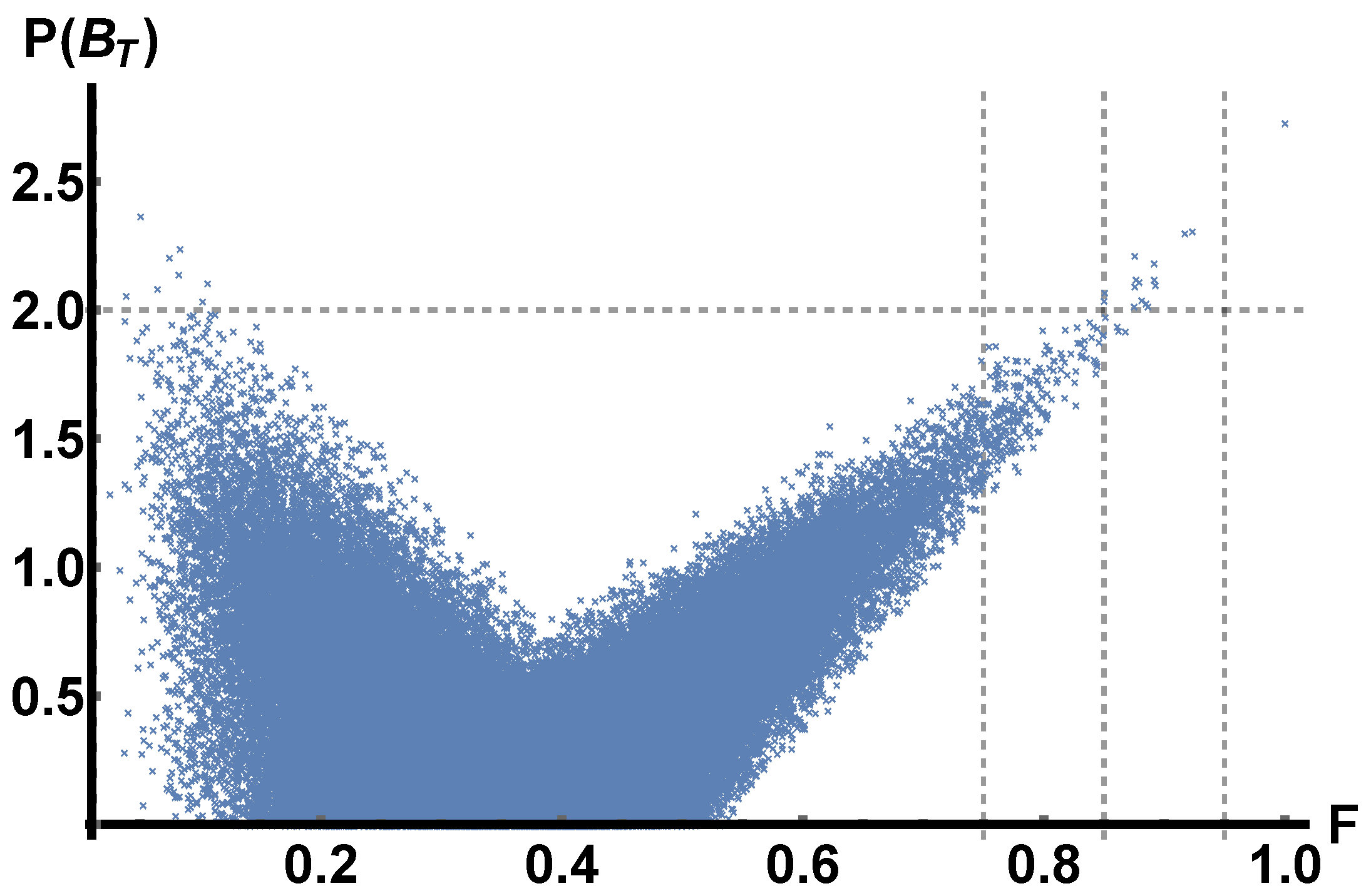
5.2. Bell-Nonclassicality vs. Fidelity
5.3. Realising the Fidelity Constraint
6. Discussion and Future Perspectives
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| CHSH | Clauser–Horne–Shimony–Holt |
| EPR | Einstein–Podolski–Rosen |
Appendix A. Target Bipartite State Considered
Appendix B. Tipicality of the Fidelity with the Bipartite State Considered
References
- Bell, J.S. On the Einstein Podolsky Rosen paradox. Phys. Phys. Fiz. 1964, 1, 195–200. [Google Scholar] [CrossRef]
- Freedman, S.J.; Clauser, J.F. Experimental Test of Local Hidden-Variable Theories. Phys. Rev. Lett. 1972, 28, 938–941. [Google Scholar] [CrossRef]
- Bohm, D. A Suggested Interpretation of the Quantum Theory in Terms of “Hidden” Variables. I. Phys. Rev. 1952, 85, 166–179. [Google Scholar] [CrossRef]
- Bohm, D. A Suggested Interpretation of the Quantum Theory in Terms of “Hidden” Variables. II. Phys. Rev. 1952, 85, 180–193. [Google Scholar] [CrossRef]
- Einstein, A.; Podolsky, B.; Rosen, N. Can Quantum-Mechanical Description of Physical Reality Be Considered Complete? Phys. Rev. 1935, 47, 777–780. [Google Scholar] [CrossRef]
- Aspect, A.; Dalibard, J.; Roger, G. Experimental Test of Bell’s Inequalities Using Time- Varying Analyzers. Phys. Rev. Lett. 1982, 49, 1804–1807. [Google Scholar] [CrossRef]
- Weihs, G.; Jennewein, T.; Simon, C.; Weinfurter, H.; Zeilinger, A. Violation of Bell’s Inequality under Strict Einstein Locality Conditions. Phys. Rev. Lett. 1998, 81, 5039–5043. [Google Scholar] [CrossRef]
- Rowe, M.A.; Kielpinski, D.; Meyer, V.; Sackett, C.A.; Itano, W.M.; Monroe, C.; Wineland, D.J. Experimental violation of a Bell’s inequality with efficient detection. Nature 2001, 409, 791–794. [Google Scholar] [CrossRef]
- Matsukevich, D.N.; Maunz, P.; Moehring, D.L.; Olmschenk, S.; Monroe, C. Bell Inequality Violation with Two Remote Atomic Qubits. Phys. Rev. Lett. 2008, 100, 150404. [Google Scholar] [CrossRef]
- Ansmann, M.; Wang, H.; Bialczak, R.C.; Hofheinz, M.; Lucero, E.; Neeley, M.; O’Connell, A.D.; Sank, D.; Weides, M.; Wenner, J.; et al. Violation of Bell’s inequality in Josephson phase qubits. Nature 2009, 461, 504–506. [Google Scholar] [CrossRef]
- Scheidl, T.; Ursin, R.; Kofler, J.; Ramelow, S.; Ma, X.S.; Herbst, T.; Ratschbacher, L.; Fedrizzi, A.; Langford, N.K.; Jennewein, T.; et al. Violation of local realism with freedom of choice. Proc. Natl. Acad. Sci. USA 2010, 107, 19708–19713. [Google Scholar] [CrossRef] [PubMed]
- Hofmann, J.; Krug, M.; Ortegel, N.; Gérard, L.; Weber, M.; Rosenfeld, W.; Weinfurter, H. Heralded Entanglement Between Widely Separated Atoms. Science 2012, 337, 72–75. [Google Scholar] [CrossRef] [PubMed]
- Hensen, B.; Bernien, H.; Dréau, A.E.; Reiserer, A.; Kalb, N.; Blok, M.S.; Ruitenberg, J.; Vermeulen, R.F.; Schouten, R.N.; Abellán, C.; et al. Loophole-free Bell inequality violation using electron spins separated by 1.3 kilometres. Nature 2015, 526, 682–686. [Google Scholar] [CrossRef] [PubMed]
- Shalm, L.K.; Meyer-Scott, E.; Christensen, B.G.; Bierhorst, P.; Wayne, M.A.; Stevens, M.J.; Gerrits, T.; Glancy, S.; Hamel, D.R.; Allman, M.S.; et al. Strong Loophole-Free Test of Local Realism. Phys. Rev. Lett. 2015, 115, 250402. [Google Scholar] [CrossRef] [PubMed]
- Giustina, M.; Versteegh, M.A.M.; Wengerowsky, S.; Handsteiner, J.; Hochrainer, A.; Phelan, K.; Steinlechner, F.; Kofler, J.; Larsson, J.A.; Abellán, C.; et al. Significant-Loophole-Free Test of Bell’s Theorem with Entangled Photons. Phys. Rev. Lett. 2015, 115, 250401. [Google Scholar] [CrossRef] [PubMed]
- Coopmans, T.; Kaniewski, J.; Schaffner, C. Robust self-testing of two-qubit states. Phys. Rev. A 2019, 99, 052123. [Google Scholar] [CrossRef]
- Šupić, I.; Bowles, J. Self-testing of quantum systems: A review. Quantum 2020, 4, 337. [Google Scholar] [CrossRef]
- Gigena, N.; Kaniewski, J. Quantum value for a family of I3322–like Bell functionals. Phys. Rev. A 2022, 106, 012401. [Google Scholar] [CrossRef]
- Gühne, O.; Hyllus, P.; Gittsovich, O.; Eisert, J. Covariance Matrices and the Separability Problem. Phys. Rev. Lett. 2007, 99, 130504. [Google Scholar] [CrossRef]
- Gittsovich, O.; Guhne, O. Quantifying entanglement with covariance matrices. Phys. Rev. A 2010, 81, 032333. [Google Scholar] [CrossRef]
- Das, T.; Karczewski, M.; Mandarino, A.; Markiewicz, M.; Woloncewicz, B.; Żukowski, M. Wave–particle complementarity: Detecting violation of local realism with photon-number resolving weak-field homodyne measurements. New J. Phys. 2022, 24, 033017. [Google Scholar] [CrossRef]
- Das, T.; Karczewski, M.; Mandarino, A.; Markiewicz, M.; Woloncewicz, B.; Żukowski, M. Remarks about Bell-nonclassicality of a single photon. Phys. Lett. A 2022, 435, 128031. [Google Scholar] [CrossRef]
- Das, T.; Karczewski, M.; Mandarino, A.; Markiewicz, M.; Woloncewicz, B.; Żukowski, M. Can single photon excitation of two spatially separated modes lead to a violation of Bell inequality via weak-field homodyne measurements? New J. Phys. 2021, 23, 073042. [Google Scholar] [CrossRef]
- Das, T.; Karczewski, M.; Mandarino, A.; Markiewicz, M.; Woloncewicz, B.; Żukowski, M. Comment on ‘Single particle nonlocality with completely independent reference states’. New J. Phys. 2022, 24, 038001. [Google Scholar] [CrossRef]
- Schlichtholz, K.; Mandarino, A.; Żukowski, M. Bosonic fields in states with undefined particle numbers possess detectable non-contextuality features, plus more. New J. Phys. 2022, 24, 103003. [Google Scholar] [CrossRef]
- Musz, M.; Kuś, M.; Życzkowski, K. Unitary quantum gates, perfect entanglers, and unistochastic maps. Phys. Rev. A 2013, 87, 022111. [Google Scholar] [CrossRef]
- Mandarino, A.; Linowski, T.; Życzkowski, K. Bipartite unitary gates and billiard dynamics in the Weyl chamber. Phys. Rev. A 2018, 98, 012335. [Google Scholar] [CrossRef]
- Bengtsson, I.; Życzkowski, K. Geometry of Quantum States: An Introduction to Quantum Entanglement; Cambridge University Press: Cambridge, UK, 2017. [Google Scholar]
- Życzkowski, K.; Penson, K.A.; Nechita, I.; Collins, B. Generating random density matrices. J. Math. Phys. 2011, 52, 062201. [Google Scholar] [CrossRef]
- Enríquez, M.; Puchała, Z.; Życzkowski, K. Minimal rényi–ingarden–urbanik entropy of multipartite quantum states. Entropy 2015, 17, 5063–5084. [Google Scholar] [CrossRef]
- Enríquez, M.; Delgado, F.; Życzkowski, K. Entanglement of three-qubit random pure states. Entropy 2018, 20, 745. [Google Scholar] [CrossRef]
- Zyczkowski, K.; Kus, M. Random unitary matrices. J. Phys. A Math. Gen. 1994, 27, 4235. [Google Scholar] [CrossRef]
- Gigena, N.; Scala, G.; Mandarino, A. Revisited aspects of the local set in CHSH Bell scenario. Int. J. Quantum Inf. 2022. [Google Scholar] [CrossRef]
- Braunstein, S.L.; Mann, A.; Revzen, M. Maximal violation of Bell inequalities for mixed states. Phys. Rev. Lett. 1992, 68, 3259–3261. [Google Scholar] [CrossRef] [PubMed]
- Capasso, V.; Fortunato, D.; Selleri, F. Sensitive observables of quantum mechanics. Int. J. Theor. Phys. 1973, 7, 319–326. [Google Scholar] [CrossRef]
- Gisin, N. Bell’s inequality holds for all non-product states. Phys. Lett. A 1991, 154, 201–202. [Google Scholar] [CrossRef]
- Gisin, N.; Peres, A. Maximal violation of Bell’s inequality for arbitrarily large spin. Phys. Lett. A 1992, 162, 15–17. [Google Scholar] [CrossRef]
- Popescu, S.; Rohrlich, D. Which states violate Bell’s inequality maximally? Phys. Lett. A 1992, 169, 411–414. [Google Scholar] [CrossRef]
- Karczewski, M.; Scala, G.; Mandarino, A.; Sainz, A.B.; Żukowski, M. Avenues to generalising Bell inequalities. J. Phys. A Math. Theor. 2022, 55, 384011. [Google Scholar] [CrossRef]
- Das, T.; Karczewski, M.; Mandarino, A.; Markiewicz, M.; Żukowski, M. Optimal Interferometry for Bell Nonclassicality Induced by a Vacuum–One-Photon Qubit. Phys. Rev. Appl. 2022, 18, 034074. [Google Scholar] [CrossRef]
- Uhlmann, A. The “transition probability” in the state space of a*-algebra. Rep. Math. Phys. 1976, 9, 273–279. [Google Scholar] [CrossRef]
- Życzkowski, K.; Sommers, H.J. Average fidelity between random quantum states. Phys. Rev. A 2005, 71, 032313. [Google Scholar] [CrossRef]
- Cirel’son, B.S. Quantum generalizations of Bell’s inequality. Lett. Math. Phys. 1980, 4, 93–100. [Google Scholar] [CrossRef]
- Gühne, O.; Tóth, G. Entanglement detection. Phys. Rep. 2009, 474, 1–75. [Google Scholar] [CrossRef]
- Verstraete, F.; Dehaene, J.; Moor, B.D. Normal forms and entanglement measures for multipartite quantum states. Phys. Rev. A 2003, 68, 012103. [Google Scholar] [CrossRef]
- Gisin, N. Hidden quantum nonlocality revealed by local filters. Phys. Lett. A 1996, 210, 151–156. [Google Scholar] [CrossRef]
- Leinaas, J.M.; Myrheim, J.; Ovrum, E. Geometrical aspects of entanglement. Phys. Rev. A 2006, 74, 012313. [Google Scholar] [CrossRef]
- Bina, M.; Mandarino, A.; Olivares, S.; Paris, M.G.A. Drawbacks of the use of fidelity to assess quantum resources. Phys. Rev. A 2014, 89, 012305. [Google Scholar] [CrossRef]
- Mandarino, A.; Bina, M.; Olivares, S.; Paris, M.G. About the use of fidelity in continuous variable systems. Int. J. Quantum Inf. 2014, 12, 1461015. [Google Scholar] [CrossRef]
- Mandarino, A.; Bina, M.; Porto, C.; Cialdi, S.; Olivares, S.; Paris, M.G.A. Assessing the significance of fidelity as a figure of merit in quantum state reconstruction of discrete and continuous-variable systems. Phys. Rev. A 2016, 93, 062118. [Google Scholar] [CrossRef]
- McKague, M. Self-testing in parallel with CHSH. Quantum 2017, 1, 1. [Google Scholar] [CrossRef]
- Coladangelo, A. Generalization of the Clauser-Horne-Shimony-Holt inequality self-testing maximally entangled states of any local dimension. Phys. Rev. A 2018, 98, 052115. [Google Scholar] [CrossRef]
- Sarbicki, G.; Scala, G.; Chruściński, D. Family of multipartite separability criteria based on a correlation tensor. Phys. Rev. A 2020, 101, 012341. [Google Scholar] [CrossRef]
- Lewenstein, M.; Kraus, B.; Cirac, J.I.; Horodecki, P. Optimization of entanglement witnesses. Phys. Rev. A 2000, 62, 052310. [Google Scholar] [CrossRef]
- Sarbicki, G.; Scala, G.; Chruściński, D. Enhanced realignment criterion vs. linear entanglement witnesses. J. Phys. A Math. Theor. 2020, 53, 455302. [Google Scholar] [CrossRef]
- Sarbicki, G.; Scala, G.; Chruściński, D. Detection Power of Separability Criteria Based on a Correlation Tensor: A Case Study. Open Syst. Inf. Dyn. 2021, 28, 2150010. [Google Scholar] [CrossRef]
- Bae, K.; Son, W. Generalized nonlocality criteria under the correlation symmetry. Phys. Rev. A 2018, 98, 022116. [Google Scholar] [CrossRef]
- Bernards, F.; Gühne, O. Generalizing Optimal Bell Inequalities. Phys. Rev. Lett. 2020, 125, 200401. [Google Scholar] [CrossRef]
- Catani, L.; Leifer, M.; Scala, G.; Schmid, D.; Spekkens, R.W. What is Nonclassical about Uncertainty Relations? Phys. Rev. Lett. 2022, 129, 240401. [Google Scholar] [CrossRef]
- Catani, L.; Leifer, M.; Scala, G.; Schmid, D.; Spekkens, R.W. What aspects of the phenomenology of interference witness nonclassicality? arXiv 2022, arXiv:2211.09850. [Google Scholar]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mandarino, A.; Scala, G. On the Fidelity Robustness of CHSH–Bell Inequality via Filtered Random States. Entropy 2023, 25, 94. https://doi.org/10.3390/e25010094
Mandarino A, Scala G. On the Fidelity Robustness of CHSH–Bell Inequality via Filtered Random States. Entropy. 2023; 25(1):94. https://doi.org/10.3390/e25010094
Chicago/Turabian StyleMandarino, Antonio, and Giovanni Scala. 2023. "On the Fidelity Robustness of CHSH–Bell Inequality via Filtered Random States" Entropy 25, no. 1: 94. https://doi.org/10.3390/e25010094
APA StyleMandarino, A., & Scala, G. (2023). On the Fidelity Robustness of CHSH–Bell Inequality via Filtered Random States. Entropy, 25(1), 94. https://doi.org/10.3390/e25010094








