Multi-User Measurement-Device-Independent Quantum Key Distribution Based on GHZ Entangled State
Abstract
:1. Introduction
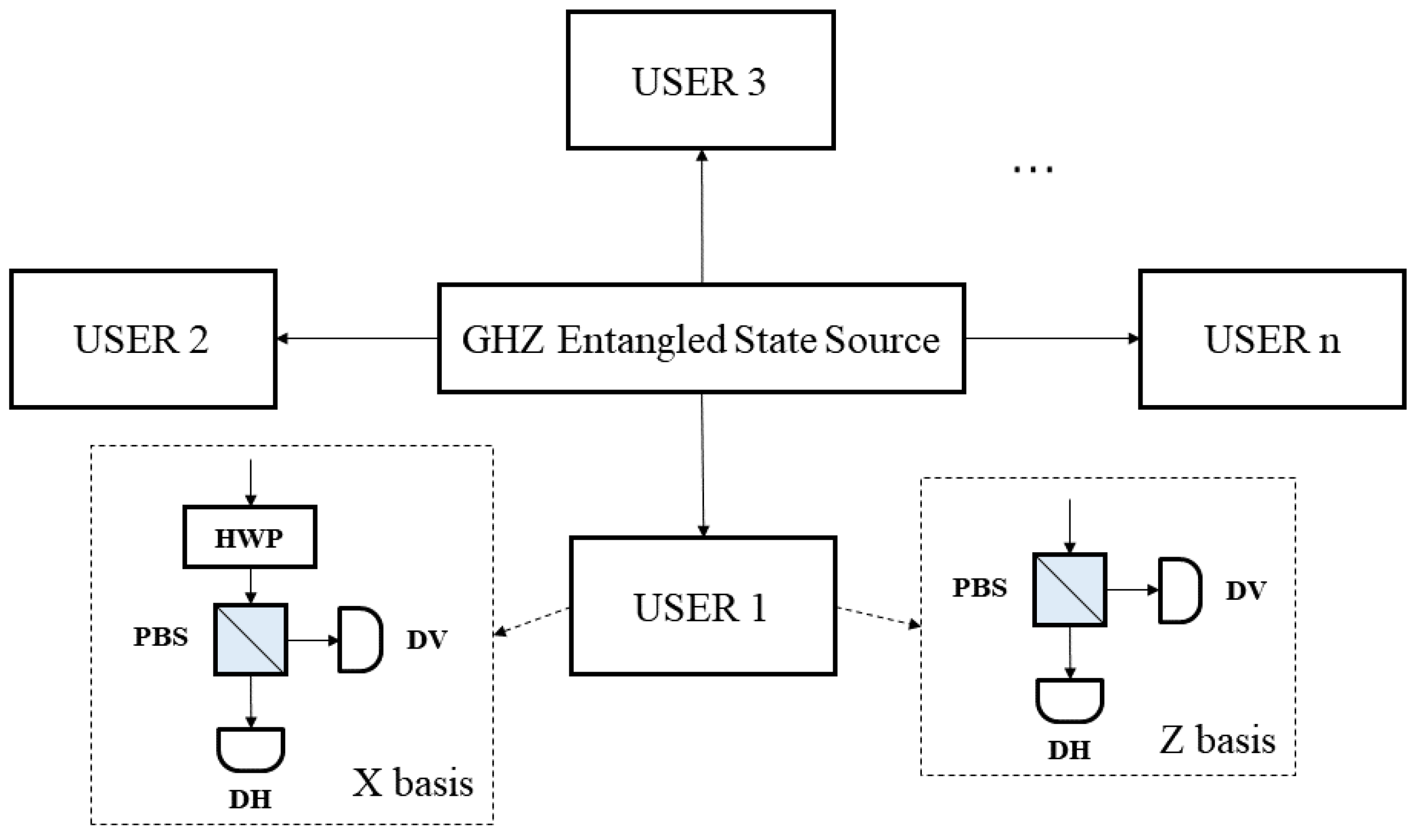
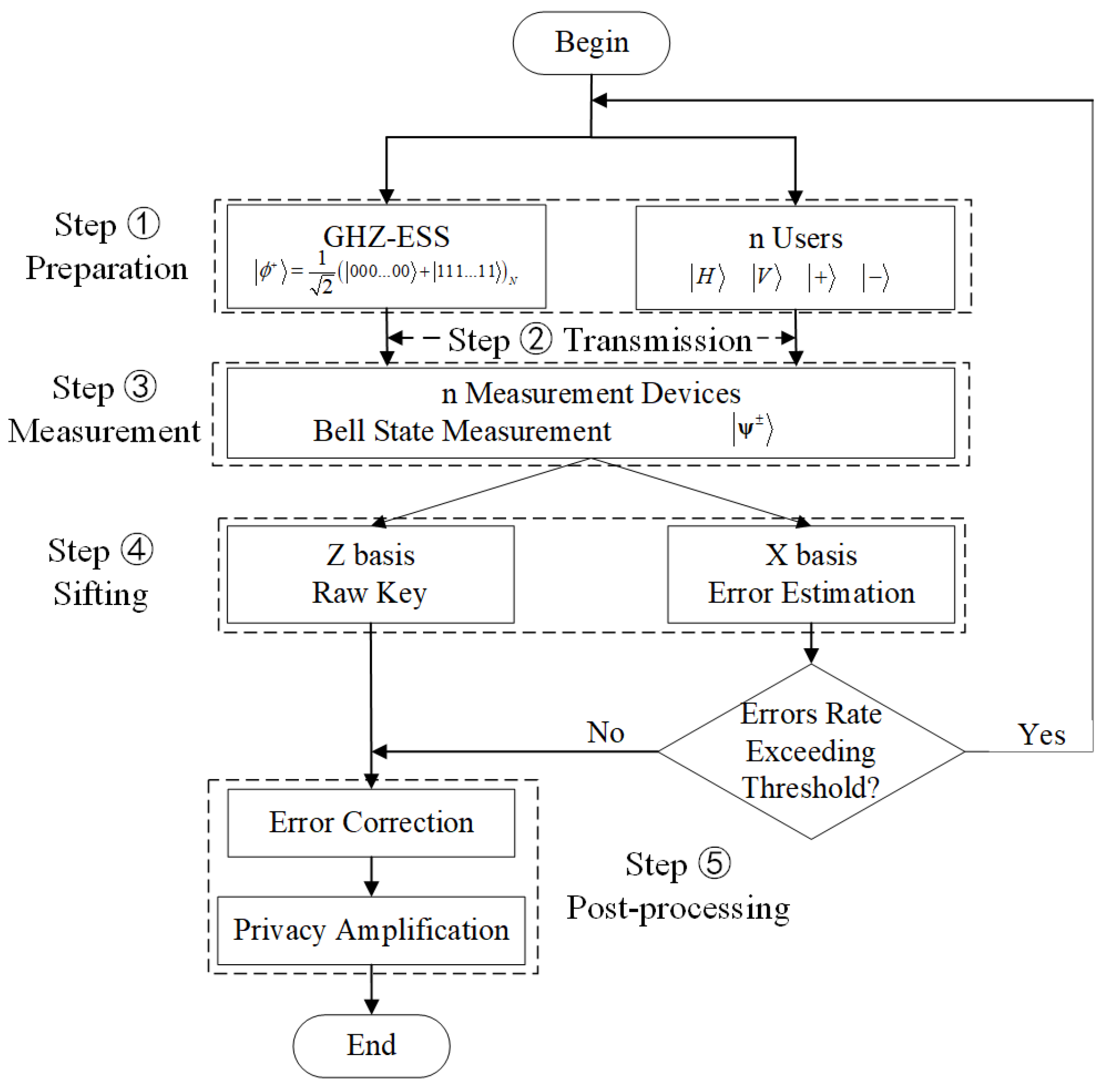
2. Protocol
3. Secure Key Rate
3.1. Key Rate of Single-Photon Source
3.2. Key Rate of Weak Coherent Source with Decoy State
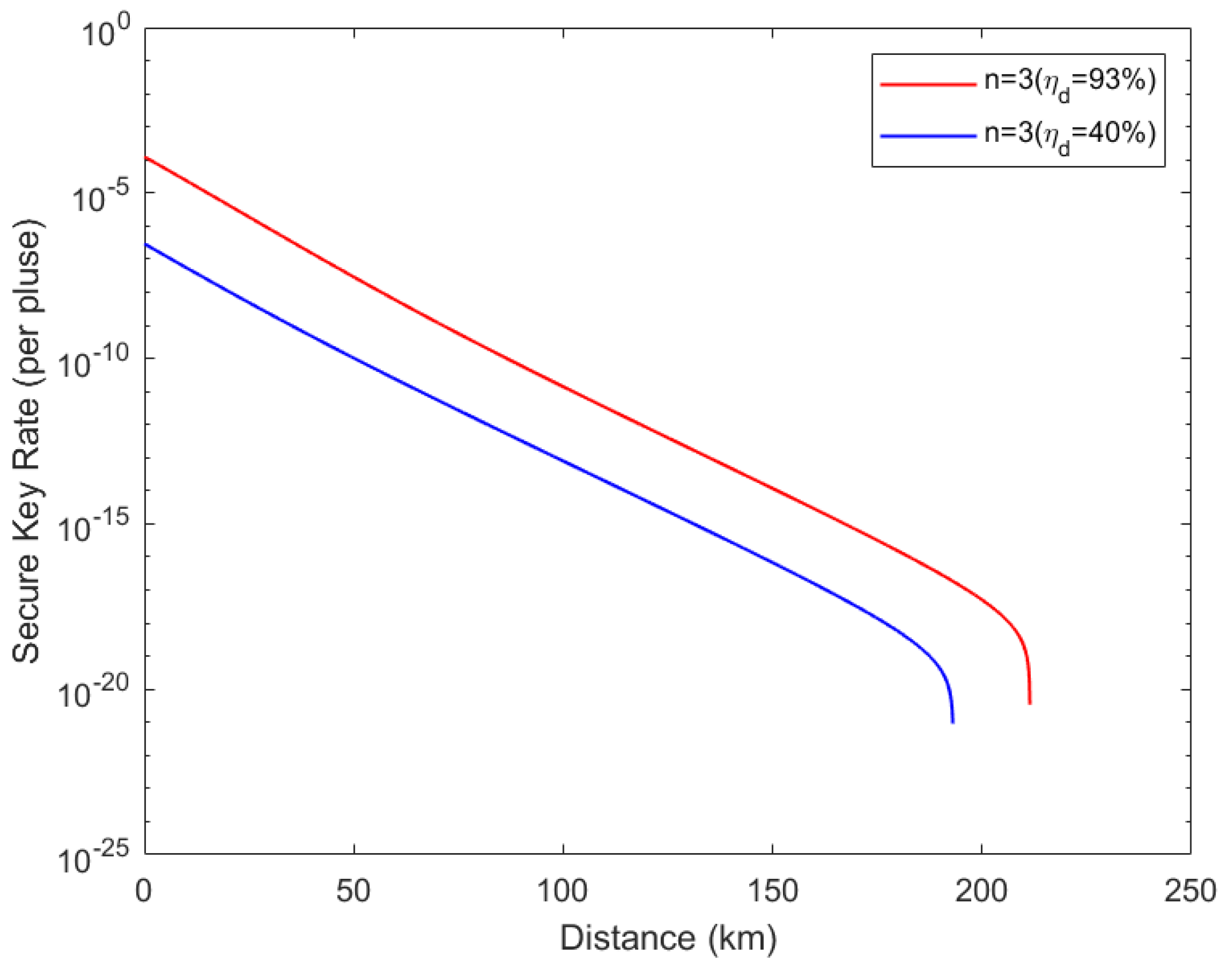
3.3. Security and Discussion
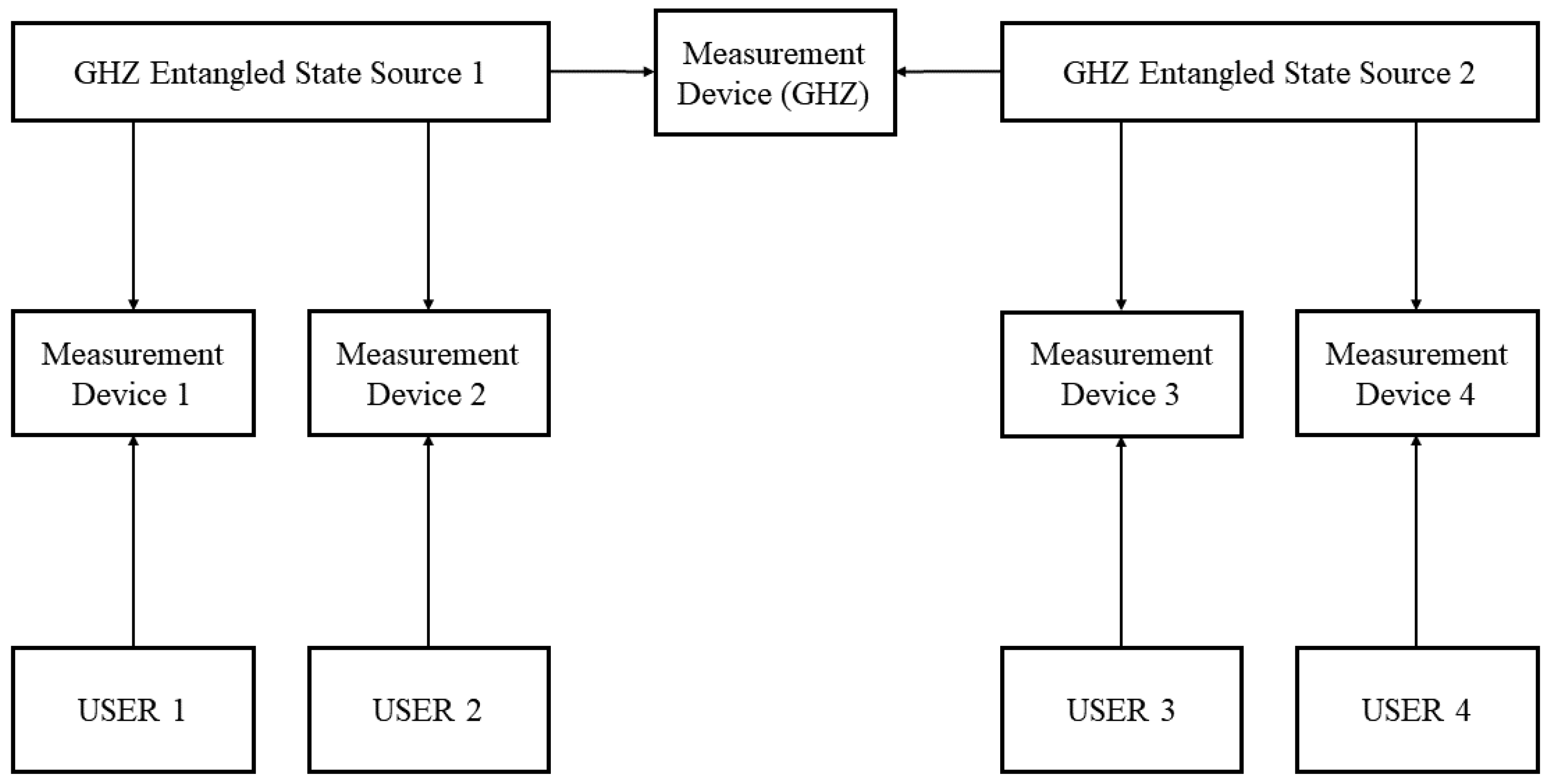
4. Expansion of Our Protocol
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Protocol Analysis
| GHZ Entangled State | MR1 | Value1 | MR2 | Value2 | MR3 | Value3 |
|---|---|---|---|---|---|---|
| 0 | 0 | 0 | ||||
| 1 | 1 | 1 | ||||
| 1 | 0 | 0 | ||||
| 0 | 1 | 1 | ||||
| 0 | 1 | 0 | ||||
| 1 | 0 | 1 | ||||
| 0 | 0 | 1 | ||||
| 1 | 1 | 0 |
Appendix B. Increasing the Secure Key Rate with an Adaptive Technique

References
- Xu, F.; Ma, X.; Zhang, Q.; Lo, H.K.; Pan, J.W. Secure quantum key distribution with realistic devices. Rev. Mod. Phys. 2020, 92, 025002. [Google Scholar] [CrossRef]
- Gisin, N.; Ribordy, G.; Tittel, W.; Zbinden, H. Quantum cryptography. Rev. Mod. Phys. 2002, 74, 145–195. [Google Scholar] [CrossRef] [Green Version]
- Bennett, C.H.; Brassard, G. Quantum Cryptography: Public Key distribution and Coin Tossing. In Proceedings of the IEEE International Conference on Computers, Systems and Signal Processing, Bangalore, India, 10–12 December 1984; Institute of Electrical and Electronics Engineers: Bangalore, India, 1984; pp. 174–179. [Google Scholar]
- Shor, P.W.; Preskill, J. Simple proof of security of the BB84 quantum key distribution protocol. Phys. Rev. Lett. 2000, 85, 441–444. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hwang, W.Y. Quantum Key Distribution with High Loss: Toward Global Secure Communication. Phys. Rev. Lett. 2003, 91, 057901. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Acín, A.; Brunner, N.; Gisin, N.; Massar, S.; Pironio, S.; Scarani, V. Device-independent security of quantum cryptography against collective attacks. Phys. Rev. Lett. 2007, 98, 230501. [Google Scholar] [CrossRef] [Green Version]
- Lo, H.K.; Curty, M.; Qi, B. Measurement-device-independent quantum key distribution. Phys. Rev. Lett. 2012, 108, 130503. [Google Scholar] [CrossRef] [Green Version]
- Wang, S.; Chen, W.; Yin, Z.Q.; Li, H.W.; He, D.Y.; Li, Y.H.; Zhou, Z.; Song, X.T.; Li, F.Y.; Wang, D.; et al. Field and long-term demonstration of a wide area quantum key distribution network. Opt. Express 2014, 22, 21739. [Google Scholar] [CrossRef] [Green Version]
- Cheng, G.; Guo, B.; Zhang, C.; Guo, J.; Fan, R. Wavelength division multiplexing quantum key distribution network using a modified plug-and-play system. Opt. Quantum Electron. 2015, 47, 1809–1817. [Google Scholar] [CrossRef]
- Chen, Y.A.; Zhang, Q.; Chen, T.Y.; Cai, W.Q.; Liao, S.K.; Zhang, J.; Chen, K.; Yin, J.; Ren, J.G.; Chen, Z.; et al. An integrated space-to-ground quantum communication network over 4,600 kilometres. Nature 2021, 589, 214–219. [Google Scholar] [CrossRef]
- Greenberger, D.M.; Horne, M.A.; Zeilinger, A. Going Beyond Bell’s Theorem. In Bell’s Theorem, Quantum Theory and Conceptions of the Universe; Kafatos, M., Ed.; Springer: Dordrecht, The Netherlands, 1989; pp. 69–72. [Google Scholar] [CrossRef]
- Multi-partite quantum cryptographic protocols with noisy ghz states. Quantum Inf. Comput. 2007, 7, 689–715. [CrossRef]
- Zhang, L.; Hu, M.; Ran, P.; Zeng, H.; Li, J.; Guo, B. Fuzzy Greenberger–Horne–Zeilinger state analyzer and multiparty measurement-device-independent quantum key distribution network. Opt. Eng. 2019, 58, 016113. [Google Scholar] [CrossRef]
- Cao, X.Y.; Gu, J.; Lu, Y.S.; Yin, H.L.; Chen, Z.B. Coherent one-way quantum conference key agreement based on twin field. New J. Phys. 2021, 23, 043002. [Google Scholar] [CrossRef]
- Grasselli, F.; Kampermann, H.; Bruß, D. Conference key agreement with single-photon interference. New J. Phys. 2019, 21, 123002. [Google Scholar] [CrossRef]
- Epping, M.; Kampermann, H.; Macchiavello, C.; Bruß, D. Multi-partite entanglement can speed up quantum key distribution in networks. New J. Phys. 2017, 19, 093012. [Google Scholar] [CrossRef]
- Cao, X.Y.; Lu, Y.S.; Li, Z.; Gu, J.; Yin, H.L.; Chen, Z.B. High Key Rate Quantum Conference Key Agreement with Unconditional Security. IEEE Access 2021, 9, 128870–128876. [Google Scholar] [CrossRef]
- Xu, F.; Qi, B.; Liao, Z.; Lo, H.K. Long distance measurement-device-independent quantum key distribution with entangled photon sources. Appl. Phys. Lett. 2013, 103, 061101. [Google Scholar] [CrossRef] [Green Version]
- Zhao, S.; Zeng, P.; Cao, W.F.; Xu, X.Y.; Zhen, Y.Z.; Ma, X.; Li, L.; Liu, N.L.; Chen, K. Phase-Matching Quantum Cryptographic Conferencing. Phys. Rev. Appl. 2020, 14, 024010. [Google Scholar] [CrossRef]
- Hong, C.K.; Ou, Z.Y.; Mandel, L. Measurement of subpicosecond time intervals between two photons by interference. Phys. Rev. Lett. 1987, 59, 2044–2046. [Google Scholar] [CrossRef] [Green Version]
- Bose, S.; Vedral, V.; Knight, P.L. Multiparticle generalization of entanglement swapping. Phys. Rev. A At. Mol. Opt. Phys. 1998, 57, 822–829. [Google Scholar] [CrossRef] [Green Version]
- Gottesman, D.; Hoi-Kwonglo, L.O.; Lütkenhaus, N.; Preskill, J. Security of quantum key distribution with imperfect devices. Quantum Inf. Comput. 2004, 4, 325–360. [Google Scholar] [CrossRef]
- Cao, W.F.; Zhen, Y.Z.; Zheng, Y.L.; Zhao, S.; Xu, F.; Li, L.; Chen, Z.B.; Liu, N.L.; Chen, K. Open-destination measurement-device-independent quantum key distribution network. Entropy 2020, 22, 1083. [Google Scholar] [CrossRef] [PubMed]
- Marsili, F.; Verma, V.B.; Stern, J.A.; Harrington, S.; Lita, A.E.; Gerrits, T.; Vayshenker, I.; Baek, B.; Shaw, M.D.; Mirin, R.P.; et al. Detecting single infrared photons with 93% system efficiency. Nat. Photonics 2013, 7, 210–214. [Google Scholar] [CrossRef]
- Ma, X.; Qi, B.; Zhao, Y.; Lo, H.K. Practical decoy state for quantum key distribution. Phys. Rev. A 2005, 72, 012326. [Google Scholar] [CrossRef] [Green Version]
- Fu, Y.; Yin, H.L.; Chen, T.Y.; Chen, Z.B. Long-distance measurement-device-independent multiparty quantum communication. Phys. Rev. Lett. 2015, 114, 090501. [Google Scholar] [CrossRef] [Green Version]
- Xu, F.; Curty, M.; Qi, B.; Lo, H.K. Practical aspects of measurement-device-independent quantum key distribution. New J. Phys. 2013, 15, 113007. [Google Scholar] [CrossRef]
- Lütkenhaus, N.; Jahma, M. Quantum key distribution with realistic states: Photon-number statistics in the photon-number splitting attack. New J. Phys. 2002, 4, 44. [Google Scholar] [CrossRef]
- Ma, X.; Razavi, M. Alternative schemes for measurement-device-independent quantum key distribution. Phys. Rev. A 2012, 86, 062319. [Google Scholar] [CrossRef] [Green Version]
- Yin, H.L.; Chen, T.Y.; Yu, Z.W.; Liu, H.; You, L.X.; Zhou, Y.H.; Chen, S.J.; Mao, Y.; Huang, M.Q.; Zhang, W.J.; et al. Measurement-Device-Independent Quantum Key Distribution over a 404 km Optical Fiber. Phys. Rev. Lett. 2016, 117, 190501. [Google Scholar] [CrossRef]
- Zhu, C.; Xu, F.; Pei, C. W-state Analyzer and Multi-party Measurement-device-independent Quantum Key Distribution. Sci. Rep. 2015, 5, 17449. [Google Scholar] [CrossRef] [Green Version]
- Li, Z.; Cao, X.Y.; Li, C.L.; Weng, C.X.; Gu, J.; Yin, H.L.; Chen, Z.B. Finite-key analysis for quantum conference key agreement with asymmetric channels. Quantum Sci. Technol. 2021, 6, 045019. [Google Scholar] [CrossRef]
- Lucamarini, M.; Yuan, Z.L.; Dynes, J.F.; Shields, A.J. Overcoming the rate-distance limit of quantum key distribution without quantum repeaters. Nature 2018, 557, 400–403. [Google Scholar] [CrossRef] [PubMed]
- Xie, Y.M.; Lu, Y.S.; Weng, C.X.; Cao, X.Y.; Jia, Z.Y.; Bao, Y.; Wang, Y.; Fu, Y.; Yin, H.L.; Chen, Z.B. Breaking the Rate-Loss Bound of Quantum Key Distribution with Asynchronous Two-Photon Interference. Phys. Rev. Appl. 2021, 10, 020315. [Google Scholar] [CrossRef]
- Zeng, P.; Zhou, H.; Wu, W.; Ma, X. Quantum key distribution surpassing the repeaterless rate-transmittance bound without global phase locking. arXiv 2022, arXiv:2201.04300. [Google Scholar]
- Azuma, K.; Tamaki, K.; Munro, W.J. All-photonic intercity quantum key distribution. Nat. Commun. 2015, 6, 10171. [Google Scholar] [CrossRef] [PubMed] [Green Version]


| GHZ State | BSM 1 | BSM 2 | BSM 3 | Alice | Bob | Charles |
|---|---|---|---|---|---|---|
| No Flip | No Flip | No Flip | ||||
| No Flip | Flip | No Flip | ||||
| No Flip | No Flip | Flip | ||||
| No Flip | Flip | Flip |
| f | |||
|---|---|---|---|
| 2% | 1.16 | 0.2 |
| Items | GHZ State MDI-QCC [26] | W State Multi-User MDI-QKD [31] | GHZ State Multi-User MDI-QKD [23] | Our Scheme |
|---|---|---|---|---|
| Entangled State | GHZ state | W state | GHZ state | GHZ state |
| Users | ≥3 | ≥4 | ≥3 | ≥3 |
| Secure Key Rate | (, 400 km between two users, weak coherent) | (, 260 km between two users, single photon) | (, 400 km between two users, single photon) | (, 400 km between two users, single photon) |
| Available Distance | 420 km between two users (weak coherent) | 260 km between two users (single photon) | 520 km between two users (single photon) | 560 km between two users (single photon) 420 km between two users (weak coherent) |
| GHZ state | USER 1 | USER 2 | USER 3 | USER 4 |
|---|---|---|---|---|
| No Flip | No Flip | Flip | Flip | |
| No Flip | Flip | Flip | No Flip | |
| No Flip | No Flip | Flip | Flip | |
| No Flip | Flip | Flip | No Flip |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hua, X.; Hu, M.; Guo, B. Multi-User Measurement-Device-Independent Quantum Key Distribution Based on GHZ Entangled State. Entropy 2022, 24, 841. https://doi.org/10.3390/e24060841
Hua X, Hu M, Guo B. Multi-User Measurement-Device-Independent Quantum Key Distribution Based on GHZ Entangled State. Entropy. 2022; 24(6):841. https://doi.org/10.3390/e24060841
Chicago/Turabian StyleHua, Ximing, Min Hu, and Banghong Guo. 2022. "Multi-User Measurement-Device-Independent Quantum Key Distribution Based on GHZ Entangled State" Entropy 24, no. 6: 841. https://doi.org/10.3390/e24060841
APA StyleHua, X., Hu, M., & Guo, B. (2022). Multi-User Measurement-Device-Independent Quantum Key Distribution Based on GHZ Entangled State. Entropy, 24(6), 841. https://doi.org/10.3390/e24060841






