Cumulative Residual q-Fisher Information and Jensen-Cumulative Residual χ2 Divergence Measures
Abstract
:1. Introduction
2. Preliminaries
3. CRQF Information Measure
q-Hazard Rate Function and Its Connection to CRQF Information Measure
- (i)
- If , then ;
- (ii)
- If , then ’
4. Generalized Cumulative Residual Divergence Measures
5. Cumulative Residual -Fisher Information for Two Well-Known Mixture Models
5.1. Arithmetic Mixture Distribution
- (i)
- If , then ;
- (ii)
- If , then ,
5.2. Harmonic Mixture Distribution
5.3. HM Distribution Having Optimal Information under CR- Divergence Measure
6. Jensen-Cumulative Residual and Parametric Version of Jensen-Cumulative Residual Fisher Divergence Measures
6.1. Jensen-Cumulative Residual Divergence Measure
6.2. Parametric Version of Jensen-Cumulative Residual Fisher Information Divergence
6.3. Connection between the P-JCRF Information and JCR- Divergence Measures
7. Application of CRQF Information Measure
8. Concluding Remarks
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Cover, T.M.; Thomas, J.A. Information theory and statistics. Elem. Inf. Theory 1991, 1, 279–335. [Google Scholar]
- Zegers, P. Fisher information properties. Entropy 2015, 17, 4918–4939. [Google Scholar] [CrossRef] [Green Version]
- Fisher, R.A. Tests of significance in harmonic analysis. Proc. R. Soc. Lond. Ser. A 1929, 125, 54–59. [Google Scholar]
- Shannon, C.E. A mathematical theory of communication. Bell. System. Tech. J. 1948, 27, 379–423. [Google Scholar] [CrossRef] [Green Version]
- Nielsen, F.; Nock, R. On the chi square and higher-order chi distances for approximating f-divergences. IEEE Signal Process. Lett. 2013, 21, 10–13. [Google Scholar] [CrossRef] [Green Version]
- Popescu, P.G.; Preda, V.; Sluşanschi, E.I. Bounds for Jeffreys-Tsallis and Jensen-Shannon-Tsallis divergences. Phys. Stat. Mech. Its Appl. 2014, 413, 280–283. [Google Scholar] [CrossRef]
- Sánchez-Moreno, P.; Zarzo, A.; Dehesa, J.S. Jensen divergence based on Fisher’s information. J. Phys. A Math. Theor. 2012, 45, 125305. [Google Scholar] [CrossRef] [Green Version]
- Bercher, J.F. Some properties of generalized Fisher information in the context of nonextensive thermostatistics. Phys. Stat. Mech. Its Appl. 2013, 392, 3140–3154. [Google Scholar] [CrossRef] [Green Version]
- Tsallis, C. Possible generalization of Boltzmann-Gibbs statistics. J. Stat. Phys. 1988, 52, 479–487. [Google Scholar] [CrossRef]
- Johnson, O.; Vignat, C. Some results concerning maximum Rényi entropy distributions. In: Annales de l’Institut Henri Poincare (B). Probab. Stat. 2007, 43, 339–351. [Google Scholar]
- Furuichi, S. On the maximum entropy principle and the minimization of the Fisher information in Tsallis statistics. J. Math. Phys. 2009, 50, 013303. [Google Scholar] [CrossRef] [Green Version]
- Lutwak, E.; Lv, S.; Yang, D.; Zhang, G. Extensions of Fisher information and Stam’s inequality. IEEE Trans. Inf. Theory 2012, 58, 1319–1327. [Google Scholar] [CrossRef]
- Kharazmi, O.; Balakrishnan, N. Cumulative residual and relative cumulative residual Fisher information and their properties. IEEE Trans. Inf. Theory 2021, 67, 6306–6312. [Google Scholar] [CrossRef]
- Rao, M.; Chen, Y.; Vemuri, B.C.; Wang, F. Cumulative residual entropy: A new measure of information. IEEE Trans. Inf. Theory 2004, 50, 1220–1228. [Google Scholar] [CrossRef]
- Yamano, T. Some properties of q-logarithm and q-exponential functions in Tsallis statistics. Phys. Stat. Mech. Its Appl. 2002, 305, 486–496. [Google Scholar] [CrossRef]
- Masi, M. A step beyond Tsallis and Rényi entropies. Phys. Lett. A 2005, 338, 217–224. [Google Scholar] [CrossRef] [Green Version]
- Barlow, R.E.; Proschan, F. Statistical Theory of Reliability and Life Testing: Probability Models; Holt, Rinehart and Winston: New York, NY, USA, 1975. [Google Scholar]
- Basu, A.; Harris, I.R.; Hjort, N.L.; Jones, M.C. Robust and efficient estimation by minimising a density power divergence. Biometrika 1998, 85, 549–559. [Google Scholar] [CrossRef] [Green Version]
- Ghosh, A.; Harris, I.R.; Maji, A.; Basu, A.; Pardo, L. A generalized divergence for statistical inference. Bernoulli 2017, 23, 2746–2783. [Google Scholar] [CrossRef] [Green Version]
- Marshall, A.W.; Olkin, I. Life Distributions; Springer: New York, NY, USA, 2007. [Google Scholar]
- Schmidt, U. Axiomatic Utility Theory Under Risk: Non-Archimedean Representations and Application to Insurance Economics; Springer: Berlin, Germany, 2012. [Google Scholar]
- Asadi, M.; Ebrahimi, N.; Kharazmi, O.; Soofi, E.S. Mixture models, Bayes Fisher information, and divergence measures. IEEE Trans. Inf. Theory 2018, 65, 2316–2321. [Google Scholar] [CrossRef]
- Cvetkovski, Z. Inequalities: Theorems, Techniques and Selected Problems; Springer: New York, NY, USA, 2012. [Google Scholar]
- Pau, G.; Fuchs, F.; Sklyar, O.; Boutros, M.; Huber, W. EBImage-an R package for image processing with applications to cellular phenotypes. Bioinformatics 2010, 26, 979–981. [Google Scholar] [CrossRef] [PubMed]


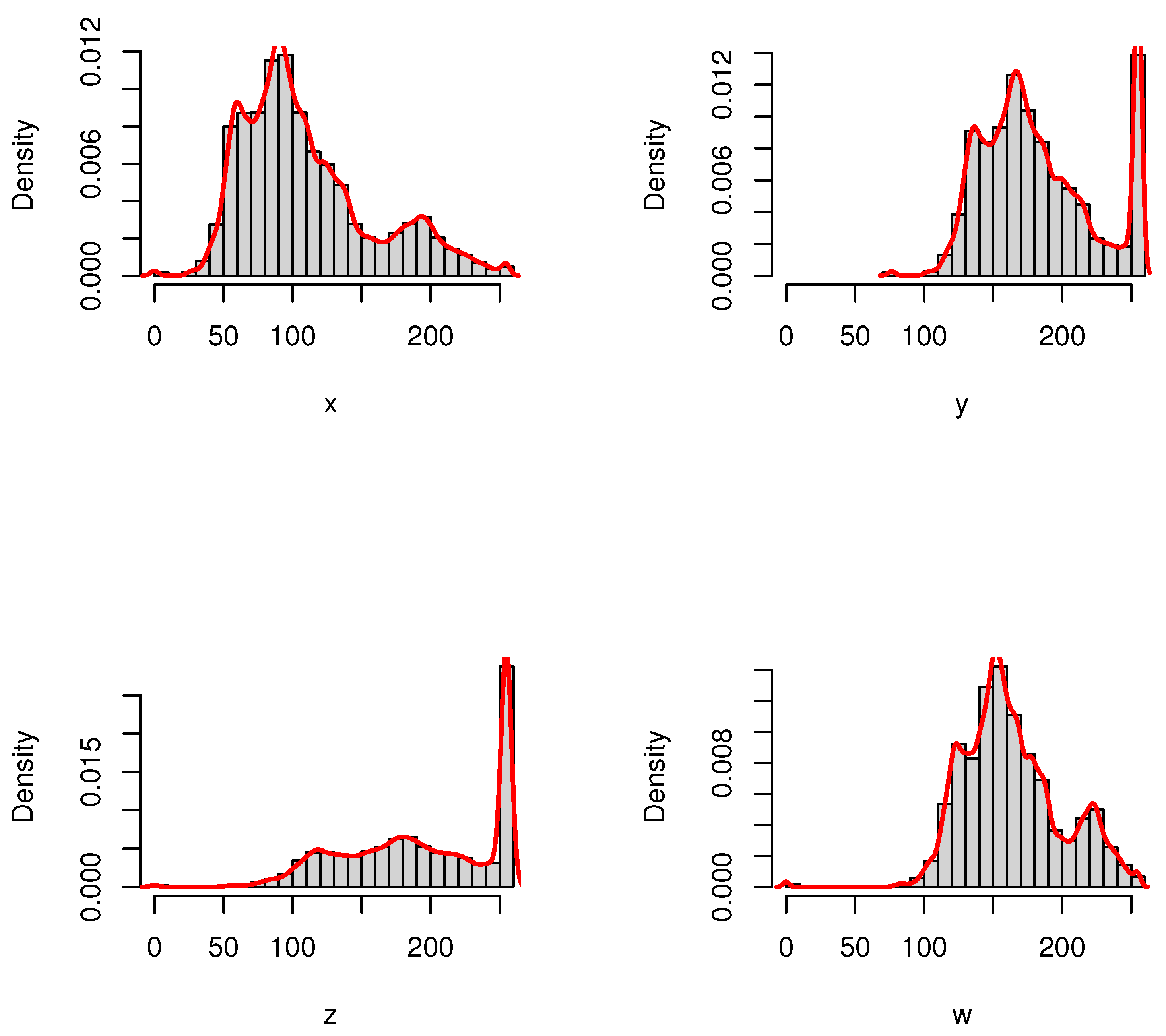
| CRQF () | CRQF () | FI | |
|---|---|---|---|
| X | 0.0068 | 0.0057 | 0.0039 |
| Y | 0.0085 | 0.0066 | 0.0447 |
| Z | 0.0096 | 0.0071 | 0.0475 |
| W | 0.0082 | 0.0067 | 0.0052 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kharazmi, O.; Balakrishnan, N.; Jamali, H. Cumulative Residual q-Fisher Information and Jensen-Cumulative Residual χ2 Divergence Measures. Entropy 2022, 24, 341. https://doi.org/10.3390/e24030341
Kharazmi O, Balakrishnan N, Jamali H. Cumulative Residual q-Fisher Information and Jensen-Cumulative Residual χ2 Divergence Measures. Entropy. 2022; 24(3):341. https://doi.org/10.3390/e24030341
Chicago/Turabian StyleKharazmi, Omid, Narayanaswamy Balakrishnan, and Hassan Jamali. 2022. "Cumulative Residual q-Fisher Information and Jensen-Cumulative Residual χ2 Divergence Measures" Entropy 24, no. 3: 341. https://doi.org/10.3390/e24030341
APA StyleKharazmi, O., Balakrishnan, N., & Jamali, H. (2022). Cumulative Residual q-Fisher Information and Jensen-Cumulative Residual χ2 Divergence Measures. Entropy, 24(3), 341. https://doi.org/10.3390/e24030341







