Quantum Information Entropy of Hyperbolic Potentials in Fractional Schrödinger Equation
Abstract
1. Introduction
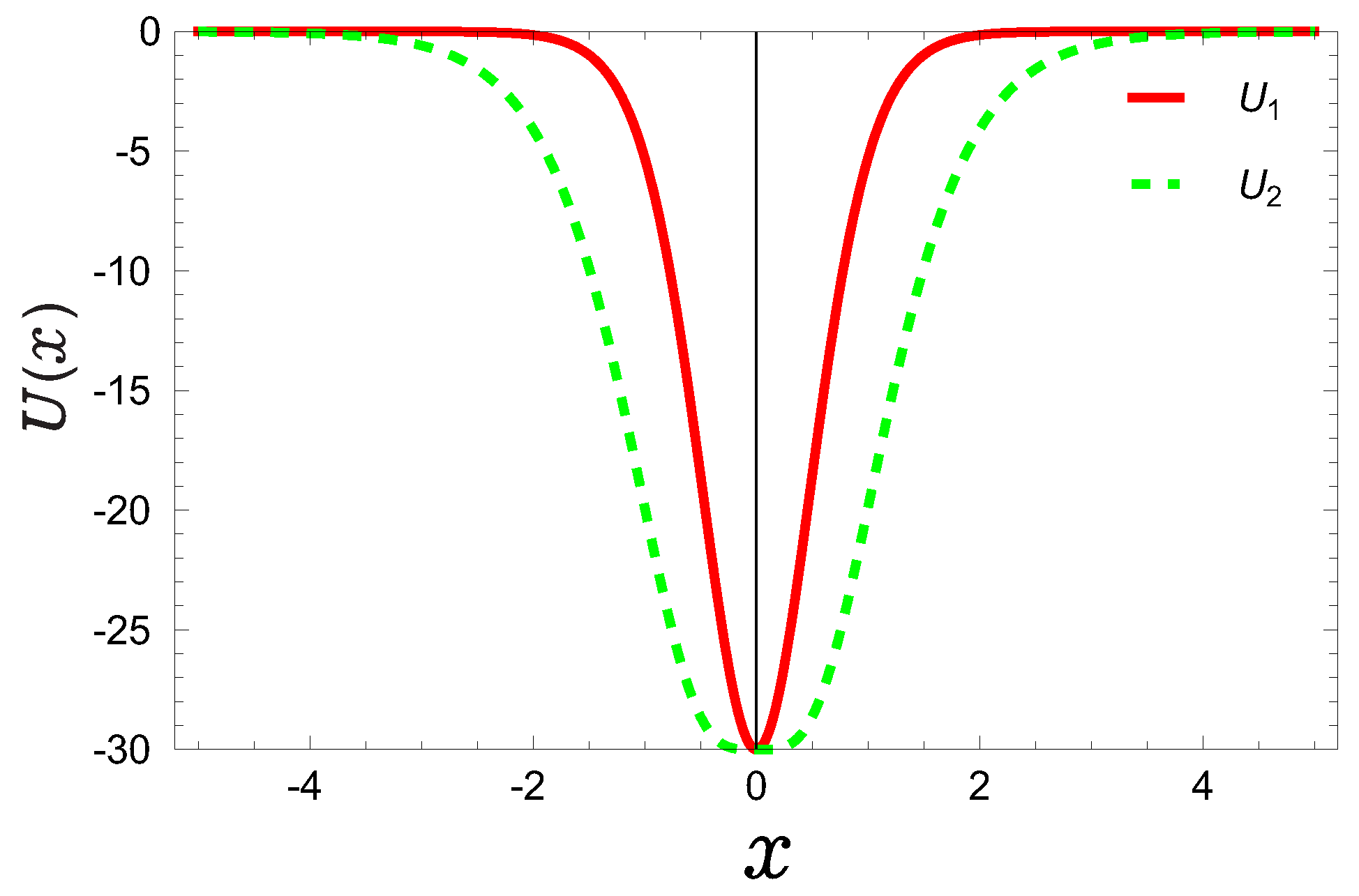
2. Formalism
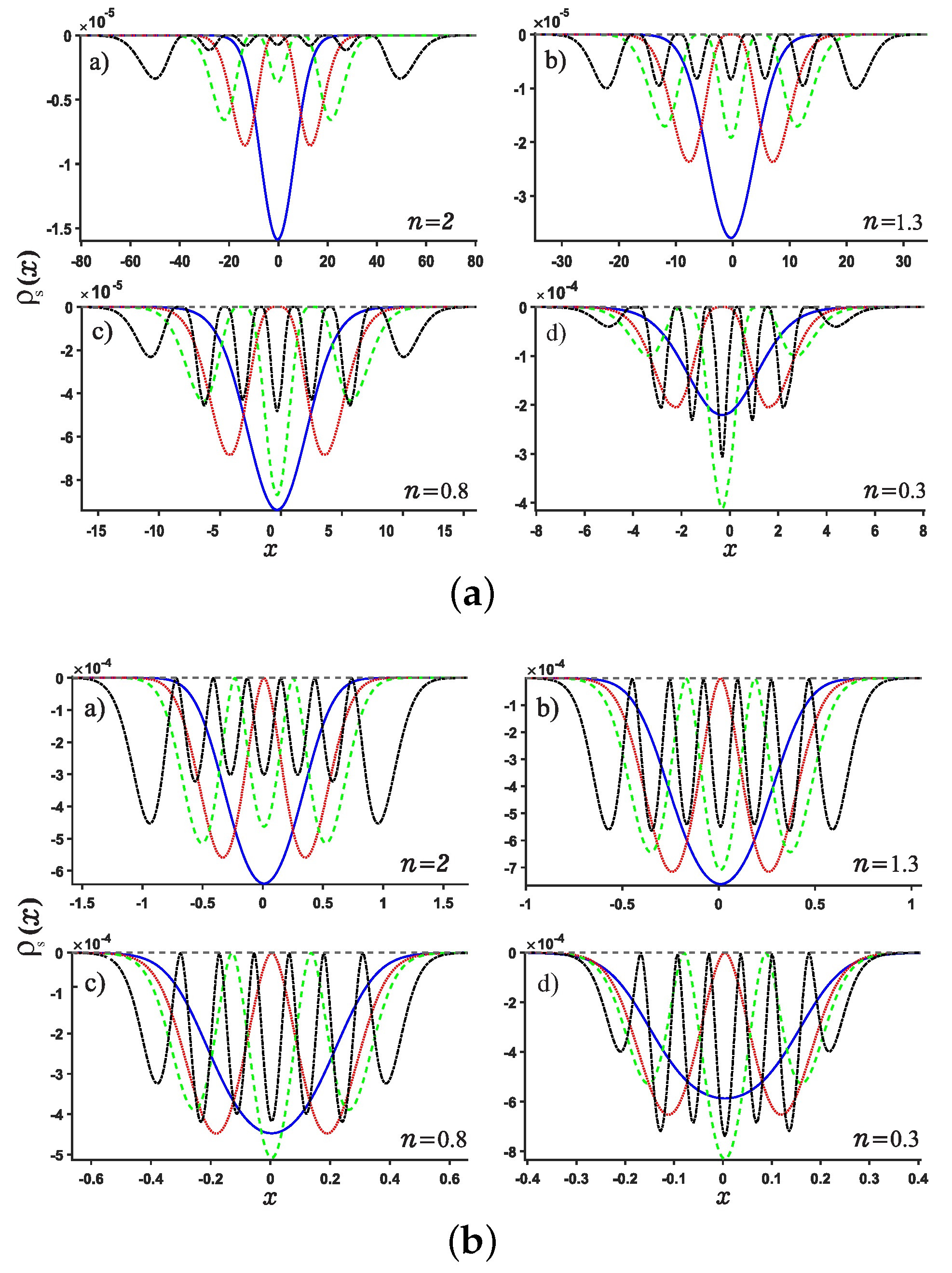
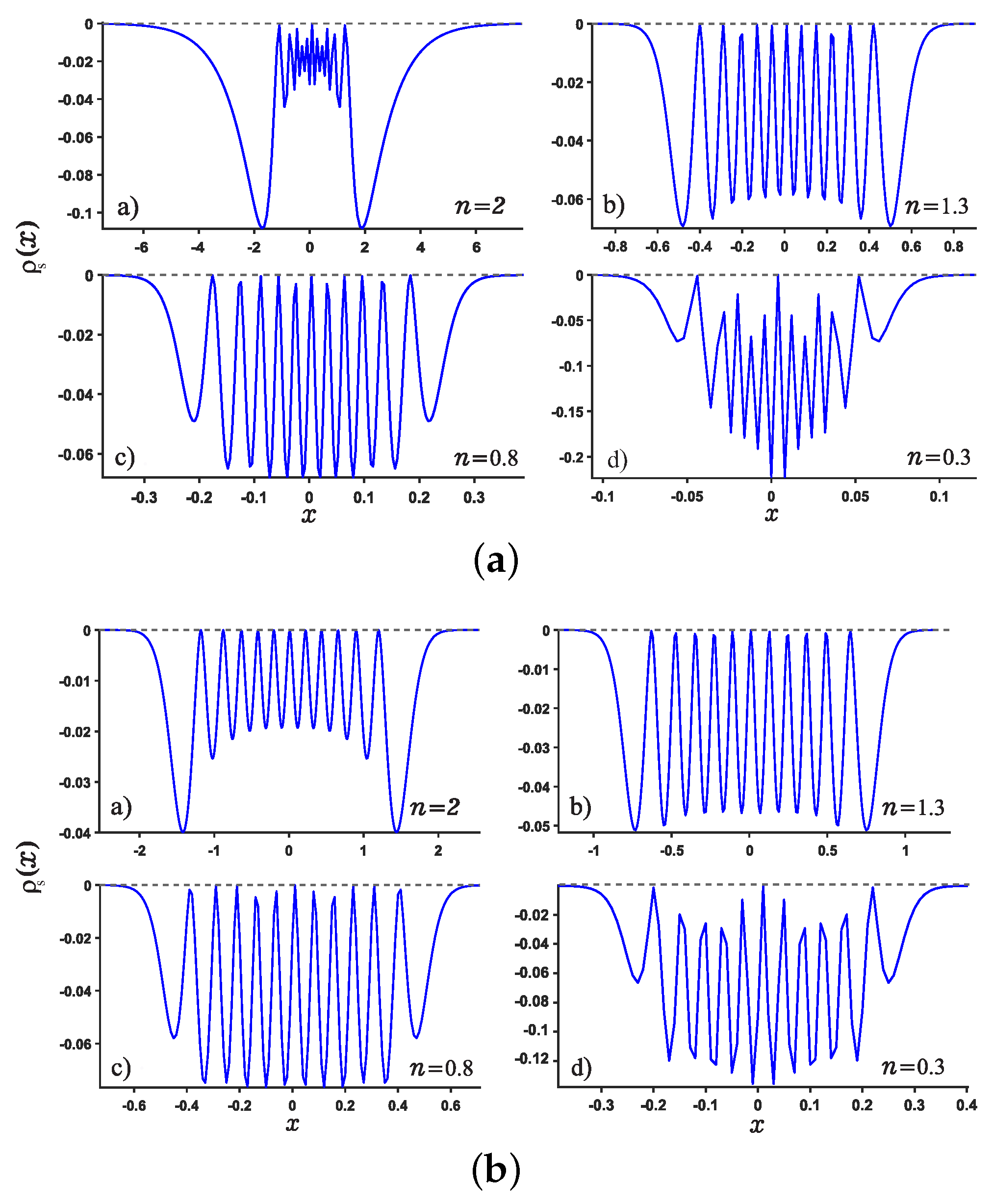
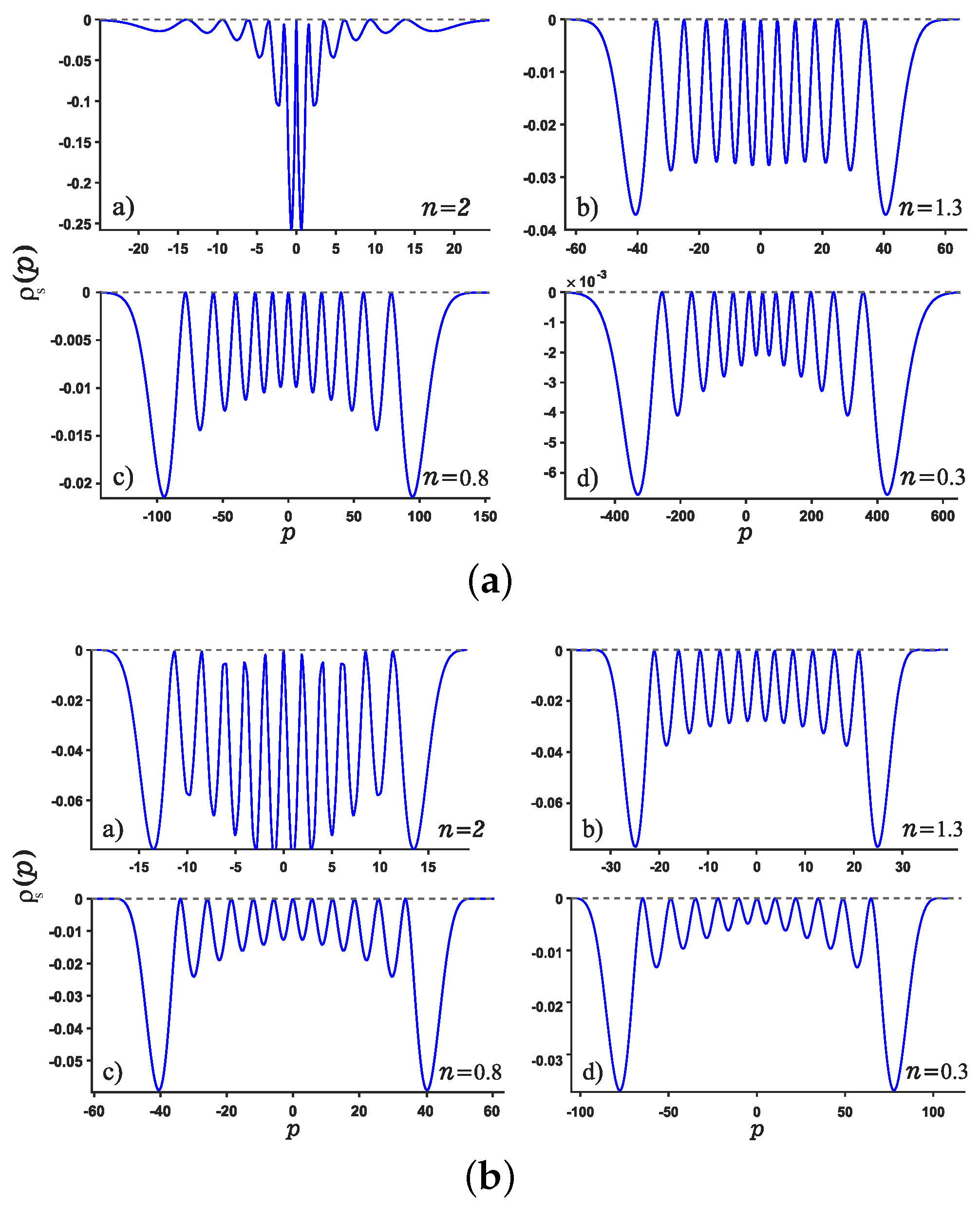
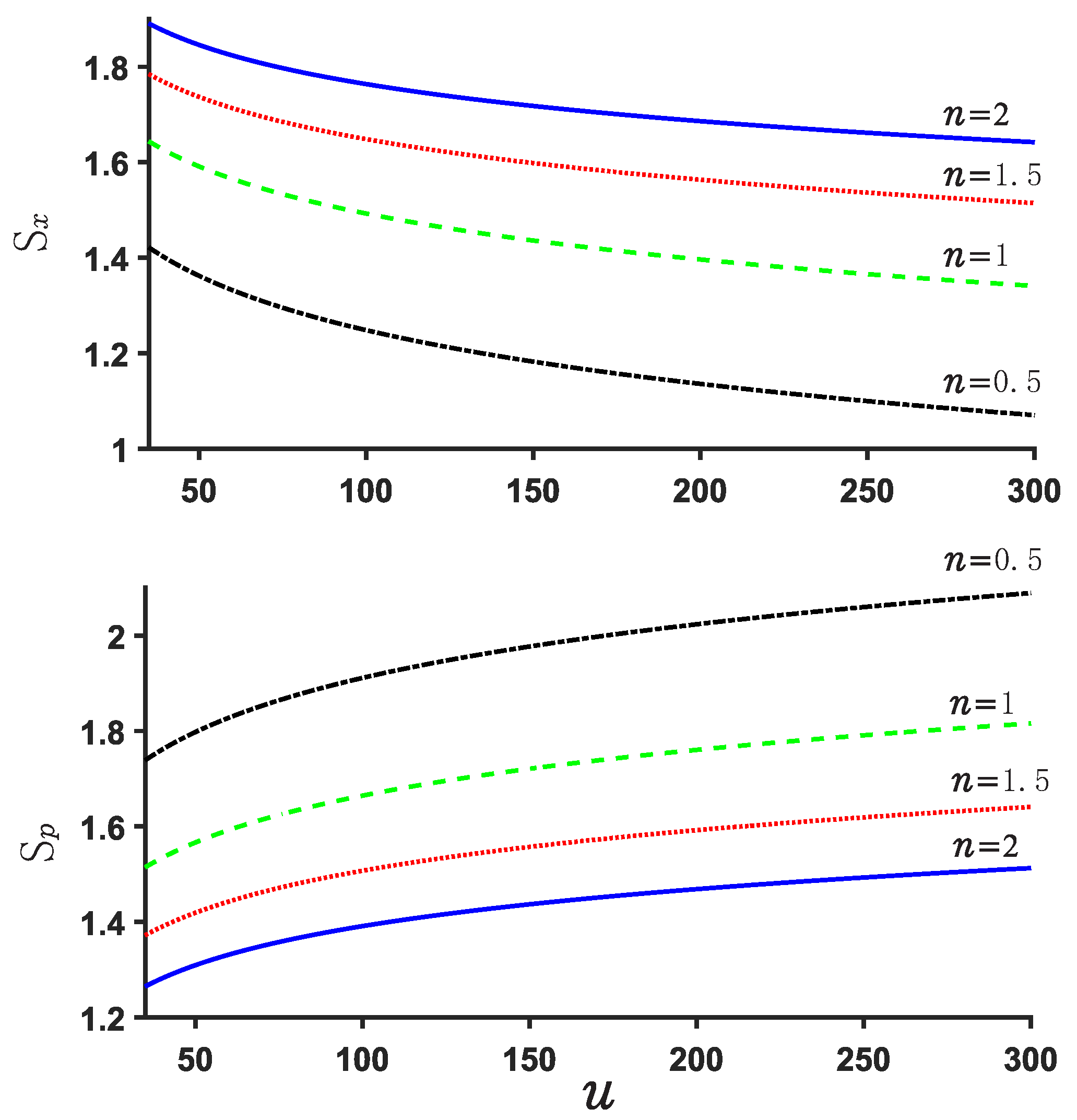
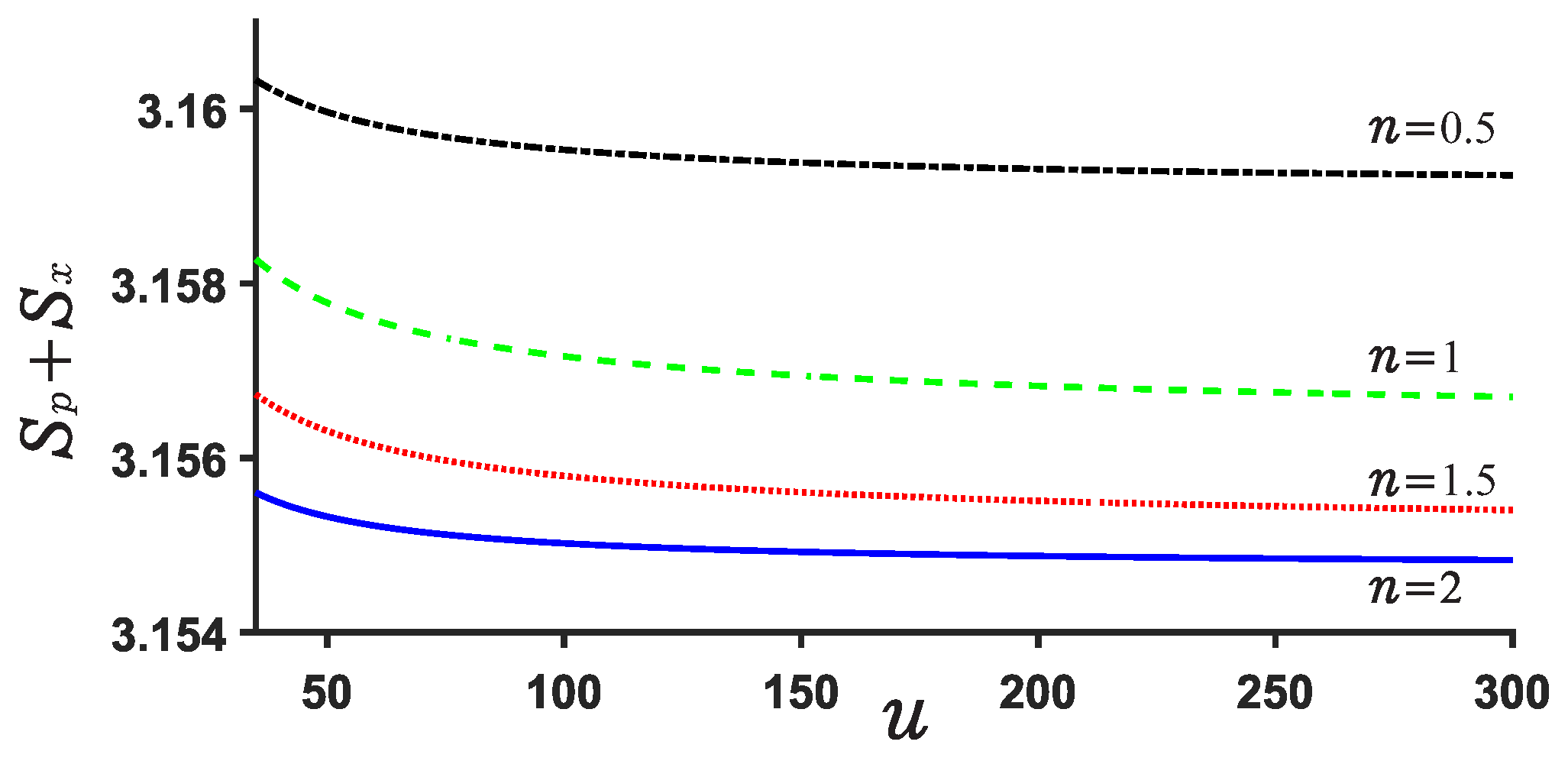
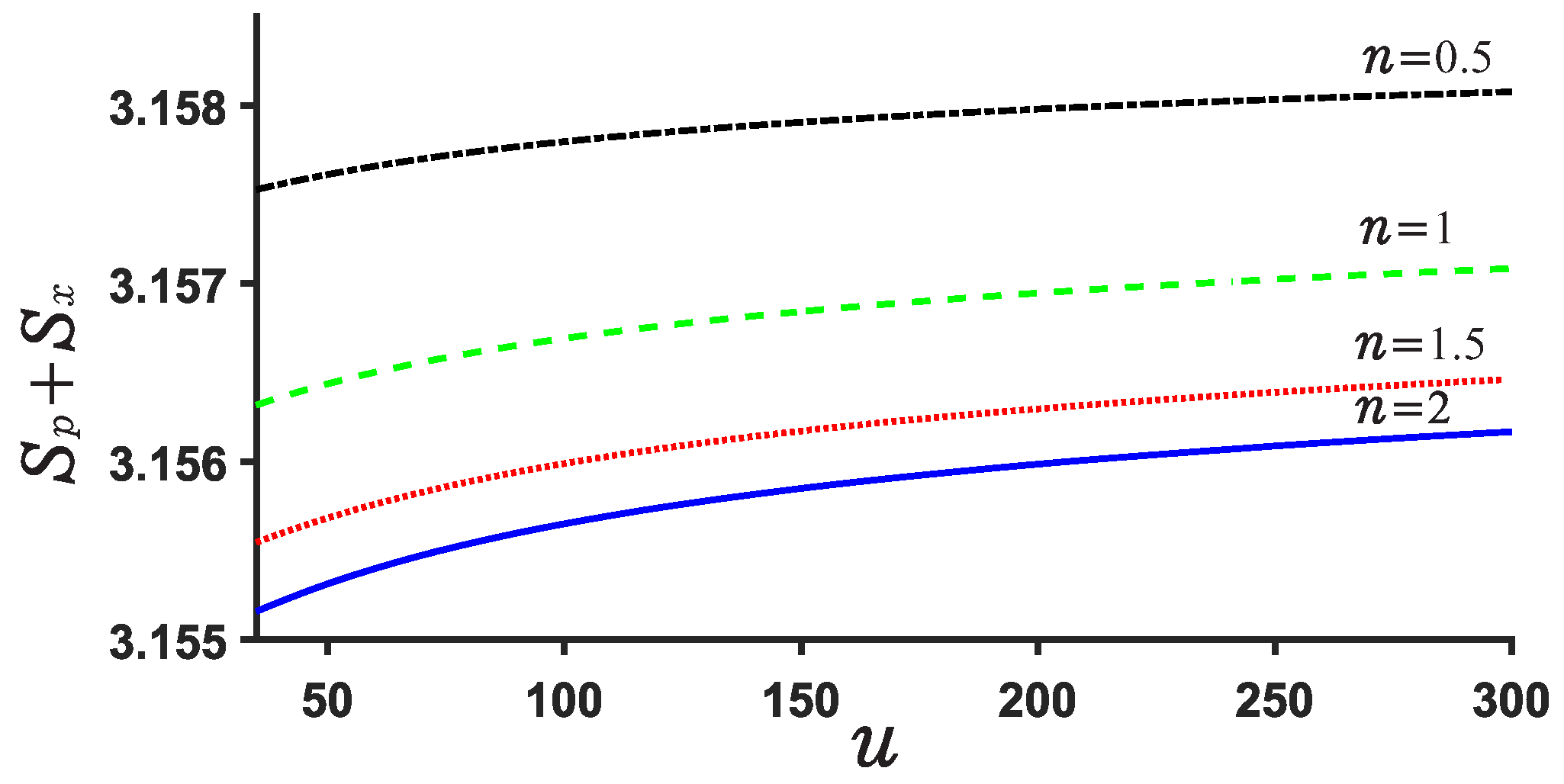
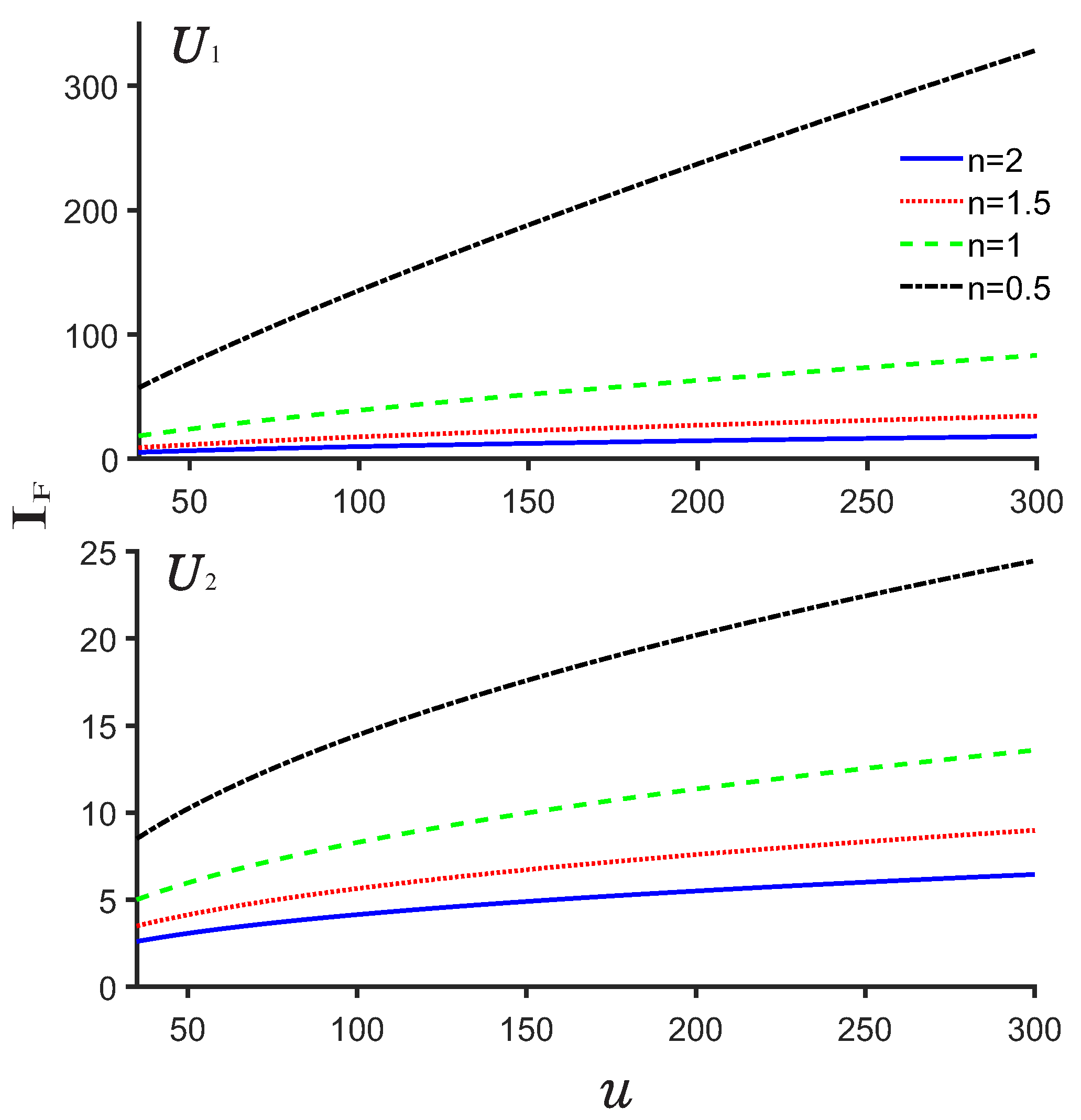
3. Results and Discussions
4. Concluding Remarks
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Shannon, C.E. A Mathematical Theory of Communication. Bell Syst. Tech. J. 1948, 27, 379. [Google Scholar] [CrossRef]
- Dehesa, J.S.; Martinez-Finkelshtein, A.; Sorokin, V.N. Information-theoretic measures for Morse and Pöschl-Teller potentials. Mol. Phys. 2006, 104, 613. [Google Scholar] [CrossRef]
- Aydiner, E.; Orta, C.; Sever, R. Quantum information entropies of the eigenstates of the Morse potential. Int. J. Mod. Phys. B 2008, 22, 231. [Google Scholar] [CrossRef]
- Yañez, R.J.; van Assche, W.; Dehesa, J.S. Position and momentum information entropies of the D-dimensional harmonic oscillator and hydrogen atom. Phys. Rev. A 1994, 50, 3065. [Google Scholar] [CrossRef] [PubMed]
- Majernik, V.; Opatrny, T. Entropic uncertainty relations for a quantum oscillator. J. Phys. A 1996, 29, 2187. [Google Scholar] [CrossRef]
- Coffey, M.W. Semiclassical position and momentum information entropy for sech2 and a family of rational potentials. Can. J. Phys. 2007, 85, 733. [Google Scholar] [CrossRef]
- Patil, S.H.; Sen, K.D. Net information measures for modified Yukawa and Hulthén potentials. Int. J. Quantum Chem. 2007, 107, 1864. [Google Scholar] [CrossRef]
- Sun, G.H.; Dong, S.H. Quantum information entropies of the eigenstates for a symmetrically trigonometric Rosen-Morse potential. Phys. Scr. 2013, 87, 045003. [Google Scholar] [CrossRef]
- Yañez-Navarro, G.; Sun, G.H.; Dytrich, T.; Launey, K.D.; Dong, S.H.; Draayer, J.P. Quantum information entropies for position-dependent mass Schrödinger problem. Ann. Phys. 2014, 348, 153. [Google Scholar] [CrossRef]
- Hazra, R.K.; Ghosh, M.; Bhattacharyya, S.P. Information entropy and level-spacing distribution based signatures of quantum chaos in electron doped 2D single carrier quantum dots. Chem. Phys. Lett. 2008, 460, 209. [Google Scholar] [CrossRef]
- Sun, G.H.; Dong, S.H.; Launey, K.D.; Dytrych, T.; Draayer, J.P. Shannon information entropy for a hyperbolic double-well potential. Int. J. Quantum Chem. 2015, 115, 891. [Google Scholar] [CrossRef]
- Majernik, V.; Majernikova, E. Standard and entropic uncertainty relations of the finite well. J. Phys. A 2002, 35, 5751. [Google Scholar]
- Song, X.D.; Sun, G.H.; Dong, S.H. Shannon information entropy for an infinite circular well. Phys. Lett. A 2015, 379, 1402. [Google Scholar] [CrossRef]
- Sun, G.H.; Popov, D.; Camacho-Nieto, O.; Dong, S.H. Shannon information entropies for position-dependent mass Schrödinger problem with a hyperbolic well. Chin. Phys. B 2015, 24, 100303. [Google Scholar]
- Sun, G.H.; Dong, S.H.; Saad, N. Quantum information entropies for an asymmetric trigonometric Rosen-Morse potential. Ann. Phys. 2013, 525, 934. [Google Scholar] [CrossRef]
- Sun, G.H.; Aoki, M.A.; Dong, S.H. Quantum information entropies of the eigenstates for the Pöschl-Teller-like potential. Chin. Phys. B 2013, 22, 050302. [Google Scholar] [CrossRef]
- Song, X.D.; Dong, S.H.; Zhang, Y. Quantum information entropy for one-dimensional system undergoing quantum phase transition. Chin. Phys. B 2016, 25, 050302. [Google Scholar] [CrossRef]
- Angulo, J.C.; Antolin, J.; Zarzo, A.; Cuchi, J.C. Maximum-entropy technique with logarithmic constraints: Estimation of atomic radial densities. Eur. Phys. J. D 1999, 7, 479. [Google Scholar] [CrossRef]
- Orlowski, A. Information entropy and squeezing of quantum fluctuations. Phys. Rev. A 1997, 56, 2545. [Google Scholar] [CrossRef]
- Salazar, S.J.C.; Laguna, H.G.; Prasad, V.; Sagar, R.P. Shannon-information entropy sum in the confined hydrogenic atom. Int. J. Quantum Phys. 2020, 120, e26188. [Google Scholar] [CrossRef]
- Ikot, A.N.; Rampho, G.J.; Amadi, P.O.; Okorie, U.S.; Sithole, M.J.; Lekala, M.L. Theoretic quantum information entropies for the generalized hyperbolic potential. Int. J. Quantum Phys. 2020, 120, e26410. [Google Scholar] [CrossRef]
- Onate, C.A.; Onyeaju, M.C.; Abolarinwa, A.; Lukman, A.F. Analytical determination of theoretic quantities for multiple potential. Sci. Rep. 2020, 10, 17542. [Google Scholar] [CrossRef] [PubMed]
- Salazar, S.J.C.; Laguna, H.G.; Dahiya, B.; Prasad, V.; Sagar, R.P. Shannon information entropy sum of the confined hydrogenic atom under the influence of an electric field. Eur. Phys. J. D 2021, 75, 127. [Google Scholar] [CrossRef]
- Dehesa, J.S.; Belega, E.D.; Toranzo, I.V.; Aptekarev, A.I. The Shannon entropy of high-dimensional hydrogenic and harmonic systems. Int. J. Quantum Chem. 2019, 119, e25977. [Google Scholar] [CrossRef]
- Mukherjee, N.; Roy, A.K. Information-entropic measures in free and confined hydrogen atom. Int. J. Quantum Chem. 2018, 118, e25596. [Google Scholar] [CrossRef]
- Ghosal, A.; Mukherjee, N.; Roy, A.K. Information entropic measures of a quantum harmonic oscillator in symmetric and asymmetric confinement within an impenetrable box. Ann. Phys. 2016, 528, 796. [Google Scholar] [CrossRef]
- Zhang, L.; Gong, L.Y.; Tong, P.Q. The geometric mean density of states and its application to one-dimensional nonuniform systems. Eur. Phys. J. B 2011, 80, 485. [Google Scholar] [CrossRef]
- Hiramoto, H.; Kohmoto, M. New Localization in a Quasiperiodic System. Phys. Rev. Lett. 1989, 62, 2714. [Google Scholar] [CrossRef]
- Gil-Barrera, C.A.; Santana-Carrillo, R.; Sun, G.H.; Dong, S.H. Quantum Information Entropies on Hyperbolic Single Potential Wells. Entropy 2022, 24, 604. [Google Scholar] [CrossRef]
- Santana-Carrillo, R.; Dong, Q.; Sun, G.H.; Silva-Ortigoza, R.; Dong, S.H. Shannon entropy of asymmetric rectangular multiple well with unequal width barrier. Results Phys. 2022, 33, 105109. [Google Scholar] [CrossRef]
- Shi, Y.J.; Sun, G.H.; Tahir, F.; Ahmadov, A.I.; He, B.; Dong, S.H. Quantum information measures of infinite spherical well. Mod. Phys. Lett. A 2018, 16, 1850088. [Google Scholar] [CrossRef]
- Sarma, S.D.; He, S.; Xie, X.C. Mobility Edge in a Model One-Dimensional Potential. Phys. Rev. Lett. 1988, 61, 2144. [Google Scholar] [CrossRef] [PubMed]
- Laskin, N. Fractional quantum mechanics. Phys. Rev. E 2000, 63, 3135. [Google Scholar] [CrossRef] [PubMed]
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations; Elsevier Science Ltd.: Amsterdam, The Netherlands, 2006. [Google Scholar]
- Celik, C.; Duman, M. Crank-Nicolson method for the fractional diffusion equation with the Riesz fractional derivative. J. Comput. Phys. 2012, 231, 1743. [Google Scholar] [CrossRef]
- Jumarie, G. Fractional Differential Calculus for Non-Differentiable Functions: Mechanics, Geometry, Stochastics, Information Theory; LAP LAMBERT Academic Publishing: Saarbrucken, Germany, 2013. [Google Scholar]
- Dong, J.P.; Xu, M.Y. Some solutions to the space fractional Schrödinger equation using momentum representation method. J. Math. Phys. 2007, 48, 072105. [Google Scholar] [CrossRef]
- El-Nabulsi, R.A. Some implications of position-dependent mass quantum fractional Hamiltonian in quantum mechanics. Eur. Phys. J. Plus 2019, 134, 192. [Google Scholar] [CrossRef]
- Kirichenko, E.V.; Stephanovich, V.A. Confinement of Lévy flights in a parabolic potential and fractional quantum oscillator. Phys. Rev. E 2018, 98, 052127. [Google Scholar] [CrossRef]
- Medina, L.Y.; Núñez-Zarur, F.; Pérez-Torres, J.F. Nonadiabatic effects in the nuclear probability and flux densities through the fractional Schrödinger equation. Int. J. Quantum Chem. 2019, 119, e25952. [Google Scholar] [CrossRef]
- Zhang, Y.; Liu, X.; Belic, M.R.; Zhong, W. Propagation Dynamics of a Light Beam in a Fractional Schrödinger Equation. Phys. Rev. Lett. 2015, 115, 180403. [Google Scholar] [CrossRef]
- Ghalandari, M.; Solaimani, M. Wave transport in fractional Schrödinger equations. Opt. Quantum Electron. 2019, 51, 303. [Google Scholar] [CrossRef]
- Zhang, Y.; Wang, R.; Zhong, H.; Zhang, J. Resonant mode conversions and Rabi oscillations in a fractional Schrödinger equation. Opt. Expr. 2017, 25, 32401. [Google Scholar] [CrossRef]
- Chen, M.; Zeng, S.; Lu, D.; Hu, W.; Guo, Q. Optical solitons, self-focusing, and wave collapse in a space-fractional Schrödinger equation with a Kerr-type nonlinearity. Phys. Rev. E 2018, 98, 022211. [Google Scholar] [CrossRef] [PubMed]
- Solaimani, M.; Dong, S.H. Quantum Information Entropies of Multiple Quantum Well Systems in Fractional Schrödinger Equations. Int. J. Quantum Chem. 2020, 120, e26113. [Google Scholar] [CrossRef]
- Hartmann, R.R.; Robinson, N.J.; Portnoi, M.E. Smooth electron waveguides in graphene. Phys. Rev. B 2010, 81, 245431. [Google Scholar] [CrossRef]
- Downing, C.A.; Portnoi, M.E. One-dimensional Coulomb problem in Dirac materials. Phys. Rev. A 2014, 90, 052116. [Google Scholar] [CrossRef]
- Hartmann, R.R.; Portnoi, M.E. Quasi-exact solution to the Dirac equation for the hyperbolic-secant potential. Phys. Rev. A 2014, 89, 012101. [Google Scholar] [CrossRef]
- Hassanabadi, H.; Zarrinkamar, S.; Yazarloo, B.H. The nonrelativistic oscillator strength of a hyperbolic-type potential. Chin. Phys. B 2013, 22, 060202. [Google Scholar] [CrossRef]
- Wang, X.H.; Chen, C.Y.; You, Y.; Lu, F.L.; Sun, D.S.; Dong, S.H. Exact solutions of the Schrödinger equation for a class of hyperbolic potential well. Chin. Phys. B 2022, 31, 040301. [Google Scholar] [CrossRef]
- Press, W.H.; Teukolsky, S.A.; Vetterling, W.T.; Flanner, B.P. Numerical Recipes, 3rd ed.; Cambridge University Press: Cambridge, UK; New York, NY, USA, 2007. [Google Scholar]
- Beckner, W. Inequalities in Fourier Analysis. Ann. Math. 1975, 102, 159. [Google Scholar] [CrossRef]
- Bialynicki-Birula, I.; Mycielski, J. Uncertainty relations for information entropy in wave mechanics. Commun. Math. Phys. 1975, 44, 129. [Google Scholar] [CrossRef]
- Sears, S.B.; Parr, R.G.; Dinur, U. On the Quantum-Mechanical Kinetic Energy as a Measure of the Information in a Distribution. Isr. J. Chem. 1980, 19, 165. [Google Scholar]
- Falaye, B.J.; Serrano, F.A.; Dong, S.H. Fisherinformation for the position-dependent mass Schrödinger system. Phys. Lett. A 2016, 380, 267. [Google Scholar] [CrossRef]
- Fisher, R.A. Theory of statistical estimation. Math. Proc. Camb. Philos. Soc. 1925, 22, 700. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Santana-Carrillo, R.; González-Flores, J.S.; Magaña-Espinal, E.; Quezada, L.F.; Sun, G.-H.; Dong, S.-H. Quantum Information Entropy of Hyperbolic Potentials in Fractional Schrödinger Equation. Entropy 2022, 24, 1516. https://doi.org/10.3390/e24111516
Santana-Carrillo R, González-Flores JS, Magaña-Espinal E, Quezada LF, Sun G-H, Dong S-H. Quantum Information Entropy of Hyperbolic Potentials in Fractional Schrödinger Equation. Entropy. 2022; 24(11):1516. https://doi.org/10.3390/e24111516
Chicago/Turabian StyleSantana-Carrillo, R., Jesus S. González-Flores, Emilio Magaña-Espinal, Luis F. Quezada, Guo-Hua Sun, and Shi-Hai Dong. 2022. "Quantum Information Entropy of Hyperbolic Potentials in Fractional Schrödinger Equation" Entropy 24, no. 11: 1516. https://doi.org/10.3390/e24111516
APA StyleSantana-Carrillo, R., González-Flores, J. S., Magaña-Espinal, E., Quezada, L. F., Sun, G.-H., & Dong, S.-H. (2022). Quantum Information Entropy of Hyperbolic Potentials in Fractional Schrödinger Equation. Entropy, 24(11), 1516. https://doi.org/10.3390/e24111516








