Non-Equilibrium Entropy and Irreversibility in Generalized Stochastic Loewner Evolution from an Information-Theoretic Perspective
Abstract
:1. Introduction
2. Model
2.1. Chordal Loewner Evolution
2.2. Langevin Dynamics as a Driving Function
3. General Formulation
3.1. Equilibrium Condition on Mathematical Plane
3.2. Entropy Production in Physical Plane
3.3. Jarzynski Equality for Generalized SLE Curve
3.4. KL Divergence Approach
3.5. Relative Loewner Entropy
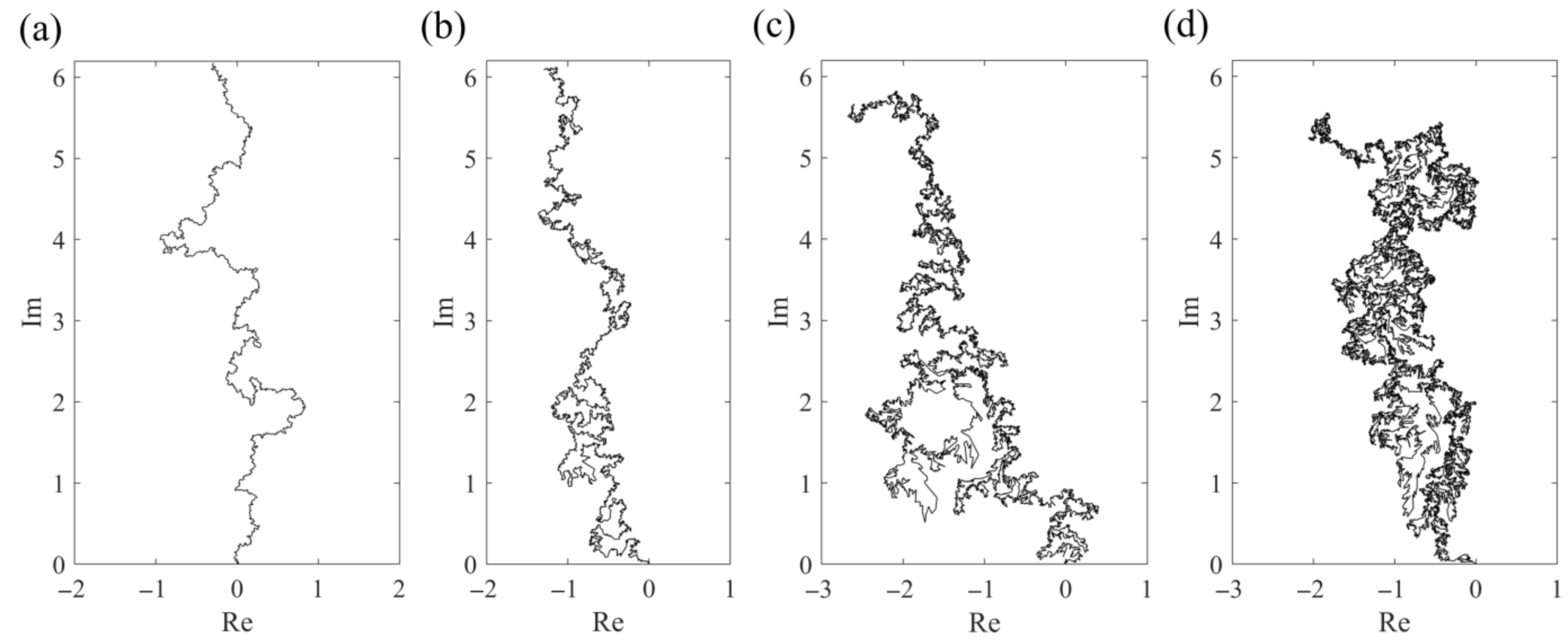
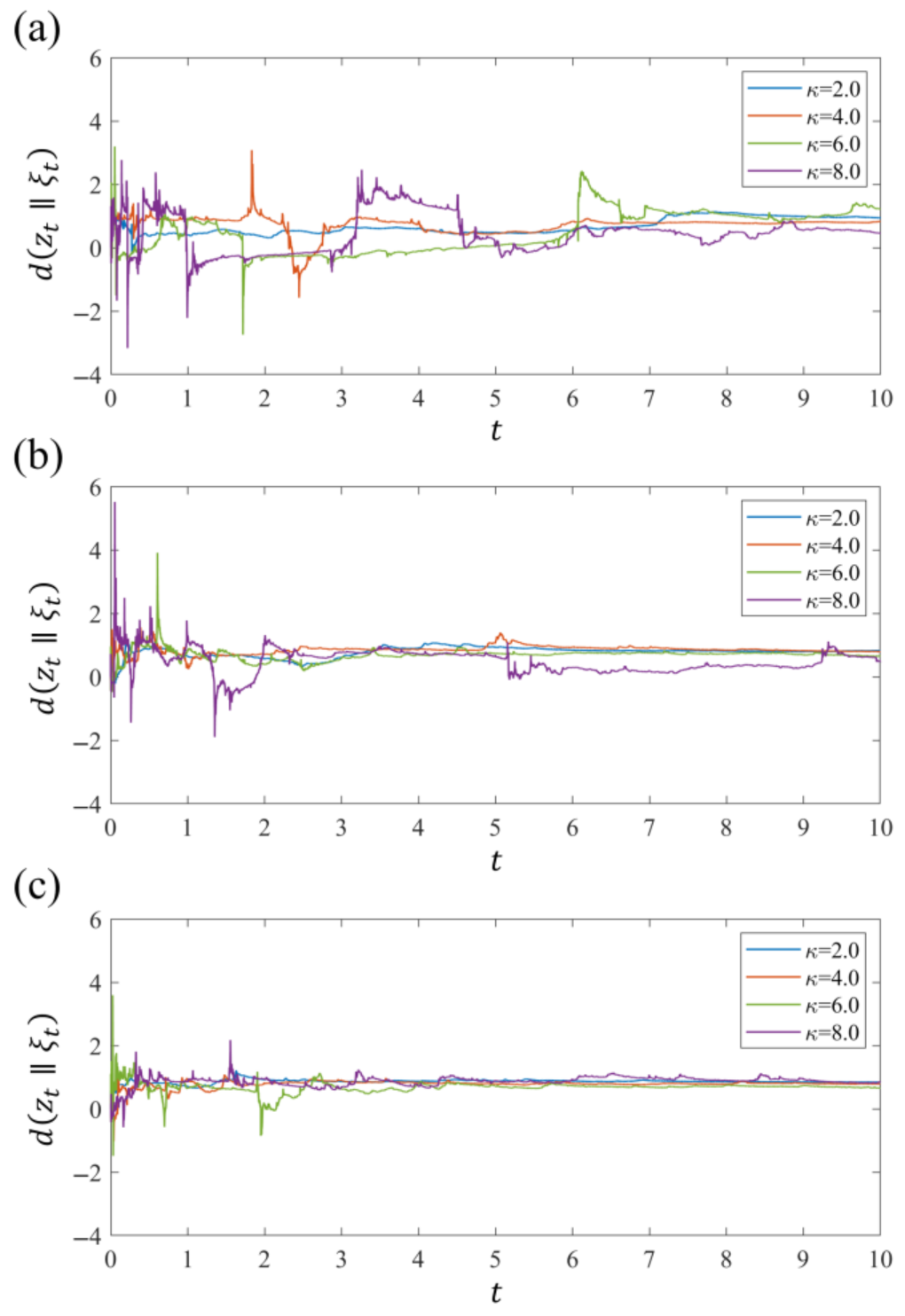
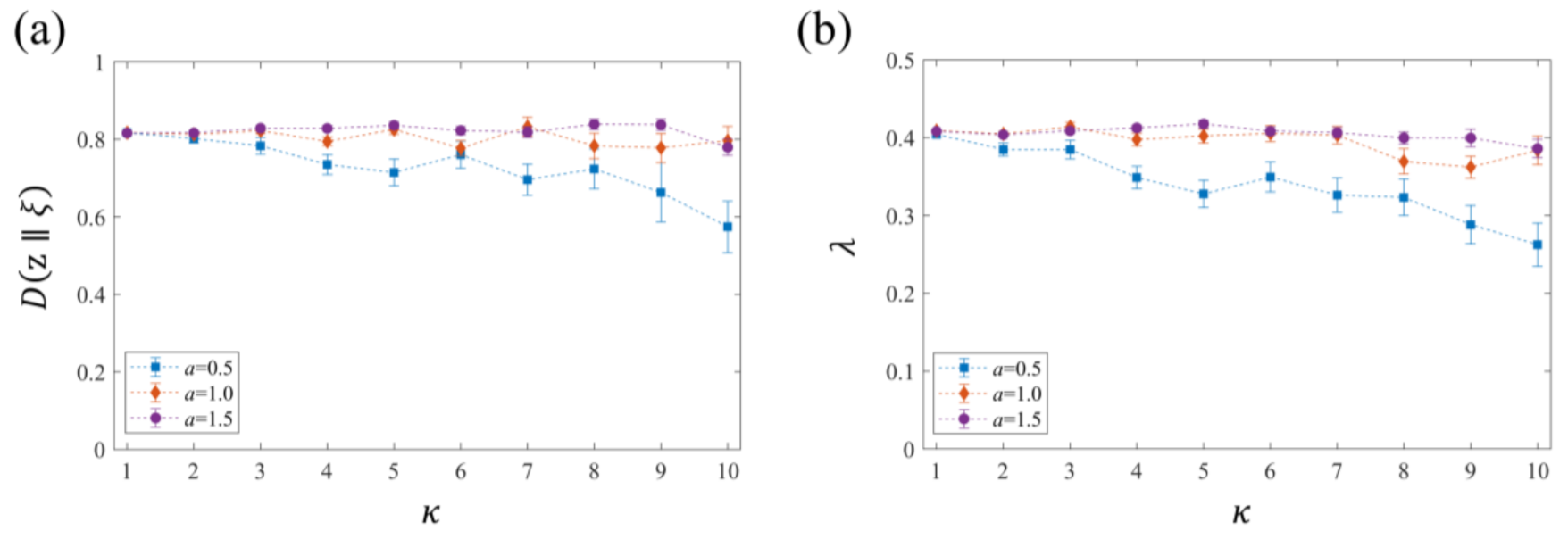
4. Numerical Tests
5. Discussion
- The Jarzynski equality and the second law of thermodynamics were generalized in terms of information theory. Our result in Equation (41) is an extension of Seifert’s expression (see refs. [10,19]). Furthermore, the term can also be interpreted as the feedback information term, denoted as in ref. [23], in Sagawa’s information thermodynamics. Hence, incorporating the relaxation process of an equilibrium state into the theory of the non-equilibrium dynamics enables us to extend the existing thermodynamical laws in an information-theoretic sense. This means that for an arbitrary 2D trajectory on in our model, the validity of the second law in the usual sense is supported by the complete time reversibility of the corresponding driving function, and otherwise (i.e., if the driving function includes several irreversible characters), we must reuptake the generalized second law .
- The entropy describing the non-equilibrium states of the individual trajectories is decomposed into additive and non-additive parts. This provides us with a novel non-equilibrium entropic measure, which we refer to as the relative Loewner entropy. In the sense that the non-equilibrium ensemble is decomposed into an equilibrium ensemble and a certain function, our result in Equation (43) is analogous to the result of Penrose et al. [55].
- If the driving function is in an equilibrium state, the relative Loewner entropy is used to determine the non-equilibrium properties (i.e., non-stationarity and changing rate of Gibbs entropy) of the 2D trajectories in the physical plane. This quantity indicates the phase space deformation under the conformal map , and is closely related to the Lyapunov-type exponent.
- If the entropy (information) of the driving function is completely communicated to the physical plane, the 2D trajectories are in the equilibrium states.
- The non-equilibrium property of the trajectories is induced by the incomplete communication of the entropy (information) between the physical and mathematical planes.
- The driving function can work as Maxwell’s Demon in the sense that it can control the feedback information .
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Penrose, O. Foundations of statistical mechanics. Rep. Prog. Phys. 1979, 42, 1937–2006. [Google Scholar] [CrossRef]
- Lindblad, C. Non-Equilibrium Entropy and Irreversibility; Kluwer: Amsterdam, The Netherlands, 1983. [Google Scholar]
- Gallavotti, G. Nonequilibrium and Irreversibility; Springer: Berlin, Germany, 2014. [Google Scholar] [CrossRef] [Green Version]
- Nicolis, G.; Prigogine, I. Self-Organization in Nonequilibrium Systems: From Dissipative Structures to Order through Fluctuations; Wiley: New York, NY, USA, 1977. [Google Scholar]
- Ruelle, D. Positivity of entropy production in nonequilibrium statistical mechanics. J. Stat. Phys. 1996, 85, 1–23. [Google Scholar] [CrossRef] [Green Version]
- Daems, D.; Nicolis, G. Entropy production and phase space volume contraction. Phys. Rev. E 1999, 59, 4000–4006. [Google Scholar] [CrossRef]
- Tomé, T. Entropy production in nonequilibrium systems described by a Fokker-Planck equation. Braz. J. Phys. 2006, 36, 1285–1289. [Google Scholar] [CrossRef] [Green Version]
- Tomé, T.; de Oliveira, M.J. Entropy production in irreversible systems described by a Fokker-Planck equation. Phys. Rev. E 2010, 82, 021120. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Tomé, T.; de Oliveira, M.J. Stochastic Dynamics and Irreversibility; Springer: Heidelberg, Germany, 2015. [Google Scholar]
- Seifert, U. Entropy production along a stochastic trajectory and an integral fluctuation theorem. Phys. Rev. Lett. 2005, 95, 040602. [Google Scholar] [CrossRef] [Green Version]
- Parrondo, J.M.; van den Broeck, C.; Kawai, R. Entropy production and the arrow of time. New J. Phys. 2009, 11. [Google Scholar] [CrossRef]
- Saha, A.; Lahiri, S.; Jayannavar, A.M. Entropy production theorems and some consequences. Phys. Rev. E 2009, 80, 011117. [Google Scholar] [CrossRef]
- Ge, H.; Qian, H. Physical origins of entropy production, free energy dissipation, and their mathematical representations. Phys. Rev. E 2010, 81, 051133. [Google Scholar] [CrossRef] [Green Version]
- Casas, G.A.; Nobre, F.D.; Curado, E.M.F. Entropy production and nonlinear Fokker-Planck equations. Phys. Rev. E 2012, 86, 061136. [Google Scholar] [CrossRef] [Green Version]
- Evans, D.J.; Cohen, E.G.D.; Morriss, G.P. Probability of second law violations in shearing steady states. Phys. Rev. Lett. 1993, 71, 2401. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gallavotti, G.; Cohen, E.G.D. Dynamical ensembles in nonequilibrium statistical mechanics. Phys. Rev. Lett. 1995, 74, 2694–2697. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Crooks, G.E. Entropy production fluctuation theorem and the nonequilibrium work relation for free energy differences. Phys. Rev. E 1999, 60, 2721–2726. [Google Scholar] [CrossRef] [Green Version]
- Kurchan, J. Fluctuation theorem for stochastic dynamics. J. Phys. A Math. Gen. 1998, 31, 3719–3729. [Google Scholar] [CrossRef] [Green Version]
- Seifert, U. Stochastic thermodynamics, fluctuation theorems and molecular machines. Rep. Prog. Phys. 2012, 75, 126001. [Google Scholar] [CrossRef] [Green Version]
- Sekimoto, K. Stochastic Energetics; Springer: Berlin, Germany, 2010. [Google Scholar] [CrossRef]
- Jaynes, E.T. Information theory and statistical mechanics. Phys. Rev. 1957, 106, 620–630. [Google Scholar] [CrossRef]
- Jaynes, E.T. Information theory and statistical mechanics II. Phys. Rev. 1957, 108, 171–190. [Google Scholar] [CrossRef]
- Sagawa, T.; Ueda, M. Generalized Jarzynski equality under nonequilibrium feedback control. Phys. Rev. Lett. 2010, 104, 090602. [Google Scholar] [CrossRef] [PubMed]
- Parrondo, J.M.; Horowitz, J.M.; Sagawa, T. Thermodynamics of information. Nat. Phys. 2015, 11, 131–139. [Google Scholar] [CrossRef]
- Pigolotti, S.; Neri, I.; Roldán, E.; Jülicher, F. Generic properties of stochastic entropy production. Phys. Rev. Lett. 2017, 119, 140604. [Google Scholar] [CrossRef]
- Neri, I.; Roldán, E.; Jülicher, F. Statistics of infima and stopping times of entropy production and applications to active molecular processes. Phys. Rev. X 2017, 7, 011019. [Google Scholar] [CrossRef]
- Li, S.-N.; Cao, B. Mathematical and information-geometrical entropy for phenomenological Fourier and non-Fourier heat conduction. Phys. Rev. E 2017, 96, 032131. [Google Scholar] [CrossRef] [Green Version]
- Tsallis, C. Possible generalization of Boltzmann-Gibbs statistics. J. Stat. Phys. 1988, 52, 479–487. [Google Scholar] [CrossRef]
- Tsallis, C. Introduction to Nonextensive Statistical Mechanics: Approaching a Complex World; Springer Science & Business Media: New York, NY, USA, 2009. [Google Scholar]
- Schramm, O. Scaling limits of loop-erased random walks and uniform spanning trees. Isr. J. Math. 2000, 118, 221–288. [Google Scholar] [CrossRef] [Green Version]
- Rohde, S.; Schramm, O. Basic properties of SLE. Ann. Math. 2005, 161, 883–924. [Google Scholar] [CrossRef] [Green Version]
- Shibasaki, Y.; Saito, M. Entropy flux in stochastic and chaotic Loewner evolutions. J. Phys. Soc. Jpn. 2020, 89, 113801. [Google Scholar] [CrossRef]
- Gruzberg, I.A.; Kadanoff, L.P. The Loewner equation: Maps and shapes. J. Stat. Phys. 2004, 114, 1183–1198. [Google Scholar] [CrossRef] [Green Version]
- Beliaev, D.; Smirnov, S. Harmonic measure and SLE. Commun. Math. Phys. 2009, 290, 577–595. [Google Scholar] [CrossRef] [Green Version]
- Oikonomou, P.; Rushkin, I.; Gruzberg, A.I.; Kadanoff, L.P. Global properties of stochastic Loewner evolution driven by Lévy processes. J. Stat. Mech. Theory Exp. 2008, 2008, P01019. [Google Scholar] [CrossRef] [Green Version]
- Najafi, M.N. Fokker-Planck equation of Schramm-Loewner evolution. Phys. Rev. E 2015, 92, 022113. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Shibasaki, Y.; Saito, M. Loewner evolution driven by one-dimensional chaotic maps. J. Phys. Soc. Jpn. 2020, 89, 054801. [Google Scholar] [CrossRef]
- Jarzynski, C. Nonequilibrium equality for free energy differences. Phys. Rev. Lett. 1997, 78, 2690–2693. [Google Scholar] [CrossRef] [Green Version]
- Kullback, S. Information Theory and Statistics; Courier Corporation: Chelmsford, MA, USA, 1997. [Google Scholar]
- Shiino, M. H-Theorem with generalized relative entropies and the Tsallis statistics. J. Phys. Soc. Jpn. 1998, 67, 3658–3660. [Google Scholar] [CrossRef]
- Qian, H. Relative entropy: Free energy associated with equilibrium fluctuations and nonequilibrium deviations. Phys. Rev. E 2001, 63, 042103. [Google Scholar] [CrossRef] [Green Version]
- Roldán, E.; Parrondo, J.M. Entropy production and Kullback-Leibler divergence between stationary trajectories of discrete systems. Phys. Rev. E 2012, 85, 031129. [Google Scholar] [CrossRef] [Green Version]
- Mazur, P.; Bedeaux, D. Causality, time-reversal invariance and the Langevin equation. Physica A Stat. Mech. Appl. 1991, 173, 155–174. [Google Scholar] [CrossRef]
- Qian, H.; Qian, M.; Tang, X. Thermodynamics of the general diffusion process: Time-reversibility and entropy production. J. Stat. Phys. 2002, 107, 1129–1141. [Google Scholar] [CrossRef] [Green Version]
- Schnakenberg, J. Network theory of microscopic and macroscopic behavior of master equation systems. Rev. Mod. Phys. 1976, 48, 571–585. [Google Scholar] [CrossRef]
- Schwämmle, V.; Nobre, F.D.; Curado, E.M. Consequences of the H theorem from nonlinear Fokker-Planck equations. Phys. Rev. E 2007, 76, 041123. [Google Scholar] [CrossRef] [Green Version]
- Brown, J.W.; Churchill, R.V. Complex Variables and Applications; McGraw-Hill Higher Education: Boston, MA, USA, 2009. [Google Scholar]
- Thomas, J.B. Introduction to Probability; Springer: New York, NY, USA, 1986. [Google Scholar] [CrossRef]
- Touchette, H. When is a quantity additive, and when is it extensive? Physica A 2002, 305, 84–88. [Google Scholar] [CrossRef] [Green Version]
- Shimada, I.; Nagashima, T. A numerical approach to ergodic problem of dissipative dynamical systems. Prog. Theor. Phys. 1979, 61, 1605–1616. [Google Scholar] [CrossRef] [Green Version]
- Sakaguchi, H. A Langevin simulation for the Feynman ratchet model. J. Phys. Soc. Jpn. 1998, 67, 709–712. [Google Scholar] [CrossRef]
- Kubo, R.R.; Toda, M.; Hashitsume, N. Statistical Physics II: Nonequilibrium Statistical Mechanics; Springer: Berlin, Germany, 1985. [Google Scholar]
- Kennedy, T. A fast algorithm for simulating the chordal Schramm–Loewner evolution. J. Stat. Phys. 2007, 128, 1125–1137. [Google Scholar] [CrossRef] [Green Version]
- Kennedy, T. Numerical computations for the Schramm-Loewner evolution. J. Stat. Phys. 2009, 137, 839–856. [Google Scholar] [CrossRef] [Green Version]
- Penrose, O.; Coveney, P.V. Is there a ‘canonical’ non-equilibrium ensemble? Proc. R. Soc. Lond. A 1994, 447, 631–646. [Google Scholar] [CrossRef]
- Rohde, S.; Wang, Y. The Loewner energy of loops and regularity of driving functions. Int. Math. Res. Not. 2021, 2021, 7433–7469. [Google Scholar] [CrossRef] [Green Version]
- Wang, Y. Equivalent descriptions of the loewner energy. Inven. Math. 2019, 218, 573–621. [Google Scholar] [CrossRef] [Green Version]
- Gallavotti, G.; Reiter, W.L.; Yngvason, J. Boltzmann’s Legacy; European Mathematical Society: Zürich, Switzerland, 2008. [Google Scholar]
- Hastings, M.B. Exact multifractal spectra for arbitrary Laplacian random walks. Phys. Rev. Lett. 2002, 88, 055506. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lyklema, J.W.; Evertsz, C.; Pietronero, L. The Laplacian random walk. EPL Europhys. Lett. 1986, 2, 77–82. [Google Scholar] [CrossRef]
- Peliti, L.; Pietronero, L. Random walks with memory. Riv. Nuovo Cim. (1978–1999) 1987, 10, 1–33. [Google Scholar] [CrossRef]
- Shibasaki, Y.; Saito, M. Loewner driving force of the interface in the 2-dimensional Ising system as a chaotic dynamical system. Chaos Interdiscip. J. Nonlinear Sci. 2020, 30, 113130. [Google Scholar] [CrossRef] [PubMed]
- Gherardi, M.; Nigro, A. q-deformed Loewner evolution. J. Stat. Phys. 2013, 152, 452–472. [Google Scholar] [CrossRef] [Green Version]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shibasaki, Y.; Saito, M. Non-Equilibrium Entropy and Irreversibility in Generalized Stochastic Loewner Evolution from an Information-Theoretic Perspective. Entropy 2021, 23, 1098. https://doi.org/10.3390/e23091098
Shibasaki Y, Saito M. Non-Equilibrium Entropy and Irreversibility in Generalized Stochastic Loewner Evolution from an Information-Theoretic Perspective. Entropy. 2021; 23(9):1098. https://doi.org/10.3390/e23091098
Chicago/Turabian StyleShibasaki, Yusuke, and Minoru Saito. 2021. "Non-Equilibrium Entropy and Irreversibility in Generalized Stochastic Loewner Evolution from an Information-Theoretic Perspective" Entropy 23, no. 9: 1098. https://doi.org/10.3390/e23091098
APA StyleShibasaki, Y., & Saito, M. (2021). Non-Equilibrium Entropy and Irreversibility in Generalized Stochastic Loewner Evolution from an Information-Theoretic Perspective. Entropy, 23(9), 1098. https://doi.org/10.3390/e23091098





