In the following, we study the properties of this entropy, and, by representing the algebra on a Hilbert space, we investigate the implications of this formula and its physical interpretations. Different features can be obtained from inequivalent representations of the -algebra .
Given a representation
, it is known that the image
is a
-subalgebra of the operator algebra
[
12]. However, we cannot represent any state
of the original algebra as a state over
. Consider the representative state
This definition makes sense if and only if, for
:
This condition is fulfilled in a faithful representation, where by definition
if and only if
. Condition (
29) is also fulfilled in the GNS representation associated with the state
, where
implies that
. In the following, we compute the entropy (
26) using a faithful representation (and later the GNS representation) and exhibit its connection with the von Neumann entropy of a distinguished representative density matrix in that representation.
4.2. Evaluation in a Faithful Representation
Let us consider a finite-dimensional
-algebra
and a finite-dimensional faithful representation
, that is
Given a state
on
, it can be represented on
by
Let us decompose the representation into irreducible sub-representations
Here,
are irreducible sub-representations. The multiplicity of the sub-representation
is
, and
The elements of
have the form
with
spanning all
, by the structure theorem (see Equation (
10)).
From representation (
41), we can obtain another, more economical faithful representation of the form
where the multiplicities are
for all
i, thus eliminating all the redundancy of our description. For the moment, we stick with the general form (
41), but we clearly expect that our results do not depend on the multiplicity
.
We rewrite the decomposition (
41) in the form
This follows by considering the unitary transformation which acts on each
as
where
is an orthonormal basis of
.
Given a state
over the
-algebra
, by Theorem 2 we can consider the unique representative density matrix
belonging to
such that
Since
is an element of the algebra, it has the form
where
are density matrices of
, and
is a probability vector. Conversely, any density matrix of the form (2) defines a state over
.
Given two states
and
, and their representative density matrices
and
, we have
Therefore, a state
is pure if and only if its density matrix is pure with respect to decompositions in density matrices of
.
Let
be a pure state, and let (
48) be its decomposition. Then, we must have that all
, except for one
i. For example, if
were both different from zero, then we could decompose
into two other density matrices of
. Thus, a pure state
has the form
for some
i, with
being a unit vector of
.
Given a state
over
, let its representative
be in the form (
48). Consider the spectral decomposition of each density matrix
,
and obtain a decomposition of the density matrix
into pure states
with
The weights of this decomposition are
. We show that this is the minimal decomposition, i.e., having the minimal Shannon entropy as in definition (
26), which is then the entropy
of the state
.
Consider a generic decomposition of
into pure states
with
:
We gather the pure states so that
has support in
. Notice that
can be expressed in the canonical form (
48), with
and
where
(if
, then all
and we can drop the corresponding terms in Equation (
54)). The Shannon entropy of the decomposition (
54) is
Here,
is the von Neumann entropy of the density matrix
, which, by Schrödinger’s theorem, is always smaller than the Shannon entropy of any other decomposition of
.
Now, the last line of (
59) is also the Shannon entropy of the decomposition (
52). Therefore, the entropy (
26) reads
This is our main result, which expresses the entropy of a state
over an algebra
in terms of the canonical decomposition (
48) of its distinguished representative density matrix
belonging to a faithful representation (
45) of
. The entropy
is given by the sum of two contributions: the Shannon entropy
of the probability vector
of the weights of the component density matrices
in the irreducible sub-representations plus the average von Neumann entropy of these components. Notice that, as expected, the result does not depend on the arbitrary multiplicities
of the representation.
On the other hand, the von Neumann entropy of the distinguished representative density matrix
in the representation (
45) in general differs from the entropy (
60) of the state
:
Indeed, it contains an additional entropic term due to the redundancy of the representation, that is the presence of multiplicities
. A similar phenomenon appears already at the level of classical thermodynamics in presence of a redundancy, e.g., for identical particles [
40,
41,
42].
The equality between the two entropies is restored if one considers the most economical representation with no multiplicities (
44). In such a case, the entropy of the state
is equal to the von Neumann entropy of its distinguished representative density matrix
and equality (
38) holds. This observation has a major consequence: since
is the von Neumann entropy of the representative density matrix of a representation with no multiplicities, it is a bona fide entropy and possesses all the desired thermodynamic properties; in particular, by Equation (
49), it is a concave function.
We have proved the following theorem which gathers our main results:
Theorem 3 (Entropy of a quantum state)
. Let be a finite dimensional -algebra. For any state ω over define its entropy asThen, is a nonnegative concave function which vanishes on pure states.Moreover, let be a faithful finite-dimensional and multiplicity-free representation of . Given a state ω, let be the unique density matrix such that for all . Then, one haswhere is the von Neumann entropy of ρ. 4.3. Thermodynamic Considerations
In this section, we discuss the physical motivations of the definition (
26) for the entropy of a quantum state
. We make use of thermodynamic considerations by extending to the algebraic framework von Neumann’s beautiful argument, based on the notions of Einstein’s gas and semipermeable walls [
1,
27]. To this purpose, some preliminary considerations are necessary.
There is no immediate definition of eigenstates in the algebraic approach, and yet they are key ingredients in von Neumann’s thermodynamic considerations. Instead, we can consider states that have a definite value for a given observable. If a state
has a definite value for an observable
A, every measurement of this observable will yield the same value
a on it. This can be expressed by saying that
and its variance is zero:
Furthermore, we assume that this property is stable in the sense that if a second measurement of the same observable is performed just after the first, the same result is obtained.
In the following, we consider the faithful representation
of a finite-dimensional
-algebra
, without multiplicities, as given by (
44), namely
with
being irreducible sub-representations. Consider an observable
and let
be its representative. Let
be its eigenstates with eigenvalues
and suppose that
A (and thus
) has non-degenerate spectrum, that is
for
. Now, if the density matrix
is the representative of the state
, then
for some
j, and
. Indeed, Equation (
64) reads
Therefore,
has no support on
whenever
. As a result,
is an eigenvalue of
, say
for some
j, and
is supported on its eigenspace. Thus, we have
We are now ready to apply von Neumann’s argument. In the previous sections we showed that, by considering the faithful multiplicity-free representation (
65), there is a one-to-one correspondence between states
over
and density matrices
belonging to
, and pure states over
correspond to vector states
belonging to
, which, by the above argument, are states with a definite value for a suitable non-degenerate observable. Moreover, we have seen that the entropy of any state
is equal to the von Neumann entropy of its distinguished representative
, as in equality (
38). Therefore, the strategy is to use von Neumann’s argument on the representation
.
Consider an ensemble of
M copies of a system prepared in a state
, represented by the density matrix
. If
M is large enough, we expect the system to follow the laws of thermodynamics. To obtain the entropy of the system, we need to evaluate the heat exchanged along a reversible transformation that brings the system from a reference state
, whose entropy
is assigned to the state
. The entropy is given by
In quantum mechanics, one chooses pure states as the reference states, and sets
. In fact, it can be proved that pure states are isoentropic, and that two pure states can be connected adiabatically [
1]. We show below that this is in general not true in the algebraic description, and that there are states that cannot be transformed into each other in this way.
Let us recall von Neumann’s argument, which makes a clever use of a peculiar feature of quantum mechanics, later on named “quantum Zeno effect” [
43,
44]. Consider two orthogonal vectors
and
in
. We explicitly construct the adiabatic transformation from
to
. Fix an integer
k, and define for
with
and
. Consider a family of non-degenerate self-adjoint operators
such that
is one of the possible eigenvectors. By measuring in sequence the observables corresponding to
on the vector state
one gets
The fraction of states that goes from
to
in the measurement of
is
and
so that for large
k we have a transformation of
into
with probability one. Assuming that in the measurement no heat exchange occurs, we have:
Since the transformation can be repeated in the opposite direction
, we get
This proof works in quantum mechanics, where the algebra of observables is the full algebra
, but has problems for a generic algebra
subject to selection rules, whose representation
is a proper subalgebra of
.
In order for the operator
to be the representative of an observable, we need
to be in
for all
. Since pure states are vector states in a subspace
of (
65),
are elements of
if and only if the vectors
and
in (
69) belong to the same Hilbert space
. Only in this case we can prove that they are isentropic. Otherwise, they cannot be transformed into each other by the procedure described above, and we cannot compare their entropies. Physically, they represent pure states belonging to disjoint phases (or sectors) that cannot be connected by any physical operation.
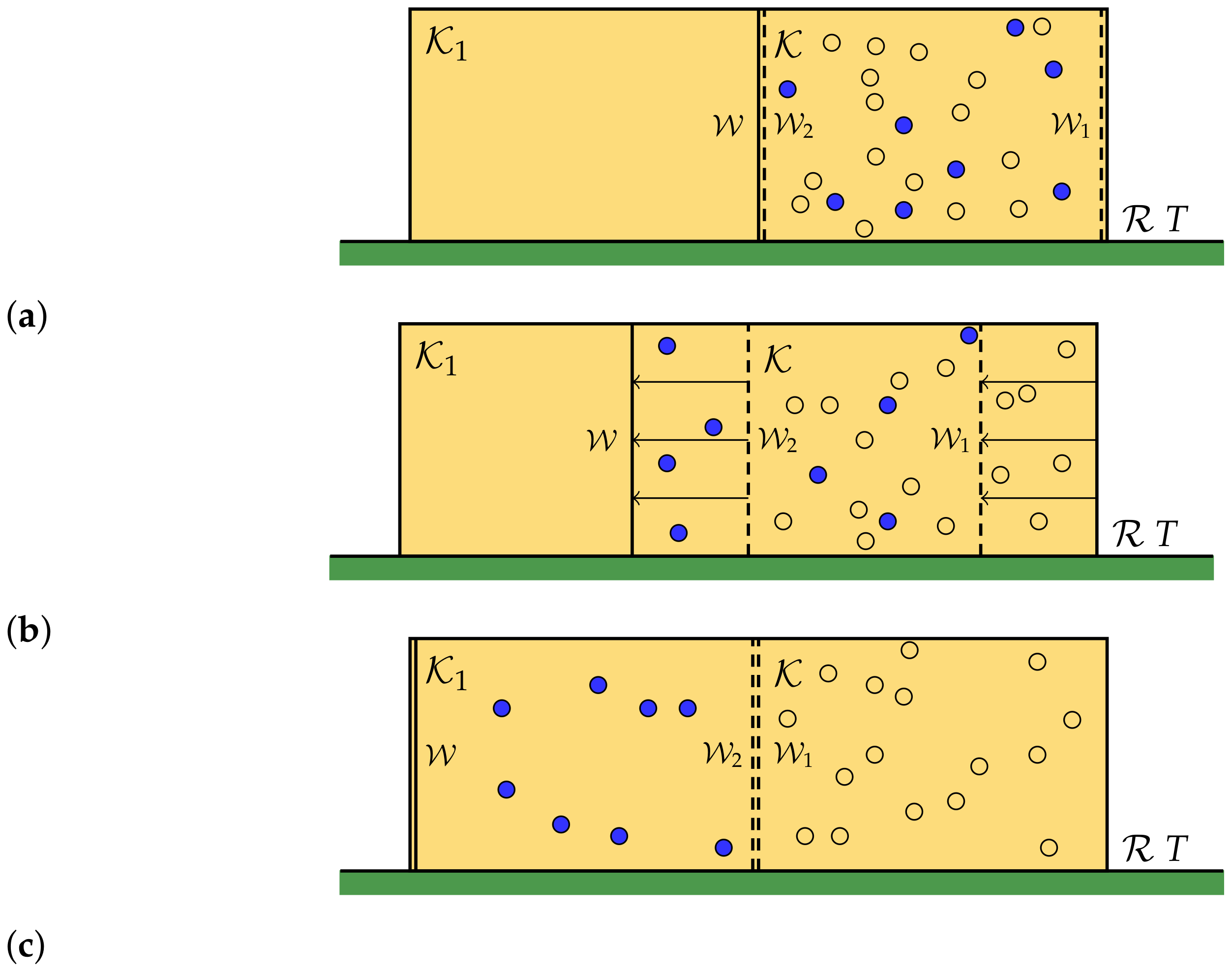
We then call the entropies of the pure states whose representatives are in , respectively. From the entropy of pure states, we obtain the entropy of a generic mixed state. We need to consider a reversible process that brings the ensemble to a final pure state. This is performed by introducing the concept of Einstein’s gas: the copies of the quantum system are inserted into boxes (a box for each copy), which are so thick and massive that the state of the system is not affected by the motion of the boxes. We then insert all these boxes into a larger box , which is kept in contact with a reservoir at temperature T. The boxes behave as a perfect gas if the temperature T is high enough.
Consider the spectral decomposition of the density matrix
corresponding to the state
in the representation
. We get that the decomposition
with
, corresponds to the decomposition into pure states of
,
where the index
i labels different sectors. Define the non-degenerate self-adjoint operator
representing the observable
A, i.e.,
, and for which
are the possible outcomes of a measurement and
are the associated eigenvectors.
To separate the pure components
of the state
represented by
, we use a semipermeable wall, constructed as a wall with some windows on it. In particular, when a box
reaches a window, we let an engine open it and measure the observable
A on the state inside the box. If the result is a given value
, the engine lets the box pass; otherwise, it reflects it. In this way, the wall is transparent for the states
and opaque for the others. Using such a wall, it is possible to separate the pure components (see
Figure 1).
This process is reversible, and we get a final configuration of equal boxes, each containing one of the components
of the gas. We then compress each box isothermally, so that the system will have the same density of the original gas (see
Figure 2). The heat exchanged in each compression is given by
The initial entropy of the gas is therefore
We now need to find the entropy of the final configuration
consisting in separated pure components of the gas. Since entropy is an extensive quantity, it is given by the sum of the entropies of the pure components:
Therefore, we finally get
where equality (
38) is used.
The entropy of the state
obtained by thermodynamic considerations in (
81) differs from
given in (
26) by an additional term,
, which is the average of the arbitrary entropies
assigned to pure states belonging to different phases. By
assuming that pure states belonging to disjoint phases have the same entropy
, we get that the thermodynamic entropy is equal to the entropy
up to an arbitrary constant, which we can set to 0. This is in agreement with the physical meaning of expression (
26), where the entropic content of a state
is obtained exclusively as a result of the mixing process with weights
of pure states
with zero entropy.
4.4. Evaluation via the GNS Construction
In this last section, we compute the entropy (
26) of a quantum state
by using the GNS representation of
. The problem of the ambiguity was studied in this framework by Balachandran, de Queiroz, and Vaidya [
15]. In particular, they described how to represent irreducible sub-representations as decomposition into pure states. This can be generalized for any decomposition.
We start with the following result [
12,
25].
Theorem 4. Let ω be a state and be its GNS representation. Then, the following conditions are equivalent.
Moreover, there is a one to one relation between positive functionals over and majorized by ω and positive operators T on in the commutant and with norm : Notice that here,
is introduced in order to make
a state. Moreover, we say that
is majorized by
if
is positive, that is:
for all
A. Observe that
majorizes
if and only if
for some state
. Therefore, the above theorem links a convex decomposition to operators on a Hilbert space. In particular, one can prove that
is pure if and only if
T is proportional to a projection
in the commutant, and the corresponding sub-representation
is irreducible [
12].
As a result, given a state
, it is equivalent to consider a decomposition into pure states
,
or a decomposition of the identity of the representation in projections
,
with
and
The weights of the decomposition are obtained by evaluating Equation (
86) at
:
Note that, if
for all
i, the projections will be orthogonal to each other, and we obtain a decomposition of the GNS representation into irreducible sub-representations,
This is the description given in [
15].
In the finite dimensional case, a decomposition into irreducible sub-representations always exists, as well as a decomposition into pure states is always possible in a convex set (by Minkowski’s theorem). We can decompose the representation as
using the unitary transformation (
46). By the structure theorem, the representation of the algebra is
and its commutant is
Thus, from (
91), the irreducible projections have the form
for some
i, with
v a unit vector in
.
Therefore, given a family of irreducible projections
, Equation (
85) becomes
with
and
In particular, the index
i labels the sub-representation
considered, while
j labels the different projections in it. From (
93), we get, for all
,
with
Consider now the normalized projection of
on
, namely
where
. By plugging (
94) and (
97) into Equation (
87), we get
with
In general, the decomposition of the identity in Equation (
95) consists of
elements. If
is an orthonormal basis of
, it can be written as
This is an orthonormal relation between
vectors of length
. We can expand the Hilbert space adding
vectors
, and obtain a complete orthonormal system in Equation (
100). Therefore, by setting
we also get complete orthonormal system in
. The operators
are defined so that they vanish on
for
.
We now evaluate the Shannon entropy of the weight
in (
98):
Since
is normalized, the term in the second line becomes
The term in the last sum takes its minimal value when
are the eigenvectors of the reduced density matrix
, becoming its von Neumann entropy. We finally get
where formula (
60) is used.
It is clear that we have re-obtained by this approach the results previously obtained by using a faithful representation. However, some properties of the entropy—concavity, for example—are somewhat hidden in this description. Nevertheless, the derivation via the GNS construction might prove itself to be useful if one would like to extend these results to the infinite-dimensional case.