Asymptotic Entanglement Sudden Death in Two Atoms with Dipole–Dipole and Ising Interactions Coupled to a Radiation Field at Non-Zero Detuning
Abstract
1. Introduction
2. The Model and Its Exact Solution
3. Time Evolution of Entanglement and Population Inversion
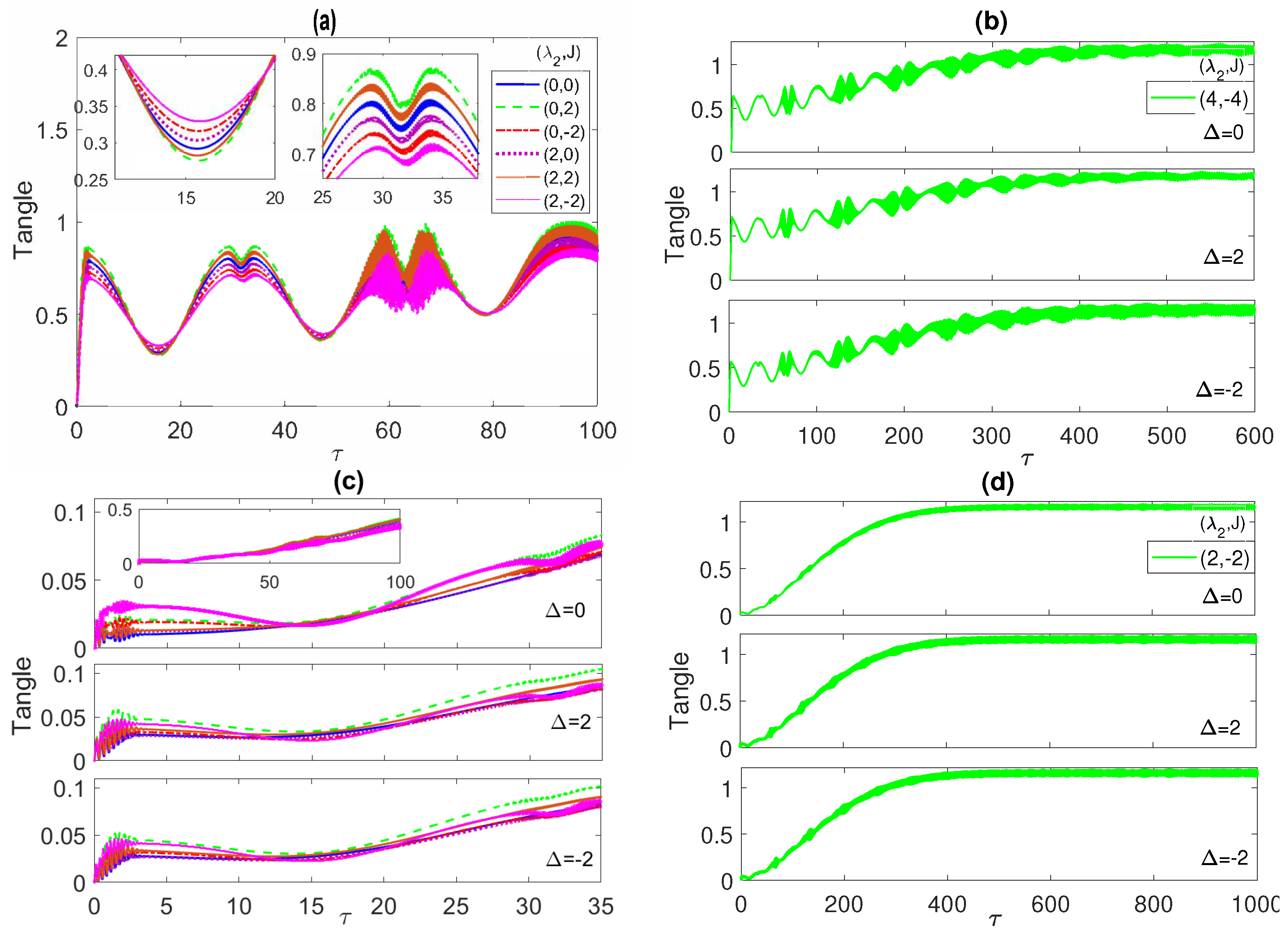
3.1. Initial Bell State
3.2. Partially Entangled Initial (W) State
3.3. Disentangled Initial State
4. Quantum Correlation between the Two Atoms and the Radiation Field
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Jaynes, E.T.; Cummings, F.W. Comparison of quantum and semiclassical radiation theories with application to the beam maser. Proc. IEEE 1963, 51, 89–109. [Google Scholar] [CrossRef]
- Nielsen, M.A.; Chuang, I.L. Quantum Computation and Quantum Information, 1st ed.; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Buluta, I.; Ashhab, S.; Nori, F. Natural and artificial atoms for quantum computation. Rep. Prog. Phys. 2011, 74, 104401. [Google Scholar] [CrossRef]
- Xiang, Z.-L.; Ashhab, S.; You, J.Q.; Nori, F. Hybrid quantum circuits: Superconducting circuits interacting with other quantum systems. Rev. Mod. Phys. 2013, 85, 623. [Google Scholar] [CrossRef]
- Lodahl, P.; Mahmoodian, S.; Stobbe, S. Interfacing single photons and single quantum dots with photonic nanostructures. Rev. Mod. Phys. 2015, 87, 347. [Google Scholar] [CrossRef]
- Wendin, G. Quantum information processing with superconducting circuits: A review. Rep. Prog. Phys. 2017, 80, 106001. [Google Scholar] [CrossRef] [PubMed]
- Yang, C.-P.; Chu, S.-I.; Han, S. Possible realization of entanglement, logical gates, and quantum-information transfer with superconducting-quantum-interference-device qubits in cavity QED. Phys. Rev. A 2003, 67, 042311. [Google Scholar] [CrossRef]
- Yang, C.-P.; Chu, S.-I.; Han, S. Quantum information transfer and entanglement with SQUID qubits in cavity QED: A dark-state scheme with tolerance for nonuniform device parameter. Phys. Rev. Lett. 2004, 92, 117902. [Google Scholar] [CrossRef] [PubMed]
- Blais, A.; Huang, R.-S.; Wallraff, A.; Girvin, S.M.; Schoelkopf, R.J. Cavity quantum electrodynamics for superconducting electrical circuits: An architecture for quantum computation. Phys. Rev. A 2004, 69, 062320. [Google Scholar] [CrossRef]
- Yamamoto, T.; Pashkin, Y.A.; Astafiev, O.; Nakamura, Y.; Tsai, J.-S. Demonstration of conditional gate operation using superconducting charge qubits. Nature 2003, 425, 941–944. [Google Scholar] [CrossRef]
- Majer, J.B.; Paauw, F.G.; Ter Haar, A.C.J.; Harmans, C.J.P.M.; Mooij, J.E. Spectroscopy on two coupled superconducting flux qubits. Phys. Rev. Lett. 2005, 94, 090501. [Google Scholar] [CrossRef]
- Steffen, M.; Ansmann, M.; Bialczak, R.C.; Katz, N.; Lucero, E.; McDermott, R.; Neeley, M.; Weig, E.M.; Cleland, A.N.; Martinis, J.M. Measurement of the entanglement of two superconducting qubits via state tomography. Science 2006, 313, 1423–1425. [Google Scholar] [CrossRef] [PubMed]
- Van der Ploeg, S.H.W.; Izmalkov, A.; van den Brink, A.M.; Hübner, U.; Grajcar, M.; Il’Ichev, E.; Meyer, H.-G.; Zagoskin, A.M. Controllable coupling of superconducting flux qubits. Phys. Rev. Lett. 2007, 98, 057004. [Google Scholar] [CrossRef] [PubMed]
- Niskanen, A.O.; Harrabi, K.; Yoshihara, F.; Nakamura, Y.; Lloyd, S.; Tsai, J.S. Quantum coherent tunable coupling of superconducting qubits. Science 2007, 316, 723–726. [Google Scholar] [CrossRef] [PubMed]
- Osnaghi, S.; Bertet, P.; Auffeves, A.; Maioli, P.; Brune, M.; Raimond, J.-M.; Haroche, S. Coherent control of an atomic collision in a cavity. Phys. Rev. Lett. 2001, 87, 037902. [Google Scholar] [CrossRef]
- Gywat, O.; Meier, F.; Loss, D.; Awschalom, D.D. Dynamics of coupled qubits interacting with an off-resonant cavity. Phys. Rev. B 2006, 73, 125336. [Google Scholar] [CrossRef]
- Guerlin, C.; Brion, E.; Esslinger, T.; Mølmer, K. Cavity quantum electrodynamics with a Rydberg-blocked atomic ensemble. Phys. Rev. A 2010, 82, 053832. [Google Scholar] [CrossRef]
- Donaire, M.; Muñoz-Castañeda, J.M.; Nieto, L. Dipole-dipole interaction in cavity QED: The weak-coupling, nondegenerate regime. Phys. Rev. A 2017, 96, 042714. [Google Scholar] [CrossRef]
- Nguyen, T.L.; Raimond, J.-M.; Sayrin, C.; Cortinas, R.; Cantat-Moltrecht, T.; Assemat, F.; Dotsenko, I.; Gleyzes, S.; Haroche, S.; Roux, G.; et al. Towards quantum simulation with circular Rydberg atoms. Phys. Rev. X 2018, 8, 011032. [Google Scholar] [CrossRef]
- Sorensen, A.; Molmer, K. Spin-Spin Interaction and Spin Squeezing in an Optical Lattice. Phys. Rev. Lett. 1999, 83, 2274. [Google Scholar] [CrossRef]
- Porras, D.; Cirac, J.I. Effective Quantum Spin Systems with Trapped Ions. Phys. Rev. Lett. 2004, 92, 207901. [Google Scholar] [CrossRef]
- Cusati, T.; Napoli, A.; Messina, A. Competition between inter- and intra-molecular energy exchanges in a simple quantum model of a dimer. J. Mol. Struct. Theochem. 2006, 769, 3. [Google Scholar] [CrossRef]
- Napoli, A.; Messina, A.; Cusati, T.; Draganescu, G. Quantum signatures in the dynamics of two dipole-dipole interacting soft dimers. Eur. Phys. J. B 2006, 50, 419. [Google Scholar] [CrossRef]
- Hartmann, M.J.; Brandão, G.S.L.; Plenio, M.B. Effective Spin Systems in Coupled Microcavities. Phys. Rev. Lett. 2007, 99, 160501. [Google Scholar] [CrossRef] [PubMed]
- Khlebnikov, S.; Sadiek, G. Decoherence by a nonlinear environment: Canonical versus microcanonical case. Phys. Rev. A 2002, 66, 032312. [Google Scholar] [CrossRef]
- Huang, Z.; Sadiek, G.; Kais, S. Time evolution of a single spin inhomogeneously coupled to an interacting spin environment. J. Chem. Phys. 2006, 124, 144513. [Google Scholar] [CrossRef] [PubMed]
- Abliz, A.; Gao, H.J.; Xie, X.C.; Wu, Y.S.; Liu, W.M. Entanglement control in an anisotropic two-qubit Heisenberg XYZ model with external magnetic fields. Phys. Rev. A 2006, 74, 052105. [Google Scholar] [CrossRef]
- Tsomokos, D.I.; Hartmann, M.J.; Huelga, S.F.; Plenio, M.B. Entanglement dynamics in chains of qubits with noise and disorder. New J. Phys. 2007, 9, 79. [Google Scholar] [CrossRef]
- Burić, N. Influence of the thermal environment on entanglement dynamics in small rings of qubits. Phys. Rev. A 2008, 77, 012321. [Google Scholar] [CrossRef]
- Dubi, Y.; Di Ventra, M. Relaxation times in an open interacting two-qubit system. Phys. Rev. A 2009, 79, 012328. [Google Scholar] [CrossRef]
- Sadiek, G.; Alkurtass, B.; Aldossary, O. Entanglement in a time-dependent coupled XY spin chain in an external magnetic field. Phys. Rev. A 2010, 82, 052337. [Google Scholar] [CrossRef]
- Xu, Q.; Sadiek, G.; Kais, S. Dynamics of entanglement in a two-dimensional spin system. Phys. Rev. A 2011, 83, 062312. [Google Scholar] [CrossRef]
- Sadiek, G.; Kais, S. Persistence of entanglement in thermal states of spin systems. J. Phys. B 2013, 46, 245501. [Google Scholar] [CrossRef]
- Duan, L.; Wang, H.; Chen, Q.-H.; Zhao, Y. Entanglement dynamics of two qubits coupled individually to Ohmic baths. J. Chem. Phys. 2013, 139, 044115. [Google Scholar] [CrossRef] [PubMed]
- Wu, N.; Nanduri, A.; Rabitz, H. Rabi oscillations, decoherence, and disentanglement in a qubit–spin-bath system. Phys. Rev. A 2014, 89, 062105. [Google Scholar] [CrossRef]
- Sadiek, G.; Almalki, S. Entanglement dynamics in Heisenberg spin chains coupled to a dissipative environment at finite temperature. Phys. Rev. A 2016, 94, 012341. [Google Scholar] [CrossRef]
- Yu, T.; Eberly, J.H. Finite-time disentanglement via spontaneous emission. Phys. Rev. Lett. 2004, 93, 140404. [Google Scholar] [CrossRef] [PubMed]
- Yu, T.; Eberly, J.H. Sudden death of entanglement. Science 2009, 323, 598–601. [Google Scholar] [CrossRef]
- Yu, T.; Eberly, J.H. Sudden death of entanglement: Classical noise effects. Opt. Commun. 2006, 264, 393–397. [Google Scholar] [CrossRef]
- Yönaç, M.; Yu, T.; Eberly, J.H. Sudden death of entanglement of two Jaynes–Cummings atoms. J. Phys. B 2006, 39, S621–S625. [Google Scholar] [CrossRef]
- Sainz, I.; Klimov, A.B.; Roa, L. Entanglement dynamics modified by an effective atomic environment. Phys. Rev. A 2006, 73, 032303. [Google Scholar] [CrossRef]
- Yönaç, M.; Yu, T.; Eberly, J.H. Pairwise concurrence dynamics: A four-qubit model. J. Phys. B 2007, 73, S45–S59. [Google Scholar] [CrossRef]
- Sainz, I.; Björk, G. Entanglement invariant for the double Jaynes-Cummings model. Phys. Rev. A 2007, 76, 042313. [Google Scholar] [CrossRef]
- Chan, S.; Reid, M.D.; Ficek, Z. Entanglement evolution of two remote and non-identical Jaynes–Cummings atoms. J. Phys. B 2009, 42, 065507. [Google Scholar] [CrossRef]
- Tavassoly, M.K.; Daneshmand, R.; Rustaee, N. Entanglement dynamics of linear and nonlinear interaction of two two-level atoms with a quantized Phase-damped field in the dispersive regime. Int. J. Theor. Phys. 2018, 57, 1645–1658. [Google Scholar] [CrossRef]
- Bai, X.-M.; Gao, C.-P.; Li, J.-Q.; Liu, N.; Liang, J.-Q. Entanglement dynamics for two spins in an optical cavity–field interaction induced decoherence and coherence revival. Opt. Express 2017, 25, 17051–17065. [Google Scholar] [CrossRef]
- Liu, R.-F.; Chen, C.-C. Role of the Bell singlet state in the suppression of disentanglement. Phys. Rev. A 2006, 74, 024102. [Google Scholar] [CrossRef]
- Ficek, Z.; Tanaś, R. Dark periods and revivals of entanglement in a two-qubit system. Phys. Rev. A 2006, 74, 024304. [Google Scholar] [CrossRef]
- Deng, T.; Yan, Y.; Chen, L.; Zhao, Y. Dynamics of the two-spin spin-boson model with a common bath. J. Chem. Phys. 2016, 144, 144102. [Google Scholar] [CrossRef]
- Li, C.; Xiao-Qiang, S.; Shou, Z. The influences of dipole–dipole interaction and detuning on the sudden death of entanglement between two atoms in the Tavis–Cummings model. Chin. Phys. B 2009, 18, 888–893. [Google Scholar] [CrossRef]
- Zhang, G.-F.; Chen, Z.-Y. The entanglement character between atoms in the non-degenerate two photons Tavis–Cummings model. Opt. Commun. 2007, 275, 274–277. [Google Scholar] [CrossRef][Green Version]
- Torres, J.M.; Sadurní, E.; Seligman, T.H. Two interacting atoms in a cavity: Exact solutions, entanglement and decoherence. J. Phys. A 2010, 43, 192002. [Google Scholar] [CrossRef]
- de los Santos-Sánchez, O.; González-Gutiérrez, C.; Récamier, J. Nonlinear Jaynes–Cummings model for two interacting two-level atoms. J. Phys. B 2016, 49, 165503. [Google Scholar] [CrossRef]
- Chathavalappil, N.; Satyanarayana, S.V.M. Schemes to avoid entanglement sudden death of decohering two qubit system. Eur. Phys. J. D 2019, 73, 36. [Google Scholar] [CrossRef]
- Zhang, H.; Fan, C.; Wu, J. In-phase and anti-phase entanglement dynamics of Rydberg atomic pairs. Opt. Exp. 2020, 28, 35350–35362. [Google Scholar] [CrossRef] [PubMed]
- Sadiek, J.; Al-Dress, W.; Abdallah, M.S. Manipulating entanglement sudden death in two coupled two-level atoms interacting off-resonance with a radiation field: An exact treatment. Opt. Exp. 2019, 27, 33799–33825. [Google Scholar] [CrossRef] [PubMed]
- Wootters, W.K. Entanglement of formation of an arbitrary state of two qubits. Phys. Rev. Lett. 1998, 80, 2245–2248. [Google Scholar] [CrossRef]
- Rungta, P.; Buzek, V.; Caves, C.M.; Hillery, H.; Milburn, G.J. Universal state inversion and concurrence in arbitrary dimensions. Phys. Rev. A 2001, 64, 042315. [Google Scholar] [CrossRef]
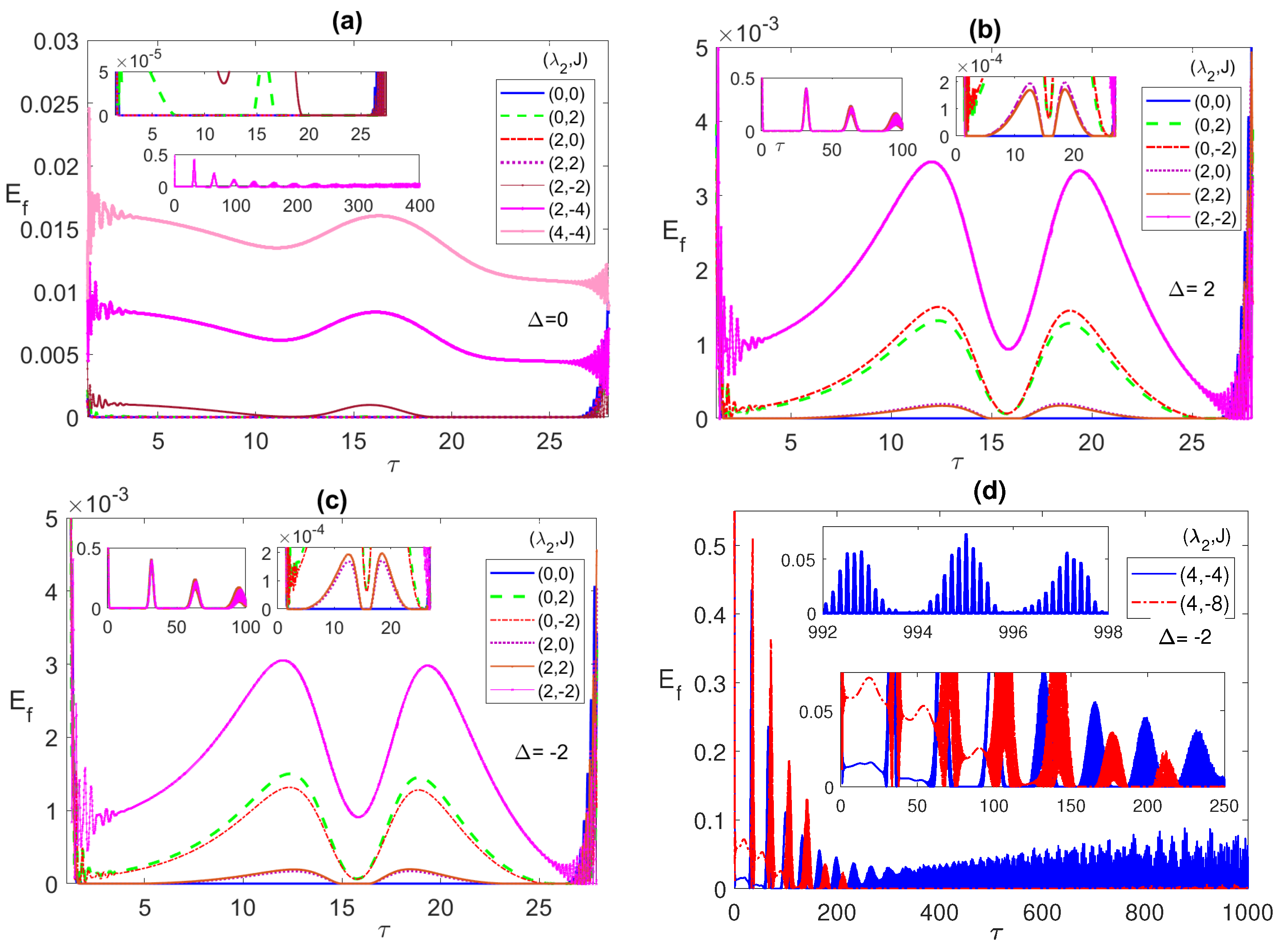


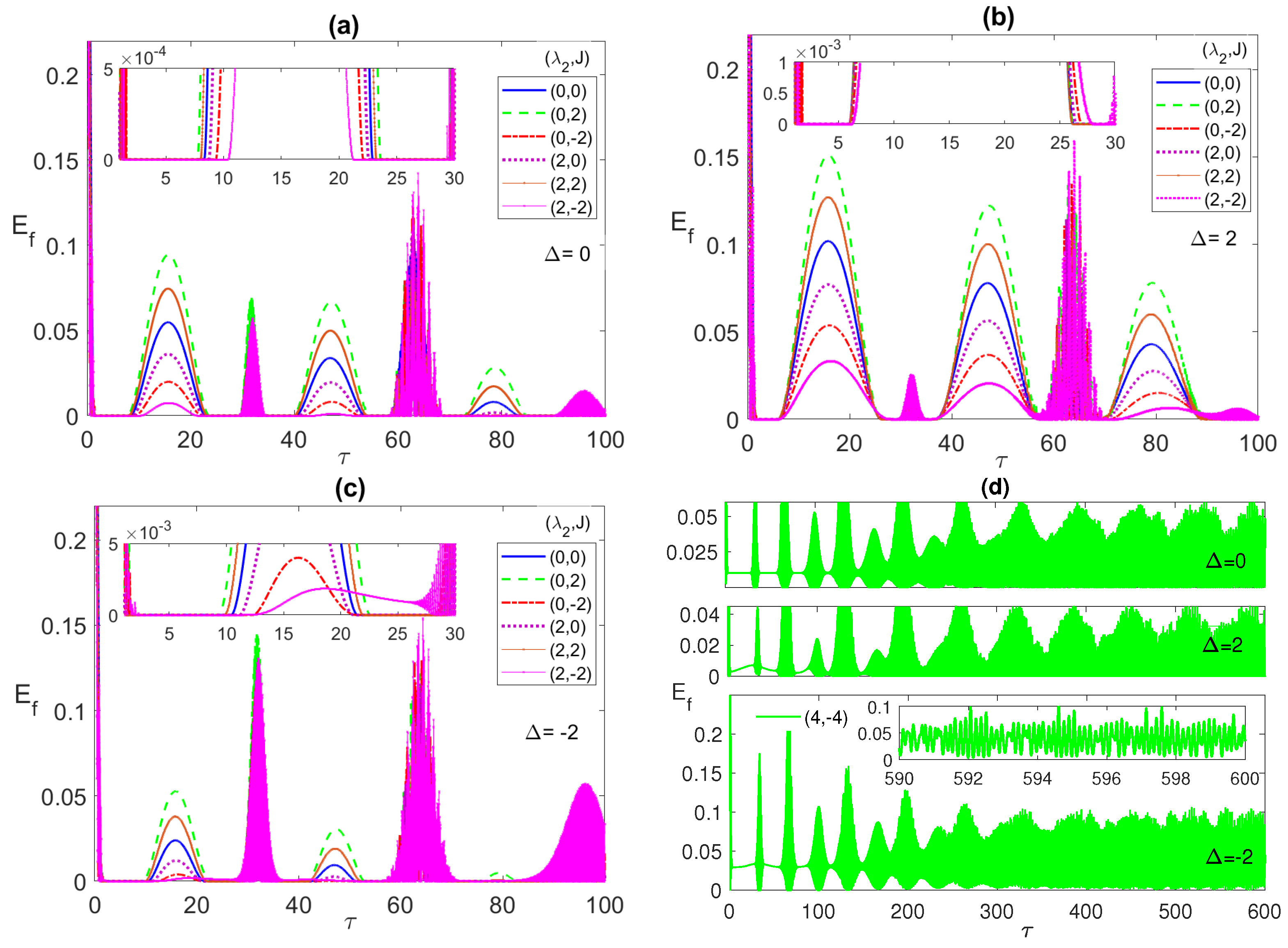


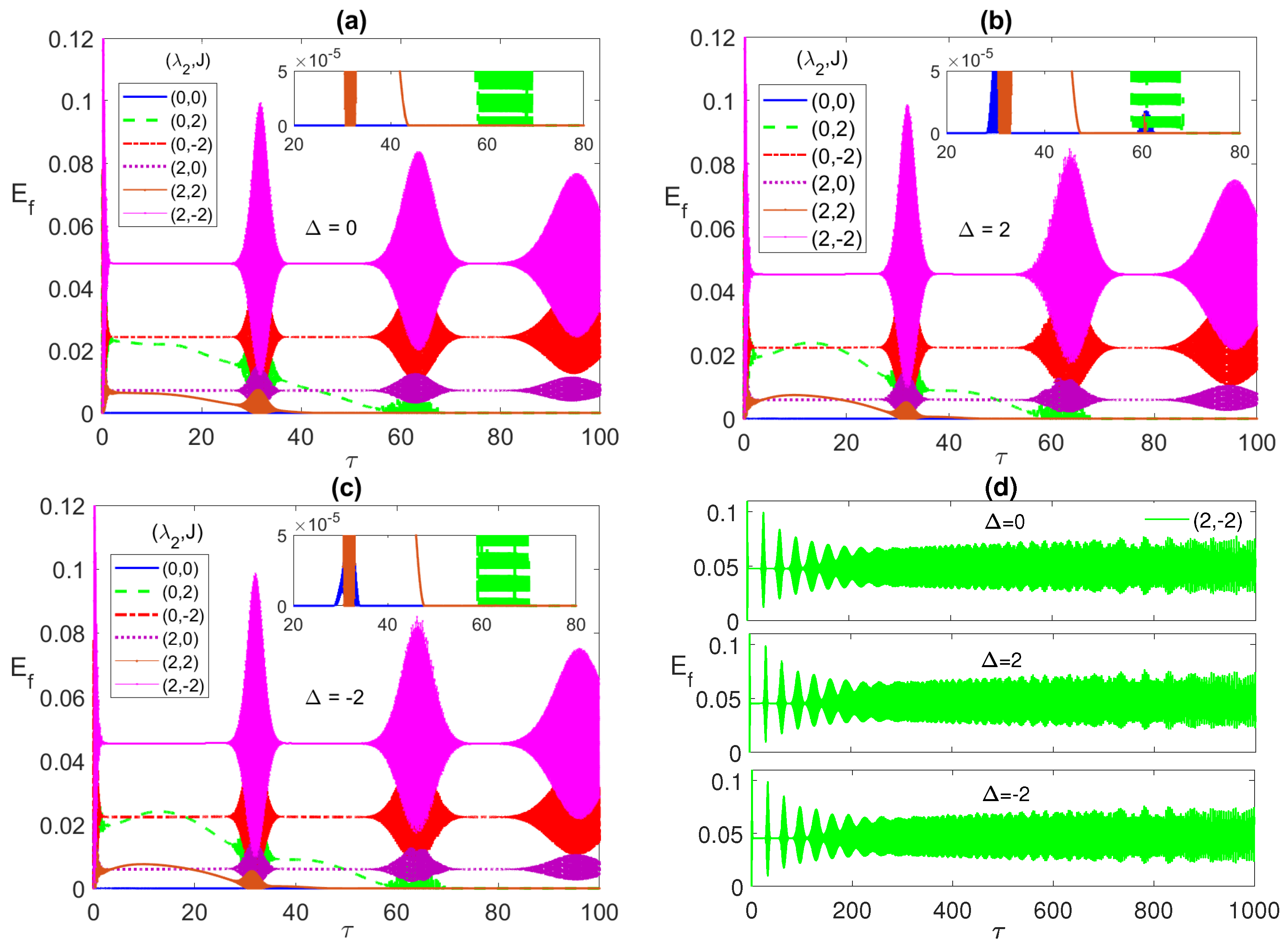


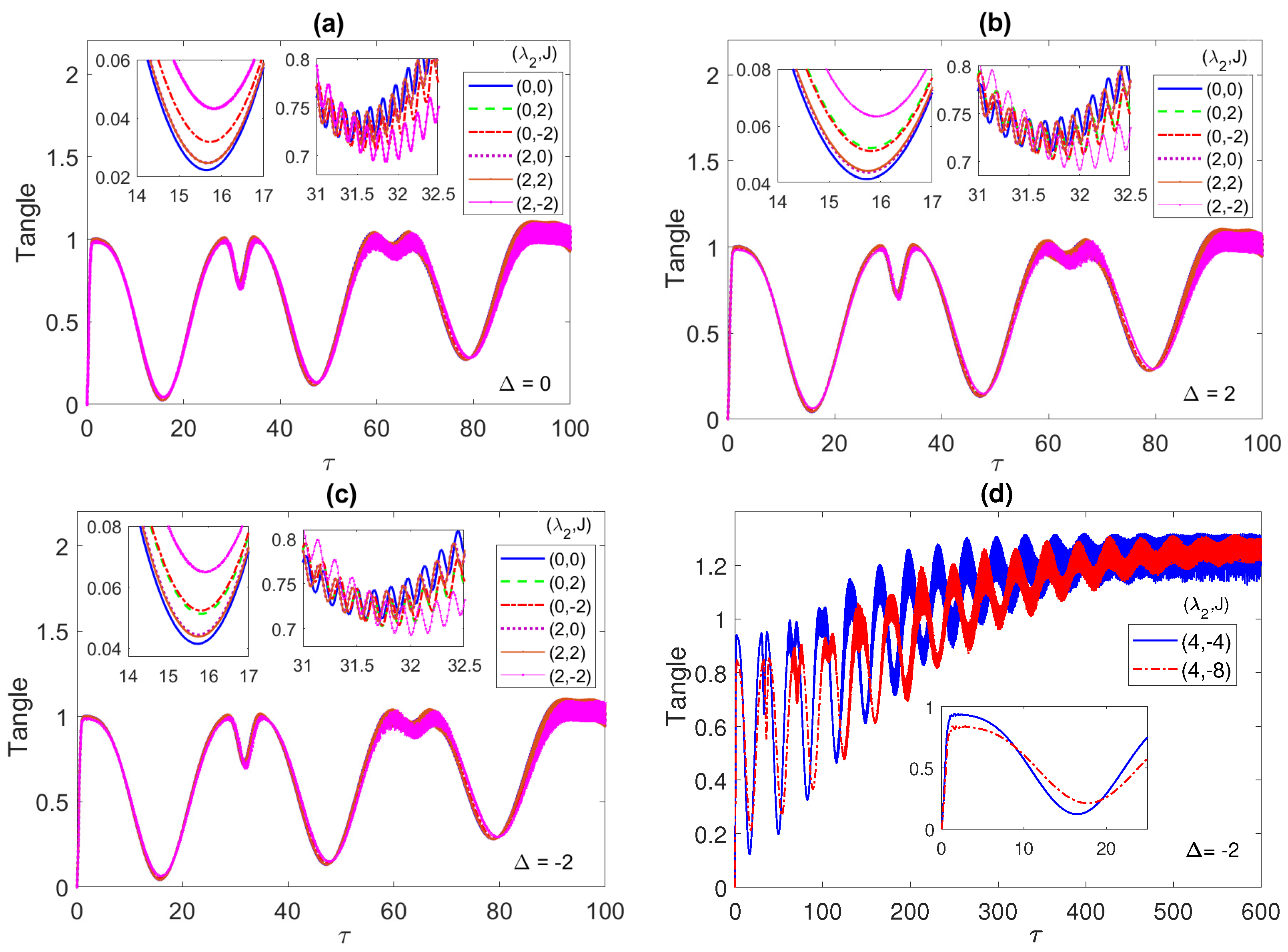
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sadiek, G.; Al-Dress, W.; Shaglel, S.; Elhag, H. Asymptotic Entanglement Sudden Death in Two Atoms with Dipole–Dipole and Ising Interactions Coupled to a Radiation Field at Non-Zero Detuning. Entropy 2021, 23, 629. https://doi.org/10.3390/e23050629
Sadiek G, Al-Dress W, Shaglel S, Elhag H. Asymptotic Entanglement Sudden Death in Two Atoms with Dipole–Dipole and Ising Interactions Coupled to a Radiation Field at Non-Zero Detuning. Entropy. 2021; 23(5):629. https://doi.org/10.3390/e23050629
Chicago/Turabian StyleSadiek, Gehad, Wiam Al-Dress, Salwa Shaglel, and Hala Elhag. 2021. "Asymptotic Entanglement Sudden Death in Two Atoms with Dipole–Dipole and Ising Interactions Coupled to a Radiation Field at Non-Zero Detuning" Entropy 23, no. 5: 629. https://doi.org/10.3390/e23050629
APA StyleSadiek, G., Al-Dress, W., Shaglel, S., & Elhag, H. (2021). Asymptotic Entanglement Sudden Death in Two Atoms with Dipole–Dipole and Ising Interactions Coupled to a Radiation Field at Non-Zero Detuning. Entropy, 23(5), 629. https://doi.org/10.3390/e23050629





