Experimental Investigation and Theoretical Modelling of a High-Pressure Pneumatic Catapult Considering Dynamic Leakage and Convection
Abstract
:1. Introduction
2. Experimental Study on Ejection of a High-Pressure Pneumatic Catapult
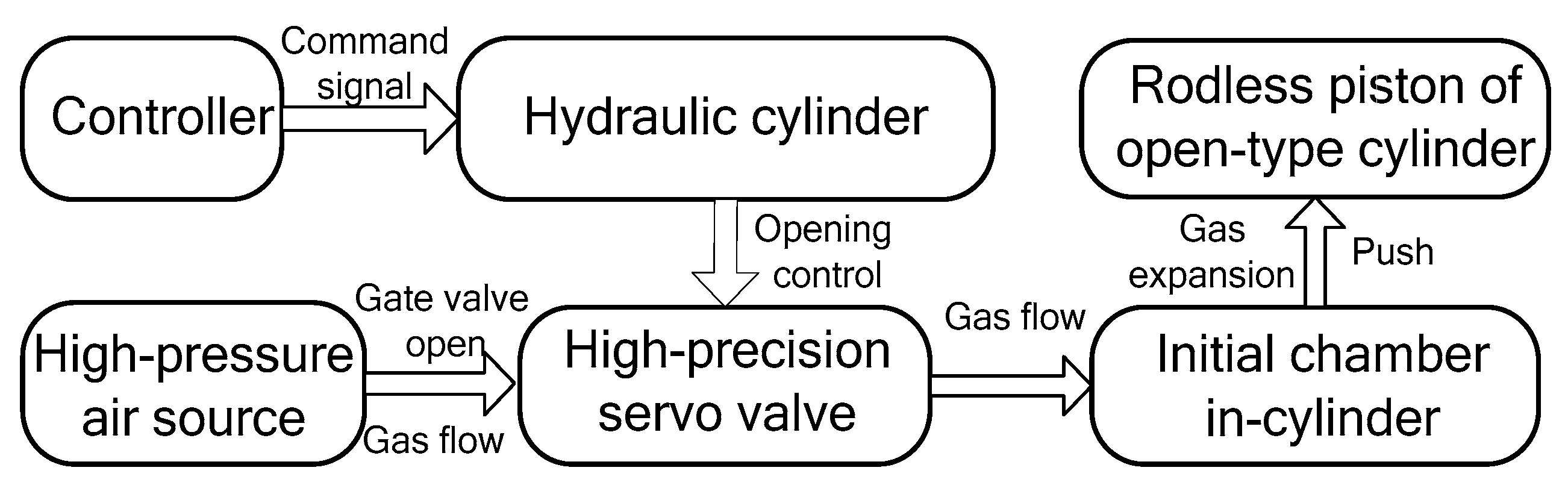
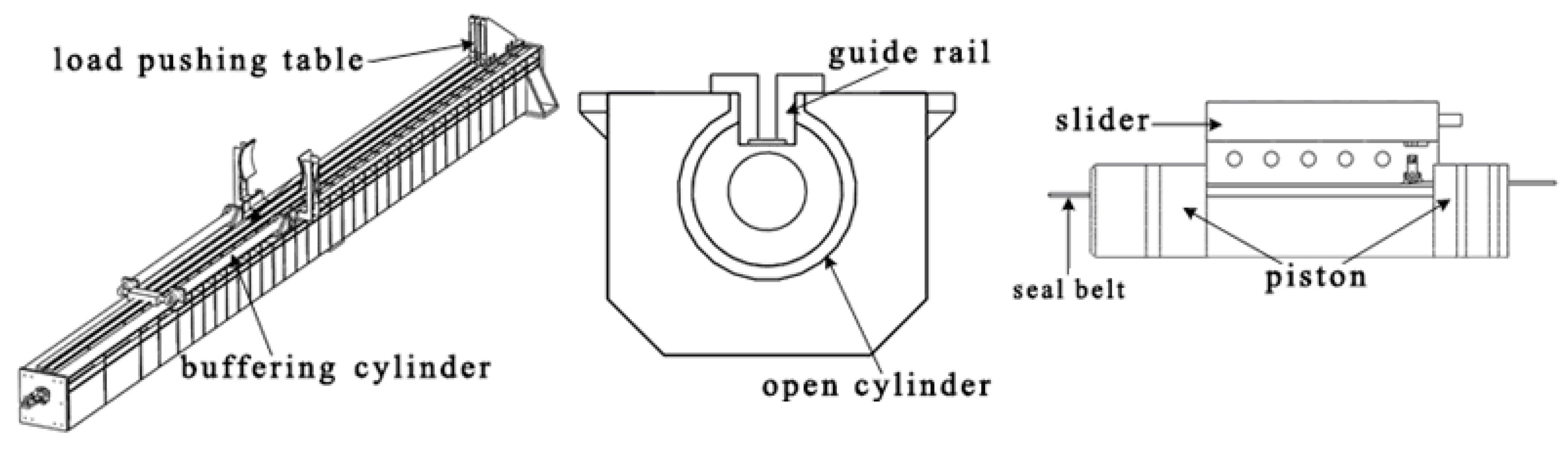
2.1. The Working Mechanism of a High-Pressure Pneumatic Catapult
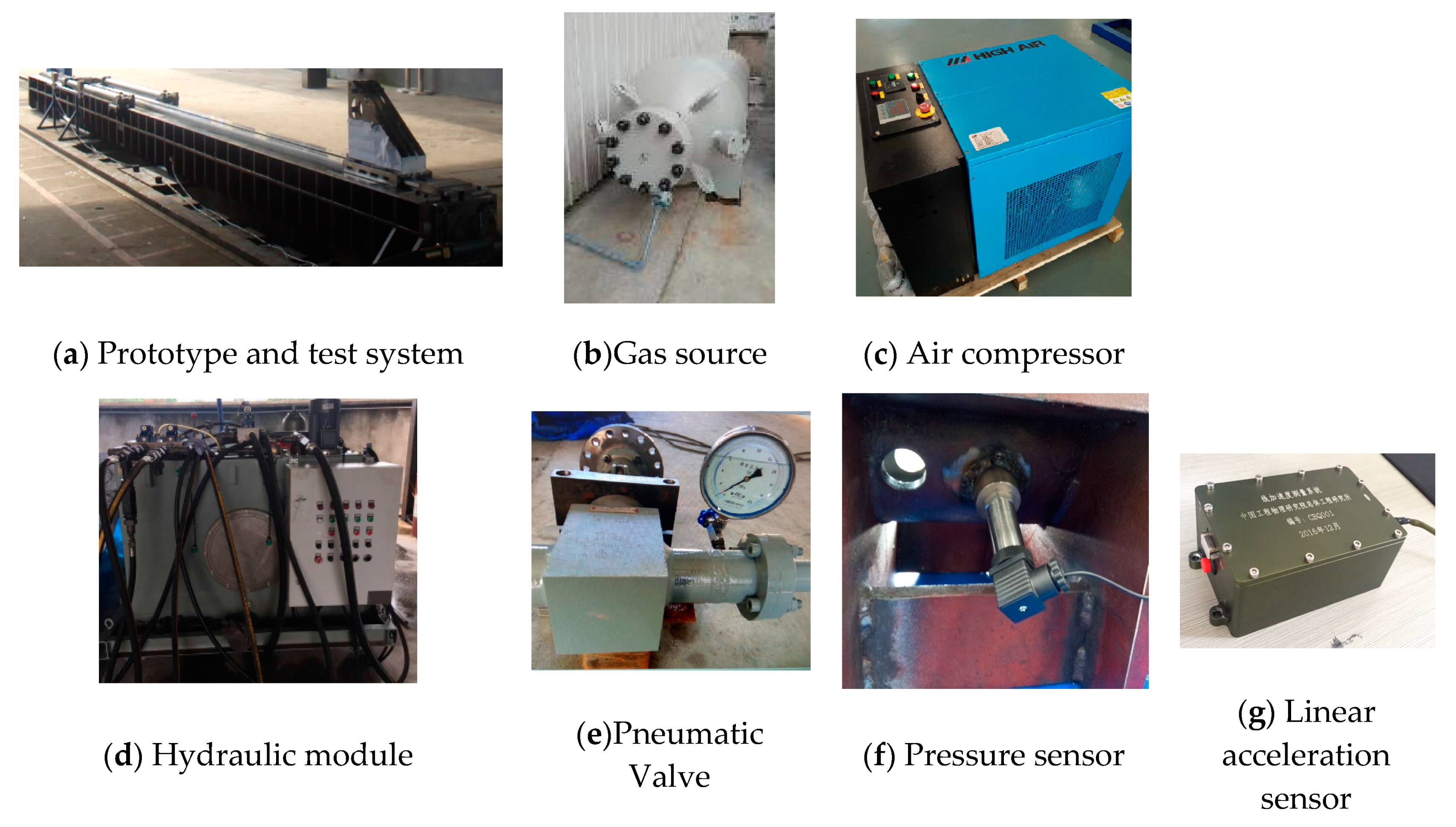
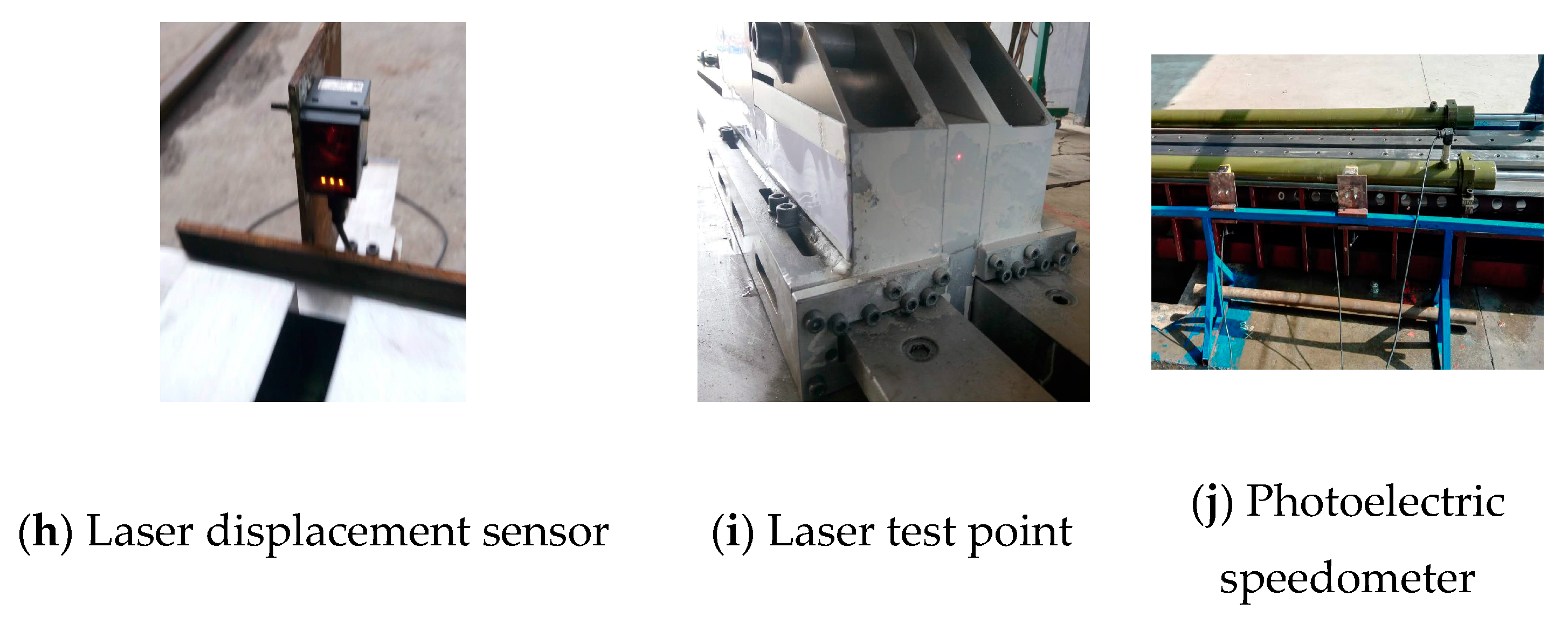
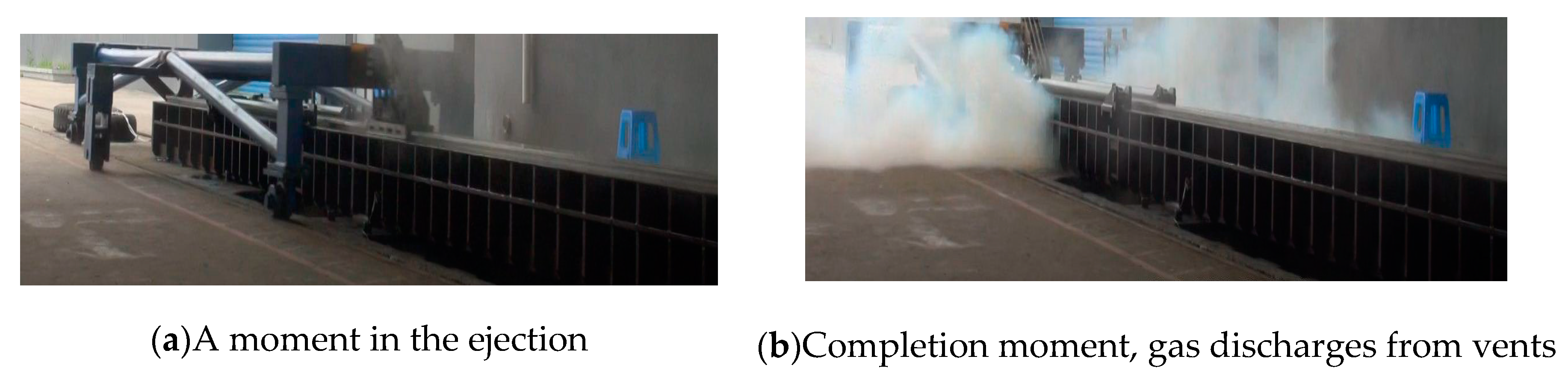
2.2. The Ejection Test System of a High-Pressure Pneumatic Catapult
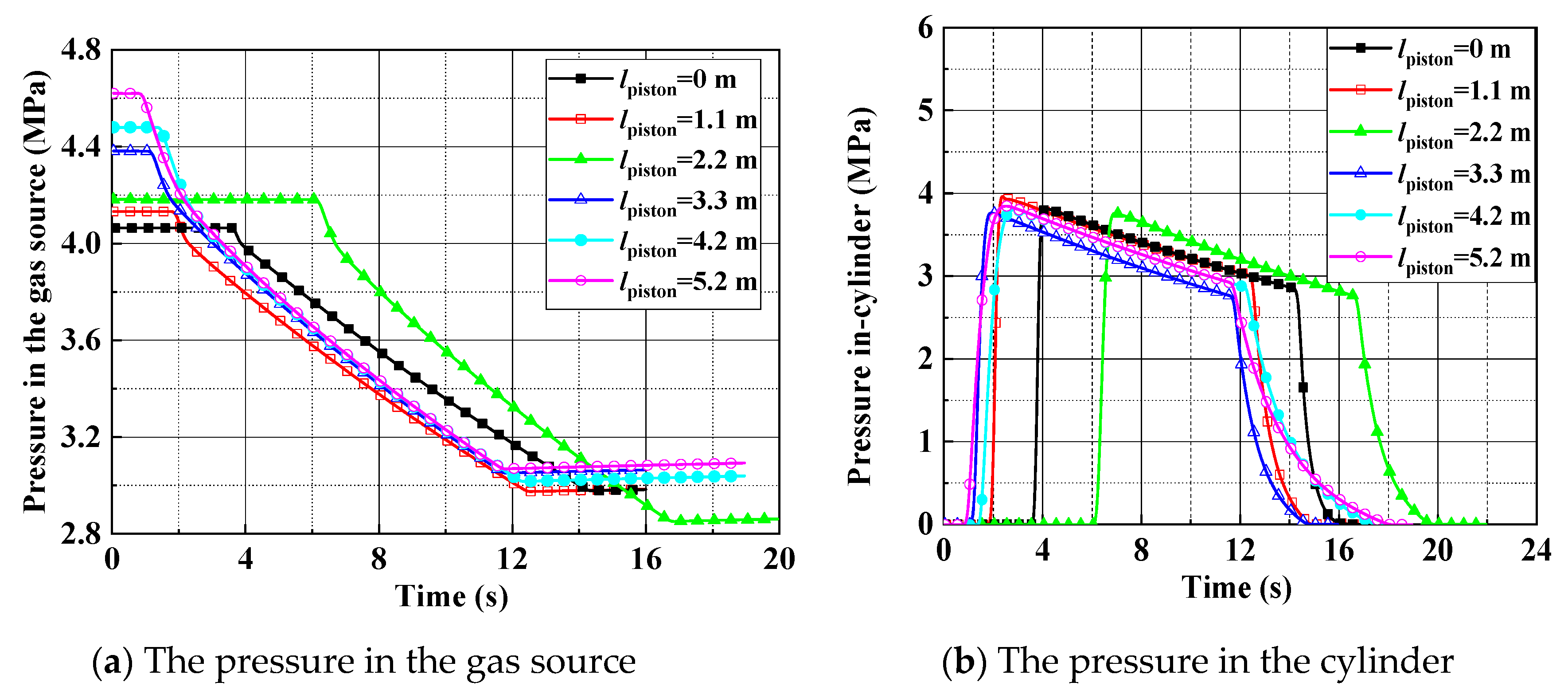
2.3. Comparison of the Ejection Testing and the Theoretical Model in the Ideal Gas State
3. Experimental Investigation Leakage of the High-Pressure Pneumatic Catapult
3.1. Schematic Diagram of the Test System on Leakage Rate
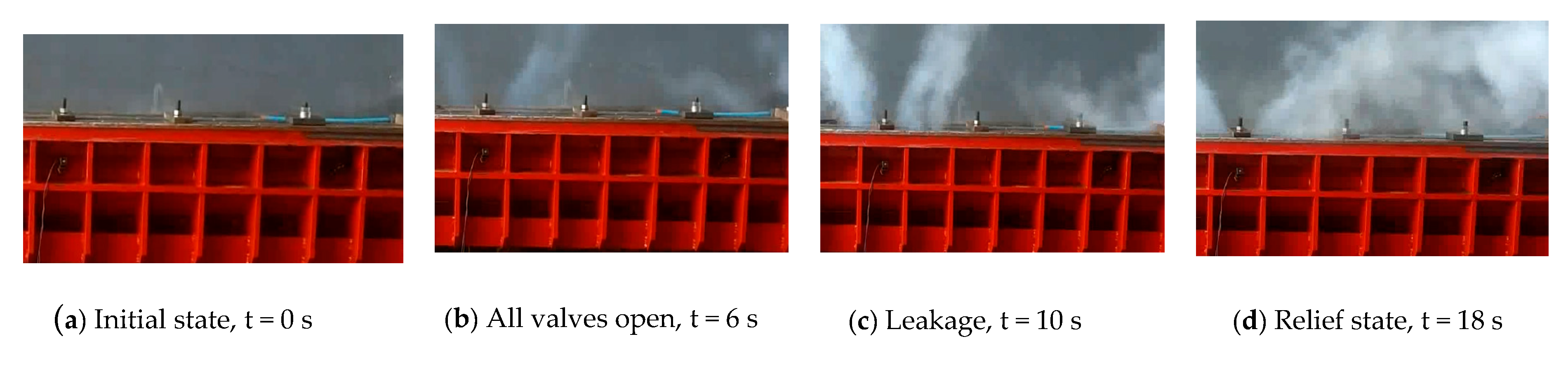
3.2. The Principle of Leakage Rate Test and Test Procedure
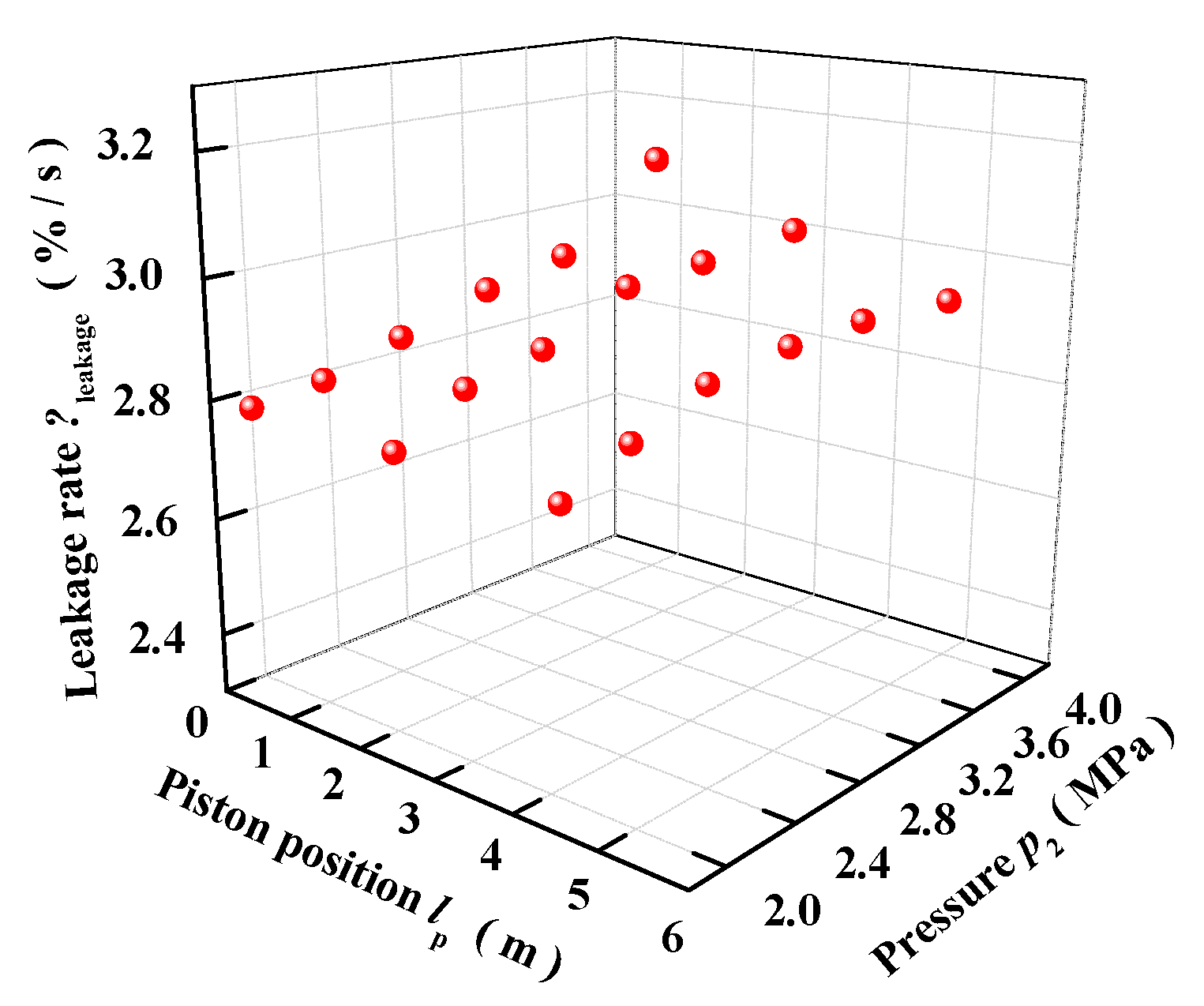
3.3. Leakage Tests and Fitting on Dynamic Leakage Rate Model
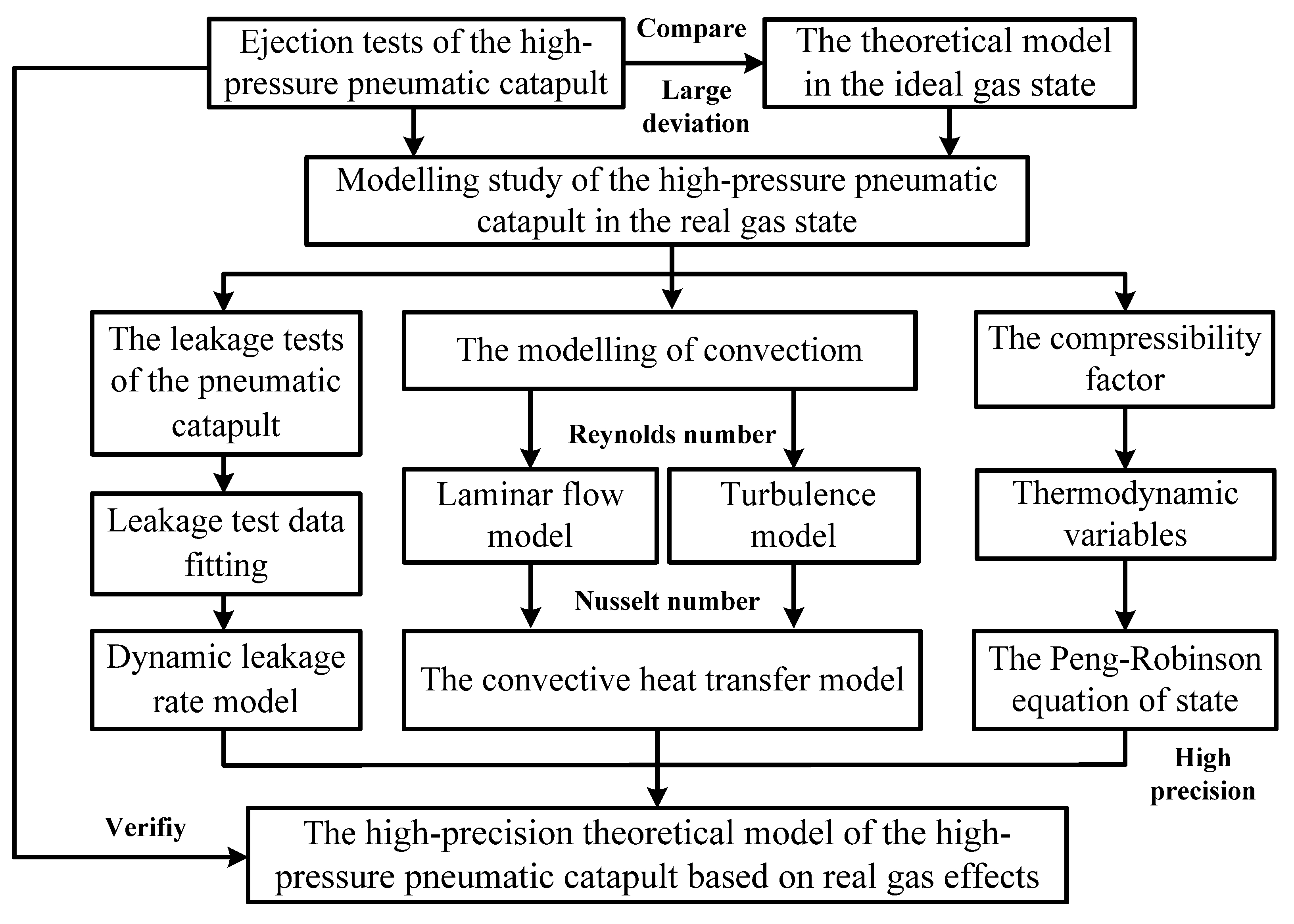
4. Modelling of the High-Pressure Pneumatic Catapult Based on Real Gas Consideration
4.1. The Theoretical Modelling Flow of the High-Pressure Pneumatic Catapult
4.2. The Convective Heat Transfer between the Working Medium and the Metal Wall
4.3. Compressibility Factor and Thermodynamic Variables of a Real Gas
4.3.1. Compressibility Factor of a Real Gas
4.3.2. Thermodynamic Variables
4.4. The Accurate Theoretical Model Considering Dynamic Leakage and Convective Heat Transfer
5. Verification of the Accuracy of the Theoretical Model with the Ejection Test
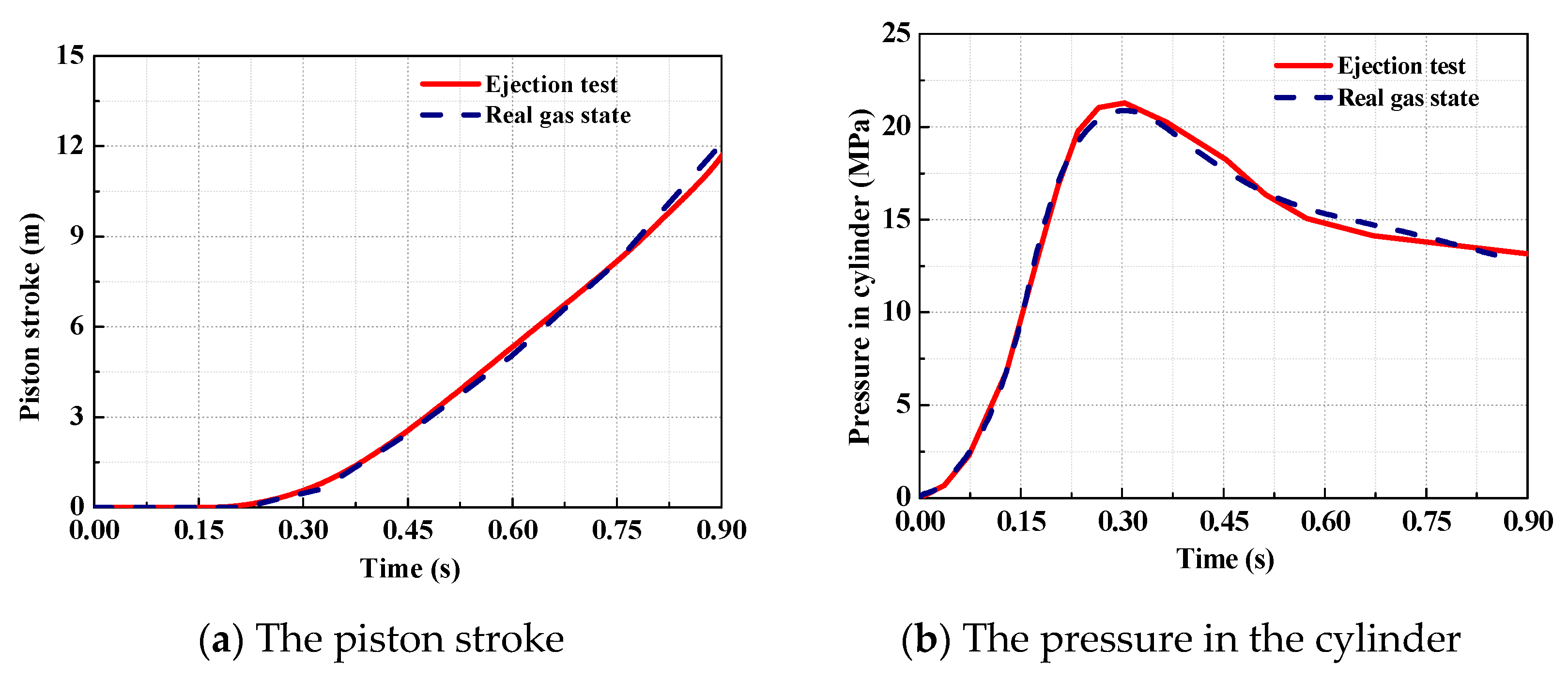
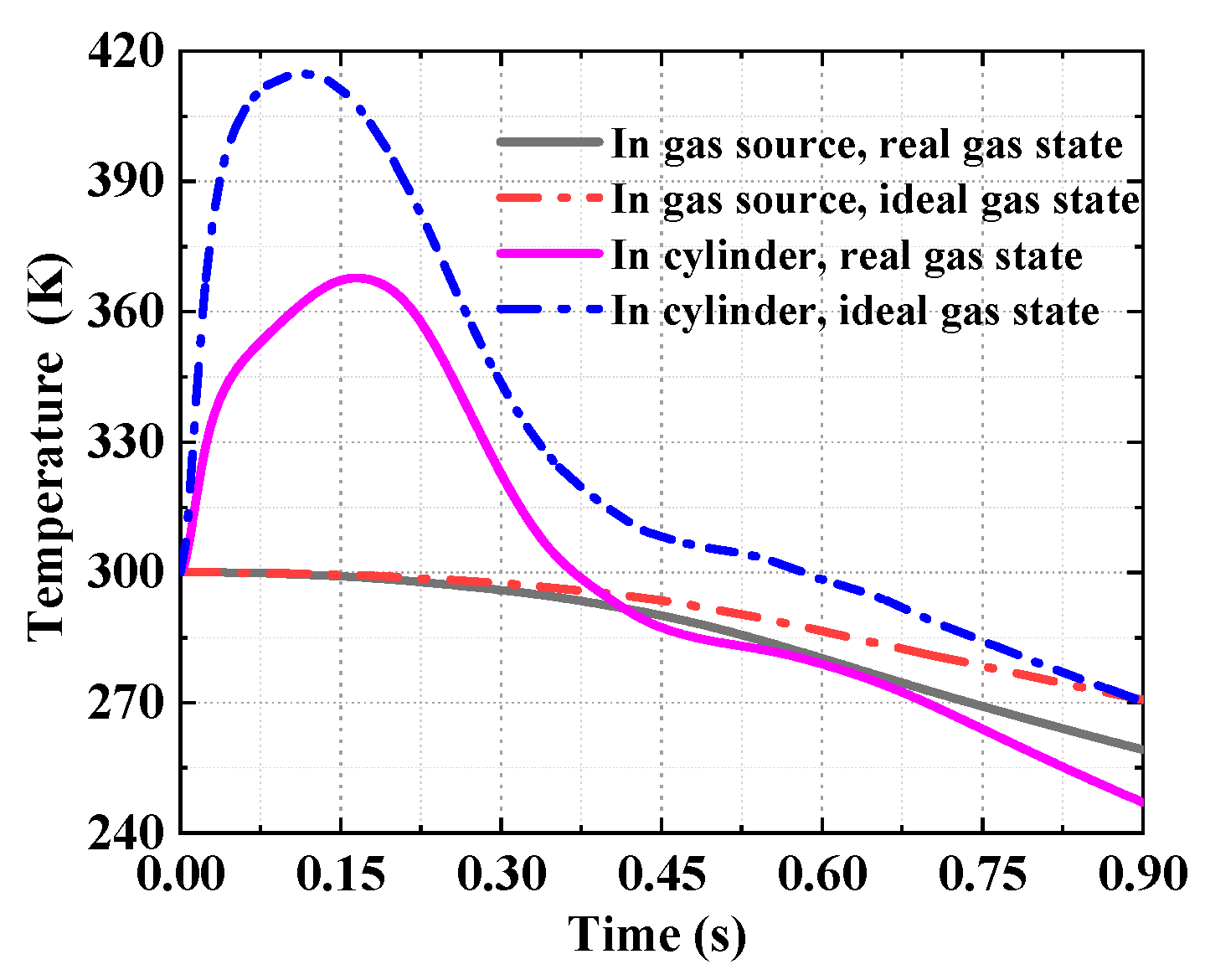
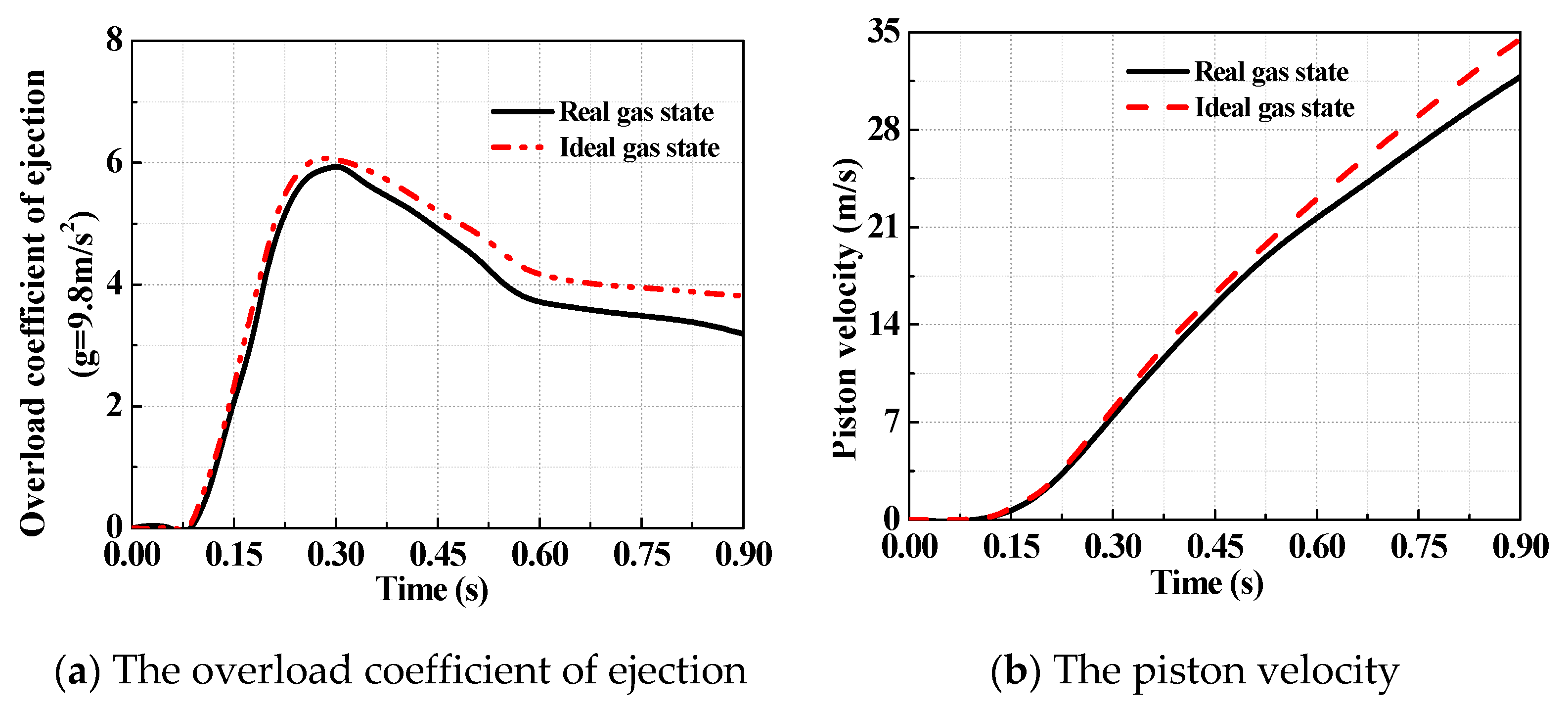
5.1. Verification of the Accuracy of the Theoretical Model Based on Real Gas Effects
5.2. Comparison of Theoretical Models of High-Pressure Pneumatic Catapult
6. Conclusions
- (1)
- It is found that the analytical results based on the ideal gas model give an overestimated performance of the catapult, in comparison to the test data. The maximum deviation of the piston stroke and the cylinder gas pressure is 16.7% and 24.5%. Consequently, the precision of the ideal gas model is unacceptable for the engineering applications.
- (2)
- The relationship of the leakage rate, pressure and stroke is fitted. It is found that the maximum leakage rate of the pneumatic catapult does not exceed 5% within the whole piston stroke, showing a good sealing performance. The leakage rate model is a key factor that affects the accuracy of the theoretical model. Taking leakage into account can improve the accuracy of the theoretical model and make the theoretical calculation more consistent with the actual situation. Regardless of the leakage, the theoretical model is not a true high precision model, and cannot be used to evaluate actual pneumatic catapults, and its practicality will be limited.
- (3)
- A corresponding convective heat transfer model between the working medium and the metal wall has been developed. In the heat transfer process, the choice of laminar flow model or turbulent heat transfer model is based on the Reynolds number.
- (4)
- Based on the Peng–Robinson equation, a theoretical model of the high-pressure pneumatic catapult has been developed, in which the effects of dynamic leakage and the forced convective heat transfer between the gas and the metal wall are taken into account. The results from the theoretical model are consistent with the data form ejection tests, with a maximum deviation of 4%, indicating much higher precision than the ideal gas model.
- (5)
- The theoretical model established can be applicable for dry gases, such as an air, N2 and CO2. It cannot be applied to catapults that use water vapor as it involves phase change of working medium in ejection. The equation of state is not applicable to the two-phase regions.
Author Contributions
Funding
Conflicts of Interest
References
- Cummins, J.J.; Nash, C.; Thomas, S.; Justice, A.; Mahadevan, S.; Adams, D.E.; Barth, E.J. Energy conservation in industrial pneumatics: A state model for predicting energetic savings using a novel pneumatic strain energy accumulator. Appl. Energy 2017, 198, 239–249. [Google Scholar] [CrossRef]
- Wasbari, F.; Bakar, R.; Gan, L.; Tahir, M.; Yusof, A.A. A review of compressed-air hybrid technology in vehicle system. Renew. Sustain. Energy Rev. 2017, 67, 935–953. [Google Scholar] [CrossRef] [Green Version]
- Gao, L.; Hu, J.; Du, J.; Li, B. Dynamic response characteristics of vacuum pressure measurement system with pneumatic long-thin tube. Vacuum 2020, 171, 108995. [Google Scholar] [CrossRef]
- Raisch, A.; Sawodny, O. Analysis and optimal sizing of pneumatic drive systems for handling tasks. Mechatronics 2019, 59, 168–177. [Google Scholar] [CrossRef]
- Ren, J.; Yang, F.; Ma, D.; Le, G.-G.; Zhong, J. Pneumatic Performance Study of a High Pressure Ejection Device Based on Real Specific Energy and Specific Enthalpy. Entropy 2014, 16, 4801–4817. [Google Scholar] [CrossRef] [Green Version]
- Fairuz, Z.; Jahn, I. The influence of real gas effects on the performance of supercritical CO2 dry gas seals. Tribol. Int. 2016, 102, 333–347. [Google Scholar] [CrossRef] [Green Version]
- Fang, Y.; Lu, Y.; Yu, X.; Roskilly, A.P. Experimental study of a pneumatic engine with heat supply to improve the overall performance. Appl. Therm. Eng. 2018, 134, 78–85. [Google Scholar] [CrossRef] [Green Version]
- Bian, J.; Cao, X.; Yang, W.; Guo, D.; Xiang, C. Prediction of supersonic condensation process of methane gas considering real gas effects. Appl. Therm. Eng. 2020, 164, 114508. [Google Scholar] [CrossRef]
- He, W.; Luo, X.; Evans, D.; Busby, J.; Garvey, S.; Parkes, D.; Wang, J. Exergy storage of compressed air in cavern and cavern volume estimation of the large-scale compressed air energy storage system. Appl. Energy 2017, 208, 745–757. [Google Scholar] [CrossRef]
- Yao, L.; Ma, D.; Ma, W.; Ren, J.; Zhong, J.; Wang, Z. Interior ballistics modelling and optimization of one-side ejection device with two-step cylinder. Acta Armamentarii 2017, 38, 466–475. [Google Scholar]
- Yang, F.; Ma, D.; Zhu, Z.; Le, G. Pneumatic Catapult Performance Research Based on the True Thermodynamic Process Analysis. Chin. J. Mech. Eng. 2013, 49, 167. [Google Scholar] [CrossRef]
- Bejan, A. Advanced Engineering Thermodynamics; John Wiley & Sons: Hoboken, NJ, USA, 2016. [Google Scholar]
- Somuncu, E.; Mamedov, B.A. An accurate investigation of second virial coefficient with Morse-Morse Spline Van der Waals potential and its utilization in the study of real systems. Fluid Phase Equilibria 2019, 499, 112255. [Google Scholar] [CrossRef]
- Costa, É.D.; Braga, J.P.; Lemes, N.H. Functional sensitivity analysis approach to retrieve the potential energy function from the quantum second virial coefficient. Phys. A Stat. Mech. Appl. 2019, 536, 122539. [Google Scholar] [CrossRef]
- González-Calderón, A. Second virial coefficient of Kihara molecules with variable range. J. Mol. Liq. 2017, 233, 528–536. [Google Scholar] [CrossRef]
- Privat, R.; Privat, Y.; Jaubert, J.-N. Can cubic equations of state be recast in the virial form? Fluid Phase Equilibria 2009, 282, 38–50. [Google Scholar] [CrossRef]
- Jean, N.J.; Romain, P. Relationship between the binary interaction parameters (kij) of the Peng-Robinson and those of the Soave-Redlich-Kwong equations of state: Application to the definition of the PR2SRK model. Fluid Phase Equilibria 2010, 295, 26–37. [Google Scholar]
- Qiao, Z.; Yang, X.; Zhang, Y. Thermodynamic-consistent multiple-relaxation-time lattice Boltzmann equation model for two-phase hydrocarbon fluids with Peng-Robinson equation of state. Int. J. Heat Mass Transf. 2019, 141, 1216–1226. [Google Scholar] [CrossRef]
- Huang, D.; Yang, D. Improved enthalpy prediction of hydrocarbon fractions with a modified alpha function for the Peng-Robinson equation of state. Fuel 2019, 255, 115840. [Google Scholar] [CrossRef]
- Gao, L.; Wu, C.; Zhang, D.; Fu, X.; Li, B. Research on a high-accuracy and high-pressure pneumatic servo valve with aerostatic bearing for precision control systems. Precis. Eng. 2019, 60, 355–367. [Google Scholar] [CrossRef]
- Liu, J.; Dang, J.; Chen, Z.; Yang, M. Impact of Pressure difference on leakage rate from rigid positive standard leak: A theoretical and experimental Study. Chin. J. Vac. Sci. Technol. 2017, 12, 1141–1145. [Google Scholar]
- Mu, L.; Liao, X.; Zhao, X.; Zhang, J.; Zou, J.; Chen, L.; Chu, H. Analytical model of leakage through an incomplete-sealed well. J. Nat. Gas Sci. Eng. 2020, 77, 103256. [Google Scholar] [CrossRef]
- Tu, R.; Xie, Q.; Yi, J.; Li, K.; Zhou, X.; Jiang, X. An experimental study on the leakage process of high pressure CO2 from a pipeline transport system. Greenh. Gases Sci. Technol. 2014, 4, 777–784. [Google Scholar] [CrossRef]
- Chen, Y.; Yu, Y.; Zhou, W.-W.; Peng, D.; Liu, Y. Heat transfer enhancement of turbulent channel flow using tandem self-oscillating inverted flags. Phys. Fluids 2018, 30, 075108. [Google Scholar] [CrossRef]
- Jia, B.-Q.; Xie, L.; Cui, X.; Fu, Q.-F. Linear stability of confined coaxial jets in the presence of gas velocity oscillations with heat and mass transfer. Phys. Fluids 2019, 31, 092101. [Google Scholar] [CrossRef]
- Zafar, F.; Alam, M. A low Reynolds number flow and heat transfer topology of a cylinder in a wake. Phys. Fluids 2018, 30, 083603. [Google Scholar] [CrossRef]
- Lemmon, E.W. Thermodynamic Properties of Air and Mixtures of Nitrogen, Argon, and Oxygen from 60 to 2000 K at Pressures to 2000 MPa. J. Phys. Chem. Ref. Data 2000, 29, 331–385. [Google Scholar] [CrossRef] [Green Version]




| The Leakage Rate ηleakage [%/s] | Piston Position lp [m] | ||||||
|---|---|---|---|---|---|---|---|
| 0.00 | 1.10 | 2.20 | 3.30 | 4.20 | 5.20 | ||
| Maximum pressure in-cylinder p2 [MPa] | 2.00 | 2.77 | 2.85 | 2.95 | 3.05 | 3.12 | 3.27 |
| 2.75 | 2.62 | 2.77 | 2.87 | 3.00 | 3.06 | 3.13 | |
| 3.80 | 2.41 | 2.57 | 2.72 | 2.82 | 2.89 | 2.95 | |
| Compressibility Factors Z | Pressure [MPa] | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 0.101 | 1 | 6 | 12 | 18 | 24 | 30 | 35 | |||
| Temperature [K] | 220 (Tr = 1.6) | P-R equation | 0.9982 | 0.9793 | 0.8960 | 0.8521 | 0.8647 | 0.9322 | 1.0969 | 1.1259 |
| N-O value | 0.9981 | 0.9938 | 0.9068 | 0.8421 | 0.8666 | 0.9425 | 1.0388 | 1.1310 | ||
| 265 (Tr = 2) | P-R equation | 0.9990 | 0.9903 | 0.9550 | 0.9421 | 0.9555 | 0.9903 | 1.0326 | 1.0762 | |
| N-O value | 0.9988 | 0.9928 | 0.9683 | 0.9588 | 0.9761 | 1.0039 | 1.0152 | 1.0276 | ||
| 331 (Tr = 2.5) | P-R equation | 0.9997 | 0.9975 | 0.9920 | 0.9989 | 1.0323 | 1.0564 | 1.0914 | 1.1137 | |
| N-O value | 0.9969 | 0.9975 | 0.9996 | 1.0101 | 1.0429 | 1.0753 | 1.1034 | 1.1328 | ||
| 463.5 (Tr = 3.5) | P-R equation | 1.00027 | 1.0038 | 1.0146 | 1.0336 | 1.0662 | 1.0929 | 1.1086 | 1.1321 | |
| N-O value | 1.0033 | 1.0042 | 1.0181 | 1.0405 | 1.0709 | 1.1067 | 1.1236 | 1.1527 | ||
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ren, J.; Zhong, J.; Yao, L.; Guan, Z. Experimental Investigation and Theoretical Modelling of a High-Pressure Pneumatic Catapult Considering Dynamic Leakage and Convection. Entropy 2020, 22, 1010. https://doi.org/10.3390/e22091010
Ren J, Zhong J, Yao L, Guan Z. Experimental Investigation and Theoretical Modelling of a High-Pressure Pneumatic Catapult Considering Dynamic Leakage and Convection. Entropy. 2020; 22(9):1010. https://doi.org/10.3390/e22091010
Chicago/Turabian StyleRen, Jie, Jianlin Zhong, Lin Yao, and Zhongwei Guan. 2020. "Experimental Investigation and Theoretical Modelling of a High-Pressure Pneumatic Catapult Considering Dynamic Leakage and Convection" Entropy 22, no. 9: 1010. https://doi.org/10.3390/e22091010
APA StyleRen, J., Zhong, J., Yao, L., & Guan, Z. (2020). Experimental Investigation and Theoretical Modelling of a High-Pressure Pneumatic Catapult Considering Dynamic Leakage and Convection. Entropy, 22(9), 1010. https://doi.org/10.3390/e22091010






