Shortcuts to Adiabaticity for Optical Beam Propagation in Nonlinear Gradient Refractive-Index Media
Abstract
1. Introduction
2. Variational Approximation Method
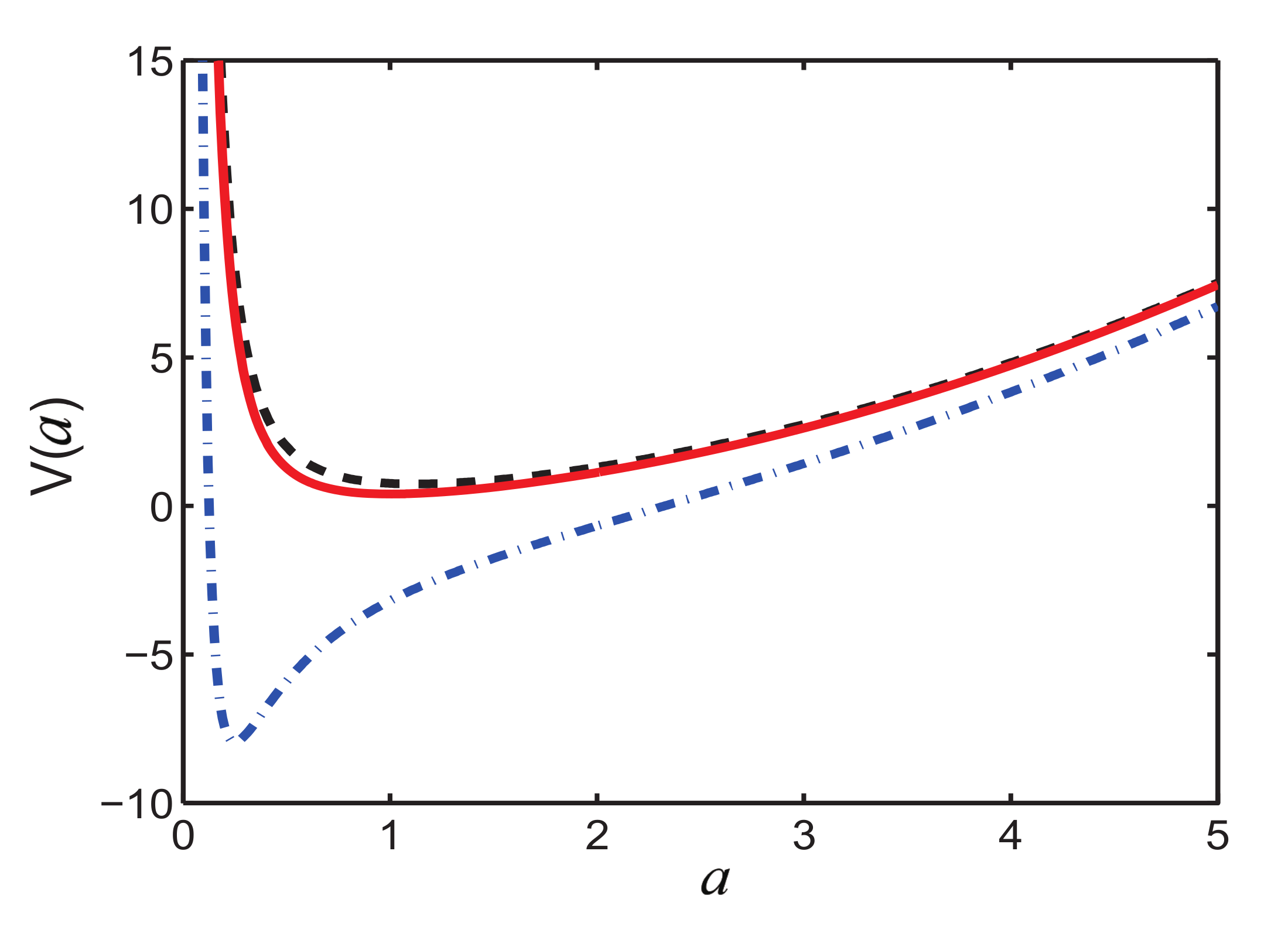
3. Effective Potential and Adiabatic Reference
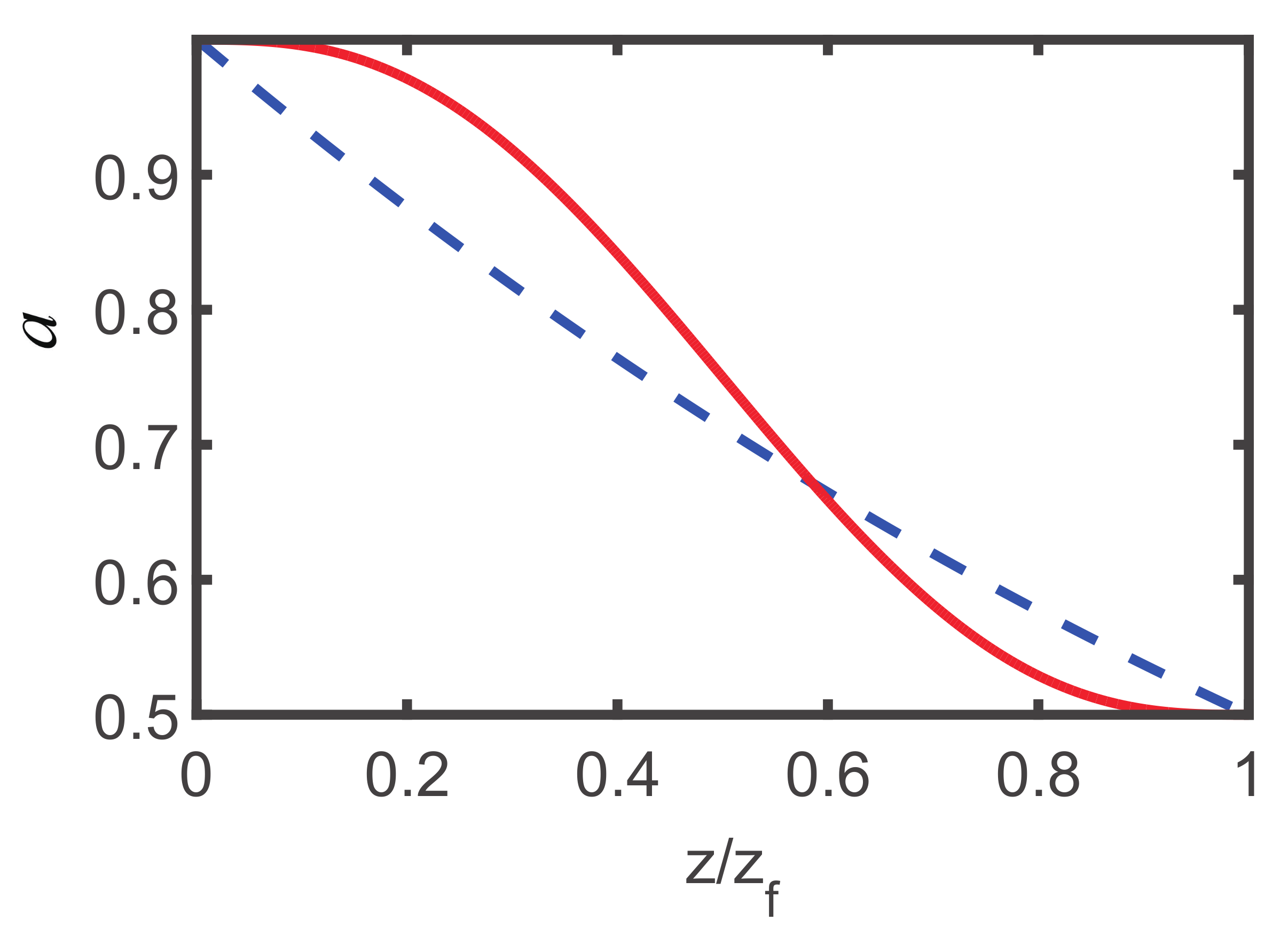
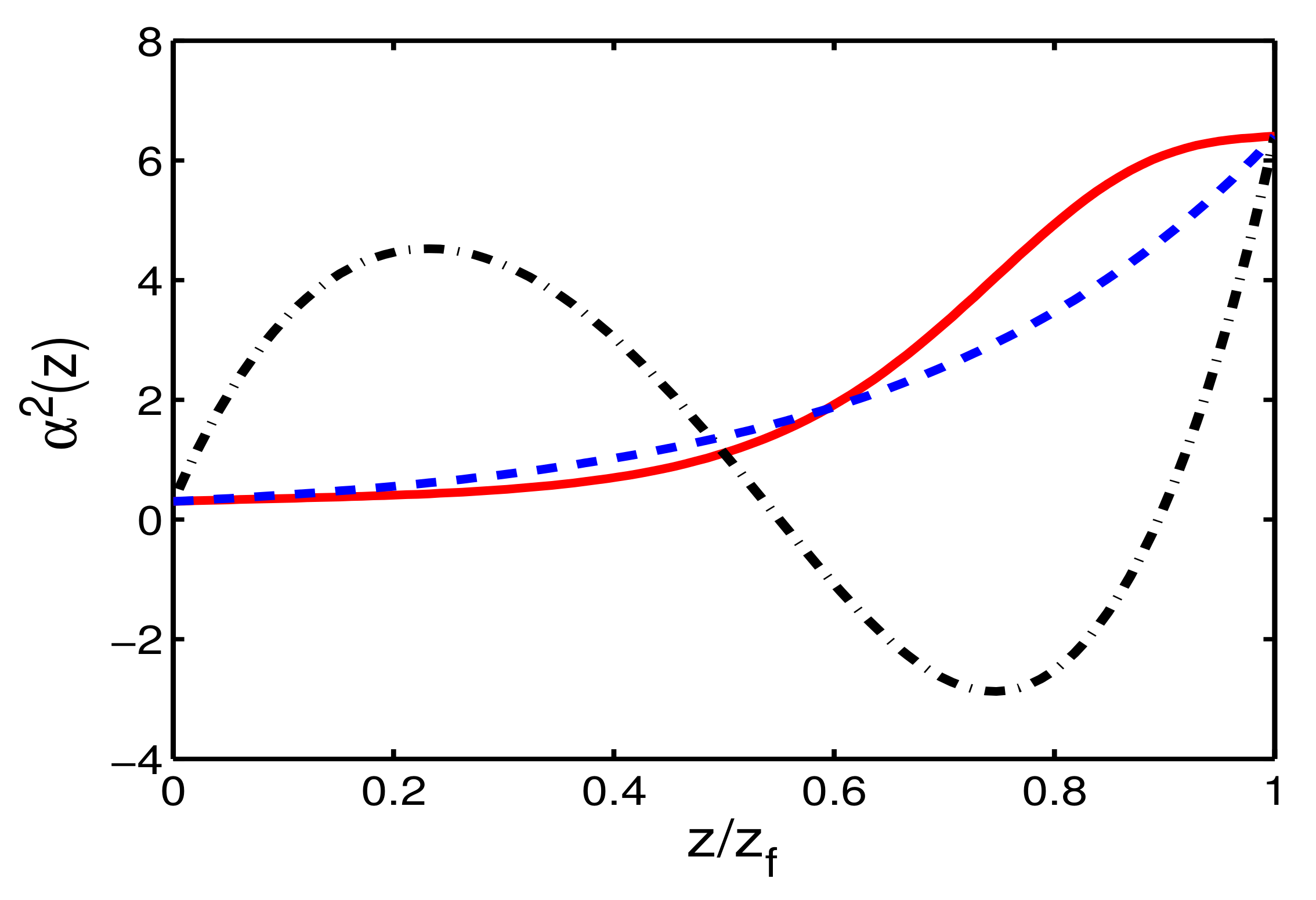
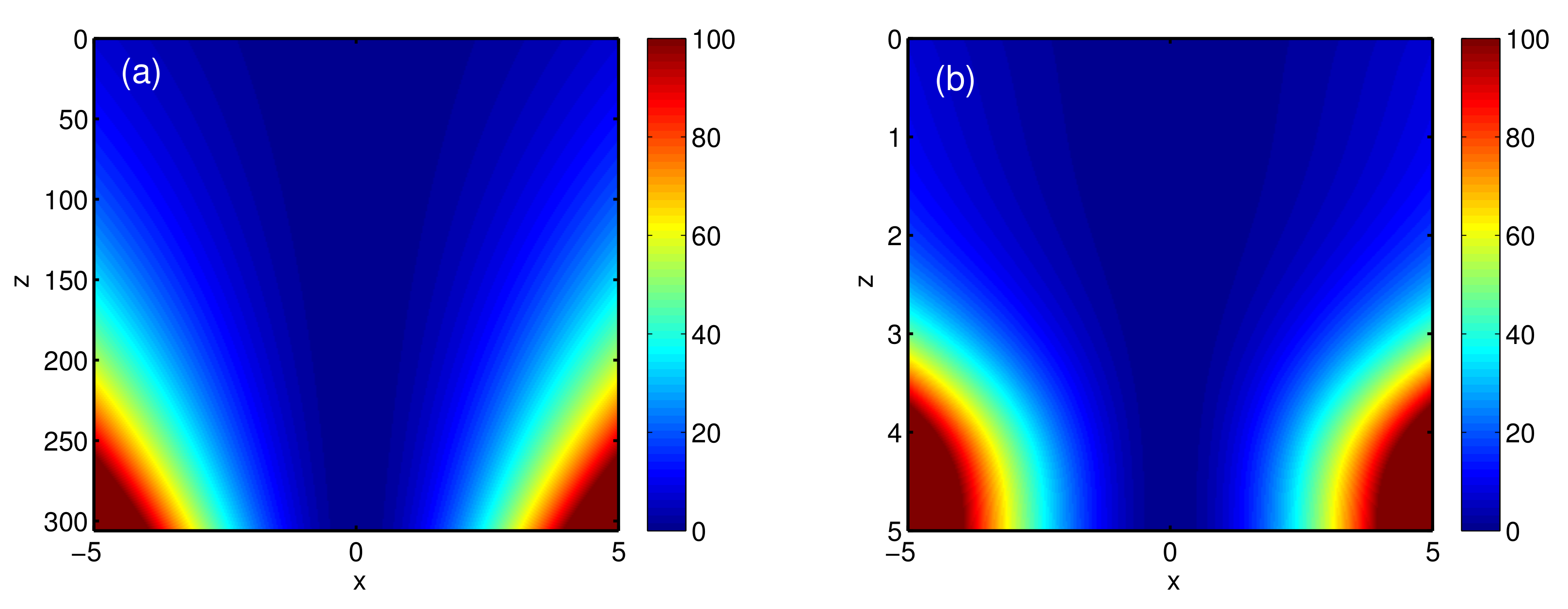
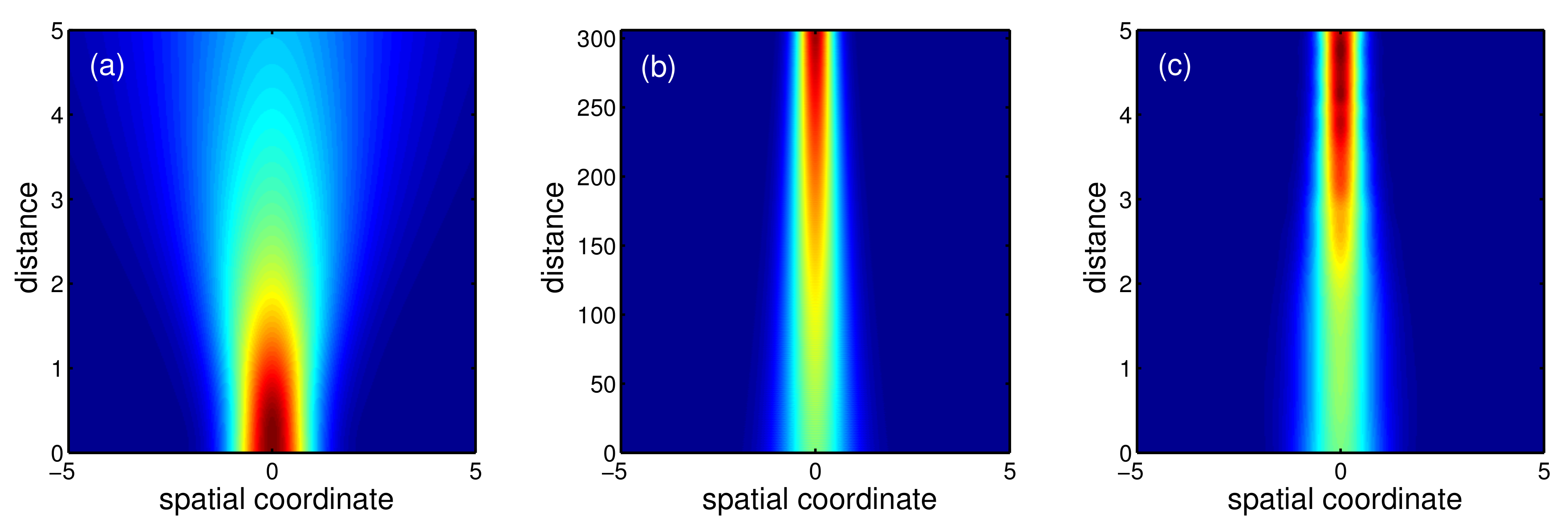
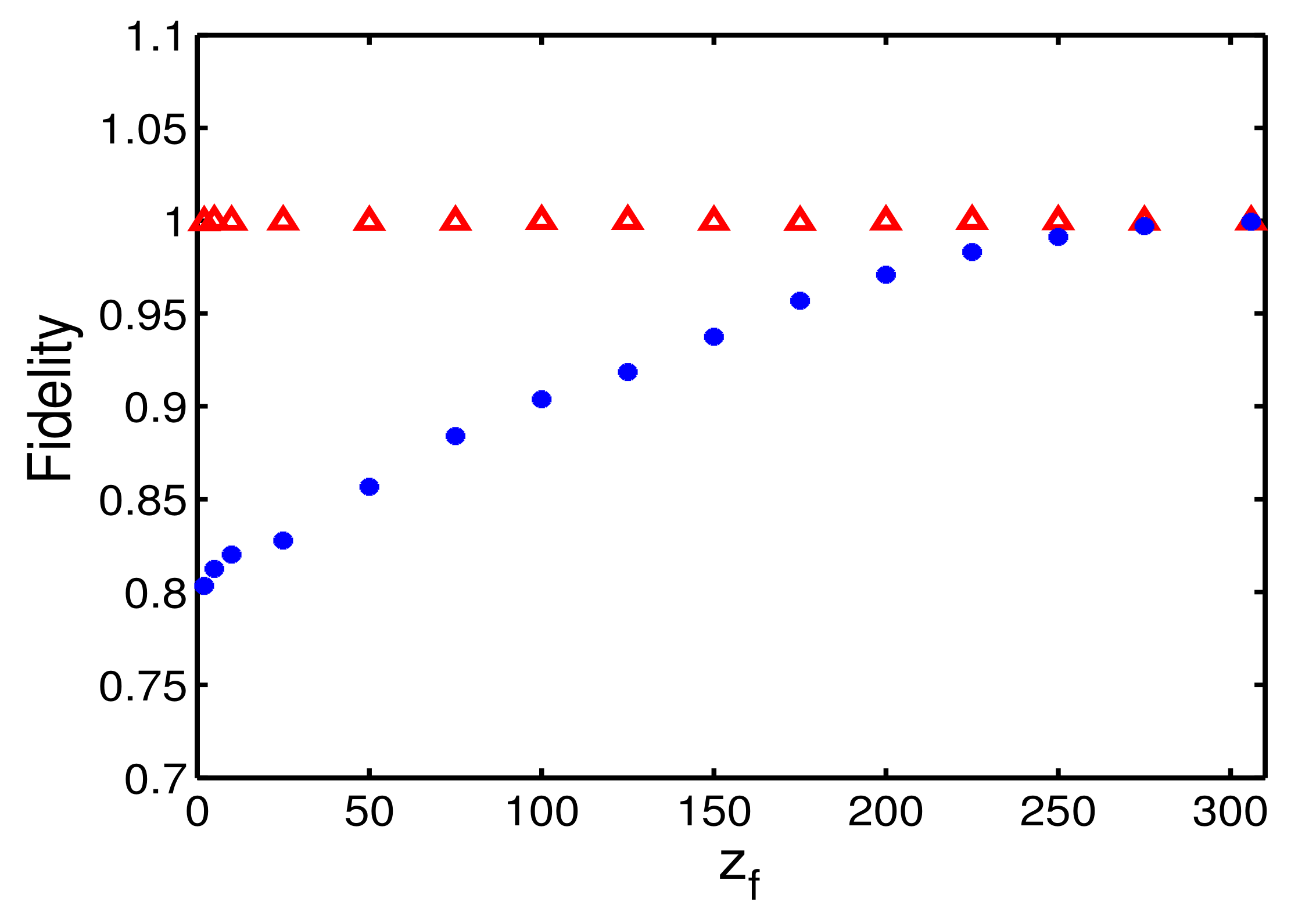
4. Inverse Engineering for Fast Compression
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Yariv, A. Quantum Electronics, 2nd ed.; John Wiley & Sons: New York, NY, USA, 1975; p. 123. [Google Scholar]
- Kogelnik, H.; Li, T. Laser beams and resonators. Appl. Opt. 1966, 5, 1550–1567. [Google Scholar] [CrossRef] [PubMed]
- Kogelnik, H. On the propagation of Gaussian beams of light through lenslike media including those with a loss or gain variation. Appl. Opt. 1965, 4, 1562–1569. [Google Scholar] [CrossRef]
- Okamoto, K. Fundamentals of Optical Waveguides, 2nd ed.; Academic Press: Cambridge, MA, USA, 2006. [Google Scholar]
- Dragoman, D.; Dragoman, M. Quantum-Classical Analogies; Springer: Berlin, Germany, 2004. [Google Scholar]
- Longhi, S. Quantum-optical analogies using photonic structures. Laser Photonics Rev. 2009, 3, 243–261. [Google Scholar] [CrossRef]
- Longhi, S.; Della Valle, G.; Ornigotti, M.; Laporta, P. Coherent tunneling by adiabatic passage in an optical waveguide system. Phy. Rev. B 2007, 76, 201101. [Google Scholar] [CrossRef]
- Rodríguez-Lara, B.; Soto-Eguibar, F.; Christodoulides, D.N. Quantum optics as a tool for photonic lattice design. Phys. Scr. 2015, 90, 068014. [Google Scholar] [CrossRef]
- Rangelov, A.A.; Vitanov, N.V. Achromatic multiple beam splitting by adiabatic passage in optical waveguides. Phys. Rev. A 2012, 85, 055803. [Google Scholar] [CrossRef]
- Mrejen, M.; Suchowski, H.; Hatakeyama, T.; Wu, C.; Feng, L.; O’Brien, K.; Wang, Y.; Zhang, X. Adiabatic elimination-based coupling control in densely packed subwavelength waveguides. Nat. Commun. 2015, 6, 7565. [Google Scholar] [CrossRef]
- Lahini, Y.; Pozzi, F.; Sorel, M.; Morandotti, R.; Christodoulides, D.N.; Silberberg, Y. Effect of nonlinearity on adiabatic evolution of light. Phys. Rev. Lett. 2008, 101, 193901. [Google Scholar] [CrossRef]
- Luo, X.; Huang, J.; Zhong, H.; Qin, X.; Xie, Q.; Kivshar, Y.S.; Lee, C. Pseudo-parity-time symmetry in optical systems. Phys. Rev. Lett. 2013, 110, 243902. [Google Scholar] [CrossRef]
- Torrontegui, E.; Ibáñez, S.; Martínez-Garaot, S. Shortcuts to adiabaticity. In Advances in Atomic, Molecular, and Optical Physics; Academic Press: Cambridge, MA, USA, 2013; Volume 62, pp. 117–169. [Google Scholar]
- Guéry-Odelin, D.; Ruschhaupt, A.; Kiely, A.; Torrontegui, E.; Martínez-Garaot, S.; Muga, J.G. Shortcuts to adiabaticity: Concepts, methods, and applications. Rev. Mod. Phys. 2019, 91, 045001. [Google Scholar] [CrossRef]
- Muga, J.G.; Chen, X.; Ruschhaupt, A.; Guéry-Odelin, D. Frictionless dynamics of Bose-Einstein condensates under fast trap variations. J. Phys. B At. Mol. Opt. Phys. 2009, 42, 241001. [Google Scholar] [CrossRef]
- Chen, X.; Ruschhaupt, A.; Schmidt, S.; del Campo, A.; Guéry-Odelin, D.; Muga, J.G. Fast optimal frictionless atom cooling in harmonic traps: Shortcut to adiabaticity. Phys. Rev. Lett. 2010, 104, 063002. [Google Scholar] [CrossRef] [PubMed]
- Demirplak, M.; Rice, S.A. Adiabatic Population Transfer with Control Fields. J. Phys. Chem. A 2003, 107, 9937–9945. [Google Scholar] [CrossRef]
- Berry, M.V. Transitionless quantum driving. J. Phys. A Math. Theor. 2009, 42, 365303. [Google Scholar] [CrossRef]
- Chen, X.; Lizuain, I.; Ruschhaupt, A.; Guéry-Odelin, D.; Muga, J. Shortcut to adiabatic passage in two-and three-level atoms. Phys. Rev. Lett. 2010, 105, 123003. [Google Scholar] [CrossRef]
- Deffner, S.; Jarzynski, C.; del Campo, A. Classical and quantum shortcuts to adiabaticity for scale-invariant driving. Phys. Rev. X 2014, 4, 021013. [Google Scholar] [CrossRef]
- Lewis, H.R. An Exact Quantum Theory of the Time-Dependent Harmonic Oscillator and of a Charged Particle in a Time-Dependent Electromagnetic Field. J. Math. Phys. 1969, 10, 1458. [Google Scholar] [CrossRef]
- Schaff, J.F.; Song, X.L.; Vignolo, P.; Labeyrie, G. Fast optimal transition between two equilibrium states. Phys. Rev. A 2010, 82, 033430. [Google Scholar] [CrossRef]
- Schaff, J.F.; Song, X.L.; Capuzzi, P.; Vignolo, P.; Labeyrie, G. Shortcut to adiabaticity for an interacting Bose-Einstein condensate. Europhys. Lett. 2010, 93, 23001. [Google Scholar] [CrossRef]
- Silveri, M.P.; Tuorila, J.A.; Thuneberg, E.V.; Paraoanu, G.S. Quantum systems under frequency modulation. Rep. Prog. Phys. 2017, 80, 056002. [Google Scholar] [CrossRef]
- Li, Y.; Wu, L.A.; Wang, Z.D. Fast ground-state cooling of mechanical resonators with time-dependent optical cavities. Phys. Rev. A 2011, 83, 043804. [Google Scholar] [CrossRef]
- Faure, S.; Ciliberto, S.; Trizac, E.; Guéry-Odelin, D. Shortcut to stationary regimes: A simple experimental demonstration. Am. J. Phys. 2019, 87, 125–129. [Google Scholar] [CrossRef]
- Stefanatos, D. Design of a photonic lattice using shortcuts to adiabaticity. Phys. Rev. A 2014, 90, 023811. [Google Scholar] [CrossRef]
- Wang, K.; Perez-Leija, A.; Weimann, S.; Szameit, A. Non-adiabatic dynamic-phase-free geometric phase in multiport photonic lattices. J. Opt. 2020, 22, 035801. [Google Scholar] [CrossRef]
- Zhang, J.; Shim, J.H.; Niemeyer, I.; Taniguchi, T.; Teraji, T.; Abe, H.; Suter, D. Experimental implementation of assisted quantum adiabatic passage in a single spin. Phys. Rev. Lett. 2013, 110, 240501. [Google Scholar] [CrossRef]
- Zhou, B.B.; Baksic, A.; Ribeiro, H.; Yale, C.G.; Heremans, F.J.; Jerger, P.C.; Auer, A.; Burkard, G.; Clerk, A.A.; Awschalom, D.D. Accelerated quantum control using superadiabatic dynamics in a solid-state lambda system. Nat. Phys. 2017, 13, 330–334. [Google Scholar] [CrossRef]
- Vepsäläinen, A.; Danilin, S.; Paraoanu, G.S. Superadiabatic population transfer in a three-level superconducting circuit. Sci. Adv. 2019, 5, eaau5999. [Google Scholar] [CrossRef]
- Stefanatos, D.; Ruths, J.; Li, J.S. Frictionless atom cooling in harmonic traps: A time-optimal approach. Phys. Rev. A 2010, 82, 063422. [Google Scholar] [CrossRef]
- Chen, X.; Torrontegui, E.; Stefanatos, D.; Li, J.S.; Muga, J.G. Optimal trajectories for efficient atomic transport without final excitation. Phys. Rev. A 2011, 84, 043415. [Google Scholar] [CrossRef]
- Lu, X.J.; Chen, X.; Alonso, J.; Muga, J.G. Fast transitionless expansions of Gaussian anharmonic traps for cold atoms: Bang-singular-bang control. Phys. Rev. A 2014, 89, 023627. [Google Scholar] [CrossRef]
- Tseng, S.Y.; Wen, R.D.; Chiu, Y.F.; Chen, X. Short and robust directional couplers designed by shortcuts to adiabaticity. Opt. Express 2014, 22, 18849–18859. [Google Scholar] [CrossRef] [PubMed]
- Tseng, S.Y. Robust coupled-waveguide devices using shortcuts to adiabaticity. Opt. Express 2014, 39, 6600–6603. [Google Scholar] [CrossRef] [PubMed]
- Chen, X.; Wen, R.D.; Tseng, S.Y. Analysis of optical directional couplers using shortcuts to adiabaticity. Opt. Express 2016, 24, 18322–18331. [Google Scholar] [CrossRef]
- Ho, C.P.; Tseng, S.Y. Optimization of adiabaticity in coupled-waveguide devices using shortcuts to adiabaticity. Opt. Express 2015, 40, 4831–4834. [Google Scholar] [CrossRef]
- Chung, H.C.; Martínez-Garaot, S.; Chen, X.; Muga, J.G.; Tseng, S.Y. Shortcuts to adiabaticity in optical waveguides. Europhys. Lett. 2019, 127, 34001. [Google Scholar] [CrossRef]
- Tseng, S.Y.; Chen, X. Engineering of fast mode conversion in multimode waveguides. Opt. Lett. 2012, 37, 5118–5120. [Google Scholar] [CrossRef]
- Chen, X.; Wang, H.W.; Ban, Y.; Tseng, S.Y. Short-length and robust polarization rotators in periodically poled lithium niobate via shortcuts to adiabaticity. Opt. Express 2014, 22, 24169–24178. [Google Scholar] [CrossRef]
- Della, V.G.; Perozziello, G.; Longhi, S. Shortcut to adiabaticity in full-wave optics for ultra-compact waveguide junctions. J. Opt. 2016, 18, 09LT03. [Google Scholar]
- Anderson, D. Variational approach to nonlinear pulse propagation in optical fibers. Phys. Rev. A 1983, 27, 3135. [Google Scholar] [CrossRef]
- Malomed, B.A. Variational methods in nonlinear fiber optics and related fields. Prog. Opt. 2002, 43, 71–193. [Google Scholar]
- Perez-Garcia, V.M.; Michinel, H.; Cirac, J.I.; Lewenstein, M.; Zoller, P. Low energy excitations of a Bose-Einstein condensate: A time-dependent variational analysis. Phys. Rev. Lett. 1996, 77, 5320. [Google Scholar] [CrossRef] [PubMed]
- Garcia-Ripoll, J.J.; Perez-Garcia, V.M.; Torres, P. Extended parametric resonances in nonlinear Schrödinger systems. Phys. Rev. Lett. 1999, 83, 1715. [Google Scholar] [CrossRef]
- Li, J.; Sun, K.; Chen, X. Shortcut to adiabatic control of soliton matter waves by tunable interaction. Sci. Rep. 2016, 6, 38258. [Google Scholar] [CrossRef]
- Li, J.; Fogarty, T.; Campbell, S.; Chen, X.; Busch, T. An efficient nonlinear Feshbach engine. New J. Phys. 2018, 20, 015005. [Google Scholar] [CrossRef]
- Paul, K.; Sarma, A.K. Nonlinear compression of temporal solitons in an optical waveguide via inverse engineering. Europhys. Lett. 2018, 121, 64001. [Google Scholar] [CrossRef]
- Xu, T.N.; Li, J.; Busch, T.; Chen, X.; Fogarty, T. Effects of coherence on quantum speed limits and shortcuts to adiabaticity in many-particle systems. Phys. Rev. Res. 2020, 2, 023125. [Google Scholar] [CrossRef]
- García-Ripoll, J.J.; Ruiz-Chamorro, A.; Torrontegui, E. Quantum control of transmon superconducting qubits. arXiv 2002, arXiv:2002.10320v2. [Google Scholar]
- Pérezgarcía, V.M.; Torres, P.; Garcíaripoll, J.J.; Michinel, H. Moment analysis of paraxial propagation in a nonlinear graded index fiber. J. Opt. B Quantum Semiclassical Opt. 2000, 2, 353. [Google Scholar] [CrossRef]
- Anderson, D.; Lisak, M.; Malomed, B.; Quirogateixeiro, M. Tunneling of an optical soliton through a fiber junction. J. Opt. Soc. Am. B 1994, 11, 2380–2384. [Google Scholar] [CrossRef]
- Quiroga-Teixeiro, M.L.; Anderson, D.; Andrekson, P.A.; Berntson, A.; Lisak, M. Efficient soliton compression by fast adiabatic amplification. J. Opt. Soc. Am. B 1996, 13, 687–692. [Google Scholar] [CrossRef]
- Eleuch, H.; Bennaceur, R. An optical soliton pair among absorbing three-level atoms. J. Opt. A Pure Appl. Opt. 2003, 5, 528. [Google Scholar] [CrossRef]
- Christodoulides, D.N.; Coskun, T.H.; Mitchell, M.; Segev, M. Multimode Incoherent Spatial Solitons in Logarithmically Saturable Nonlinear Media. Phys. Rev. Lett. 1998, 80, 2310. [Google Scholar] [CrossRef]
- Coskun, T.H.; Christodoulides, D.N.; Kim, Y.R.; Chen, Z.G.; Soljacic, M.; Segev, M. Bright spatial solitons on a partially incoherent background. Phys. Rev. Lett. 2000, 84, 2374. [Google Scholar] [CrossRef] [PubMed]
- Huang, T.Y.; Boris, A.; Malomed; Chen, X. Shortcuts to adiabaticity for an interacting Bose-Einstein condensate via exact solutions of the generalized Ermakov equation. Chaos 2020, 30, 053131. [Google Scholar] [CrossRef]
- Landau, L.D.; Lifshitz, E.M. Mechanics, 2nd ed.; Pergamon: Oxford, UK, 1969. [Google Scholar]
- Kruglov, V.I.; Peacock, A.C.; Harvey, J.D. Exact self-similar solutions of the generalized nonlinear Schrödinger equation with distributed coefficients. Phys. Rev. Lett. 2003, 90, 113902. [Google Scholar] [CrossRef] [PubMed]
- Eleuch, H.; Bennaceur, R. Autocorrelation function of microcavity-emitting field in the non-linear regime. Eur. Phys. J. D. 2010, 57, 259–264. [Google Scholar] [CrossRef]
- Mohamed, A.B.; Eleuch, H. Non-classical effects in cavity QED containing a nonlinear optical medium and a quantum well: Entanglement and non-Gaussanity. Eur. Phys. J. D. 2015, 69, 191. [Google Scholar] [CrossRef]
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kong, Q.; Ying, H.; Chen, X. Shortcuts to Adiabaticity for Optical Beam Propagation in Nonlinear Gradient Refractive-Index Media. Entropy 2020, 22, 673. https://doi.org/10.3390/e22060673
Kong Q, Ying H, Chen X. Shortcuts to Adiabaticity for Optical Beam Propagation in Nonlinear Gradient Refractive-Index Media. Entropy. 2020; 22(6):673. https://doi.org/10.3390/e22060673
Chicago/Turabian StyleKong, Qian, Huimin Ying, and Xi Chen. 2020. "Shortcuts to Adiabaticity for Optical Beam Propagation in Nonlinear Gradient Refractive-Index Media" Entropy 22, no. 6: 673. https://doi.org/10.3390/e22060673
APA StyleKong, Q., Ying, H., & Chen, X. (2020). Shortcuts to Adiabaticity for Optical Beam Propagation in Nonlinear Gradient Refractive-Index Media. Entropy, 22(6), 673. https://doi.org/10.3390/e22060673





