Shannon Entropy as an Indicator for Sorting Processes in Hydrothermal Systems
Abstract
1. Introduction
2. Materials and Methods
2.1. Rock Sample Sets
2.2. Shannon Entropy
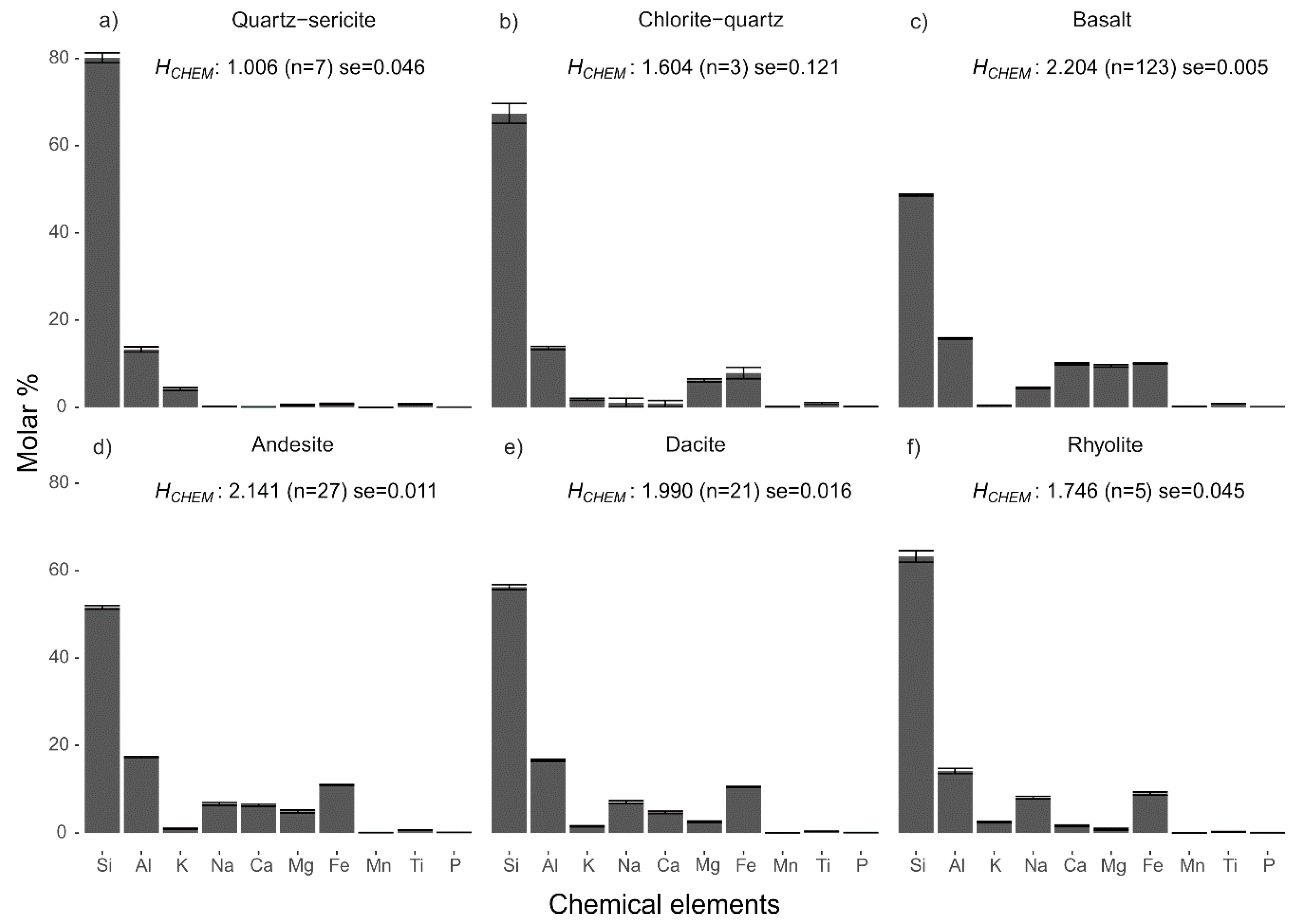
2.2.1. Chemical Shannon Entropy (HCHEM)
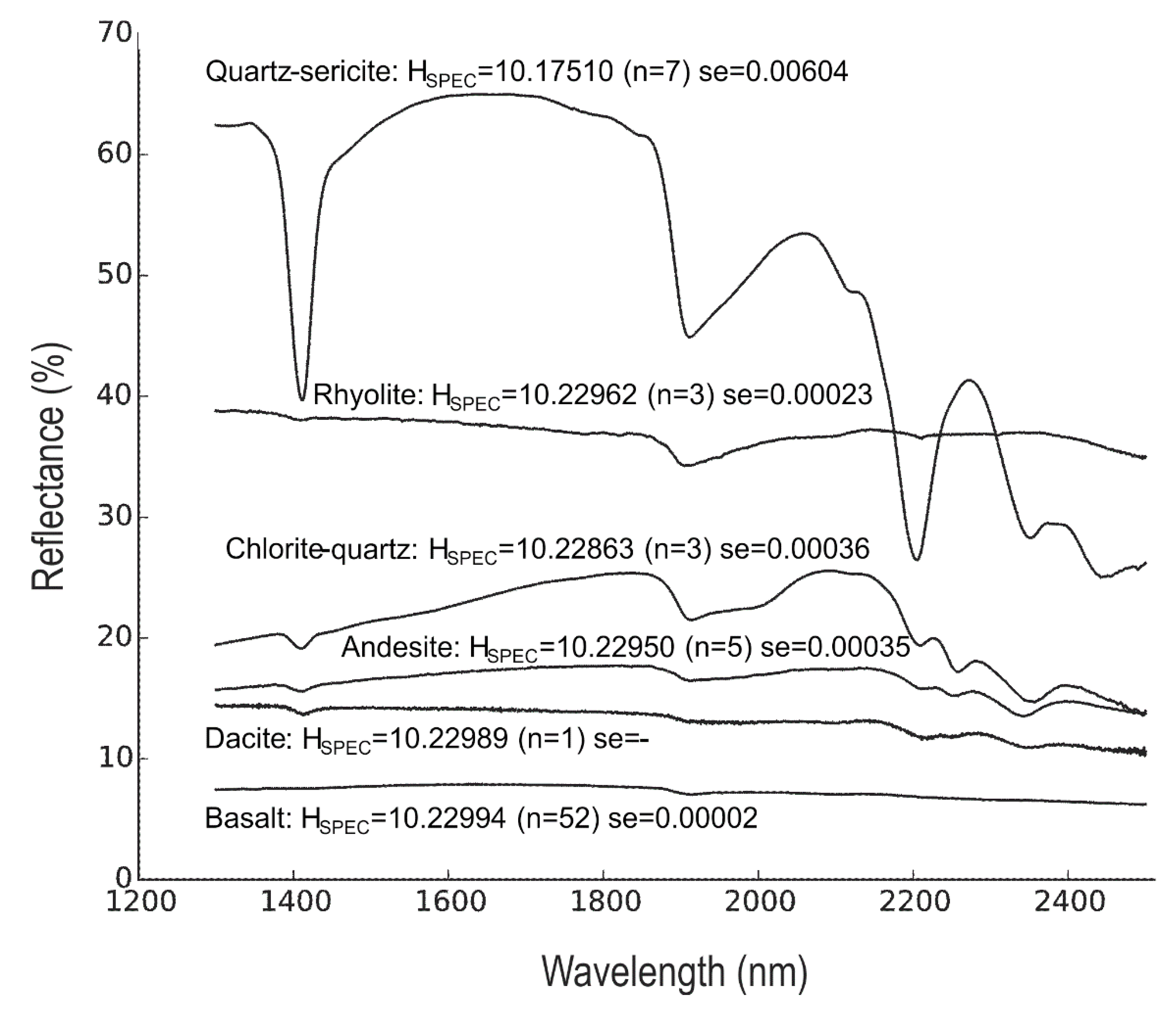
2.2.2. Spectral Shannon Entropy (HSPEC)
2.2.3. Change in Shannon Entropy
3. Results
3.1. Chemical Shannon Entropy
3.2. Spectral Shannon Entropy
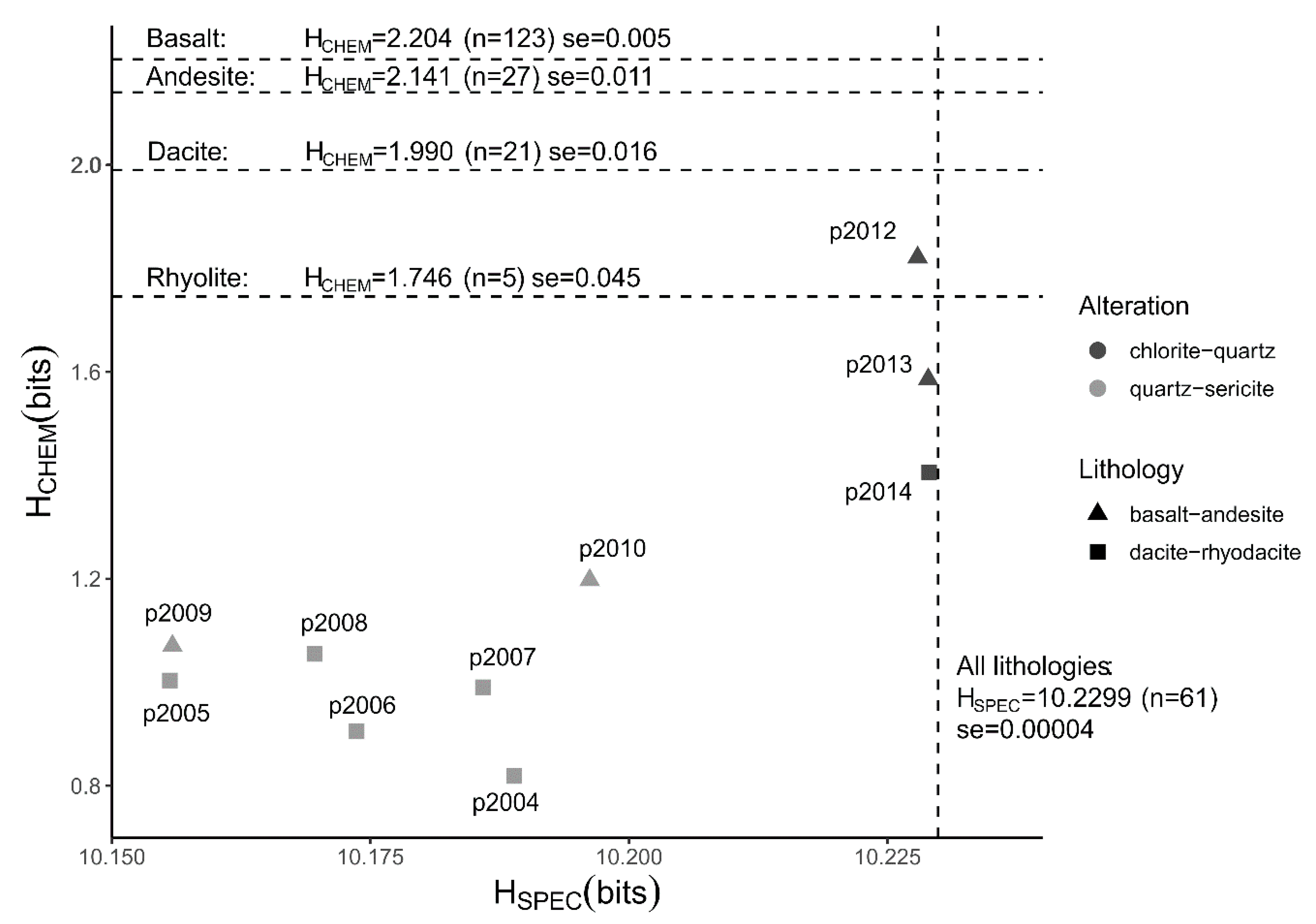
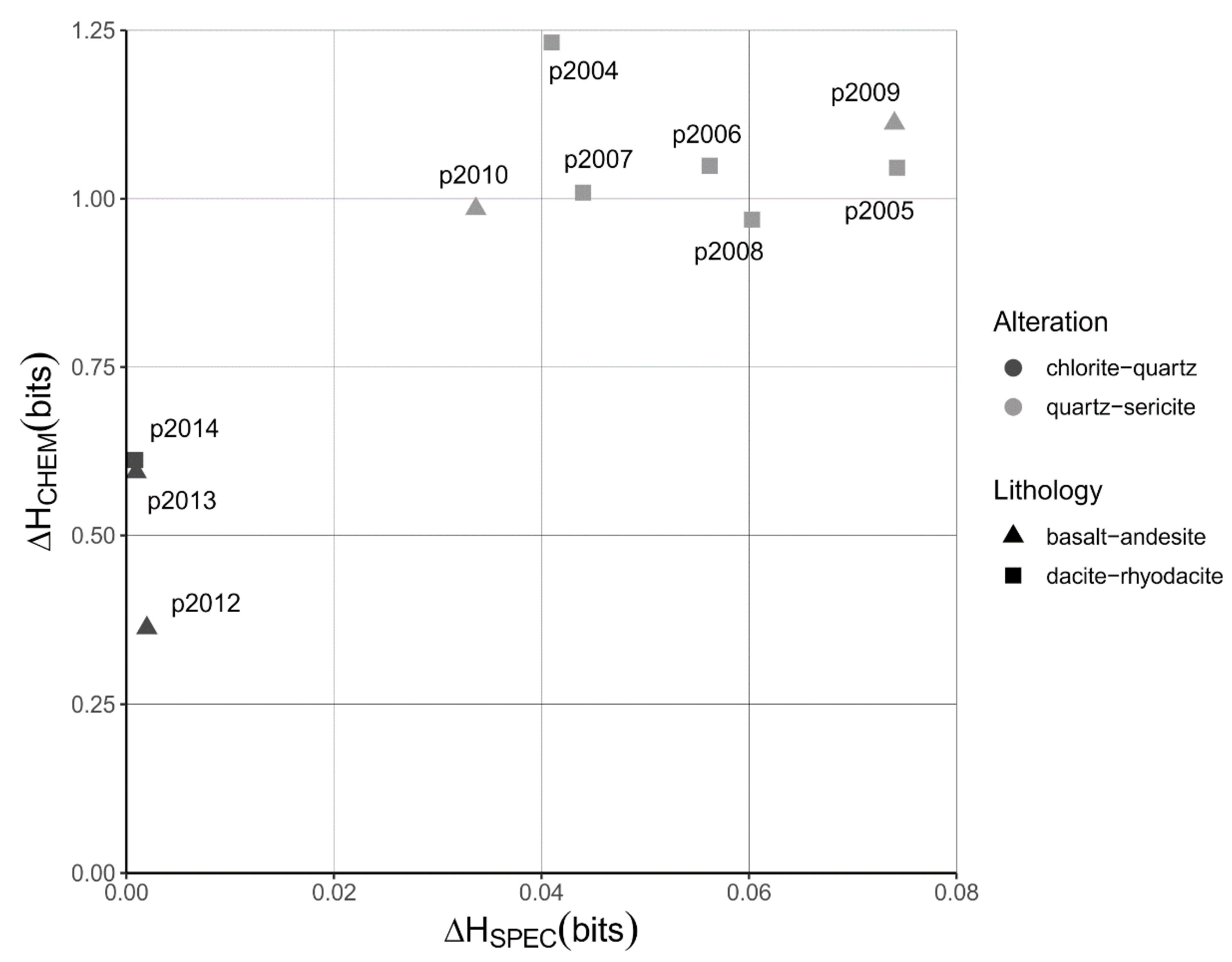
3.3. Changes in the Shannon Entropies
4. Discussion
4.1. Interpretation of the Shannon Entropy
4.2. Relationship between Heat, Hydrothermal Alteration and Shannon Entropy
4.3. Mineralized and Early Life Environments
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A
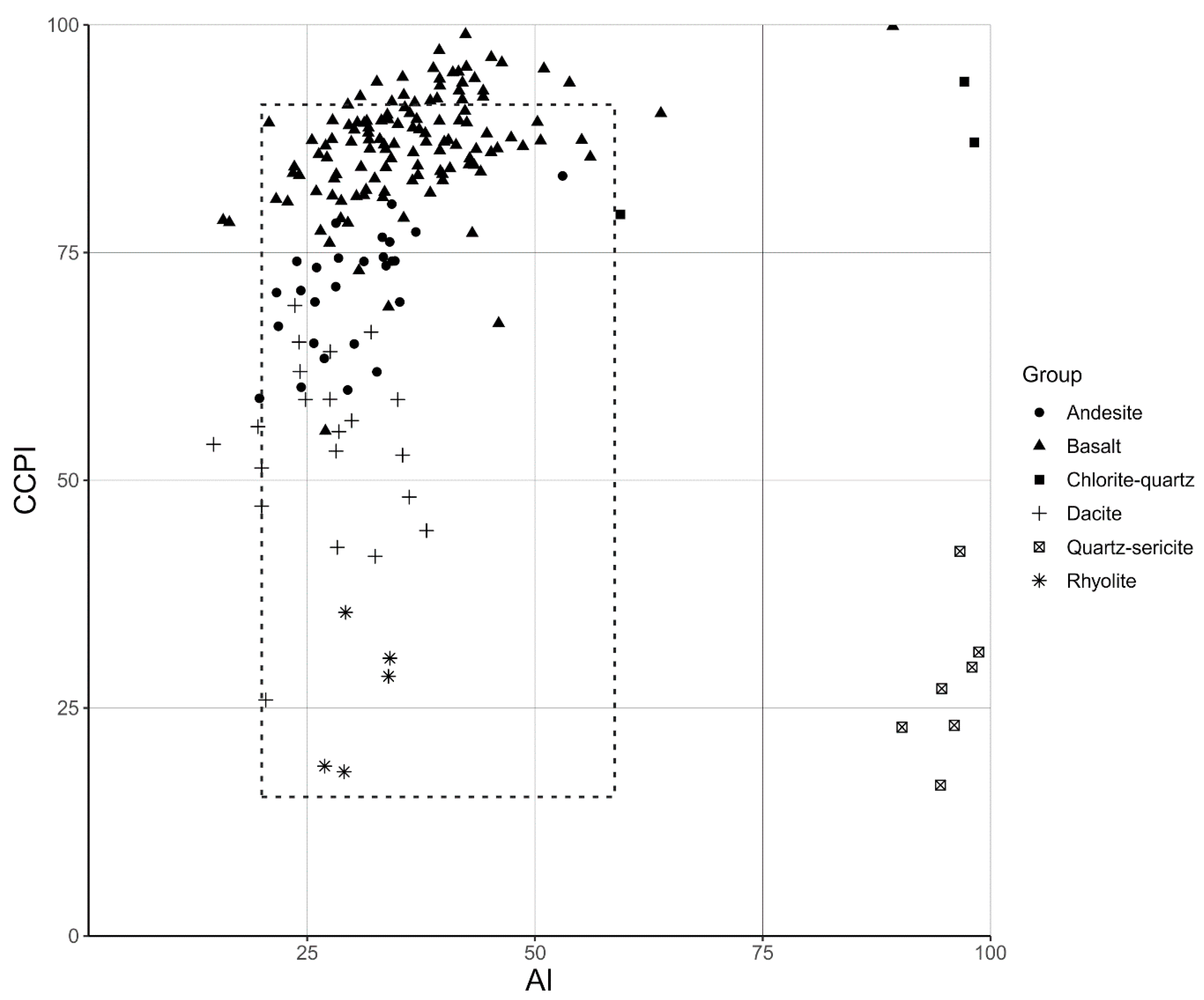
Appendix B

References
- Hemley, J.J.; Jones, W.R. Chemical aspects of hydrothermal alteration with emphasis on hydrogen metasomatism. Econ. Geol. 1964, 59, 538–569. [Google Scholar] [CrossRef]
- Pirajno, F. Hydrothermal Processes and Mineral Systems; Springer: Berlin, Germany, 2008; p. 1250. [Google Scholar] [CrossRef]
- Gifkins, C.; Herrmann, W.; Large, R.R. Altered Volcanic Rocks: A Guide to Description and Interpretation; Centre for Ore Deposit Research, University of Tasmania: Hobert, Australia, 2005. [Google Scholar]
- Pirajno, F. Hydrothermal Mineral Deposits: Principles and Fundamental Concepts for the Exploration Geologist; Springer: Berlin, Germany; New York, NY, USA, 1992; p. 709. [Google Scholar] [CrossRef]
- Djokic, T.; Van Kranendonk, M.J.; Campbell, K.A.; Walter, M.R.; Ward, C.R. Earliest signs of life on land preserved in ca. 3.5 Ga hot spring deposits. Nat. Commun. 2017, 8. [Google Scholar] [CrossRef] [PubMed]
- Baross, J.A.; Hoffman, S.E. Submarine hydrothermal vents and associated gradient environments as sites for the origin and evolution of life. Orig. Life Evol. Biosph. 1985, 15, 327–345. [Google Scholar] [CrossRef]
- Large, R.R.; Gemmell, J.B.; Paulick, H.; Huston, D.L. The alteration box plot: A simple approach to understanding the relationship between alteration mineralogy and lithogeochemistry associated with volcanic-hosted massive sulfide deposits. Econ. Geol. Bull. Soc. 2001, 96, 957–971. [Google Scholar] [CrossRef]
- Brauhart, C.W.; Huston, D.L.; Groves, D.I.; Mikucki, E.J.; Gardoll, S.J. Geochemical mass-transfer patterns as indicators of the architecture of a complete volcanic-hosted massive sulfide hydrothermal alteration system, Panorama district, Pilbara, Western Australia. Econ. Geol. Bull. Soc. 2001, 96, 1263–1278. [Google Scholar] [CrossRef]
- Lampinen, H.M.; Laukamp, C.; Occhipinti, S.A.; Hardy, L. Mineral footprints of the Paleoproterozoic sediment-hosted Abra Pb-Zn-Cu-Au deposit Capricorn Orogen, Western Australia. Ore Geol. Rev. 2019, 104, 436–461. [Google Scholar] [CrossRef]
- Van Ruitenbeek, F.J.A.; Cudahy, T.; Hale, M.; van der Meer, F.D. Tracing fluid pathways in fossil hydrothermal systems with near-infrared spectroscopy. Geology 2005, 33, 597–600. [Google Scholar] [CrossRef]
- Shannon, C.E. A mathematical theory of communication. Bell Syst. Tech. J. 1948, 27, 379–423. [Google Scholar] [CrossRef]
- Cover, T.M.; Thomas, J.A. Elements of Information Theory; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2006; p. 748. [Google Scholar] [CrossRef]
- Van Ruitenbeek, F.J.A.; Hein, K.A.A. Rock Sample Data from the Footwall of the Kangaroo Caves Cu-Zn deposit, Pilbara, Western Australia; DANS Easy: The Hague, The Netherlands, 2019. [Google Scholar] [CrossRef]
- Van Ruitenbeek, F.J.A.; van der Werff, H.M.A.; Hein, K.A.A.; van der Meer, F.D. Detection of pre-defined boundaries between hydrothermal alteration zones using rotation-variant template matching. Comput. Geosci.-UK 2008, 34, 1815–1826. [Google Scholar] [CrossRef]
- Brauhart, C.W.; Groves, D.I.; Morant, P. Regional alteration systems associated with volcanogenic massive sulfide mineralization at Panorama, Pilbara, Western Australia. Econ. Geol. Bull. Soc. 1998, 93, 292–302. [Google Scholar] [CrossRef]
- Smithies, R.H.; Champion, D.C.; van Kranendonk, M.J.; Hickman, A.H. Geochemistry of Volcanic Rocks of the Northern Pilbara Craton, Western Australia; Report 104; Western Australia Geological Survey: Perth, Australia, 2007; p. 47.
- Van Kranendonk, M.J.; Hickman, A.H.; Smithies, R.H.; Nelson, D.R.; Pike, G. Geology and tectonic evolution of the archean North Pilbara terrain, Pilbara Craton, Western Australia. Econ. Geol. Bull. Soc. 2002, 97, 695–732. [Google Scholar] [CrossRef]
- Baldridge, A.M.; Hook, S.J.; Grove, C.I.; Rivera, G. The ASTER spectral library version 2.0. Remote Sens. Environ. 2009, 113, 711–715. [Google Scholar] [CrossRef]
- Floyd, P.A.; Winchester, J.A. Identification and discrimination of altered and metamorphosed volcanic-rocks using immobile elements. Chem. Geol. 1978, 21, 291–306. [Google Scholar] [CrossRef]
- Clark, R.N.; King, T.V.V.; Klejwa, M.; Swayze, G.A.; Vergo, N. High spectral resolution reflectance spectroscopy of minerals. J. Geophys. Res.-Solid 1990, 95, 12653–12680. [Google Scholar] [CrossRef]
- Clark, R.N. Spectroscopy of rocks and minerals, and principles of spectroscopy. In Manual of Remote Sensing; Rencz, A.N., Ed.; John Wiley and Sons: New York, NY, USA, 1999; Volume 3, pp. 3–58. [Google Scholar]
- Brauhart, C.W.; Huston, D.L.; Andrew, A.S. Oxygen isotope mapping in the Panorama VMS district, Pilbara Craton, Western Australia: Applications to estimating temperatures of alteration and to exploration. Miner. Depos. 2000, 35, 727–740. [Google Scholar] [CrossRef]
- Ganguly, J. Thermodynamics in Earth and Planetary Sciences; Springer: Berlin/Heidelberg, Germany, 2008; p. 501. [Google Scholar] [CrossRef]
- Jaynes, E.T. Gibbs vs Boltzmann entropies. Am. J. Phys. 1965, 33, 391. [Google Scholar] [CrossRef]
- Batty, M. Spatial entropy. Geogr. Anal. 1974, 6, 1–31. [Google Scholar] [CrossRef]
- Westall, F.; Hickman-Lewis, K.; Hinman, N.; Gautret, P.; Campbell, K.A.; Breheret, J.G.; Foucher, F.; Hubert, A.; Sorieul, S.; Dass, A.V.; et al. A hydrothermal-sedimentary context for the origin of life. Astrobiology 2018, 18, 259–293. [Google Scholar] [CrossRef] [PubMed]
- Farmer, V.C. The Infrared Spectra of Minerals; Mineralogical Society: London, UK, 1974; p. 539. [Google Scholar]
- England, J.L. Statistical physics of self-replication. J. Chem. Phys. 2013, 139. [Google Scholar] [CrossRef] [PubMed]
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
van Ruitenbeek, F.J.A.; Goseling, J.; Bakker, W.H.; Hein, K.A.A. Shannon Entropy as an Indicator for Sorting Processes in Hydrothermal Systems. Entropy 2020, 22, 656. https://doi.org/10.3390/e22060656
van Ruitenbeek FJA, Goseling J, Bakker WH, Hein KAA. Shannon Entropy as an Indicator for Sorting Processes in Hydrothermal Systems. Entropy. 2020; 22(6):656. https://doi.org/10.3390/e22060656
Chicago/Turabian Stylevan Ruitenbeek, Frank J. A., Jasper Goseling, Wim H. Bakker, and Kim A. A. Hein. 2020. "Shannon Entropy as an Indicator for Sorting Processes in Hydrothermal Systems" Entropy 22, no. 6: 656. https://doi.org/10.3390/e22060656
APA Stylevan Ruitenbeek, F. J. A., Goseling, J., Bakker, W. H., & Hein, K. A. A. (2020). Shannon Entropy as an Indicator for Sorting Processes in Hydrothermal Systems. Entropy, 22(6), 656. https://doi.org/10.3390/e22060656




