Evanescent Wave Approximation for Non-Hermitian Hamiltonians
Abstract
1. Introduction
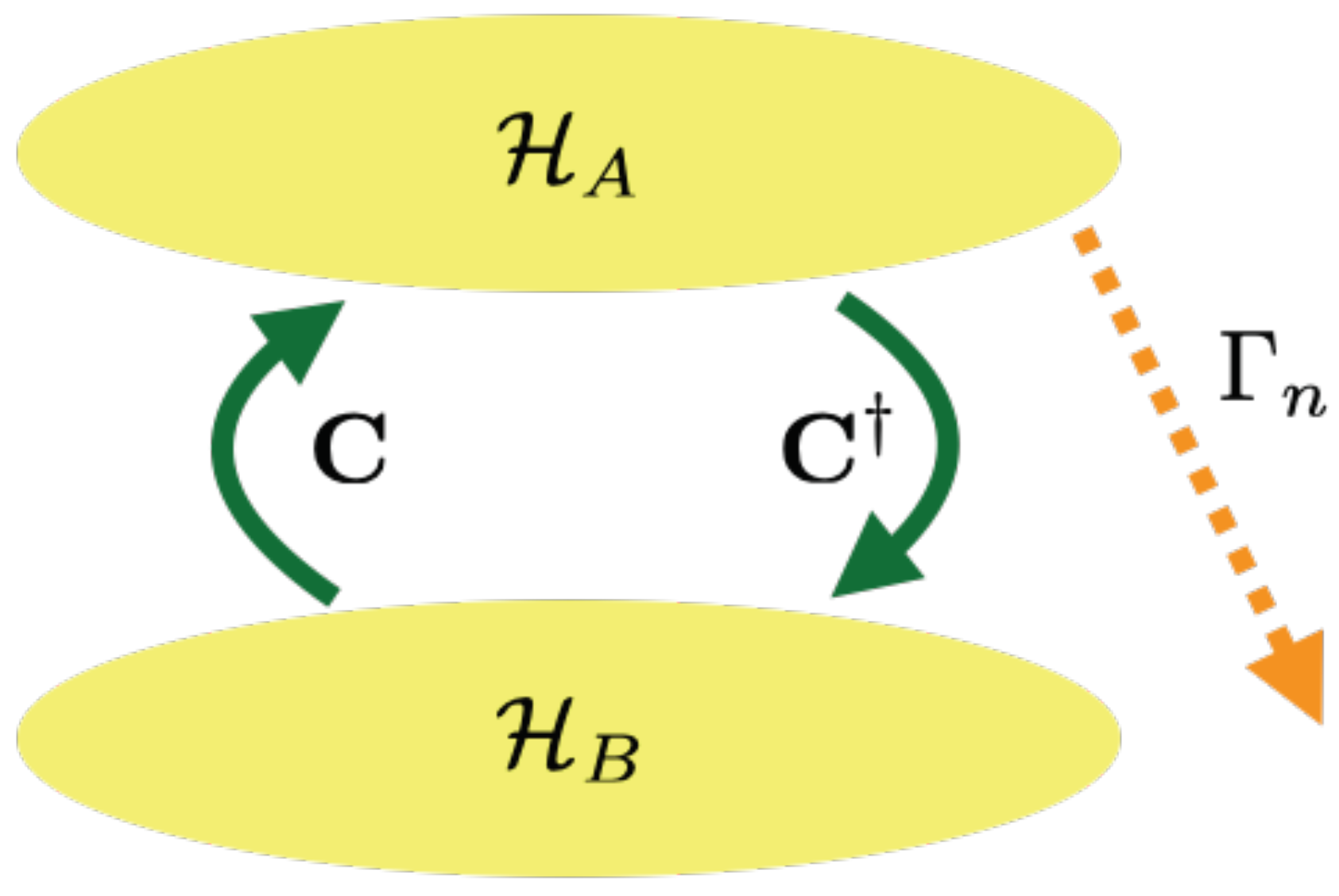
2. Handling the Model
2.1. Non-Hermitian Hamiltonian Model
2.2. Effective Hamiltonian: The Evanescent Wave Approximation
2.3. Quantum Zeno Effect
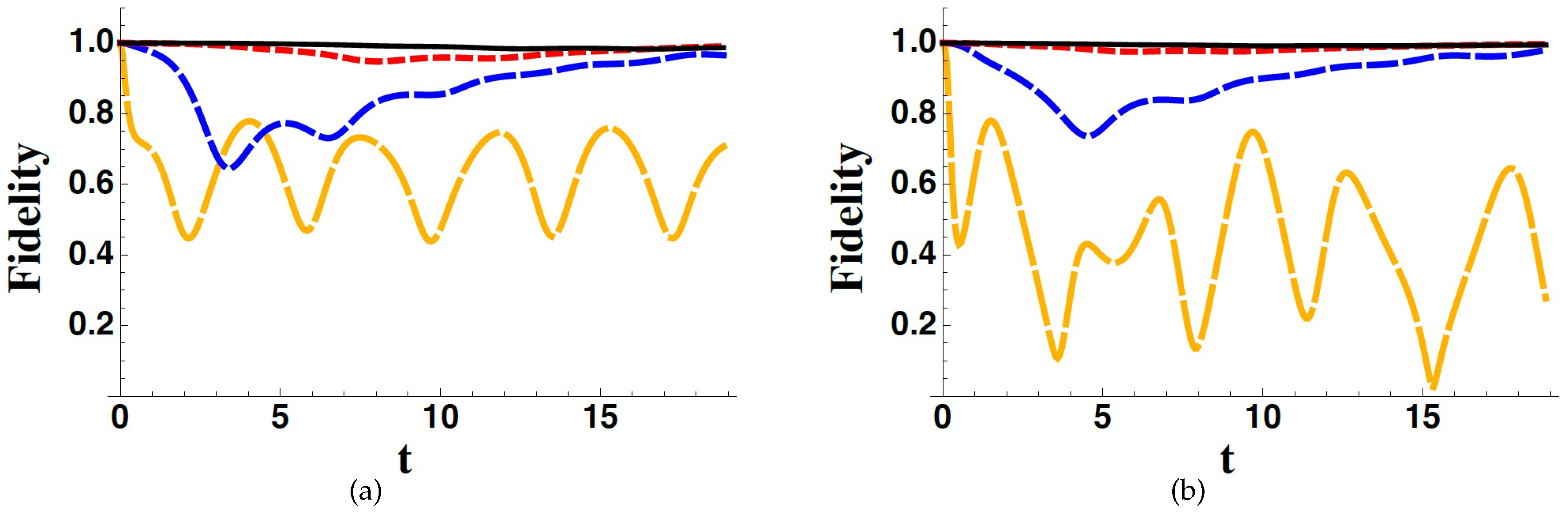
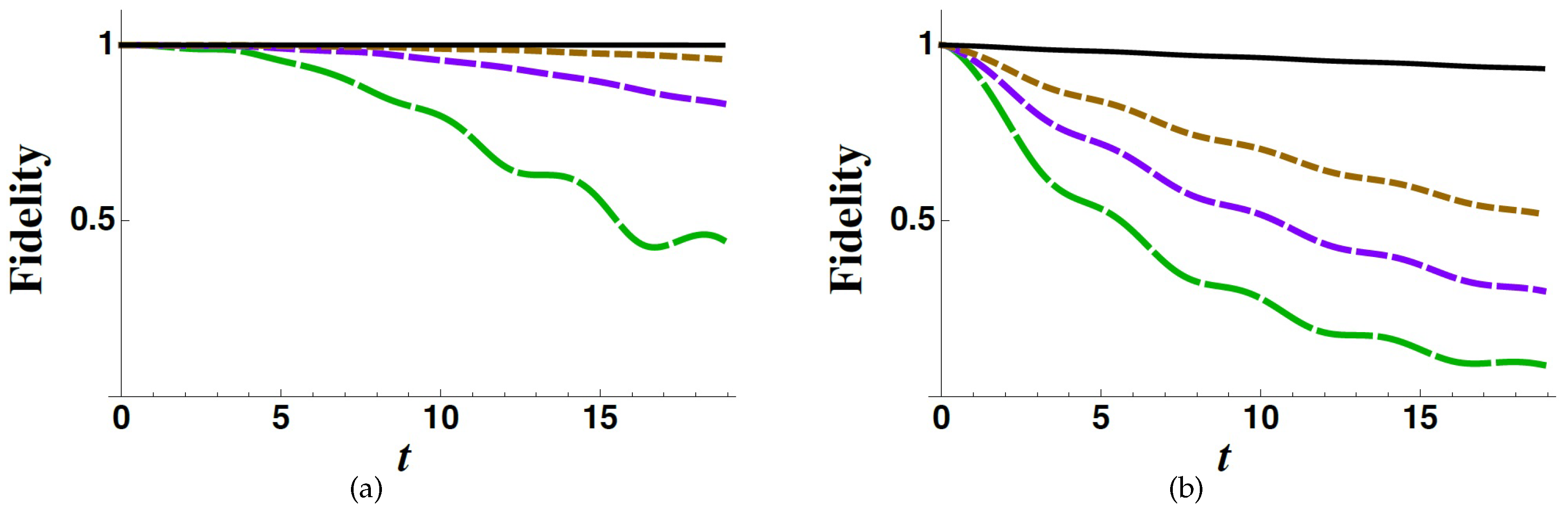
3. Numerical Analysis
4. Methods
4.1. Derivation of the Non-Hermitian Hamiltonian
4.2. Smallness of
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| NHH | Non-Hermitian Hamiltonian |
| RWA | Rotating Wave Approximation |
| EWA | Evanescent Wave Approximation |
References
- Xie, Q.; Zhong, H.; Batchelor, M.T.; Lee, C. The quantum Rabi model: Solution and dynamics. J. Phys. A Math. Theor. 2017, 50, 113001. [Google Scholar] [CrossRef]
- Shore, B.W.; Knight, P.L. The Jaynes–Cummings model. J. Mod. Opt. 1993, 40, 1195. [Google Scholar] [CrossRef]
- Puri, R.R.; Bullough, R.K. Quantum electrodynamics of an atom making two-photon transitions in an ideal cavity. J. Opt. Soc. Am. B 1988, 5, 2021–2028. [Google Scholar] [CrossRef]
- Steinbach, J.; Twamley, J.; Knight, P.L. Engineering two-mode interactions in ion traps. Phys. Rev. A 1997, 56, 4815. [Google Scholar] [CrossRef]
- Rahav, S.; Gilary, I.; Fishman, S. Effective Hamiltonians for periodically driven systems. Phys. Rev. A 2003, 68, 013820. [Google Scholar] [CrossRef]
- Aniello, P. A new perturbative expansion of the time evolution operator associated with a quantum system. J. Opt. B Quantum Semiclass. Opt. 2005, 7, S507–S522. [Google Scholar] [CrossRef]
- Shao, W.; Wu, C.; Feng, X.L. Generalized James’ effective Hamiltonian method. Phys. Rev. A 2017, 95, 032124. [Google Scholar] [CrossRef]
- Allen, L.; Eberly, J.H. Optical Resonance and Two-Level Atoms; John Wiley: New York, NY, USA, 1975. [Google Scholar]
- Bender, C.M.; Boettcher, S. Real Spectra in Non-Hermitian Hamiltonians Having Symmetry. Phys. Rev. Lett. 1998, 80, 5243. [Google Scholar] [CrossRef]
- Muga, J.G.; Echanobe, J.; del Campo, A.; Lizuain, I. Generalized relation between pulsed and continuous measurements in the quantum Zeno effect. J. Phys. B At. Mol. Opt. Phys. 2008, 41, 175501. [Google Scholar] [CrossRef]
- Rudner, M.S.; Levitov, L.S. Topological Transition in a Non-Hermitian Quantum Walk. Phys. Rev. Lett. 2009, 102, 065703. [Google Scholar] [CrossRef]
- Feng, L.; Ayache, M.; Huang, J.; Xu, Y.-L.; Lu, M.-H.; Chen, Y.-F.; Fainman, Y.; Scherer, A. Nonreciprocal Light Propagation in a Silicon Photonic Circuit. Science 2011, 333, 729. [Google Scholar] [CrossRef]
- Regensburger, A.; Bersch, C.; Miri, M.-A.; Onishchukov, G.; Christodoulides, D.N.; Peschel, U. Parity–time synthetic photonic lattices. Nature 2012, 488, 167. [Google Scholar] [CrossRef] [PubMed]
- Fyodorov, Y.V.; Savin, D.V. Statistics of Resonance Width Shifts as a Signature of Eigenfunction Nonorthogonality. Phys. Rev. Lett. 2012, 108, 184101. [Google Scholar] [CrossRef] [PubMed]
- Gros, J.B.; Kuhl, U.; Legrand, O.; Mortessagne, F.; Richalot, E.; Savin, D.V. Experimental Width Shift Distribution: A Test of Nonorthogonality for Local and Global Perturbations. Phys. Rev. Lett. 2014, 113, 224101. [Google Scholar] [CrossRef] [PubMed]
- Ashida, Y.; Furukawa, S.; Ueda, M. Parity-time-symmetric quantum critical phenomena. Nat. Commun. 2017, 8, 15791. [Google Scholar] [CrossRef] [PubMed]
- Nakagawa, M.; Kawakami, N.; Ueda, M. Non-Hermitian Kondo Effect in Ultracold Alkaline-Earth Atoms. Phys. Rev. Lett. 2018, 121, 203001. [Google Scholar] [CrossRef]
- Kawabata, K.; Higashikawa, S.; Gong, Z.; Ashida, Y.; Ueda, M. Topological unification of time-reversal and particle-hole symmetries in non-Hermitian physics. Nat. Commun. 2019, 10, 297. [Google Scholar] [CrossRef]
- Militello, B. Three-state Landau-Zener model in the presence of dissipation. Phys. Rev. A 2019, 99, 033415. [Google Scholar] [CrossRef]
- Militello, B. Detuning-induced robustness of a three-state Landau-Zener model against dissipation. Phys. Rev. A 2019, 99, 063412. [Google Scholar] [CrossRef]
- Michishita, Y.; Peters, R. Equivalence of Effective Non-Hermitian Hamiltonians in the Context of Open Quantum Systems and Strongly Correlated Electron Systems. Phys. Rev. Lett. 2020, 124, 196401. [Google Scholar] [CrossRef]
- Misra, B.; Sudarshan, E.C.G. Time evolution of unstable quantum states and a resolution of Zeno’s paradox. J. Math. Phys. 1997, 18, 7456. [Google Scholar]
- Presilla, C.; Onofrio, R.; Tambini, U. Measurement Quantum Mechanics and Experiments on Quantum Zeno Effect. Ann. Phys. 1996, 248, 95. [Google Scholar] [CrossRef]
- Home, D.; Whitaker, M.A.B. A Conceptual Analysis of Quantum Zeno; Paradox, Measurement, and Experiment. Ann. Phys. 1997, 258, 237. [Google Scholar] [CrossRef][Green Version]
- Schulman, L.S. Continuous and pulsed observations in the quantum Zeno effect. Phys. Rev. A 1998, 57, 1509. [Google Scholar] [CrossRef]
- Panov, A.D. General equation for Zeno-like effects in spontaneous exponential decay. Phys. Lett. A 1999, 260, 441. [Google Scholar] [CrossRef][Green Version]
- Audretsch, J.; Mensky, M.B.; Panov, A.D. Zeno effect preventing Rabi transitions onto an unstable energy level. Phys. Lett. A 1999, 261, 44. [Google Scholar] [CrossRef][Green Version]
- Facchi, P.; Pascazio, S. Quantum Zeno and inverse quantum Zeno effects. Prog. Opt. 2001. [Google Scholar] [CrossRef]
- Militello, B.; Messina, A.; Napoli, A. Driven Appearance and Disappearance of Quantum Zeno Effect in the Dynamics of a Four-level Trapped Ion. Fortschr. Phys. 2001, 49, 1041. [Google Scholar] [CrossRef]
- Facchi, P.; Pascazio, S. Quantum Zeno Subspaces. Phys. Rev. Lett. 2002, 89, 080401. [Google Scholar] [CrossRef]
- Facchi, P.; Pascazio, S. Quantum Zeno dynamics: Mathematical and physical aspects. J. Phys. A Math. Theor. 2008, 41, 493001. [Google Scholar] [CrossRef]
- Facchi, P.; Marmo, G.; Pascazio, S. Quantum Zeno dynamics and quantum Zeno subspaces. J. Phys. Conf. Ser. 2009, 196, 012017. [Google Scholar] [CrossRef]
- Militello, B.; Scala, M.; Messina, A.; Vitanov, N.V. Zeno-like phenomena in STIRAP processes. Phys. Scr. 2011, 2011, 014019. [Google Scholar] [CrossRef]
- Militello, B.; Napoli, A. Hilbert space partitioning for non-Hermitian Hamiltonians: Fromoff-resonance to Zeno subspaces. Phys. Lett. A 2020, 384, 126355. [Google Scholar] [CrossRef]
- Gardiner, C.W.; Zoller, P. Quantum Noise; Springer: Berlin, Germany, 2000. [Google Scholar]
- Breuer, H.-P.; Petruccione, F. The Theory of Open Quantum Systems; Oxford University Press: Oxford, UK, 2002. [Google Scholar]

© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Militello, B.; Napoli, A. Evanescent Wave Approximation for Non-Hermitian Hamiltonians. Entropy 2020, 22, 624. https://doi.org/10.3390/e22060624
Militello B, Napoli A. Evanescent Wave Approximation for Non-Hermitian Hamiltonians. Entropy. 2020; 22(6):624. https://doi.org/10.3390/e22060624
Chicago/Turabian StyleMilitello, Benedetto, and Anna Napoli. 2020. "Evanescent Wave Approximation for Non-Hermitian Hamiltonians" Entropy 22, no. 6: 624. https://doi.org/10.3390/e22060624
APA StyleMilitello, B., & Napoli, A. (2020). Evanescent Wave Approximation for Non-Hermitian Hamiltonians. Entropy, 22(6), 624. https://doi.org/10.3390/e22060624





