What Is So Special about Quantum Clicks?
Abstract
1. Quantum Hocus Pocus
2. General Principles for Object/Observable Construction
- negative; that is, dangerous, such as poisonous snakes, predators, atmospheric phenomena; or
- positive; that which qualifies as prey/loot/prize with respect to nutrition or joy, such as eating/drinking/reproducing/breathing.
- What qualifies a lump of stuff to be subjected to object/observable construction and become “an object” or “an observable” is its function with respect to us: otherwise—that is if it does not kill us or we cannot eat it et cetera—we might as well not perceive it as an individual entity separate from the rest of the stuff surrounding us.
- One might also speculate that every cub or human infant reenacts this structuralization of the environment–which was previously perceived ubiquitous, as a whole and non-separated (cf. also Piaget) from the cognitive agent–the whole issue of “external” versus “internal” comes into mind.
- as a consequence we as scientists have to be aware of these “hard-wired” conceptualizations or object constructions we and our species grew up with as “evident”, which have served our species well, but which eventually are too rigid and non-adaptive to be useful for the upcoming (deo volente) progressive research programs of Nature.
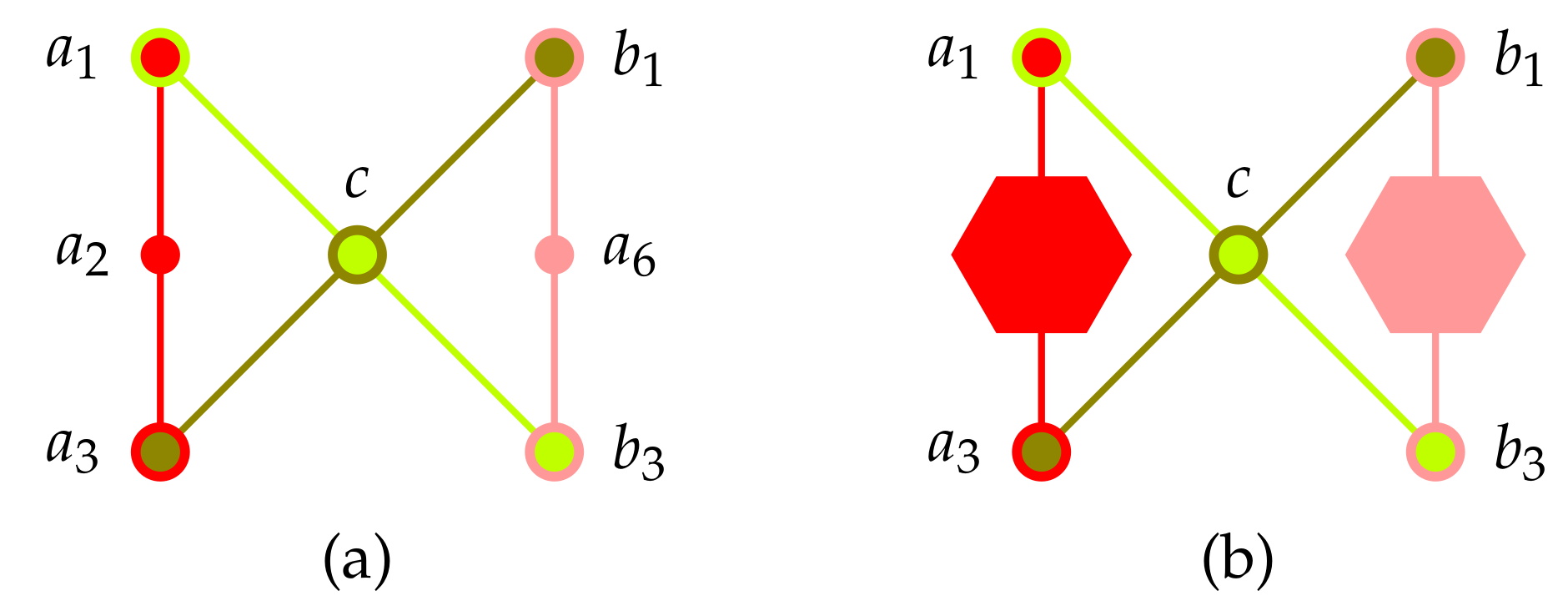
3. Context and Greechie Orthogonality Hypergraphs
- entire contexts (Boolean subalgebras, blocks) are drawn as smooth lines, such as straight (unbroken) lines, circles or ellipses;
- the atomic propositions of the context are drawn as circles; and
- contexts intertwining at a single atomic proposition are represented as non-smoothly connected lines, broken at that proposition.
4. General Principles for Probabilities of Objects/Observables
- A1:
- classical (sub)sets of finite (possibly extended) propositional/observable structures entail Kolmogorovian-type probabilities. In particular, they imply that within one and the same context, the corresponding probabilities are
- K1
- (non-negativity): non-negative real numbers;
- K2
- (unity): of unit measure; that is, the probability of the occurrence of a complete set of propositions/observables is one;
- K3
- (additivity): the probabilities of mutually exclusive events add up; that is, the probability of occurrence of all of them is the sum of the probabilities of occurrence of all of them; that is, .
- A2:
- (extended unity): Suppose there are two contexts and . Then the sum of the conditional probabilities of all the elements of the second context, relative to any single element of the first context, adds up to one [37].
5. Classical Predictions: Truth Assignments and Probabilities
5.1. Truth Assignments as Two-Valued Measures, Frame Functions and Admissibility of Probabilities
- The (quantum) logic community uses the term two-valued state; or, alternatively, valuation for a total function v on all elements of some logic L mapping such that (Definition 2.1.1, p. 20 in [39])
- (a)
- ,
- (b)
- if is a sequence of mutually orthogonal elements in L—in particular, this applies to atoms within the same context (block, Boolean subalgebra)—then the two-valued state is additive on those elements ; that is, additivity holds:
- Gleason has used the term frame function [36] (p. 886) of weight 1 for a separable Hilbert space as a total, real-valued (not necessarily two-valued) function f defined on the (surface of the) unit sphere of such that if represents an orthonormal basis of , then additivityholds for all orthonormal bases (contexts, blocks) of the logic based on .
- A dichotomic total function will be called strongly admissible if
- SAD1
- within every context , a single atom is assigned the value one: ; and
- SAD2
- all other atoms in that context are assigned the value zero: . Physically this amounts to only one elementary proposition being true; the rest of them are false. (One may think of an array of mutually exclusively firing detectors.)
- SAD3
- Non-contextuality, stated explicitly: The value of any observable, and, in particular, of an atom in which two contexts intertwine, does not depend on the context. It is context-independent.
- To cope with value indefiniteness (cf. Section 5.7.3), a weaker form of admissibility was proposed [40,41,42,43] which is no total function but rather is a partial function which may remain undefined (indefinite) on some elements of L: A dichotomic partial function will be called admissible if the following two conditions hold for every context C of L:
- WAD1
- if there exists a with , then for all ;
- WAD2
- if there exists a with for all , then ;
- WAD3
- the value assignments of all other elements of the logic not covered by, if necessary, successive application of the admissibility rules, are undefined and thus the atom remains value indefinite.
5.2. Boole’s Conditions of Possible Experience
- (i)
- the classical probabilities of certain events, as well as
- (ii)
- the classical probabilities of their (joint) occurrence, formalizable by products of the former “elementary” probabilities (i),
5.3. The Convex Polytope Method
5.3.1. Why Consider Classical Correlation Polytopes when Dealing with Quantized Systems?
- they represent a systematic way of enumerating the probability distributions and deriving constraints—Boole’s conditions of possible experience—on them;
- one can be sure that these constraints and bounds are optimal in the sense that they are guaranteed to yield inequalities which are the best criteria for classicality.
5.3.2. What Terms May Enter Classical Correlation Polytopes?
5.3.3. General Framework for Computing Boole’s Conditions of Possible Experience
- define the terms which should enter the bounds;
- (a)
- if the bounds should be on the probabilities: evaluate all two-valued measures interpretable as truth assignments;
- (b)
- if the bounds should be on the expectations: evaluate all value assignments of the observables;
- (c)
- if (as for non-contextual inequalities) the bounds should be on some pre-defined quantities: evaluate all such value definite pre-assigned quantities;
- arrange these terms into vectors whose components are all evaluated for a fixed two-valued state, one state at a time; one vector per two-valued state (truth assignment), or (for expectations) per value assignments of the observables, or (for non-contextual inequalities) per value-assignment;
- consider the set of all obtained vectors as vertices of a convex polytope;
- solve the convex hull problem by computing the convex hull, thereby finding the smallest convex polytope containing all these vertices. The solution can be represented as the half-spaces (characterizing the facets of the polytope) formalized by (in)equalities—(in)equalities which can be identified with Boole’s conditions of possible experience.
5.4. Non-Intertwined Contexts: Einstein-Podolsky-Rosen Type “Explosion” Setups of Joint Distributions
5.5. Truth Assignments and Predictions for Intertwined Contexts
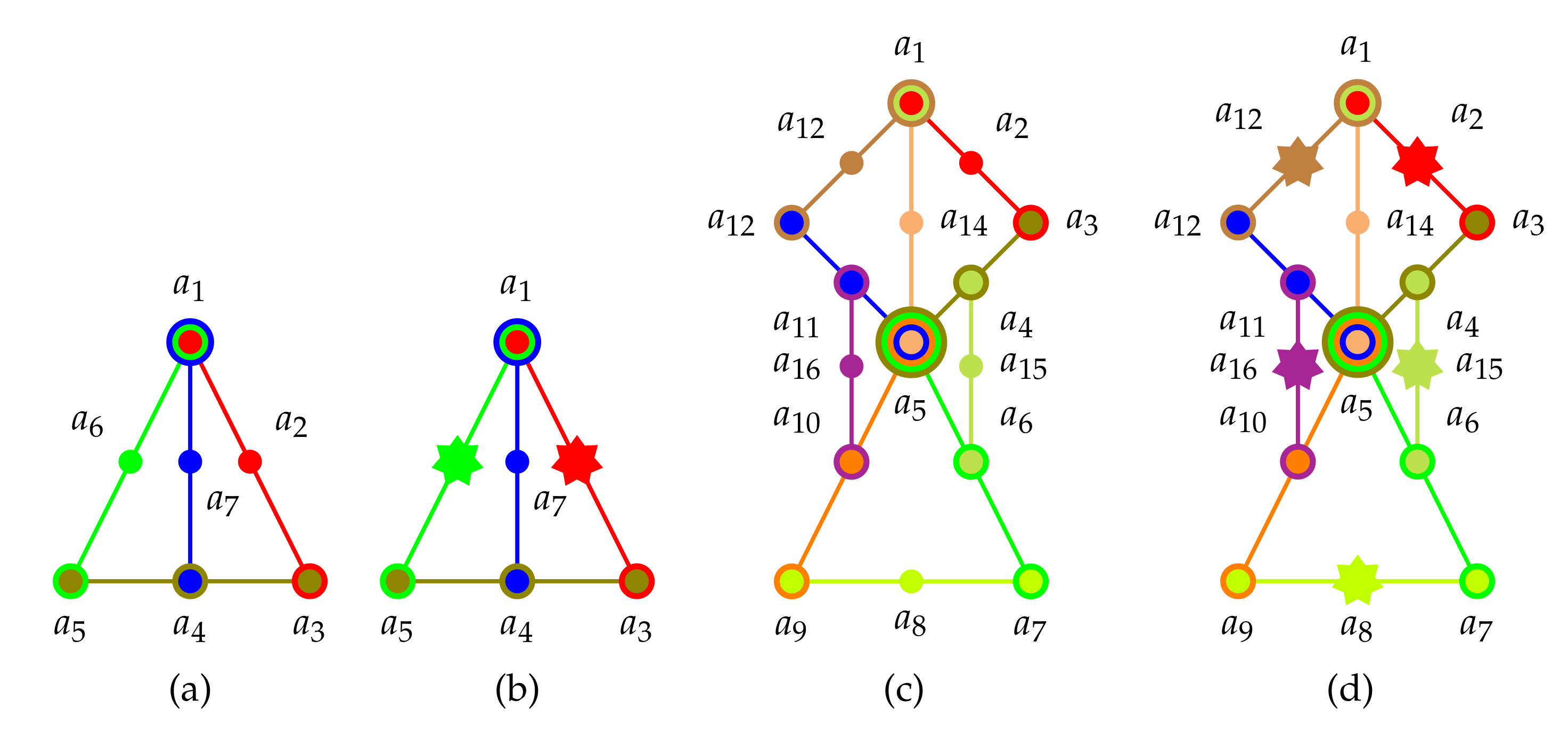
- 0th order gadget: a single context (aka clique/block/Boolean (sub)algebra/maximal observable/orthonormal basis);
- 1st order “firefly” gadget: two contexts connected in a single intertwining atom;
- 2nd order gadget: two 1st order firefly gadgets connected in a single intertwining atom;
- 3rd order house/pentagon/pentagram gadget: one firefly and one 2nd order gadget connected in two intertwining atoms to form a cyclic orthogonality diagram (hypergraph);
- 4th order true-implies-false (TIFS)/01-(maybe better 10)-gadget: e.g., a Specker bug consisting of two pentagon gadgets connected by an entire context; as well as extensions thereof to arbitrary angles for terminal (“extreme”) points;
- 5th order true-implies-true (TITS)/11-gadget: e.g., Kochen and Specker’s , consisting of one 10-gadget and one firefly gadget, connected at the respective terminal points;
- 6th order gadget: e.g., Kochen and Specker’s , consisting of a combo of two 11-gadgets, connected by their common firefly gadgets;
5.5.1. Probabilities on the Firefly Gadget
5.5.2. Probabilities on the House/Pentagon/Pentagram
5.5.3. Deterministic Predictions and Probabilities on the Specker Bug
5.5.4. Deterministic Predictions of Kochen-Specker’s “True Implies True” Logic
5.6. Beyond Classical Embedability
5.6.1. Deterministic Predictions on a Combo of Two Interlinked Specker Bugs
5.6.2. Deterministic Predictions on Observables with a Nun-Unital Set Of Two-Valued States
5.6.3. Direct Probabilistic Criteria against Value Definiteness from Constraints on Two-Valued Measures
5.7. Finite Propositional Structures Admitting Neither Truth Assignments nor Predictions
5.7.1. Scarcity of Two-Valued States
5.7.2. Chromatic Number of the Sphere
5.7.3. Exploring Value Indefiniteness
- (i)
- either ,
- (ii)
- or and ,
- (iii)
- or and .
5.7.4. How Can You Measure a Contradiction?
5.7.5. Non-Contextual Inequalities
6. Quantum Predictions: Probabilities and Expectations
6.1. Gleason-Type Continuity
6.2. Comparison of Classical and Quantum form of Correlations
6.3. Min-Max Principle
- All such terms, in particular, probabilities and nth order correlations or expectations, have a quantum realization as self-adjoint transformations. As coherent superpositions (linear sums and differences) of self-adjoint transformations are again self-adjoint transformations (and thus normal operators), they are subject to the spectral theorem. Therefore, effectively, all those terms are “bundled together” to give a single “comprehensive” (for Boole’s conditions of possible experience) observable.
- The spectral theorem, when applied to self-adjoint transformations obtained from substituting the quantum terms for the classical terms, yields an eigensystem consisting of all (pure or non-pure) states, as well as the associated eigenvalues which, according to the quantum mechanical axioms, serve as the measurement outcomes corresponding to the combined, bundled, “comprehensive”, observables. (In the usual Einstein-Podolsky-Rosen “explosion type” setup these quantities will be highly non-local.) The important observation is that this “comprehensive” (for Boole’s conditions of possible experience) observable encodes or includes all possible one-by-one measurements on each one of the single terms alone, at least insofar as they pertain to Boole’s conditions.
- By taking the minimal and the maximal eigenvalue in the spectral sum of this comprehensive observable one, therefore, obtains the minimal and the maximal measurement outcomes “reachable” by quantization.
- take all terms containing probabilities, correlations or expectations and the constant real-valued coefficients which are their multiplicative factors; thereby excluding single constant numerical values (which could be written on “the other” side of the inequality; resulting if what might look like “” (usually, these inequalities, for reasons of operationalizability, as discussed earlier, do not include highter than 2rd order correlations), and thereby define a function T;
- in the transition “quantization” step substitute all classical probabilities and correlations or expectations with the respective quantum self-adjoint operators, such as for two spin- particles, , , , , as demanded by the inequality. Please note that since the coefficients in are all real-valued, and because for arbitrary self-adjoint transformations , the real-valued weighted sum of self-adjoint transformations is again self-adjoint.
- Finally, compute the eigensystem of ; in particular the largest eigenvalue and the associated projector which, in the non-degenerate case, is the dyadic product of the “maximal state” , or .
- In a last step, maximize (and find the associated eigenvector ) with respect to variations of the parameters incurred in step (ii).
6.3.1. Expectations from Quantum Bounds
6.3.2. Quantum Bounds on the House/Pentagon/Pentagram Logic
6.3.3. Quantum Bounds on the Cabello, Estebaranz and García-Alcaine Logic
7. Epistemologic Deceptions
Supplementary Materials
Funding
Acknowledgments
Conflicts of Interest
References and Note
- Bell, J.S. Against ‘measurement’. Phys. World 1990, 3, 33–41. [Google Scholar] [CrossRef]
- Svozil, K. Quantum hocus-pocus. Ethics Sci. Environ. Politics 2016, 16, 25–30. [Google Scholar] [CrossRef][Green Version]
- Clauser, J. Early History of Bell’s Theorem. In Quantum (Un)speakables: From Bell to Quantum Information; Bertlmann, R., Zeilinger, A., Eds.; Springer: Berlin, Germany, 2002; pp. 61–96. [Google Scholar] [CrossRef]
- Mermin, D.N. What’s Wrong with this Pillow? Phys. Today 1989, 42, 9–11. [Google Scholar] [CrossRef]
- Mermin, D.N. Could Feynman Have Said This? Phys. Today 1989, 57, 10–11. [Google Scholar] [CrossRef]
- Feynman, R.P. The Character of Physical Law; MIT Press: Cambridge, MA, USA, 1965. [Google Scholar]
- Fuchs, C.A.; Peres, A. Quantum theory needs no ‘Interpretation’. Phys. Today 2000, 53, 70–71. [Google Scholar] [CrossRef]
- Englert, B.G. On quantum theory. Eur. Phys. J. D 2013, 67, 1–16. [Google Scholar] [CrossRef]
- Halmos, P.R. Finite-Dimensional Vector Spaces; Undergraduate Texts in Mathematics; Springer: New York, NY, USA, 1958. [Google Scholar] [CrossRef]
- Dyson, F.J. Divergence of Perturbation Theory in Quantum Electrodynamics. Phys. Rev. 1952, 85, 631–632. [Google Scholar] [CrossRef]
- Le Guillou, J.C.; Zinn-Justin, J. Large-Order Behaviour of Perturbation Theory; Current Physics-Sources and Comments; Elsevier: Amsterdam, The Netherlands, 2013; Volume 7. [Google Scholar]
- Pernice, S.A.; Oleaga, G. Divergence of perturbation theory: Steps towards a convergent series. Phys. Rev. D 1998, 57, 1144–1158. [Google Scholar] [CrossRef]
- Popper, K.R. Logik der Forschung; Springer: Berlin, Germany, 1934. [Google Scholar] [CrossRef]
- Lakatos, I. The Methodology of Scientific Research Programmes; Philosophical Papers; Cambridge University Press: Cambridge, UK, 2012; Volume 1. [Google Scholar] [CrossRef]
- Birkhoff, G.; von Neumann, J. The Logic of Quantum Mechanics. Ann. Math. 1936, 37, 823–843. [Google Scholar] [CrossRef]
- Berkeley, G. A Treatise Concerning the Principles of Human Knowledge; Aaron Rhames, for Jeremy Pepyat; Bookseller: Dublin, Ireland, 1710. [Google Scholar]
- Plato. The Republic; Cambridge Texts in the History of Political Thought; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Gigerenzer, G. Gut Feelings: The Intelligence of the Unconscious; Viking: New York, NY, USA, 2007. [Google Scholar]
- Chabris, C.; Simons, D. The Invisible Gorilla And Other Ways Our Intuitions Deceive US; Crown Archetype: New York, NY, USA, 2010. [Google Scholar]
- Kahneman, D. Thinking, Fast and Slow; Farrar, Straus and Giroux: New York, NY, USA, 2011. [Google Scholar]
- Urbaniok, F. Darwin Schlägt Kant. Über die Schwächen der Menschlichen Vernunft und Ihre Fatalen Folgen; Orell Füssli Verlag: Zürich, Switzerland, 2020. [Google Scholar]
- Gladwell, M. Blink: The Power of Thinking without Thinking; Little, Brown and Co.: New York, NY, USA, 2005. [Google Scholar]
- Chamorro-Premuzic, T. Confidence: Overcoming Low Self-Esteem, Insecurity, and Self-Doubt; Hudson Street Press, a Member of Penguin Group (USA): New York, NY, USA, 2013. [Google Scholar]
- Svozil, K. Faithful orthogonal representations of graphs from partition logics. arXiv 2019, arXiv:1810.10423. [Google Scholar] [CrossRef]
- Dvurečenskij, A.; Pulmannová, S.; Svozil, K. Partition Logics, Orthoalgebras and Automata. Helv. Phys. Acta 1995, 68, 407–428. [Google Scholar] [CrossRef]
- Svozil, K. Logical equivalence between generalized urn models and finite automata. Int. J. Theor. Phys. 2005, 44, 745–754. [Google Scholar] [CrossRef]
- Svozil, K. Contexts in quantum, classical and partition logic. In Handbook of Quantum Logic and Quantum Structures; Engesser, K., Gabbay, D.M., Lehmann, D., Eds.; Elsevier: Amsterdam, The Netherlands, 2009; pp. 551–586. [Google Scholar] [CrossRef]
- Wright, R. Generalized urn models. Found. Phys. 1990, 20, 881–903. [Google Scholar] [CrossRef]
- Schaller, M.; Svozil, K. Partition logics of automata. Il Nuovo Cim. B 1994, 109, 167–176. [Google Scholar] [CrossRef]
- Svozil, K. Randomness & Undecidability in Physics; World Scientific: Singapore, 1993. [Google Scholar] [CrossRef]
- Schaller, M.; Svozil, K. Automaton partition logic versus quantum logic. Int. J. Theor. Phys. 1995, 34, 1741–1750. [Google Scholar] [CrossRef]
- Schaller, M.; Svozil, K. Automaton logic. Int. J. Theor. Phys. 1996, 35, 911–940. [Google Scholar] [CrossRef]
- Greechie, R.J. Orthomodular lattices admitting no states. J. Comb. Theory Ser. A 1971, 10, 119–132. [Google Scholar] [CrossRef]
- Kalmbach, G. Orthomodular Lattices; London Mathematical Society Monographs; Academic Press: London, UK, 1983; Volume 18. [Google Scholar]
- Svozil, K.; Tkadlec, J. Greechie diagrams, nonexistence of measures in quantum logics and Kochen—Specker type constructions. J. Math. Phys. 1996, 37, 5380–5401. [Google Scholar] [CrossRef]
- Gleason, A.M. Measures on the closed subspaces of a Hilbert space. J. Math. Mech. 1957, 6, 885–893. [Google Scholar] [CrossRef]
- Svozil, K. Kolmogorov-type conditional probabilities among distinct contexts. arXiv 2018, arXiv:1903.10424. [Google Scholar]
- Specker, E. Die Logik nicht gleichzeitig entscheidbarer Aussagen. Dialectica 1960, 14, 239–246. [Google Scholar] [CrossRef]
- Pták, P.; Pulmannová, S. Orthomodular Structures as Quantum Logics. Intrinsic Properties, State Space and Probabilistic Topics; Fundamental Theories of Physics; Kluwer Academic Publishers: Berlin, Germany, 1991; Volume 44. [Google Scholar]
- Abbott, A.A.; Calude, C.S.; Svozil, K. A quantum random number generator certified by value indefiniteness. Math. Struct. Comput. Sci. 2014, 24, e240303. [Google Scholar] [CrossRef]
- Abbott, A.A.; Calude, C.S.; Conder, J.; Svozil, K. Strong Kochen-Specker theorem and incomputability of quantum randomness. Phys. Rev. A 2012, 86, 062109. [Google Scholar] [CrossRef]
- Abbott, A.A.; Calude, C.S.; Svozil, K. Value-indefinite observables are almost everywhere. Phys. Rev. A 2014, 89, 032109. [Google Scholar] [CrossRef]
- Abbott, A.A.; Calude, C.S.; Svozil, K. A variant of the Kochen-Specker theorem localising value indefiniteness. J. Math. Phys. 2015, 56, 102201. [Google Scholar] [CrossRef]
- Yu, S.; Oh, C.H. State-Independent Proof of Kochen-Specker Theorem with 13 Rays. Phys. Rev. Lett. 2012, 108, 030402. [Google Scholar] [CrossRef]
- Boole, G. An Investigation of the Laws of Thought; Walton and Maberly: London, UK; MacMillan and Co.: New York, NY, USA; Cambridge University Press: Cambridge, UK, 2009. [Google Scholar] [CrossRef]
- Boole, G. On the Theory of Probabilities. Philos. Trans. R. Soc. Lond. 1862, 152, 225–252. [Google Scholar] [CrossRef]
- Fréchet, M. Généralisation du théorème des probabilités totales. Fundam. Math. 1935, 25, 379–387. [Google Scholar] [CrossRef]
- Hailperin, T. Best Possible Inequalities for the Probability of a Logical Function of Events. Am. Math. Mon. 1965, 72, 343–359. [Google Scholar] [CrossRef]
- Hailperin, T. Boole’s Logic and Probability: Critical Exposition from the Standpoint of Contemporary Algebra, Logic and Probability Theory, 2nd ed.; Studies in Logic and the Foundations of Mathematics; Elsevier Science Ltd.: Amsterdam, The Netherlands, 1986; Volume 85. [Google Scholar]
- Ursic, S. A Linear Characterization of NP-Complete Problems. In Proceedings of the 7th International Conference on Automated Deduction, Napa, CA, USA, 14–16 May 1984; Shostak, R.E., Ed.; Springer: New York, NY, USA, 1984; pp. 80–100. [Google Scholar] [CrossRef]
- Ursic, S. Generalizing Fuzzy Logic Probabilistic Inferences. In Proceedings of the Second Conference on Uncertainty in Artificial Intelligence, Philadelphia, PA, USA, 8–10 August 1986; AUAI Press: Arlington, WV, USA, 1986; pp. 303–310. [Google Scholar]
- Ursic, S. Generalizing Fuzzy Logic Probabilistic Inferences. In Uncertainty in Artificial Intelligence 2 (UAI1986); Lemmer, J.F., Kanal, L.N., Eds.; North Holland: Amsterdam, The Netherlands, 1988; pp. 337–362. [Google Scholar]
- Beltrametti, E.G.; Maçzyński, M.J. On a characterization of classical and nonclassical probabilities. J. Math. Phys. 1991, 32, 1280–1286. [Google Scholar] [CrossRef]
- Pykacz, J.; Santos, E. Hidden variables in quantum logic approach reexamined. J. Math. Phys. 1991, 32, 1287–1292. [Google Scholar] [CrossRef]
- Sylvia, P.; Majernik, V. Bell inequalities on quantum logics. J. Math. Phys. 1992, 33, 2173–2178. [Google Scholar] [CrossRef]
- Beltrametti, E.G.; Maçzyński, M.J. On the characterization of probabilities: A generalization of Bell’s inequalities. J. Math. Phys. 1993, 34, 4919–4929. [Google Scholar] [CrossRef]
- Beltrametti, E.G.; Maçzyński, M.J. On Bell-type inequalities. Found. Phys. 1994, 24, 1153–1159. [Google Scholar] [CrossRef]
- Dvurečenskij, A.; Länger, H. Bell-type inequalities in horizontal sums of Boolean algebras. Found. Phys. 1994, 24, 1195–1202. [Google Scholar] [CrossRef]
- Beltrametti, E.G.; Del Noce, C.; Maçzyński, M.J. Characterization and Deduction of Bell-Type Inequalities. In The Foundations of Quantum Mechanics—Historical Analysis and Open Questions: Lecce, 1993; Garola, C., Rossi, A., Eds.; Springer: Berlin, Germany, 1995; pp. 35–41. [Google Scholar] [CrossRef]
- Beltrametti, E.G.; Maçzyński, M.J. On the range of non-classical probability. Rep. Math. Phys. 1995, 36, 195–213. [Google Scholar] [CrossRef]
- Del Noce, C. An algorithm for finding Bell-type inequalities. Found. Phys. Lett. 1995, 8, 213–229. [Google Scholar] [CrossRef]
- Länger, H.; Maçzyński, M.J. On a characterization of probability measures on Boolean algebras and some orthomodular lattices. Math. Slovaca 1995, 45, 455–468. [Google Scholar]
- Dvurečenskij, A.; Länger, H. Bell-type inequalities in orthomodular lattices. I. Inequalities of order 2. Int. J. Theor. Phys. 1995, 34, 995–1024. [Google Scholar] [CrossRef]
- Dvurečenskij, A.; Länger, H. Bell-type inequalities in orthomodular lattices. II. Inequalities of higher order. Int. J. Theor. Phys. 1995, 34, 1025–1036. [Google Scholar] [CrossRef]
- Beltrametti, E.G.; Bugajski, S. The Bell phenomenon in classical frameworks. J. Phys. A Math. Gen. Phys. 1996, 29, 247–261. [Google Scholar] [CrossRef]
- Pulmannová, S. Hidden Variables and Bell Inequalities on Quantum Logics. Found. Phys. 2002, 32, 193–216. [Google Scholar] [CrossRef]
- Bell, J.S. On the Einstein Podolsky Rosen paradox. Phys. Phys. Fiz. 1964, 1, 195–200. [Google Scholar] [CrossRef]
- Artaud, A. Le théâtre et Son Double; Gallimard: Paris, France, 1938. [Google Scholar]
- Foulis, D.J.; Randall, C.H. Empirical Logic and Quantum Mechanics. In Logic and Probability in Quantum Mechanics; Suppes, P., Ed.; Springer: Berlin, Germany, 1976; pp. 73–103. [Google Scholar] [CrossRef]
- Einstein, A.; Podolsky, B.; Rosen, N. Can quantum-mechanical description of physical reality be considered complete? Phys. Rev. 1935, 47, 777–780. [Google Scholar] [CrossRef]
- Sakurai, J.J.; Napolitano, J.J. Modern Quantum Mechanics, 2nd ed.; Pearson Education: Boston, MA, USA, 2011. [Google Scholar]
- Pitowsky, I. From George Boole to John Bell: The origin of Bell’s inequality. In Bell’s Theorem, Quantum Theory and the Conceptions of the Universe; Kafatos, M., Ed.; Fundamental Theories of Physics; Kluwer Academic Publishers: Berlin, Germany, 1989; Volume 37, pp. 37–49. [Google Scholar] [CrossRef]
- Wigner, E.P. On Hidden Variables and Quantum Mechanical Probabilities. Am. J. Phys. 1970, 38, 1005–1009. [Google Scholar] [CrossRef]
- Fine, A. Hidden Variables, Joint Probability, and the Bell Inequalities. Phys. Rev. Lett. 1982, 48, 291–295. [Google Scholar] [CrossRef]
- Froissart, M. Constructive generalization of Bell’s inequalities. Il Nuovo Cim. B 1981, 64, 241–251. [Google Scholar] [CrossRef]
- Cirel’son, B.S. Some results and problems on quantum Bell-type inequalities. Hadron. J. Suppl. 1993, 8, 329–345. [Google Scholar]
- Bell, J.S. Introduction to the hidden-variable question. In Proceedings of the 49th International School of Physics “Enrico Fermi”. Course IL: Foundations of Quantum Mechanics, Varenna on Lake Como, Villa Monastero, Italy, 11–29 June 1970; d’Espagnat, B., di Fisica, S.I., Eds.; Academic Press: New York, NY, USA, 1971; Volume 49, pp. 171–181. [Google Scholar]
- Pitowsky, I. The range of quantum probabilities. J. Math. Phys. 1986, 27, 1556–1565. [Google Scholar] [CrossRef]
- Pitowsky, I. Quantum Probability—Quantum Logic; Lecture Notes in Physics; Springer: Berlin, Germany, 1989; Volume 321. [Google Scholar] [CrossRef]
- Pitowsky, I. Correlation polytopes their geometry and complexity. Math. Program. 1991, 50, 395–414. [Google Scholar] [CrossRef]
- Pitowsky, I. George Boole’s ‘Conditions of Possible Experience’ and the Quantum Puzzle. Br. J. Philos. Sci. 1994, 45, 95–125. [Google Scholar] [CrossRef]
- Pitowsky, I.; Svozil, K. New optimal tests of quantum nonlocality. Phys. Rev. A 2001, 64, 014102. [Google Scholar] [CrossRef]
- Garg, A.; Mermin, D.N. Farkas’s Lemma and the nature of reality: Statistical implications of quantum correlations. Found. Phys. 1984, 14, 1–39. [Google Scholar] [CrossRef]
- Bishop, E.; Leeuw, K.D. The representations of linear functionals by measures on sets of extreme points. Ann. Fourier Inst. 1959, 9, 305–331. [Google Scholar] [CrossRef]
- Vorob’ev, N.N. Consistent Families of Measures and Their Extensions. Theory Probab. Appl. 1962, 7, 147–163. [Google Scholar] [CrossRef]
- Kellerer, H.G. Verteilungsfunktionen mit gegebenen Marginalverteilungen. Z. Wahrscheinlichkeitstheorie Verwandte Geb. 1964, 3, 247–270. [Google Scholar] [CrossRef]
- Kellerer, H.G. Duality theorems for marginal problems. Z. Wahrscheinlichkeitstheorie Erwandte Geb. 1984, 67, 399–432. [Google Scholar] [CrossRef]
- Klyachko, A.A.; Can, M.A.; Binicioğlu, S.; Shumovsky, A.S. Simple Test for Hidden Variables in Spin-1 Systems. Phys. Rev. Lett. 2008, 101, 020403. [Google Scholar] [CrossRef]
- Klyachko, A.A. Coherent states, entanglement, and geometric invariant theory. arXiv 2002, arXiv:quant-ph/0206012. [Google Scholar]
- Svozil, K. On generalized probabilities: Correlation polytopes for automaton logic and generalized urn models, extensions of quantum mechanics and parameter cheats. arXiv 2001, arXiv:quant-ph/0012066. [Google Scholar]
- Svozil, K. Generalized event structures and probabilities. In Information and Complexity; Burgin, M., Calude, C.S., Eds.; World Scientific: Singapore, 2016; Volume 6, pp. 276–300. [Google Scholar] [CrossRef]
- Pykacz, J. On Bell-Type Inequalities in Quantum Logics. The Concept of Probability. In Proceedings of the Delphi Conference, Delphi, Greece, 21–23 October 1987; Bitsakis, E.I., Nicolaides, C.A., Eds.; Springer: Berlin, Germany, 1989; pp. 115–120. [Google Scholar] [CrossRef]
- Fukuda, K. Frequently Asked Questions in Polyhedral Computation. Available online: http://citeseerx.ist.psu.edu/viewdoc/download?doi=10.1.1.55.3786&rep=rep1&type=pdf (accessed on 29 July 2017).
- Ziegler, G.M. Lectures on Polytopes; Springer: New York, NY, USA, 1994. [Google Scholar]
- Henk, M.; Richter-Gebert, J.; Ziegler, G.M. Basic properties of convex polytopes. In Handbook of Discrete and Computational Geometry, 2nd ed.; Goodman, J.E., O’Rourke, J., Eds.; Chapman and Hall/CRC Press Company: Boca Raton, FL, USA, 2004; pp. 355–383. [Google Scholar] [CrossRef]
- Avis, D.; Bremner, D.; Seidel, R. How Good Are Convex Hull Algorithms? Comput. Geom. Theory Appl. 1997, 7, 265–301. [Google Scholar] [CrossRef]
- McMullen, P.; Shephard, G.C. Convex Polytopes and the Upper Bound Conjecture; London Mathematical Society Lecture Notes Series 3; Cambridge University Press: Cambridge, UK, 1971. [Google Scholar]
- Schrijver, A. Theory of Linear and Integer Programming; Wiley Series in Discrete Mathematics & Optimization; John Wiley & Sons: New York, NY, USA, 1998. [Google Scholar]
- Grünbaum, B. Convex Polytopes, 2nd ed.; Graduate Texts in Mathematics; Springer: New York, NY, USA, 2003; Volume 221. [Google Scholar] [CrossRef]
- Cabello, A. Experimentally Testable State-Independent Quantum Contextuality. Phys. Rev. Lett. 2008, 101, 210401. [Google Scholar] [CrossRef] [PubMed]
- Bell, J.S. On the Problem of Hidden Variables in Quantum Mechanics. Rev. Mod. Phys. 1966, 38, 447–452. [Google Scholar] [CrossRef]
- Clauser, J.F.; Horne, M.A.; Shimony, A.; Holt, R.A. Proposed Experiment to Test Local Hidden-Variable Theories. Phys. Rev. Lett. 1969, 23, 880–884. [Google Scholar] [CrossRef]
- Schimpf, M.; Svozil, K. A glance at singlet states and four-partite correlations. Math. Slovaca 2010, 60, 701–722. [Google Scholar] [CrossRef]
- Fukuda, K. Cdd and Cddplus Homepage, Cddlib Package Cddlib-094h. 2000–2017. Available online: http://www.inf.ethz.ch/personal/fukudak/cdd_home/ (accessed on 1 July 2017).
- Free Software Foundation. GMP, Arithmetic without Limitations, The GNU Multiple Precision Arithmetic Library gmp-6.1.2.tar.lz. 1991–2020. Available online: https://gmplib.org/ (accessed on 29 July 2017).
- Fukuda, K.; Prodon, A. Double description method revisited. In Proceedings of the Combinatorics and Computer Science: 8th Franco-Japanese and 4th Franco-Chinese Conference, Brest, France, 3–5 July 1995; Deza, M., Euler, R., Manoussakis, I., Eds.; Lecture Notes in Computer Science (LNCS). Springer: Berlin, Germany, 1996; Volume 1120, pp. 91–111. [Google Scholar] [CrossRef]
- Avis, D.; Fukuda, K.; Picozzi, S. On canonical representations of convex polyhedra. Mathematical Software. In Proceedings of the First International Congress of Mathematical Software, Beijing, China, 17–19 August 2002; Cohen, A.M., Gao, X.S., Takayama, N., Eds.; World Scientific: Singapore, 2002; pp. 350–360. [Google Scholar] [CrossRef]
- Svozil, K. Physical Causality. Determinism, Randomness and Uncaused Events; Springer: Berlin, Germany, 2018. [Google Scholar] [CrossRef]
- Sliwa, C. Symmetries of the Bell correlation inequalities. Phys. Lett. A 2003, 317, 165–168. [Google Scholar] [CrossRef]
- Colins, D.; Gisin, N. A relevant two qbit Bell inequality inequivalent to the CHSH inequality. J. Phys. A Math. Gen. 2004, 37, 1775–1787. [Google Scholar] [CrossRef]
- Werner, R.F.; Wolf, M.M. All-multipartite Bell-correlation inequalities for two dichotomic observables per site. Phys. Rev. A 2001, 64, 032112. [Google Scholar] [CrossRef]
- Żukowski, M.; Brukner, V. Bell’s Theorem for General N-Qubit States. Phys. Rev. Lett. 2002, 88, 210401. [Google Scholar] [CrossRef]
- Pitowsky, I. Most Bell Operators do not Significantly Violate Locality. arXiv 2002, arXiv:quant-ph/0202053. [Google Scholar]
- Schachner, G. The structure of Bell inequalities. arXiv 2003, arXiv:quant-ph/0312117. [Google Scholar]
- Bohr, N. Discussion with Einstein on epistemological problems in atomic physics. In Albert Einstein: Philosopher-Scientist; Schilpp, P.A., Ed.; The Library of Living Philosophers; Open Court: Evanston, IL, USA, 1949; pp. 200–241. [Google Scholar] [CrossRef]
- Navara, M.; Rogalewicz, V. The pasting constructions for orthomodular posets. Math. Nachr. 1991, 154, 157–168. [Google Scholar] [CrossRef]
- Tutte, W.T. A Short Proof of the Factor Theorem for Finite Graphs. Can. J. Math. 1954, 6, 347–352. [Google Scholar] [CrossRef]
- Szabó, J. Good characterizations for some degree constrained subgraphs. J. Comb. Theory Ser. B 2009, 99, 436–446. [Google Scholar] [CrossRef]
- Ramanathan, R.; Rosicka, M.; Horodecki, K.; Pironio, S.; Horodecki, M.; Horodecki, P. Gadget structures in proofs of the Kochen-Specker theorem. arXiv 2018, arXiv:1807.00113. [Google Scholar]
- Pitowsky, I. Infinite and finite Gleason’s theorems and the logic of indeterminacy. J. Math. Phys. 1998, 39, 218–228. [Google Scholar] [CrossRef]
- Svozil, K. New Forms of Quantum Value Indefiniteness Suggest That Incompatible Views on Contexts Are Epistemic. Entropy 2018, 20, 406. [Google Scholar] [CrossRef]
- Kochen, S.; Specker, E.P. The Problem of Hidden Variables in Quantum Mechanics. J. Math. Mech. 1967, 17, 59–87. [Google Scholar] [CrossRef]
- Cohen, D.W. An Introduction to Hilbert Space and Quantum Logic; Problem Books in Mathematics; Springer: New York, NY, USA, 1989. [Google Scholar] [CrossRef]
- Lovász, L. On the Shannon capacity of a graph. IEEE Trans. Inf. Theory 1979, 25, 1–7. [Google Scholar] [CrossRef]
- Lovász, L.; Saks, M.; Schrijver, A. Orthogonal representations and connectivity of graphs. Linear Algebra Appl. 1989, 114-115, 439–454. [Google Scholar] [CrossRef]
- Solís-Encina, A.; Portillo, J.R. Orthogonal Representation of Graphs. arXiv 2015, arXiv:1504.03662. [Google Scholar]
- Beran, L. Orthomodular Lattices. Algebraic Approach; Reidel, D., Ed.; Mathematics and its Applications; Springer: Berlin, Germany, 1985; Volume 18. [Google Scholar] [CrossRef]
- Birkhoff, G. Applications of lattice algebra. Math. Proc. Camb. Philos. Soc. 1934, 30, 115–122. [Google Scholar] [CrossRef]
- Wright, R. The state of the pentagon. A nonclassical example. In Mathematical Foundations of Quantum Theory; Marlow, A.R., Ed.; Academic Press: New York, NY, USA, 1978; pp. 255–274. [Google Scholar]
- Bub, J.; Stairs, A. Contextuality and Nonlocality in ‘No Signaling’ Theories. Found. Phys. 2009, 39, 690–711. [Google Scholar] [CrossRef]
- Bub, J.; Stairs, A. Contextuality in Quantum Mechanics: Testing the Klyachko Inequality. arXiv 2010, arXiv:1006.0500. [Google Scholar]
- Badzia̧g, P.; Bengtsson, I.; Cabello, A.; Granström, H.; Larsson, J.A. Pentagrams and Paradoxes. Found. Phys. 2011, 41, 414–423. [Google Scholar] [CrossRef]
- Specker, E. Algebra of Quantum Observables and Related Constructive Mathematical Methods; Seminar; Vienna University of Technology: Vienna, Austria, 1996. [Google Scholar]
- Kochen, S.; Specker, E.P. Logical Structures arising in quantum theory. The Theory of Models. In Proceedings of the 1963 International Symposium at Berkeley, Berkeley, CA, USA, 11–23 June 1963; North Holland: Amsterdam, The Netherlands, 1965; pp. 177–189. [Google Scholar]
- Pitowsky, I. Betting on the outcomes of measurements: A Bayesian theory of quantum probability. Stud. Hist. Philos. Sci. Part B 2003, 34, 395–414. [Google Scholar] [CrossRef]
- Pitowsky, I. Quantum Mechanics as a Theory of Probability. In Physical Theory and its Interpretation; Demopoulos, W., Pitowsky, I., Eds.; The Western Ontario Series in Philosophy of Science; Springer: Berlin, Germany, 2006; Volume 72, pp. 213–240. [Google Scholar] [CrossRef]
- Greechie, R.J. Some results from the combinatorial approach to quantum logic. Synthese 1974, 29, 113–127. [Google Scholar] [CrossRef]
- Greechie, R.J. Some results from the combinatorial approach to quantum logic. In Logic and Probability in Quantum Mechanics; Suppes, P., Ed.; Springer: Dordrecht, The Netherlands, 1976; pp. 105–119. [Google Scholar] [CrossRef]
- Redhead, M. Incompleteness, Nonlocality, and Realism: A Prolegomenon to the Philosophy of Quantum Mechanics; Clarendon Press: Oxford, UK, 1990. [Google Scholar]
- Belinfante, F.J. A Survey of Hidden-Variables Theories; International Series of Monographs in Natural Philosophy 55; Pergamon Press: Oxford, UK, 1973. [Google Scholar] [CrossRef]
- Stairs, A. Quantum logic, realism, and value definiteness. Philos. Sci. 1983, 50, 578–602. [Google Scholar] [CrossRef]
- Clifton, R.K. Getting contextual and nonlocal elements-of-reality the easy way. Am. J. Phys. 1993, 61, 443–447. [Google Scholar] [CrossRef]
- Tkadlec, J. Greechie diagrams of small quantum logics with small state spaces. Int. J. Theor. Phys. 1998, 37, 203–209. [Google Scholar] [CrossRef]
- Svozil, K. Quantum Scholasticism: On Quantum Contexts, Counterfactuals, and the Absurdities of Quantum Omniscience. Inf. Sci. 2009, 179, 535–541. [Google Scholar] [CrossRef]
- Cabello, A.; Portillo, J.R.; Solís, A.; Svozil, K. Minimal true-implies-false and true-implies-true sets of propositions in noncontextual hidden-variable theories. Phys. Rev. A 2018, 98, 012106. [Google Scholar] [CrossRef]
- Cabello, A. A simple proof of the Kochen-Specker theorem. Eur. J. Phys. 1994, 15, 179–183. [Google Scholar] [CrossRef]
- Cabello, A. Pruebas Algebraicas de Imposibilidad de Variables Ocultas en Mecánica Cuántica. Ph.D. Thesis, Universidad Complutense de Madrid, Madrid, Spain, 1996. [Google Scholar]
- Svozil, K. Classical Predictions for Intertwined Quantum Observables Are Contingent and Thus Inconclusive. Quantum Rep. 2020, 2, 18. [Google Scholar] [CrossRef]
- Pitowsky, I. Substitution and Truth in Quantum Logic. Philos. Sci. 1982, 49, 380–401. [Google Scholar] [CrossRef]
- Johansen, H.B. Comment on Getting contextual and nonlocal elements-of-reality the easy way. Am. J. Phys. 1994, 62, 471. [Google Scholar] [CrossRef]
- Vermaas, P.E. Comment on Getting contextual and nonlocal elements-of-reality the easy way. Am. J. Phys. 1994, 62, 658. [Google Scholar] [CrossRef]
- Cabello, A.; García-Alcaine, G. Bell-Kochen-Specker theorem for any finite dimension n≥3. J. Phys. A Math. Gen. Phys. 1996, 29, 1025–1036. [Google Scholar] [CrossRef]
- Godsil, C.D.; Zaks, J. Coloring the Sphere; University of Waterloo Research Report CORR 88-12; University of Waterloo: Waterloo, ON, Canada; Available online: https://arxiv.org/abs/1201.0486 (accessed on 15 May 2020).
- Havlicek, H.; Krenn, G.; Summhammer, J.; Svozil, K. Colouring the rational quantum sphere and the Kochen-Specker theorem. J. Phys. A Math. Gen. 2001, 34, 3071–3077. [Google Scholar] [CrossRef]
- Kalmbach, G. Measures and Hilbert Lattices; World Scientific: Singapore, 1986. [Google Scholar]
- Schütte, K. Letter to Ernst Specker, Kiel, dated 22 April 1965. A second letter to Ernst Specker from Kurt Schütte in Munich, dated 30 November 1983, hints on how Schütte had obtained this configuration: “Zugrunde liegt offenbar ein Schließungssatz einer vollelliptischen Ebene in einer speziellen Form, in der zwischen den Punkten und Geraden der Konfiguratton möglichst viel Polaritäten auftreten, um die Berechenbarkeit der Formel sicherzustellen und mit möglichst wenig Variablen auszukommen”.
- Clavadetscher-Seeberger, E. Eine Partielle Prädikatenlogik. Ph.D. Thesis, ETH-Zürich, Zürich, Switzerland, 1983. [Google Scholar]
- Hardy, L. Quantum mechanics, local realistic theories, and Lorentz-invariant realistic theories. Phys. Rev. Lett. 1992, 68, 2981–2984. [Google Scholar] [CrossRef]
- Hardy, L. Nonlocality for two particles without inequalities for almost all entangled states. Phys. Rev. Lett. 1993, 71, 1665–1668. [Google Scholar] [CrossRef] [PubMed]
- Boschi, D.; Branca, S.; De Martini, F.; Hardy, L. Ladder Proof of Nonlocality without Inequalities: Theoretical and Experimental Results. Phys. Rev. Lett. 1997, 79, 2755–2758. [Google Scholar] [CrossRef]
- Cabello, A.; García-Alcaine, G. A hidden-variables versus quantum mechanics experiment. J. Phys. A Math. Gen. Phys. 1995, 28, 3719–3724. [Google Scholar] [CrossRef]
- Cabello, A.; Estebaranz, J.M.; García-Alcaine, G. Bell-Kochen-Specker theorem: A proof with 18 vectors. Phys. Lett. A 1996, 212, 183–187. [Google Scholar] [CrossRef]
- Cabello, A. No-hidden-variables proof for two spin- particles preselected and postselected in unentangled states. Phys. Rev. A 1997, 55, 4109–4111. [Google Scholar] [CrossRef]
- Chen, J.L.; Cabello, A.; Xu, Z.P.; Su, H.Y.; Wu, C.; Kwek, L.C. Hardy’s paradox for high-dimensional systems. Phys. Rev. A 2013, 88, 062116. [Google Scholar] [CrossRef]
- Cabello, A.; Badziag, P.; Terra Cunha, M.; Bourennane, M. Simple Hardy-Like Proof of Quantum Contextuality. Phys. Rev. Lett. 2013, 111, 180404. [Google Scholar] [CrossRef]
- Bridgman, P.W. A Physicist’s Second Reaction to Mengenlehre. Scr. Math. 1934, 2, 101–117, 224–234. [Google Scholar]
- Tkadlec, J. Representations of Orthomodular Structures: Nanjing. In Ordered Algebraic Structures; Holland, C.W., Ed.; Algebra, Logic and Applications; Gordon and Breach Science Publishers: Amsterdam, The Netherlands, 2001; Volume 16, pp. 153–158. [Google Scholar]
- Peres, A. Two simple proofs of the Kochen-Specker theorem. J. Phys. A Math. Gen. 1991, 24, L175–L178. [Google Scholar] [CrossRef]
- Zimba, J.; Penrose, R. On Bell non-locality without probabilities: More curious geometry. Stud. Hist. Philos. Mod. Phys. 1993, 24, 697–720. [Google Scholar] [CrossRef]
- Peres, A. Generalized Kochen-Specker theorem. Found. Phys. 1996, 26, 807–812. [Google Scholar] [CrossRef][Green Version]
- Kernaghan, M. Bell-Kochen-Specker theorem for 20 vectors. J. Phys. A Math. Gen. 1994, 27, L829. [Google Scholar] [CrossRef]
- Mermin, D.N. Hidden variables and the two theorems of John Bell. Rev. Mod. Phys. 1993, 65, 803–815. [Google Scholar] [CrossRef]
- Bub, J. Schütte’s tautology and the Kochen–Specker theorem. Found. Phys. 1996, 26, 787–806. [Google Scholar] [CrossRef]
- Cabello, A.; Estebaranz, J.M.; García-Alcaine, G. New variants of the Bell-Kochen-Specker theorem. Phys. Lett. A 1996, 218, 115–118. [Google Scholar] [CrossRef][Green Version]
- Calude, C.S.; Hertling, P.H.; Svozil, K. Kochen-Specker Theorem: Two Geometrical Proofs. Tatra Mt. Math. Publ. 1998, 15, 133–142. [Google Scholar]
- Tkadlec, J. Diagrams of Kochen-Specker type constructions. Int. J. Theor. Phys. 2000, 39, 921–926. [Google Scholar] [CrossRef]
- Pavičić, M.; Merlet, J.P.; Megill, N. Exhaustive Enumeration of Kochen-Specker Vector Systems; Research Report RR-5388, INRIA, Report nr. RR-5388; French Institute for Research in Computer Science and Automation: Rocquencourt, France, 2004; Available online: https://hal.inria.fr/inria-00070615 (accessed on 15 May 2020).
- Smith, D. Orthomodular Bell-Kochen-Specker Theorem. Int. J. Theor. Phys. 2004, 43, 2023–2027. [Google Scholar] [CrossRef]
- Pavičić, M.; Merlet, J.P.; McKay, B.; Megill, N.D. Kochen-Specker vectors. J. Phys. A Math. Gen. 2005, 38, 1577–1592. [Google Scholar] [CrossRef]
- Arends, F.; Ouaknine, J.; Wampler, C.W. On Searching for Small Kochen-Specker Vector Systems. In Proceedings of the Graph-Theoretic Concepts in Computer Science: 37th International Workshop, WG 2011, Teplá Monastery, Czech Republic, 21–24 June 2011; Kolman, P., Kratochvíl, J., Eds.; Revised Papers. Springer: Berlin, Germany, 2011; pp. 23–34. [Google Scholar] [CrossRef]
- Waegell, M.; Aravind, P.K.; Megill, N.D.; Pavicic, M. Parity Proofs of the Bell-Kochen-Specker Theorem Based on the 600-cell. Found. Phys. 2011, 41, 883–904. [Google Scholar] [CrossRef]
- Waegell, M.; Aravind, P.K. Parity proofs of the Kochen-Specker theorem based on 60 complex rays in four dimensions. J. Phys. A Math. Theor. 2011, 44, 505303. [Google Scholar] [CrossRef]
- Planat, M. On small proofs of the Bell-Kochen-Specker theorem for two, three and four qubits. Eur. Phys. J. Plus 2012, 127, 1–11. [Google Scholar] [CrossRef]
- Lisonek, P.; Badziag, P.; Portillo, J.R.; Cabello, A. Kochen-Specker set with seven contexts. Phys. Rev. A 2014, 89, 042101. [Google Scholar] [CrossRef]
- Pavičić, M.; Waegell, M.; Megill, N.D.; Aravind, P.K. Automated generation of Kochen-Specker sets. Sci. Rep. 2019, 9. [Google Scholar] [CrossRef] [PubMed]
- Pavičić, M. Hypergraph Contextuality. Entropy 2019, 21, 1107. [Google Scholar] [CrossRef]
- Uijlen, S.; Westerbaan, B. A Kochen-Specker System Has at Least 22 Vectors. New Gener. Comput. 2016, 34, 3–23. [Google Scholar] [CrossRef]
- Xu, Z.P.; Chen, J.L.; Gühne, O. Proof of the Peres conjecture for contextuality. arXiv 2020, arXiv:2001.07656. [Google Scholar]
- Cabello, A. Kochen-Specker theorem and experimental test on hidden variables. Int. J. Mod. Phys. A 2000, 15, 2813–2820. [Google Scholar] [CrossRef]
- Godsil, C.D. Coloring Spheres; Talk and Private Communication; Oxford University Press: Oxford, UK, 2016. [Google Scholar]
- Cabello, A. Why you should care about quantum contextuality: Lessons from the contextuality wars, 2017. In Proceedings of the Conference on “Foundations of Quantum Mechanics and Technology (FQMT)”, Växjö, Sweden, 12–15 June 2017. [Google Scholar]
- Meyer, D.A. Finite precision measurement nullifies the Kochen-Specker theorem. Phys. Rev. Lett. 1999, 83, 3751–3754. [Google Scholar] [CrossRef]
- Kent, A. Noncontextual Hidden Variables and Physical Measurements. Phys. Rev. Lett. 1999, 83, 3755–3757. [Google Scholar] [CrossRef]
- Clifton, R.; Kent, A. Simulating quantum mechanics by Non-contextual hidden variables. Proc. R. Soc. London A Math. Phys. Eng. Sci. 2000, 456, 210–2114. [Google Scholar] [CrossRef]
- Mermin, D.N. A Kochen-Specker Theorem for Imprecisely Specified Measurement. arXiv 1999, arXiv:quant-ph/9912081. [Google Scholar]
- Breuer, T. Kochen-Specker Theorem for Finite Precision Spin-One Measurements. Phys. Rev. Lett. 2002, 88, 240402. [Google Scholar] [CrossRef] [PubMed]
- Breuer, T. Another No-Go Theorem for Hidden Variable Models of Inaccurate Spin 1 Measurements. Philos. Sci. 2003, 70, 1368–1379. [Google Scholar] [CrossRef]
- Barrett, J.; Kent, A. Non-contextuality, finite precision measurement and the Kochen-Specker theorem. Stud. Hist. Philos. Sci. Part B 2004, 35, 151–176. [Google Scholar] [CrossRef]
- Peres, A. Finite precision measurement nullifies Euclid’s postulates. arXiv 2003, arXiv:quant-ph/0310035. [Google Scholar]
- Hrushovski, E.; Pitowsky, I. Generalizations of Kochen and Specker’s theorem and the effectiveness of Gleason’s theorem. Stud. Hist. Philos. Sci. Part B 2004, 35, 177–194. [Google Scholar] [CrossRef]
- Svozil, K. Unscrambling the Quantum Omelette. Int. J. Theor. Phys. 2014, 53, 3648–3657. [Google Scholar] [CrossRef]
- Hasegawa, Y.; Loidl, R.; Badurek, G.; Baron, M.; Rauch, H. Violation of a Bell-like inequality in single-neutron interferometry. Nature 2003, 425, 45–48. [Google Scholar] [CrossRef]
- Hasegawa, Y.; Loidl, R.; Badurek, G.; Baron, M.; Rauch, H. Quantum Contextuality in a Single-Neutron Optical Experiment. Phys. Rev. Lett. 2006, 97, 230401. [Google Scholar] [CrossRef]
- Cabello, A.; Filipp, S.; Rauch, H.; Hasegawa, Y. Proposed Experiment for Testing Quantum Contextuality with Neutrons. Phys. Rev. Lett. 2008, 100, 130404. [Google Scholar] [CrossRef] [PubMed]
- Bartosik, H.; Klepp, J.; Schmitzer, C.; Sponar, S.; Cabello, A.; Rauch, H.; Hasegawa, Y. Experimental Test of Quantum Contextuality in Neutron Interferometry. Phys. Rev. Lett. 2009, 103, 040403. [Google Scholar] [CrossRef] [PubMed]
- Kirchmair, G.; Zähringer, F.; Gerritsma, R.; Kleinmann, M.; Gühne, O.; Cabello, A.; Blatt, R.; Roos, C.F. State-independent experimental test of quantum contextuality. Nature 2009, 460, 494–497. [Google Scholar] [CrossRef]
- Greenberger, D.M.; Horne, M.A.; Zeilinger, A. Going beyond Bell’s theorem. In Bell’s Theorem, Quantum Theory, and Conceptions of the Universe; Kafatos, M., Ed.; Fundamental Theories of Physics; Kluwer Academic Publishers: Berlin, Germany, 1989; Volume 37, pp. 69–72. [Google Scholar] [CrossRef]
- Simon, C.; Zukowski, M.; Weinfurter, H.; Zeilinger, A. Feasible “Kochen-Specker” Experiment with Single Particles. Phys. Rev. Lett. 2000, 85, 1783–1786. [Google Scholar] [CrossRef] [PubMed]
- Pan, J.W.; Bouwmeester, D.; Daniell, M.; Weinfurter, H.; Zeilinger, A. Experimental test of quantum nonlocality in three-photon Greenberger-Horne-Zeilinger entanglement. Nature 2000, 403, 515–519. [Google Scholar] [CrossRef] [PubMed]
- Bengtsson, I.; Blanchfield, K.; Cabello, A. A Kochen-Specker inequality from a SIC. Phys. Lett. A 2012, 376, 374–376. [Google Scholar] [CrossRef]
- Araújo, M.; Quintino, M.T.; Budroni, C.; Cunha, M.T.; Cabello, A. All noncontextuality inequalities for the n-cycle scenario. Phys. Rev. A 2013, 88, 022118. [Google Scholar] [CrossRef]
- Dirac, P.A.M. The Physical Interpretation of the Quantum Dynamics. Proc. R. Soc. London A Math. Phys. Eng. Sci. 1927, 113, 621–641. [Google Scholar] [CrossRef]
- Dirac, P.A.M. The Principles of Quantum Mechanics, 4th ed.; Oxford University Press: Oxford, UK, 1958. [Google Scholar]
- Jordan, P. Über eine neue Begründung der Quantenmechanik. Z. Phys. 1927, 40, 809–838. [Google Scholar] [CrossRef]
- Von Neumann, J. Wahrscheinlichkeitstheoretischer Aufbau der Quantenmechanik. (German) [Probabilistic structure of quantum mechanics]. Nachr. Ges. Wiss. Gott. Math. Phys. Kl. 1927, 1, 245–272. [Google Scholar]
- Von Neumann, J. Mathematische Grundlagen der Quantenmechanik, 2nd ed.; Springer: Berlin, Germany, 1996. [Google Scholar] [CrossRef]
- Von Neumann, J. Mathematical Foundations of Quantum Mechanics; Princeton University Press: Princeton, NJ, USA, 1955. [Google Scholar]
- Born, M. Zur Quantenmechanik der Stoßvorgänge. Z. Phys. 1926, 37, 863–867. [Google Scholar] [CrossRef]
- Dirac, P.A.M. The Futility of War. In The World-Wide Implications of a Nuclear War. 1st Session; Etim, E., Stipcich, S., Eds.; The Science and Culture Series–Nuclear Strategy and Peace Technology; World Scientific: Singapore, 1992; Volume 1, pp. 173–175. [Google Scholar] [CrossRef]
- Lüders, G. Über die Zustandsänderung durch den Meßprozeß. Ann. Phys. 1950, 443, 322–328. [Google Scholar] [CrossRef]
- Lüders, G. Concerning the state-change due to the measurement process. Ann. Phys. 2006, 15, 663–670. [Google Scholar] [CrossRef][Green Version]
- Busch, P.; Lahti, P. Lüders Rule. In Compendium of Quantum Physics; Greenberger, D., Hentschel, K., Weinert, F., Eds.; Springer: Berlin, Germany, 2009; pp. 356–358. [Google Scholar] [CrossRef]
- Hájek, A. Interpretations of Probability. In The Stanford Encyclopedia of Philosophy, Winter 2012 ed.; Zalta, E.N., Ed.; Metaphysics Research Lab, Stanford University: Stanford, CA, USA, 2012. [Google Scholar]
- Zierler, N.; Schlessinger, M. Boolean embeddings of orthomodular sets and quantum logic. Duke Math. J. 1965, 32, 251–262. [Google Scholar] [CrossRef]
- Kamber, F. Zweiwertige Wahrscheinlichkeitsfunktionen auf orthokomplementären Verbänden. Math. Ann. 1965, 158, 158–196. [Google Scholar] [CrossRef]
- Cooke, R.; Keane, M.; Moran, W. An elementary proof of Gleason’s theorem. Math. Proc. Camb. Philos. Soc. 1985, 98, 117–128. [Google Scholar] [CrossRef]
- Dvurečenskij, A. Gleason’s Theorem and Its Applications; Mathematics and its Applications; Kluwer Academic Publishers: Berlin, Germany, 1993; Volume 60. [Google Scholar] [CrossRef]
- Richman, F.; Bridges, D. A constructive proof of Gleason’s theorem. J. Funct. Anal. 1999, 162, 287–312. [Google Scholar] [CrossRef]
- Grötschel, M.; Lovász, L.; Schrijver, A. Relaxations of Vertex Packing. J. Comb. Theory Ser. B 1986, 40, 330–343. [Google Scholar] [CrossRef]
- Grötschel, M.; Lovász, L.; Schrijver, A. Geometric Algorithms and Combinatorial Optimization, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 1993; Volume 2. [Google Scholar] [CrossRef]
- Knuth, D.E. The Sandwich Theorem. Electron. J. Comb. 1994, 1. [Google Scholar] [CrossRef]
- Cabello, A.; Severini, S.; Winter, A. Graph-Theoretic Approach to Quantum Correlations. Phys. Rev. Lett. 2014, 112, 040401. [Google Scholar] [CrossRef]
- Mackey, G.W. Quantum Mechanics and Hilbert Space. Am. Math. Mon. 1957, 64, 45–57. [Google Scholar] [CrossRef]
- Alda, V. On 0-1 measures for projectors I. Apl. Mat. 1980, 25, 373–374. [Google Scholar]
- Alda, V. On 0-1 measures for projectors II. Apl. Mat. 1981, 26, 57–58. [Google Scholar]
- Svozil, K. Physical (A)Causality; Fundamental Theories of Physics; Springer: Berlin, Germany, 2018; Volume 192. [Google Scholar] [CrossRef]
- Peres, A. Unperformed experiments have no results. Am. J. Phys. 1978, 46, 745–747. [Google Scholar] [CrossRef]
- Krenn, G.; Svozil, K. Stronger-than-quantum correlations. Found. Phys. 1998, 28, 971–984. [Google Scholar] [CrossRef][Green Version]
- Popescu, S.; Rohrlich, D. Action and passion at a distance. In Potentiality, Entanglement and Passion-at-a-Distance: Quantum Mechanical Studies for Abner Shimony, Volume Two (Boston Studies in the Philosophy of Science); Cohen, R.S., Horne, M., Stachel, J., Eds.; Kluwer Academic Publishers: Berlin, Germany, 1997; pp. 197–206. [Google Scholar] [CrossRef]
- Popescu, S. Nonlocality beyond quantum mechanics. Nat. Phys. 2014, 10, 264–270. [Google Scholar] [CrossRef]
- Pironio, S.; Acín, A.; Massar, S.; de la Giroday, A.B.; Matsukevich, D.N.; Maunz, P.; Olmschenk, S.; Hayes, D.; Luo, L.; Manning, T.A.; et al. Random numbers certified by Bell’s theorem. Nature 2010, 464, 1021–1024. [Google Scholar] [CrossRef]
- Um, M.; Zhang, X.; Zhang, J.; Wang, Y.; Yangchao, S.; Deng, D.L.; Duan, L.M.; Kim, K. Experimental Certification of Random Numbers via Quantum Contextuality. Sci. Rep. 2013, 3, 1–7. [Google Scholar] [CrossRef]
- Specker, E. Der Satz vom Maximum in der rekursiven Analysis. In Constructivity in Mathematics: Proceedings of the Colloquium Held at Amsterdam, 1957; Heyting, A., Ed.; North-Holland Publishing Company: Amsterdam, The Netherlands, 1959; pp. 254–265. [Google Scholar] [CrossRef]
- Filipp, S.; Svozil, K. Generalizing Tsirelson’s Bound on Bell Inequalities Using a Min-Max Principle. Phys. Rev. Lett. 2004, 93, 130407. [Google Scholar] [CrossRef]
- Pitowsky, I. Range Theorems for Quantum Probability and Entanglement. In Quantum Theory: Reconsideration of Foundations, Proceeding of the 2001 Vaxjo Conference; Khrennikov, A., Ed.; World Scientific: Singapore, 2002; pp. 299–308. [Google Scholar]
- Pitowsky, I. Geometry of quantum correlations. Phys. Rev. A 2008, 77, 062109. [Google Scholar] [CrossRef]
- Cirel’son, B.S. Quantum generalizations of Bell’s inequality. Lett. Math. Phys. 1980, 4, 93–100. [Google Scholar] [CrossRef]
- Tsirel’son, B.S. Quantum analogues of the Bell inequalities. The case of two spatially separated domains. J. Sov. Math. 1987, 36, 557–570. [Google Scholar] [CrossRef]
- Filipp, S.; Svozil, K. Testing the bounds on quantum probabilities. Phys. Rev. A 2004, 69, 032101. [Google Scholar] [CrossRef]
- Filipp, S.; Svozil, K. Tracing the bounds on Bell-type inequalities. In Proceedings of the Foundations of Probability and Physics-3, Växjö, Sweden, 7–12 June 2004; Khrennikov, A., Ed.; American Institute of Physics: Melville, NY, USA, 2005; Volume 750, pp. 87–94. [Google Scholar] [CrossRef]
- Reed, M.; Simon, B. Methods of Modern Mathematical Physics IV: Analysis of Operators; Methods of Modern Mathematical Physics Volume; Academic Press: New York, NY, USA, 1978; Volume 4. [Google Scholar]
- Teschl, G. Mathematical Methods in Quantum Mechanics with Applications to Schrödinger Operators; Graduate Studies in Mathematics; American Mathematical Society: Providence, RI, USA, 2009; Volume 99. [Google Scholar]
- Tkadlec, J.; (IST, Klosterneuburg, Austria). Personal Communication, 1995.
- Freud, S. Ratschläge für den Arzt bei der psychoanalytischen Behandlung. In Gesammelte Werke. Chronologisch geordnet. Achter Band. Werke aus den Jahren 1909–1913; Freud, A., Bibring, E., Hoffer, W., Kris, E., Isakower, O., Eds.; Fischer: Frankfurt am Main, Germany, 1999; pp. 376–387. [Google Scholar]
- Freud, S. Recommendations to Physicians Practising Psycho-Analysis. In The Standard Edition of the Complete Psychological Works of Sigmund Freud, Volume XII (1911–1913): The Case of Schreber, Papers on Technique and Other Works; Freud, A.F.A., Strachey, A., Tyson, A., Eds.; The Hogarth Press; The Institute of Psycho-Analysis: London, UK, 1958; pp. 109–120. [Google Scholar]
- Zeilinger, A. The message of the quantum. Nature 2005, 438, 743. [Google Scholar] [CrossRef] [PubMed]
- Specker, E. Subject Header Re: pla, 2000. Personal Mail Message to Karl Svozil, 20 October 2000.
- Trimmer, J.D. The Present Situation in Quantum Mechanics: A Translation of Schrödinger’s “Cat Paradox” Paper. Proc. Am. Philos. Soc. 1980, 124, 323–338. [Google Scholar]
- Svozil, K. Roots and (Re)sources of Value (In)definiteness Versus Contextuality. In Quantum, Probability, Logic: The Work and Influence of Itamar Pitowsky; Hemmo, M., Shenker, O., Eds.; Jerusalem Studies in Philosophy and History of Science (JSPS); Springer International Publishing: Berlin, Germany, 2020; Volume 1, pp. 521–544. [Google Scholar] [CrossRef]
- Braunstein, S.L.; Kimble, H.J. A posteriori teleportation. Nature 1998, 394, 840–841. [Google Scholar] [CrossRef][Green Version]
- Bouwmeester, D.; Pan, J.W.; Daniell, M.; Weinfurter, H.; Zukowski, M.; Zeilinger, A. Reply: A posteriori teleportation. Nature 1998, 394, 841. [Google Scholar] [CrossRef]
- Bouwmeester, D.; Pan, J.W.; Mattle, K.; Eibl, M.; Weinfurter, H.; Zeilinger, A. Experimental quantum teleportation. Nature 1997, 390, 575–579. [Google Scholar] [CrossRef]
- Svozil, K. Extrinsic-intrinsic concept and complementarity. In Inside versus Outside; Atmanspacher, H., Dalenoort, G.J., Eds.; Springer Series in Synergetics; Springer: Berlin Germany, 1994; Volume 63, pp. 273–288. [Google Scholar] [CrossRef]
- Toffoli, T. The Role of the Observer in Uniform Systems. In Applied General Systems Research: Recent Developments and Trends; Klir, G.J., Ed.; Plenum Press: New York, NY, USA; Springer: Boston, MA, USA, 1978; pp. 395–400. [Google Scholar] [CrossRef]




| # | ||||||||
|---|---|---|---|---|---|---|---|---|
| 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| 0 | 0 | 0 | 1 | 0 | 0 | 0 | 0 | |
| 0 | 0 | 1 | 0 | 0 | 0 | 0 | 0 | |
| 0 | 0 | 1 | 1 | 0 | 0 | 0 | 0 | |
| 0 | 1 | 0 | 0 | 0 | 0 | 0 | 0 | |
| 0 | 1 | 0 | 1 | 0 | 0 | 0 | 1 | |
| 0 | 1 | 1 | 0 | 0 | 0 | 1 | 0 | |
| 0 | 1 | 1 | 1 | 0 | 0 | 1 | 1 | |
| 1 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| 1 | 0 | 0 | 1 | 0 | 1 | 0 | 0 | |
| 1 | 0 | 1 | 0 | 1 | 0 | 0 | 0 | |
| 1 | 0 | 1 | 1 | 1 | 1 | 0 | 0 | |
| 1 | 1 | 0 | 0 | 0 | 0 | 0 | 0 | |
| 1 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | |
| 1 | 1 | 1 | 0 | 1 | 0 | 1 | 0 | |
| 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| # | |||||
|---|---|---|---|---|---|
| 0 | 0 | 0 | 0 | 1 | |
| 0 | 1 | 0 | 1 | 0 | |
| 0 | 1 | 1 | 0 | 0 | |
| 1 | 0 | 1 | 0 | 0 | |
| 1 | 0 | 0 | 1 | 0 |
| # | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | |
| 1 | 0 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | |
| 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 0 | |
| 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | |
| 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 1 | |
| 0 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | |
| 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | |
| 0 | 1 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | |
| 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | |
| 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | |
| 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | |
| 0 | 0 | 0 | 0 | 0 |
| # | |||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | |
| 1 | 0 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 0 | 0 | |
| 1 | 0 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 0 | 0 | |
| 0 | 1 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 1 | 1 | |
| 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | |
| 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | |
| 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | |
| 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | |
| 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | |
| 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 1 | |
| 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | |
| 0 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 1 | |
| 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | |
| 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | 0 |
| # | c | ||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 0 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | |
| 1 | 0 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | |
| 1 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 0 | 0 | 0 | |
| 1 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 0 | 0 | 0 | |
| 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | |
| 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | |
| 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | |
| 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | |
| 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | |
| 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | |
| 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | |
| 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | |
| 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 1 | 0 | |
| 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | |
| 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | |
| 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | |
| 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | |
| 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | |
| 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | |
| 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | |
| 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | |
| 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 1 | 0 | |
| 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | |
| 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 0 |
| # | ||||||||||||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 0 | 1 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 0 | 0 | 1 | 1 | 1 | 1 | 1 | 1 | 0 | 0 | 1 | 1 | 1 | 1 | |
| 0 | 1 | 0 | 1 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 0 | 1 | 0 | 1 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | 1 | 1 | 0 | 1 | 1 | 1 | 1 | 1 | 0 | 1 | 0 | |
| 0 | 1 | 0 | 1 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 1 | 0 | 0 | 0 | 0 | 1 | 0 | 1 | 1 | 1 | 0 | 0 | 1 | 1 | 0 | 1 | 1 | 1 | 0 | |
| 0 | 1 | 0 | 0 | 1 | 1 | 0 | 0 | 0 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | 1 | 1 | 1 | 0 | 0 | 1 | 1 | 1 | 1 | 0 | 0 | |
| 0 | 1 | 0 | 0 | 1 | 1 | 0 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 1 | 0 | 0 | 1 | 1 | 1 | 1 | 1 | 1 | |
| 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 0 | 0 | 0 | 0 | 1 | 1 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 0 | 1 | 0 | 1 | 1 | 1 | 1 | 1 | 0 | 1 | 0 | 1 | |
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Svozil, K. What Is So Special about Quantum Clicks? Entropy 2020, 22, 602. https://doi.org/10.3390/e22060602
Svozil K. What Is So Special about Quantum Clicks? Entropy. 2020; 22(6):602. https://doi.org/10.3390/e22060602
Chicago/Turabian StyleSvozil, Karl. 2020. "What Is So Special about Quantum Clicks?" Entropy 22, no. 6: 602. https://doi.org/10.3390/e22060602
APA StyleSvozil, K. (2020). What Is So Special about Quantum Clicks? Entropy, 22(6), 602. https://doi.org/10.3390/e22060602





