1. Introduction
In dealing with mixed states of a physical system, one has to be careful when speaking about entanglement. The definition of bipartite mixed state entanglement is unique (although problems may arise in dealing with multipartite entanglement [
1]), but its quantification relies on several different criteria and it is not yet fully developed: many difficulties have arisen in the definition of physically sensible measures [
2,
3]. The main problem affecting a few known mixed state entanglement measures is indeed the fact that extending a measure from a pure state case to a mixed state case usually requires challenging maximization procedures over all its possible pure state decompositions [
4,
5,
6]. Notwithstanding, the investigation of the connection between entanglement and mixedness exhibited by a quantum system is of great interest, for example, in quantum computation theory [
7,
8] and in quantum teleportation [
9]. The threshold of mixedness exhibited by a quantum system compatible with the occurrence of entanglement between two parties of the same system has been analyzed, leading for example to the so-called Kus-Zyczkowski ball of absolutely separable states [
10,
11,
12,
13]. Quite recently, possible links between entanglement and easily measurable observables have been exploited to define experimental protocols aimed at measuring quantum correlations [
14,
15,
16]. The use of measurable quantities as entanglement witnesses for a wide class of systems has been known for some time [
17,
18], but an analogous possibility amounting at entanglement measures is a recent and growing challenge. To the present day, some bounds for entanglement are measured in terms of correlation functions in spin systems [
19] or using quantum quenches [
20]. Indeed, an experimental measure of entanglement is, generally speaking, out of reach because of the difficulty in addressing the local properties of many-particle systems and of the fundamental non-linearity of entanglement quantifiers. For this reason, the best one can do is to provide experimentally accessible bounds on some entanglement quantifiers [
21]. The aim of this paper is to build a bound to the entanglement degree in a general bipartition of a physical system in a mixed state. We are going to establish an upper bound to the negativity
N [
22] in terms of the linear entropy
. We are thus studying what is called negative partial transpose (NPT) entanglement. It should however be emphasized that a non-zero negativity is a sufficient but not necessary condition to detect entanglement, since positive partial transpose (PPT, or bound) entanglement exists across bipartitions of dimensions higher than
, which cannot be detected by means of the negativity criterion [
23]. Our investigation contributes to the topical debate concerning a link between quantum correlations and mixedness [
24]. We stress that our result is of experimental interest since the bound on
N may easily be evaluated by measuring the linear entropy.
2. An Upper Bound to the Negativity in Terms of Linear Entropy
Consider a
d-dimensional system S in a state described by the density matrix
where each
represents a pure state, and define a bipartition into two subsystems S
and S
with dimensions
and
respectively (
). It is common [
19] to define negativity as
where
,
is the matrix obtained through a partial transposition with respect to the subsystem
and
is the trace norm (
). In what follows we will call
. By construction,
, with
for maximally entangled states only. Furthermore, the linear entropy
in our system is defined as
where
is a measure of mixedness in terms of the purity
of the state,
being the Hilbert–Schmidt norm of
(
). By definition,
for any pure state while
for maximally mixed states. It is easy to see that there exists a link between the trace norm of an operator
O in a
d-dimensional Hilbert space and its Hilbert–Schmidt norm. Such a link can be expressed as
where
is the
i-th eigenvalue of
O and the so-called Chebyshev sum inequality
has been used. Since, in addition, the Hilbert–Schmidt norm is invariant under partial transposition, one readily gets a first explicit link between negativity and mixedness
, valid for generic
d-dimensional systems, in the form of an upper bound, which reads
Equation (
5) provides an upper bound to the negativity
N in terms of
and thus, in view of Equation (
3), in terms of the linear entropy. This bound imposes a maximal zero value for
N only for a maximally mixed state, the maximum being taken over all bipartite quantum states of the same purity. It is known [
10,
11], however, that no quantum state of
S can exhibit bipartite entanglement if its purity is smaller than or equal to
. In other words, the noise due to the mixedness of the state is too high for bipartite correlations to exist. Additionally, in the case of a pure (or almost pure) state, the bound becomes useless as long as the bipartition is not “balanced” (by “balanced” we mean a bipartition where
). It indeed becomes greater than one (thus being unable to give information about entanglement) for mixedness smaller than
, which might even approach 1 in some specific cases (recall that, by definition,
). We however expect entanglement to be unbounded only in the case of pure states (
). In the following we show that bound (
5) can be strengthened.
3. Strengthening the Previous Bound
Observe firstly that the rank
of
is not greater than
(equal to
d) when
is pure (maximally mixed). For this reason we write
By construction,
since any pure state can be written in Schmidt decomposition consisting of
vectors, and
because a maximally mixed state is proportional to identity. Since by definition
holds for any physical system, Equation (
5) may be substituted by the following inequality:
Note however that there are at least some physical systems for which the function in (
6), due to the maximization procedure involved in its definition, is always equal to
d in the range
, showing then a discontinuity at
as
Since we want our result to hold generally, independently of the particular system analyzed, Equation (
7) cannot improve Equation (
5) because even for slightly mixed states
we have a priori no information on
which might be equal to
d, tracing back Equation (
7) to Equation (
5). Despite this, we may correct (
7) exploiting the expectation that for very low mixedness some of these eigenvalues are much smaller than the others. Indeed, for all the
, non-vanishing eigenvalues appearing in Equation (
4) are treated on equal footing in going from
to
. To properly take into account the difference between them, go back to Equation (
1) and define a reference pure state
at will among the ones having the largest occupation probability
. The spectrum of
consists of
non-zero eigenvalues
and of
zero eigenvalues
.
We call the former
-class eigenvalues and the latter
-class eigenvalues, and obviously the latter class does not contribute to
. In order to strengthen (
5) we are interested in the spectrum of
which generally consists of
d non-zero eigenvalues. Unfortunately, then, we cannot directly introduce analogous
- and
-classes to identify which eigenvalues contribute to the sum involved in Equation (
4) comparatively much less than the other ones, when the state
possesses a low mixedness degree and is thus very close to a pure state. To overcome this difficulty, let us consider a parameter-dependent class of density matrices associated to the given
with
, such that
and
. This means that, for all
i,
In addition, we assume that all
are continuous functions of
x. Thus,
continuously connects
and
and, as a consequence, any
is continuously connected to a particular eigenvalue of
, which will be the the corresponding mixed state
-class eigenvalue
. In this way one can define the function
as the eigenvalue of
having the property
and so the
-class eigenvalue for
as
We emphasize at this point that the results of this paper do not depend on the explicit functional dependence of
on
x, which can be chosen at will provided it satisfies conditions (11), nor on the range of variability of
x itself. Indeed,
and
x are just mathematical tools, with (in general) no physical meaning. To save some writing and in view of Equation (
4), we put
and notice that
. We can now state (see
Appendix A for a proof) the following.
Lemma 1. Given a state ρ of a system in a d-dimensional Hilbert space, and the associated reference pure state , for any set of states satisfying (9) and (11) there exists a value such that for any . This result is of course based on the possibility to define a reference pure state . As previously explained, such a possibility rests on the assumed existence of one (or a subset of) in one pure-state decomposition of ρ, such that . In other words, one (or a subset of) pure state(s) must be occupied with probability higher (and not equal to) the populations of the rest of the pure states. If this is verified, then the reference pure state is well defined. Lemma 1 allows us to find a function
such that
and
Starting from the identity
and applying the Chebyshev sum inequality term-by-term, we obtain
where Lemma 1 has been exploited. Expressing Equation (
17) in terms of negativity and purity, we finally get
Bound (
18) improves bound (
7) for high purity when
is small (i.e.,
), generally becoming greater than
at low purity. In addition, it still suffers the same drawback as
, not vanishing when
. In such a case one has to consider the lower bound
on purity, below which no entanglement survives. In order to take such a bound into account, instead of distinguishing among
and
eigenvalues of
, we can divide them into non-negative ones
and negative ones
. In this way, calling
and
the numbers of negative and non-negative eigenvalues, respectively, and applying the Lagrange multiplier method to the function
subjected to constraints
and
, one finds
Bound (
19) can be exploited to show that no entanglement can survive at purity lower than
. Indeed, for entanglement to exist, at least one eigenvalue has to be negative. However, by normalization, it always has to be true that
and this implies that, as long as
, purity
cannot be smaller than
as expected. However, in general, the number of negative eigenvalues is not known. In these cases, the best one can do is to look for the maximum, with respect to
, of the right-hand side of (
19), leading unfortunately once again to bound (
5) on
N. However, since always
, where
is the Heaviside step function, defining
, we state our final and main result as
valid for every possible bipartition of a quantum system, independent of its (finite) dimension, its detailed structure, or its properties. It is worth stressing that computing negativity quickly becomes a very hard task as the dimension of the Hilbert space grows, while the evaluation of purity can be performed without particular efforts. We emphasize in addition that bound
Q in Equation (
20) only depends on purity, and is completely determined once a bipartition of the physical system is fixed and purity is known. This means that an experimental measure of purity allows the extraction of information about the maximal degree of bipartite entanglement one can find in the system under scrutiny. Some purity-measuring protocols, or at least purity estimations based on experimental data, have been proposed. They are based on statistical analysis of homodyne distributions, obtained measuring radiation field tomograms [
25], on the properties of graph states [
26], or on the availability of many different copies of the state over which separable measurements are performed [
27]. In all cases where a measure of purity is possible, an experimental estimation of bipartite entanglement is available thanks to Equation (
20), which is then actually experimentally accessible.
4. Crossover between and and Numerical Results
As commented previously, bounds
(Equation (
5)) and
(Equation (
18)) can supply information about bipartite entanglement in two different setups. Indeed,
is accurate enough for a balanced bipartition (i.e., when
) but fails when
since it rapidly becomes greater than 1. To solve this problem, we obtained the bound
which, by construction, provides nontrivial information about bipartite entanglement in an unbalanced bipartition
, but may not work properly for a balanced one. In order to build the last bound
in the previous section we started with a more detailed analysis of the structure of the eigenvalues after partial transposition. This analysis leads us, specifically, to study the rank of the partial transpose, as this is one key parameter used in the Chebyshev inequality. In this section we demonstrate the existence of a special regime of the purity wherein the helpfulness of
against
emerges in a very transparent way. It is actually a very easy task to show that our new bound
works better than the old one,
(i.e.,
), when the purity
P is greater than a critical value given in terms of the total Hilbert space dimension and of the subdimensions of the bipartition—that is, when
Two limiting cases are easily studied directly from Equation (
21): for a perfectly balanced bipartition (
), one gets
and, since by definition
, in this case the bound
is smaller (and therefore works better) than the bound
for any possible quantum state. In the opposite limit, in a strongly unbalanced bipartition, one can roughly approximate
and, since by definition
, this leads to
. Taking into account the natural bounds for the purity of a quantum state, this in turn means that in such a limit
for any quantum state or, in other words, our new bound always works better. This behavior can be clearly seen in
Figure 1, where the dependence of
on
d and
is shown together with the natural limiting values of
.
To better exemplify this behavior, we report here results of numerical simulations performed with the aid of the
package for Mathematica [
28], by which random quantum states were generated in different dimensions, uniformly distributed according to different metrics. On these states, we tested bounds
and
.
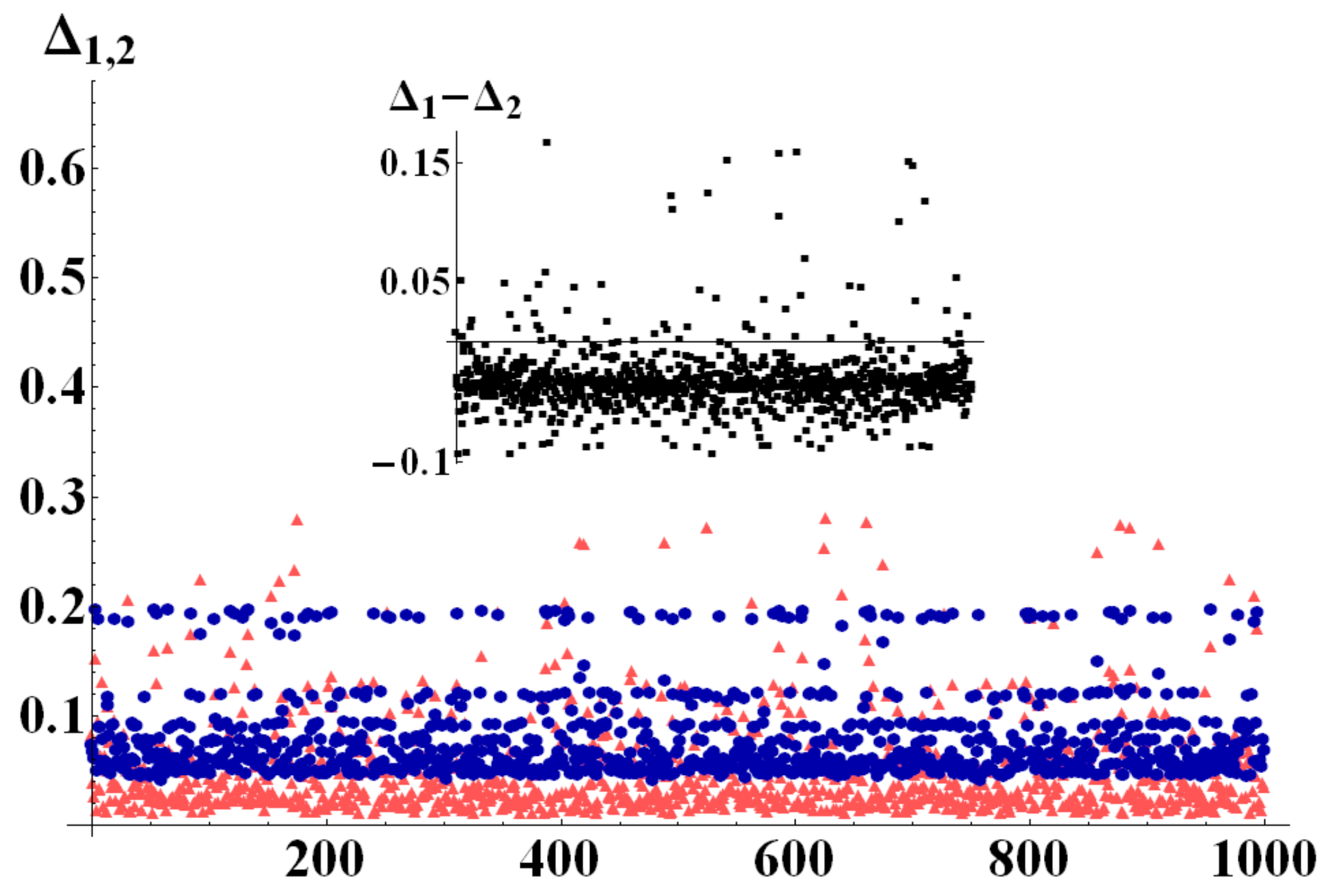
Figure 2,
Figure 3 and
Figure 4 show the differences
of the bounds with the negativity of the state, once a bipartition was fixed. In particular, in a first run of simulations (
Figure 2) we generated
perfectly balanced bipartite states (such that
), randomly choosing the dimension of the two subsystems for each quantum state within the range
. The results in
Figure 2 clearly show that
for all the analyzed states. The second run of simulations was performed with
randomly chosen in
and
. In this case, as can be seen in
Figure 3 the difference
has no fixed sign. The two subdimensions are, indeed, such that the critical value of purity
in Equation (
21) is neither extremely close to
nor to 1. As can be noticed from the inset of
Figure 3 which shows the difference
, however, on the average it is still true that
. The third set of numerical data, finally, was obtained generating
random states with subdimensions
and
randomly drawn in
. In this limit the value of
is very close to the minimum of purity and we therefore expect
to work better than
for almost any state. This is indeed confirmed by the simulations shown in
Figure 4, in which
. As an example application of our results, consider a single two-level system interacting with a spin system composed of
spins, each of which lives in a
dimensional Hilbert space. Therefore, the total system Hilbert space has dimension
. Let us suppose the spin system is a chain of 10 spin
(which is a relatively small system, very far from its thermodynamic limit). The total Hilbert space dimension will then be
, and considering the natural bipartition into the two-level system and the spin chain, one has
and
. For such a system, the critical value of purity
in Equation (
21) is
The lower value of purity for which bipartite entanglement can survive is, as stated previously,
. Therefore, for all the total states having purity
, bound
in Equation (
18) works better than
. Only for the small fraction of states having an extremely low purity in the range
bound
gives better information than
. This again shows that, for unbalanced bipartitions (and even in the case of a relatively small number of individual components of the total system),
works much better than
.
5. Application to Thermal Entanglement
Of particular interest is the application of the results of this paper to the case of thermal entanglement, where both linear entropy and its link to negativity acquire a much clearer meaning. A recent result [
29] indeed shows how the canonical ensemble description of thermal equilibrium stems from the existence of quantum correlations between a system and its thermal bath. In view of this it has been shown that it is possible, with a very small statistical error, to replace the system + bath microcanonical ensemble with a pure state inside the suitable energy shell, still obtaining the appropriate thermal statistics characterizing Gibbs distribution. In this context, then, the linear entropy of the mixed Gibbs state provides a system/bath entanglement measure. The mixedness of a quantum state can originate from the fact that the quantum system S is entangled to another, external system E. If this is the case, the reduced state of the system S is mixed. Moreover, if S and E are maximally entangled, the reduced state of S is maximally mixed. Hence, when one looks at the mixedness of the state of S, one can see it as measuring the amount of entanglement across the S + E bipartition, provided S + E is in a pure state. In this case (and in this case only), our bounds can be seen as resulting from monogamy of quantum correlations: the more S is entangled to E (hence, the more mixed the state of S is), the less subparts of S can create entanglement between them (because they are both already strongly entangled with E). This gives an intuition of the physical origin of our bounds in the case of S being coupled to another system E, and when the two can be considered to be in a pure total state. This is exactly the situation suggested in [
29] as being at the origin of Gibbs thermal states. In this sense, thermal mixedness in S can be seen as the result of entanglement in a pure S + E state, E being the thermal environment. Equation (
20) can then be viewed as a monogamy relation, describing the competition between two kinds of quantum correlations—internal ones measured by negativity and external ones measured by entropy. On the other hand, it is known that some thermodynamic quantities (e.g., heat capacity or internal energy) can be used as entanglement witnesses [
18], and recent works have shown an even closer link between heat capacity and entanglement for particular systems [
30,
31]. The result of this paper suggests this link might hold very generally. Indeed, in the case of a Gibbs equilibrium state,
can be given by the expression
where
is the
i-th energy level of the system and
Z is its partition function,
being the inverse temperature in units of
. Heat capacity in a finite dimensional system reads
There is then a similarity between
and
as given by Equations (
23) and (
24), suggesting how a measure of the latter, together with little knowledge about the energy spectrum of the physical system, might supply significant information on the linear entropy of the system and, as a consequence, on its maximal degree of internal bipartite entanglement. This triggers interest in further future investigation on a detailed analysis of the relation between
and
which, in turn, might supply us with an easily experimentally measurable entanglement bound as well as highlight how the origin of thermodynamic properties is strongly related to non-classical correlations and monogamy effects. Such a connection, and the usefulness of the bounds derived in the previous sections, can be exemplified with a simple three-qutrit system with a parameter-dependent Hamiltonian
where
is the spin operator of the
i-th particle,
, and
are real interaction parameters. This effective Hamiltonian operator describes a system consisting of two ultracold atoms (spins labeled as 1 and 2) in a two-well optical lattice and in the Mott insulator phase, where thus the tunneling term in the usual Bose–Hubbard picture is accounted for as a second-order perturbative term, both coupled with a third atom (labeled as 0) via a Heisenberg-like interaction. An external magnetic field is also present, uniformly coupled to the three atoms. Such a system is a generalization of the one studied in [
31], where a deep connection between thermal entanglement and heat capacity in parameter space has been shown. Hamiltonian (
25) is analytically diagonalizable, thus allowing us to obtain explicit expressions for thermodynamic quantities characterizing the Gibbs equilibrium state of the three-atom system, together with the negativity of the reduced state of the two quadratically coupled spins.
The mathematical origin of the connection between heat capacity and negativity was already discussed in [
31] and is ultimately due to the presence of level crossing in the low-lying energy eigenvalues of the system. Here we want to show how the existence of the strong connection between purity and negativity, expressed by bound (
20), can give some hints for a physical explanation of such an effect, and moreover to exemplify how bound (
20) can often supply important information on the amount of thermal entanglement.
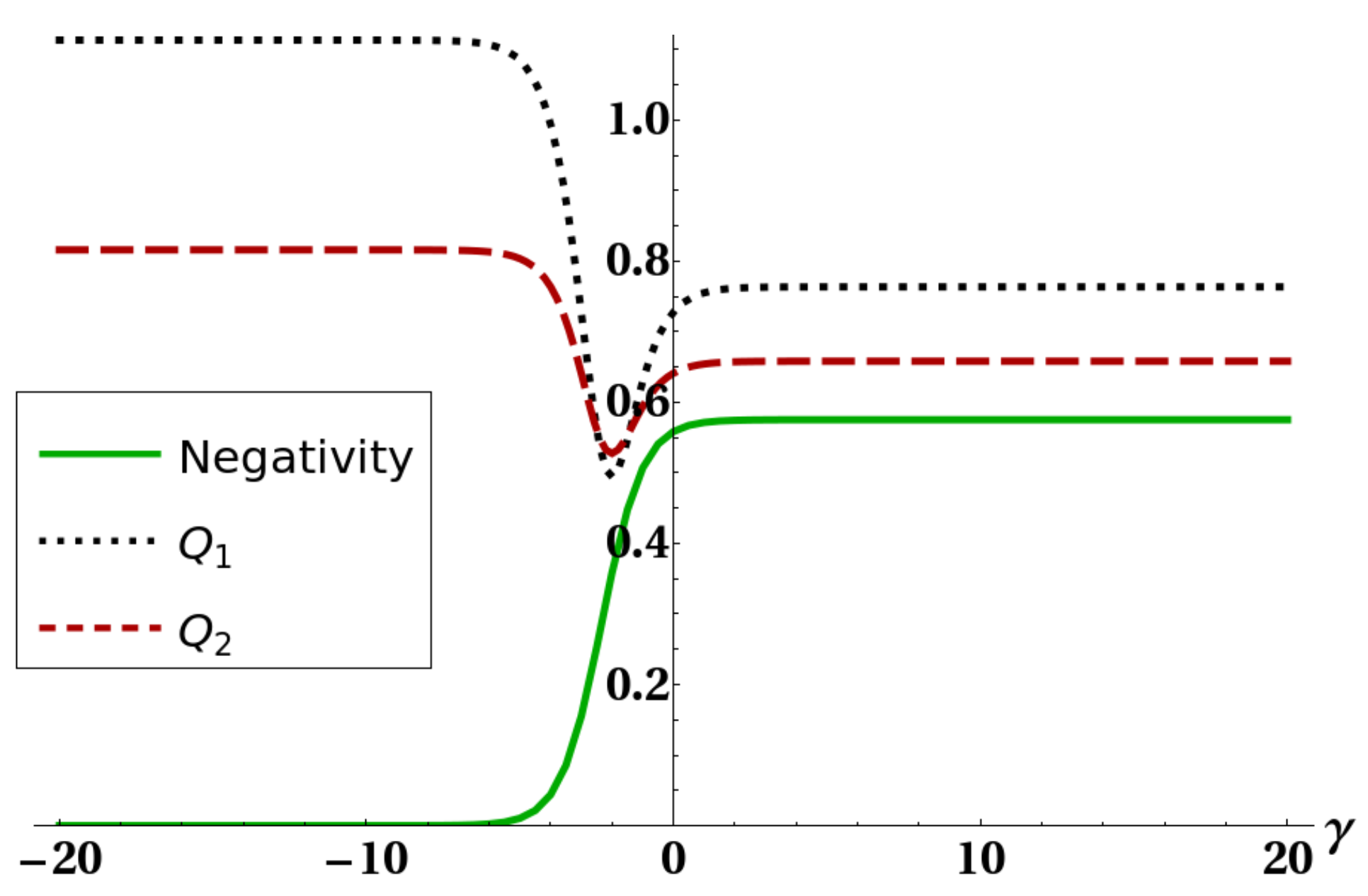
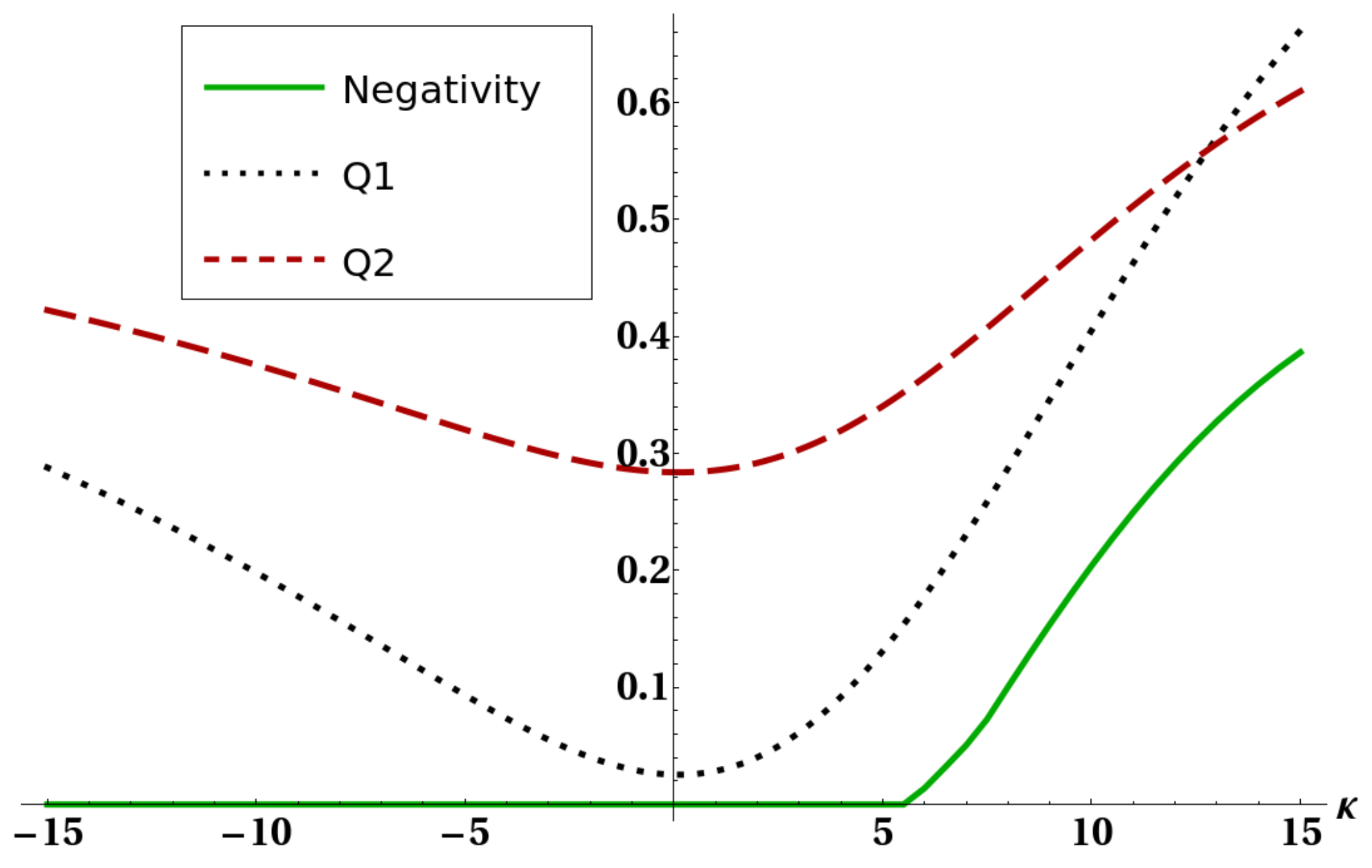
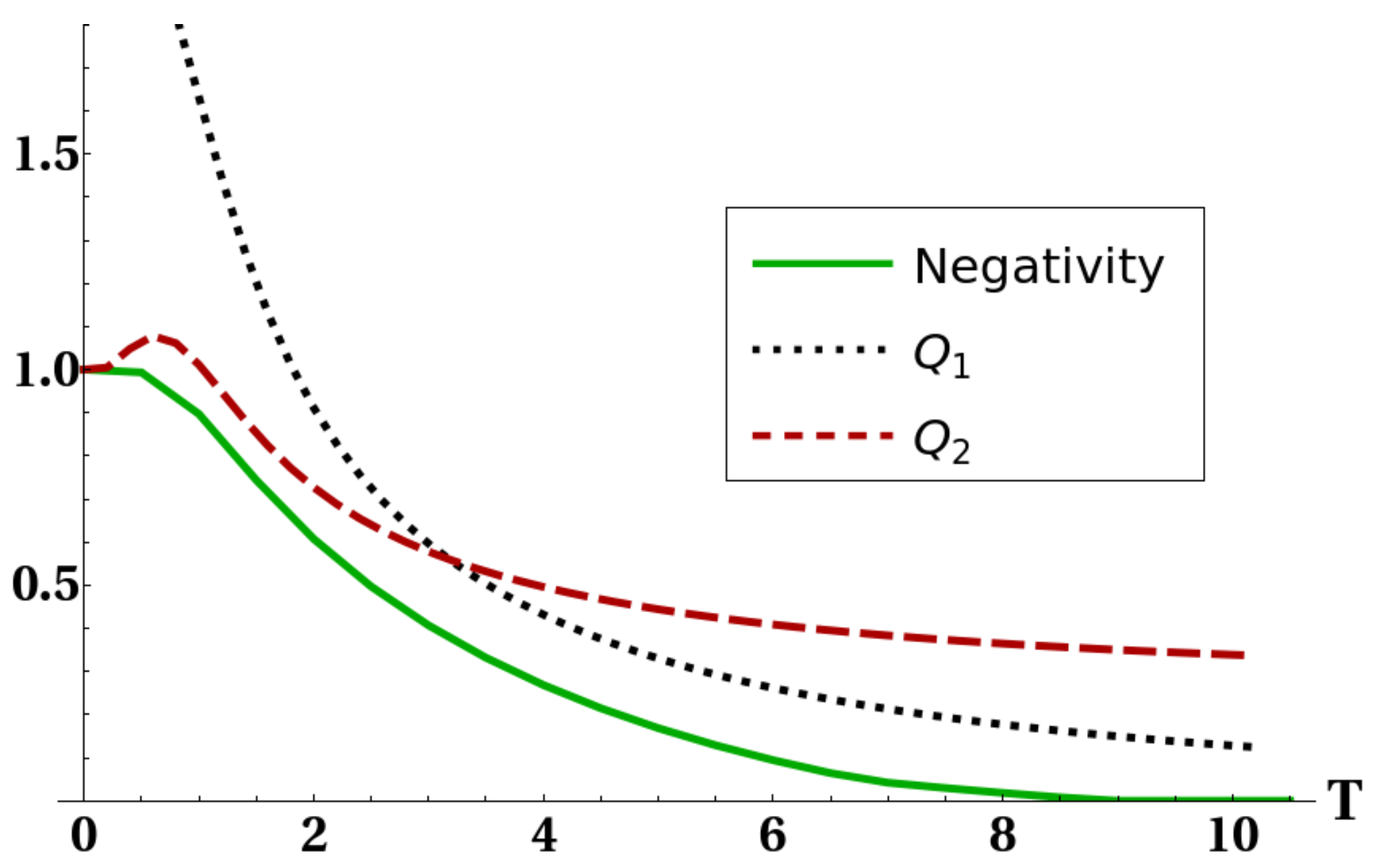
Figure 5 shows how the connection between thermal entanglement and heat capacity highlighted in [
31] is still present despite the interaction with a third atom.
Figure 6 and
Figure 7 show bounds (
5) and (
18), together with the negativity of the reduced state of two atoms, versus a certain interaction parameter in the Hamiltonian.
Figure 8 finally shows the same quantities versus temperature for fixed Hamiltonian parameters. All energies in the plots are expressed in units of
. It is worth stressing here that, in all these plots, bound
is not shown. The reason is that, in order to preserve thermal entanglement, the temperature in our simulations had to be kept at most of the same order of magnitude of spin–spin interactions, and in such a regime
has not yet crossed the threshold
so
was constantly equal to one. It is clearly shown in
Figure 6 and
Figure 8 how bound
given in (
5) can become, as discussed, larger than 1. In all these cases (except for a small temperature range in
Figure 8, however, (
18) is still able to sensibly bound negativity. In all the plots shown, and in general every time the bounds (
5) and (
18) are applied to the particular system analyzed here, one always gets useful information about bipartite entanglement in the form, of course, of an upper bound. However, such a bound gets very close to zero in some particular cases (e.g.,
Figure 7 and
Figure 8), strongly restricting the allowed range of values for the negativity. It is then shown that (
20) is able to produce non-trivial results. It is worth noting that in
Figure 5 there are ranges of the parameter
where the negativity and the heat capacity exhibit simultaneous plateaus. This fact, also previously shown and commented in [
31], in view of Equation (
20) and the strong link between heat capacity and the mixedness
of a quantum state, legitimizes the deduction that in the parameter regions of very low negativity the heat capacity may be assumed as almost constant.