Relationship between the Transport Coefficients of Polar Substances and Entropy
Abstract
1. Introduction
2. Equation of State and Transport Properties
3. Diffusion Coefficient
4. Viscosity Coefficient
5. Thermal Conductivity Coefficient
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Van der Spoel, D.; Lindahl, E.; Hess, B.; van Buuren, A.R.; Apol, E.; Meulenhoff, P.J.; Tieleman, D.P.; Sijbers, A.L.T.M.; Feenstra, K.A.; van Drunen, R.; et al. Gromacs User Manual Version 4.5.4; The GROMACS Development Teams at the Royal Institute of Technology and Uppsala University: Uppsala, Sweden, 2010. [Google Scholar]
- CAS Databases. Available online: https://www.cas.org/about/cas-content (accessed on 20 December 2019).
- Hirschfelder, J.O.; Curtiss, C.F.; Bird, R.B. The Molecular Theory of Gases and Liquids; Wiley-Interscience: Hoboken, NJ, USA, 1964. [Google Scholar]
- Miller, C.C. The Stokes-Einstein Law for Diffusion in Solution. Proc. R. Soc. Math. Phys. Eng. Sci. 1924, 106, 724–749. [Google Scholar] [CrossRef]
- Sutherland, W. LXXV. A dynamical theory of diffusion for non-electrolytes and the molecular mass of albumin. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1905, 9, 781–785. [Google Scholar] [CrossRef]
- Einstein, A. Über die von der molekularkinetischen Theorie der Wärme geforderte Bewegung von in ruhenden Flüssigkeiten suspendierten Teilchen. Ann. Der Phys. 1905, 322, 549–560. [Google Scholar] [CrossRef]
- Vorob’ev, V.S. Application of the New Scaling Relations and Global Isomorphism to the Study of Liquid–Vapor Saturation Pressure. J. Phys. Chem. 2012, 116, 4248–4254. [Google Scholar] [CrossRef] [PubMed]
- Kulinskii, V.L. Global isomorphism between the Lennard-Jones fluids and the Ising model. J. Chem. Phys. 2010, 133, 034121. [Google Scholar] [CrossRef]
- Bulavin, L.; Cheplak, V.; Kulinskii, V.L. Global Isomorphism Approach: Main Results and Perspectives. In Springer Proceedings in Physics; Springer International Publishing: Berlin, Germany, 2015; pp. 53–75. [Google Scholar] [CrossRef]
- Ingebrigtsen, T.S.; Schrøder, T.B.; Dyre, J.C. Isomorphs in Model Molecular Liquids. J. Phys. Chem. B 2012, 116, 1018–1034. [Google Scholar] [CrossRef]
- Dyre, J.C. Isomorph theory of physical aging. J. Chem. Phys. 2018, 148, 154502. [Google Scholar] [CrossRef]
- Dyre, J.C. Perspective: Excess-entropy scaling. J. Chem. Phys. 2018, 149, 210901. [Google Scholar] [CrossRef]
- Dyakonov, G. Questions of the Similarity Theory in the Field of Physical and Chemical Processes; Publishing House of the USSR Academy of Sciences: Moscow, Russia, 1956. (In Russian) [Google Scholar]
- Usmanov, A. On an Additional Condition for the Similarity of Molecular Processes; Publishing House of the USSR Academy of Sciences: Moscow, Russia, 1959. (In Russian) [Google Scholar]
- Mukhamedzyanov, G.; Usmanov, A. Thermal Conductivity of Organic Liquids; ACS Publications: Washington, DC, USA, 1971. (In Russian) [Google Scholar]
- Rosenfeld, Y. Relation between the transport coefficients and the internal entropy of simple systems. Phys. Rev. A 1977, 15, 2545–2549. [Google Scholar] [CrossRef]
- Dzugutov, M. A universal scaling law for atomic diffusion in condensed matter. Nature 1996, 381, 137–139. [Google Scholar] [CrossRef]
- Dzugutov, M. Addendum: A universal scaling law for atomic diffusion in condensed matter. Nature 2001, 411, 720. [Google Scholar] [CrossRef]
- Galliero, G.; Boned, C.; Fernández, J. Scaling of the viscosity of the Lennard-Jones chain fluid model, argon, and some normal alkanes. J. Chem. Phys. 2011, 134, 064505. [Google Scholar] [CrossRef] [PubMed]
- Bell, I.H.; Messerly, R.; Thol, M.; Costigliola, L.; Dyre, J.C. Modified Entropy Scaling of the Transport Properties of the Lennard-Jones Fluid. J. Phys. Chem. B 2019, 123, 6345–6363. [Google Scholar] [CrossRef] [PubMed]
- Bell, I.H.; Hellmann, R.; Harvey, A.H. Zero-Density Limit of the Residual Entropy Scaling of Transport Properties. J. Chem. Eng. Data 2019. [Google Scholar] [CrossRef]
- Galliero, G.; Boned, C. Thermal conductivity of the Lennard-Jones chain fluid model. Phys. Rev. E 2009, 80. [Google Scholar] [CrossRef]
- Ojovan, M.I. Thermodynamic Parameters of Bonds in Glassy Materials from Shear Viscosity Coefficient Data. Int. J. Appl. Glass Sci. 2014, 5, 22–25. [Google Scholar] [CrossRef]
- Novak, L. Self-Diffusion Coefficient and Viscosity in Fluids. Int. J. Chem. React. Eng. 2011, 9. [Google Scholar] [CrossRef]
- Novak, L.T. Predictive Corresponding-States Viscosity Model for the Entire Fluid Region: n-Alkanes. Ind. Eng. Chem. Res. 2013, 52, 6841–6847. [Google Scholar] [CrossRef]
- Novak, L.T. Predicting Fluid Viscosity of Nonassociating Molecules. Ind. Eng. Chem. Res. 2015, 54, 5830–5835. [Google Scholar] [CrossRef]
- Novak, L.T. Fluid Viscosity-Residual Entropy Correlation. Int. J. Chem. React. Eng. 2011, 9. [Google Scholar] [CrossRef]
- Klinov, A.; Kazantsev, S.; Dyaconov, G.; Dyakonov, S. Analytical Equation of State for Lennard-Jones Fluids; Herald of The Kazan Technological University: Kazan, Russia, 2010; pp. 10–16. (In Russian) [Google Scholar]
- Vogel, E.; Jäger, B.; Hellmann, R.; Bich, E. Ab initiopair potential energy curve for the argon atom pair and thermophysical properties for the dilute argon gas. II. Thermophysical properties for low-density argon. Mol. Phys. 2010, 108, 3335–3352. [Google Scholar] [CrossRef]
- Bich, E.; Hellmann, R.; Vogel, E. Ab initiopotential energy curve for the neon atom pair and thermophysical properties for the dilute neon gas. II. Thermophysical properties for low-density neon. Mol. Phys. 2008, 106, 813–825. [Google Scholar] [CrossRef]
- Jäger, B.; Hellmann, R.; Bich, E.; Vogel, E. State-of-the-art ab initio potential energy curve for the krypton atom pair and thermophysical properties of dilute krypton gas. J. Chem. Phys. 2016, 144, 114304. [Google Scholar] [CrossRef] [PubMed]
- Hellmann, R.; Bich, E.; Vogel, E. Ab initio intermolecular potential energy surface and second pressure virial coefficients of methane. J. Chem. Phys. 2008, 128, 214303. [Google Scholar] [CrossRef] [PubMed]
- Water Models. Available online: http://www1.lsbu.ac.uk/water/water_models.html (accessed on 20 December 2019).
- Stockmayer, W.H. Second Virial Coefficients of Polar Gases. J. Chem. Phys. 1941, 9, 398–402. [Google Scholar] [CrossRef]
- Matsunaga, N.; Nagashima, A. Prediction of the transport properties of gaseous water and its isotopes at high temperatures. J. Phys. Chem. 1983, 87, 5268–5279. [Google Scholar] [CrossRef]
- Mourits, F.M.; Rummens, F.H.A. A critical evaluation of Lennard–Jones and Stockmayer potential parameters and of some correlation methods. Can. J. Chem. 1977, 55, 3007–3020. [Google Scholar] [CrossRef]
- Wagner, W.; Pruß, A. The IAPWS Formulation 1995 for the Thermodynamic Properties of Ordinary Water Substance for General and Scientific Use. J. Phys. Chem. Ref. Data 2002, 31, 387–535. [Google Scholar] [CrossRef]
- Tillner-Roth, R.; Harms-Watzenberg, F.; Baehr, H. Eine neue Fundamentalgleichung für Ammoniak. DKV Tagungsbericht 1993, 20, 167–180. [Google Scholar]
- Schroeder, J.A.; Penoncello, S.G.; Schroeder, J.S. A Fundamental Equation of State for Ethanol. J. Phys. Chem. Ref. Data 2014, 43, 043102. [Google Scholar] [CrossRef]
- Piazza, L.; Span, R. An equation of state for methanol including the association term of SAFT. Fluid Phase Equilibria 2013, 349, 12–24. [Google Scholar] [CrossRef]
- Huber, M.L.; Perkins, R.A.; Laesecke, A.; Friend, D.G.; Sengers, J.V.; Assael, M.J.; Metaxa, I.N.; Vogel, E.; Mareš, R.; Miyagawa, K. New International Formulation for the Viscosity of H2O. J. Phys. Chem. Ref. Data 2009, 38, 101–125. [Google Scholar] [CrossRef]
- Fenghour, A.; Wakeham, W.A.; Vesovic, V.; Watson, J.T.R.; Millat, J.; Vogel, E. The Viscosity of Ammonia. J. Phys. Chem. Ref. Data 1995, 24, 1649–1667. [Google Scholar] [CrossRef]
- Xiang, H.W.; Laesecke, A.; Huber, M.L. A New Reference Correlation for the Viscosity of Methanol. J. Phys. Chem. Ref. Data 2006, 35, 1597–1620. [Google Scholar] [CrossRef]
- Kiselev, S.B.; Ely, J.F.; Abdulagatov, I.M.; Huber, M.L. Generalized SAFT-DFT/DMT Model for the Thermodynamic, Interfacial, and Transport Properties of Associating Fluids: Application forn-Alkanols. Ind. Eng. Chem. Res. 2005, 44, 6916–6927. [Google Scholar] [CrossRef]
- Huber, M.L.; Perkins, R.A.; Friend, D.G.; Sengers, J.V.; Assael, M.J.; Metaxa, I.N.; Miyagawa, K.; Hellmann, R.; Vogel, E. New International Formulation for the Thermal Conductivity of H2O. J. Phys. Chem. Ref. Data 2012, 41, 033102. [Google Scholar] [CrossRef]
- Tufeu, R.; Ivanov, D.Y.; Garrabos, Y.; Neindre, B.L. Thermal Conductivity of Ammonia in a Large Temperature and Pressure Range Including the Critical Region. Berichte Bunsenges. Phys. Chem. 1984, 88, 422–427. [Google Scholar] [CrossRef]
- Sykioti, E.A.; Assael, M.J.; Huber, M.L.; Perkins, R.A. Reference Correlation of the Thermal Conductivity of Methanol from the Triple Point to 660 K and up to 245 MPa. J. Phys. Chem. Ref. Data 2013, 42, 043101. [Google Scholar] [CrossRef]
- Assael, M.J.; Sykioti, E.A.; Huber, M.L.; Perkins, R.A. Reference Correlation of the Thermal Conductivity of Ethanol from the Triple Point to 600 K and up to 245 MPa. J. Phys. Chem. Ref. Data 2013, 42, 023102. [Google Scholar] [CrossRef]
- PureEOS (julia library). Available online: https://github.com/KSTU/PureEOS.jl (accessed on 20 December 2019).
- Bell, I.H.; Wronski, J.; Quoilin, S.; Lemort, V. Pure and Pseudo-pure Fluid Thermophysical Property Evaluation and the Open-Source Thermophysical Property Library CoolProp. Ind. Eng. Chem. Res. 2014, 53, 2498–2508. [Google Scholar] [CrossRef]
- Suárez-Iglesias, O.; Medina, I.; de los Ángeles Sanz, M.; Pizarro, C.; Bueno, J.L. Self-Diffusion in Molecular Fluids and Noble Gases: Available Data. J. Chem. Eng. Data 2015, 60, 2757–2817. [Google Scholar] [CrossRef]
- Abraham, M.J.; Murtola, T.; Schulz, R.; Páll, S.; Smith, J.C.; Hess, B.; Lindahl, E. GROMACS: High performance molecular simulations through multi-level parallelism from laptops to supercomputers. SoftwareX 2015, 1-2, 19–25. [Google Scholar] [CrossRef]
- Kutzner, C.; Páll, S.; Fechner, M.; Esztermann, A.; Groot, B.L.; Grubmüller, H. More bang for your buck: Improved use of GPU nodes for GROMACS 2018. J. Comput. Chem. 2019, 40, 2418–2431. [Google Scholar] [CrossRef] [PubMed]
- Schnabel, T.; Vrabec, J.; Hasse, H. Henry’s law constants of methane, nitrogen, oxygen and carbon dioxide in ethanol from 273 to 498 K: Prediction from molecular simulation. Fluid Phase Equilibria 2005, 233, 134–143. [Google Scholar] [CrossRef]
- Monchick, L.; Mason, E.A. Transport Properties of Polar Gases. J. Chem. Phys. 1961, 35, 1676–1697. [Google Scholar] [CrossRef]
- Abramson, E.H. Viscosity of water measured to pressures of 6GPa and temperatures of 300 C. Phys. Rev. E 2007, 76. [Google Scholar] [CrossRef]
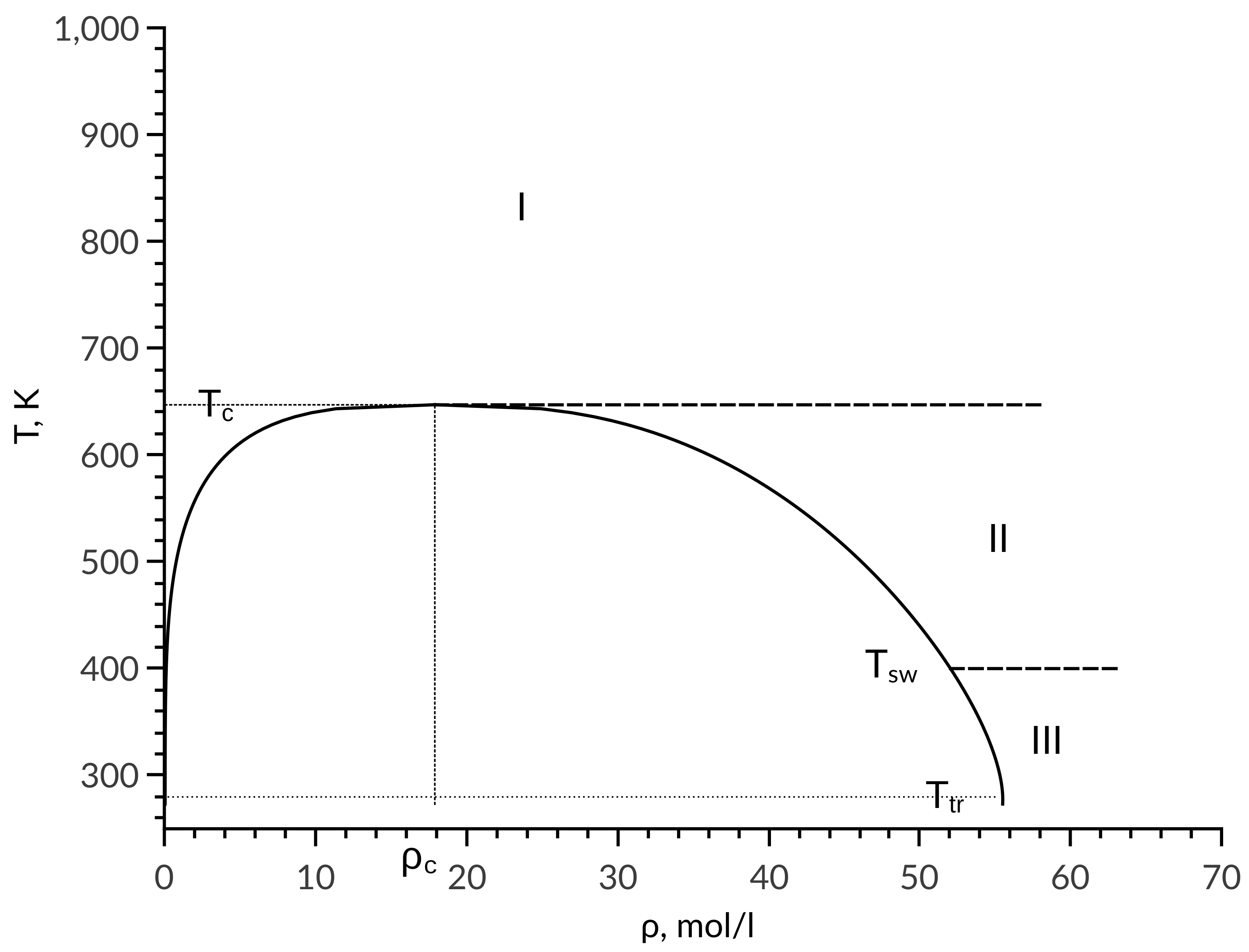
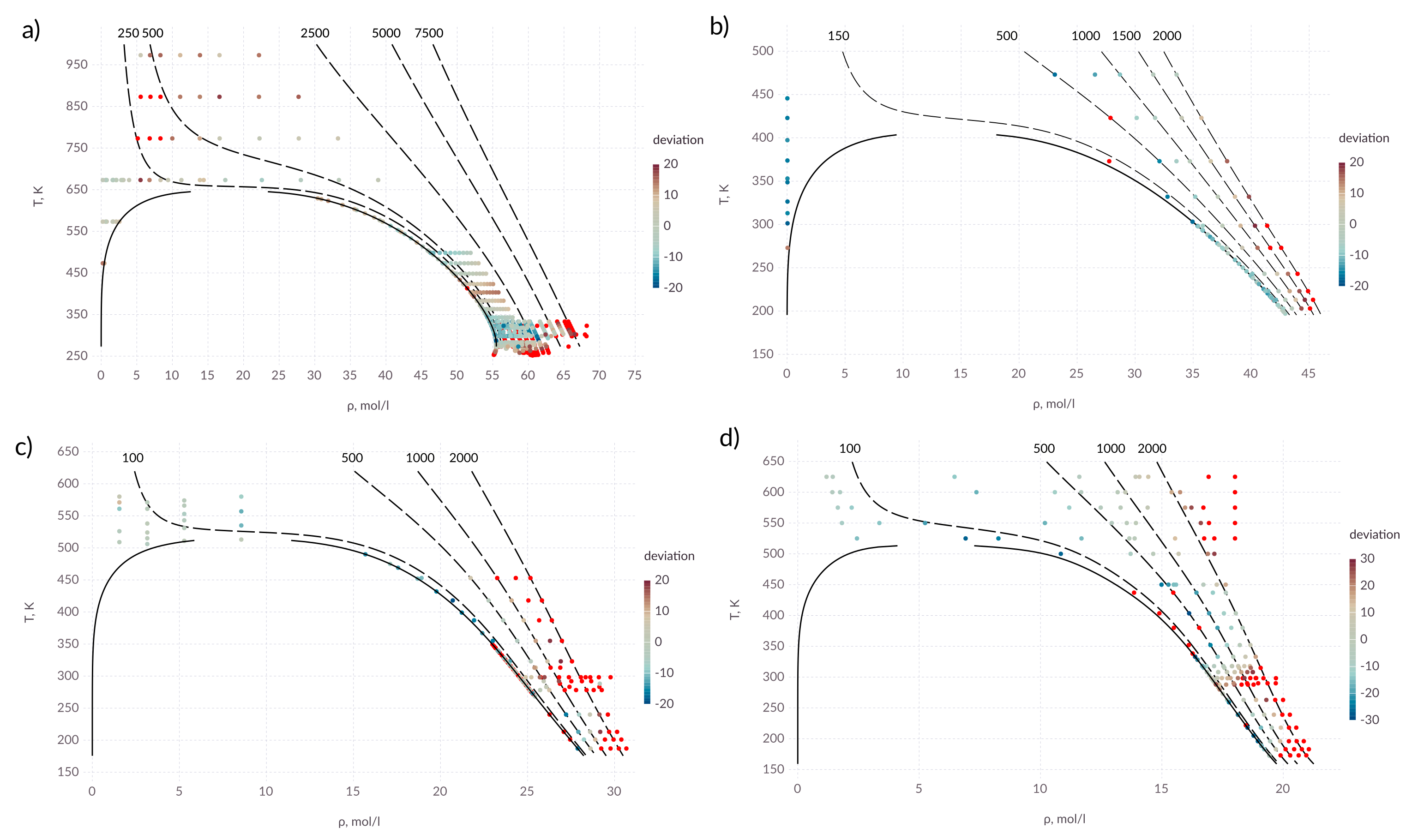

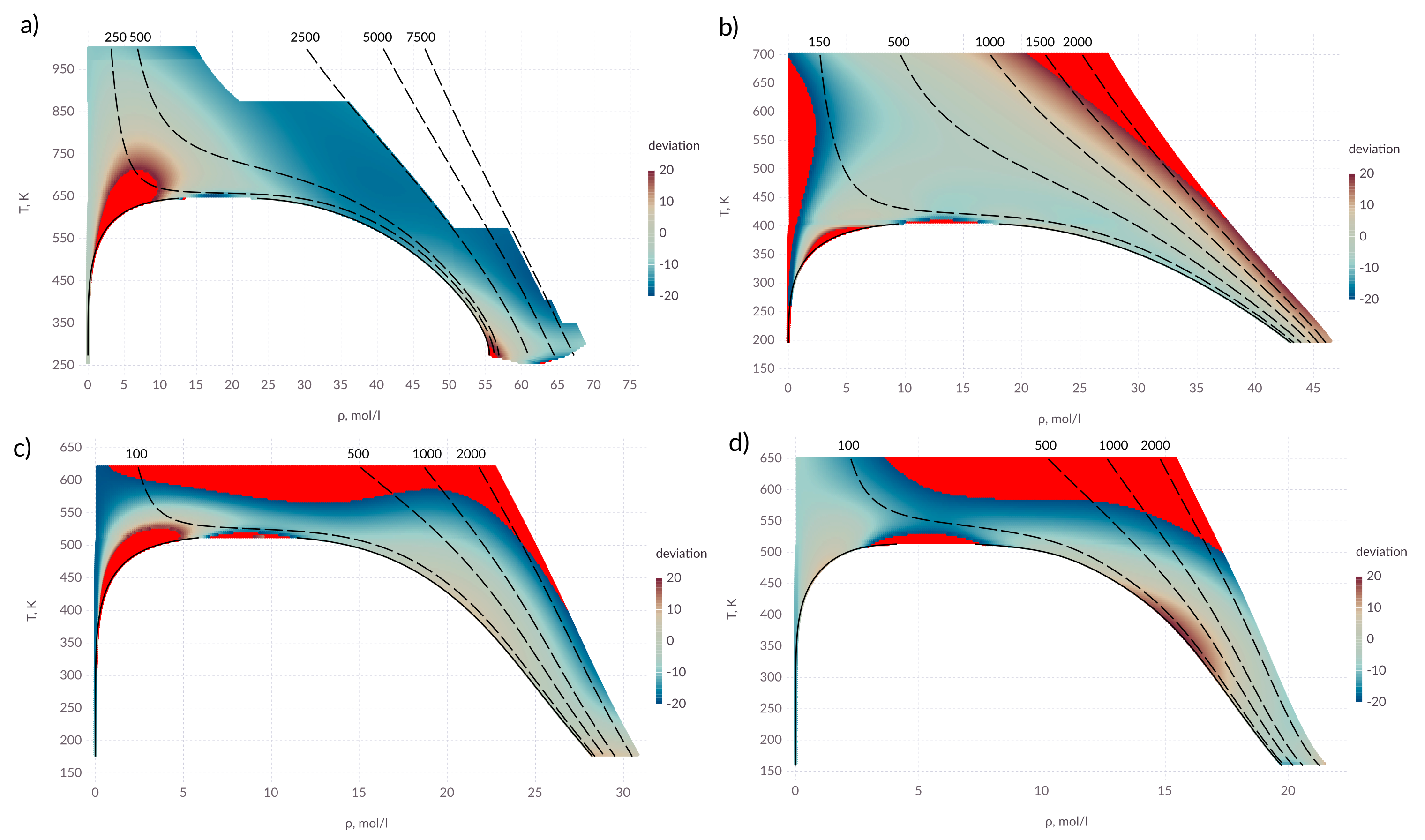
| Substance | , K | , nm | |
|---|---|---|---|
| water [35] | 470 | 0.259 | 1.5 |
| ammonia [36] | 309.9 | 0.3215 | 1.52 |
| ethanol [36] | 385.2 | 0.3657 | 1.1 |
| methanol [36] | 373.3 | 0.4299 | 0.855 |
| Substance | |||
|---|---|---|---|
| water | 0.0 | 0.2637 | 0.7621 |
| ammonia | 0.0388 | 0.3707 | 0.6264 |
| methanol | 0.03364 | 0.4212 | 0.8633 |
| ethanol | 0.1748 | 0.2804 | 0.8387 |
| Substance | |||
|---|---|---|---|
| water | −0.4019 | −0.1914 | −0.6052 |
| ammonia | −0.6809 | −0.151 | −0.3485 |
| methanol | −0.4132 | −0.2940 | −0.7253 |
| ethanol | −0.3881 | −0.2665 | −0.6644 |
| Substance | ||
|---|---|---|
| water | −0.1803 | −0.0006337 |
| ammonia | −0.3915 | −0.001032 |
| methanol | −0.344 | 0.0001427 |
| ethanol | −0.2914 | 0.0001376 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Anashkin, I.; Dyakonov, S.; Dyakonov, G. Relationship between the Transport Coefficients of Polar Substances and Entropy. Entropy 2020, 22, 13. https://doi.org/10.3390/e22010013
Anashkin I, Dyakonov S, Dyakonov G. Relationship between the Transport Coefficients of Polar Substances and Entropy. Entropy. 2020; 22(1):13. https://doi.org/10.3390/e22010013
Chicago/Turabian StyleAnashkin, Ivan, Sergey Dyakonov, and German Dyakonov. 2020. "Relationship between the Transport Coefficients of Polar Substances and Entropy" Entropy 22, no. 1: 13. https://doi.org/10.3390/e22010013
APA StyleAnashkin, I., Dyakonov, S., & Dyakonov, G. (2020). Relationship between the Transport Coefficients of Polar Substances and Entropy. Entropy, 22(1), 13. https://doi.org/10.3390/e22010013





