Finite-Time Synchronization of Markovian Jumping Complex Networks with Non-Identical Nodes and Impulsive Effects
Abstract
1. Introduction
- The article considers a class of Markovian jumping complex dynamic networks with non-identical nodes and impulsive effects. The system model is more comprehensive and closer to engineering practice. The finite-time method has such outstanding disturbance rejection capability that the results of this subject are of great significance.
- We propose a new one-norm-based Lyapunov function to solve the difficult points induced by non-identical nodes and impulsive effects. Also, we use the monotonicity to analyze the finite-time synchronization instead of the traditional theorem, and settling time can be theoretically estimated for a given network.
- Without drawing into any uncertain parameters, the finite time synchronization of dynamic systems is guaranteed by using the stochastic analysis technique, the M-matrix technique and some effective conditions.
2. Model Description and Preliminaries
- (1)
- All the eigenvalues of A have positive real parts.
- (2)
- A is a nonsingular Minkowski matrix (M-matrix).
- (3)
- exists and all the elments of are nonnegative.
3. Finite-Time Synchronization of the Complex Networks
- (1)
- when , andthe complex dynamic networks (3) can achieve synchronization with systems (2) in finite time, and the settling time is
- (2)
- when , the complex dynamic networks (3) is synchronized onto systems (2) in finite time, and the settling time is
- (3)
- when , the complex dynamic networks (3) is synchronized onto systems (2) within finite time, and the settling time iswhere , represents the Lipschitz coefficient of ith node, , , , defining has been referred to cursorily in Lemma 3, . is the initial value of the Markovian chain, .
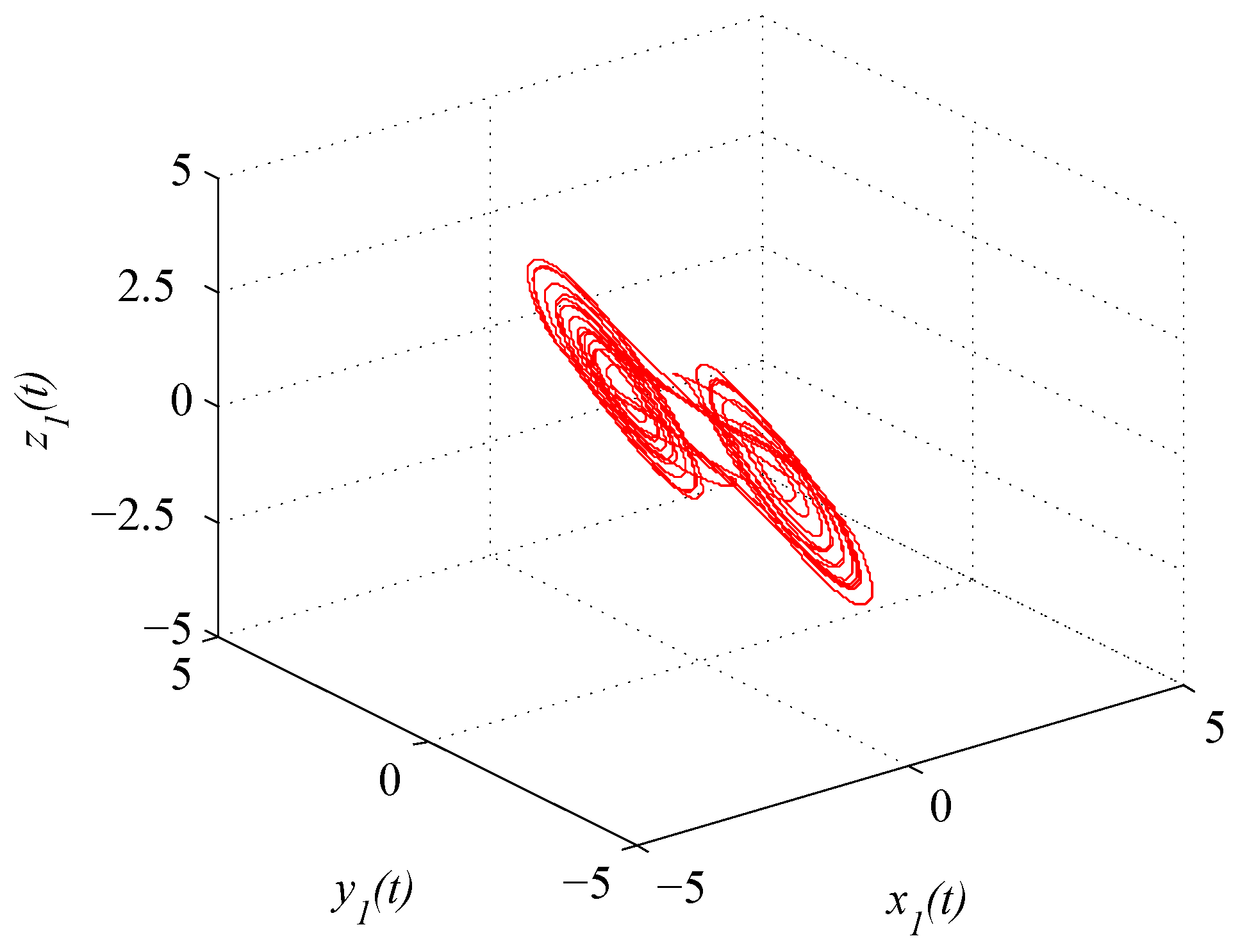
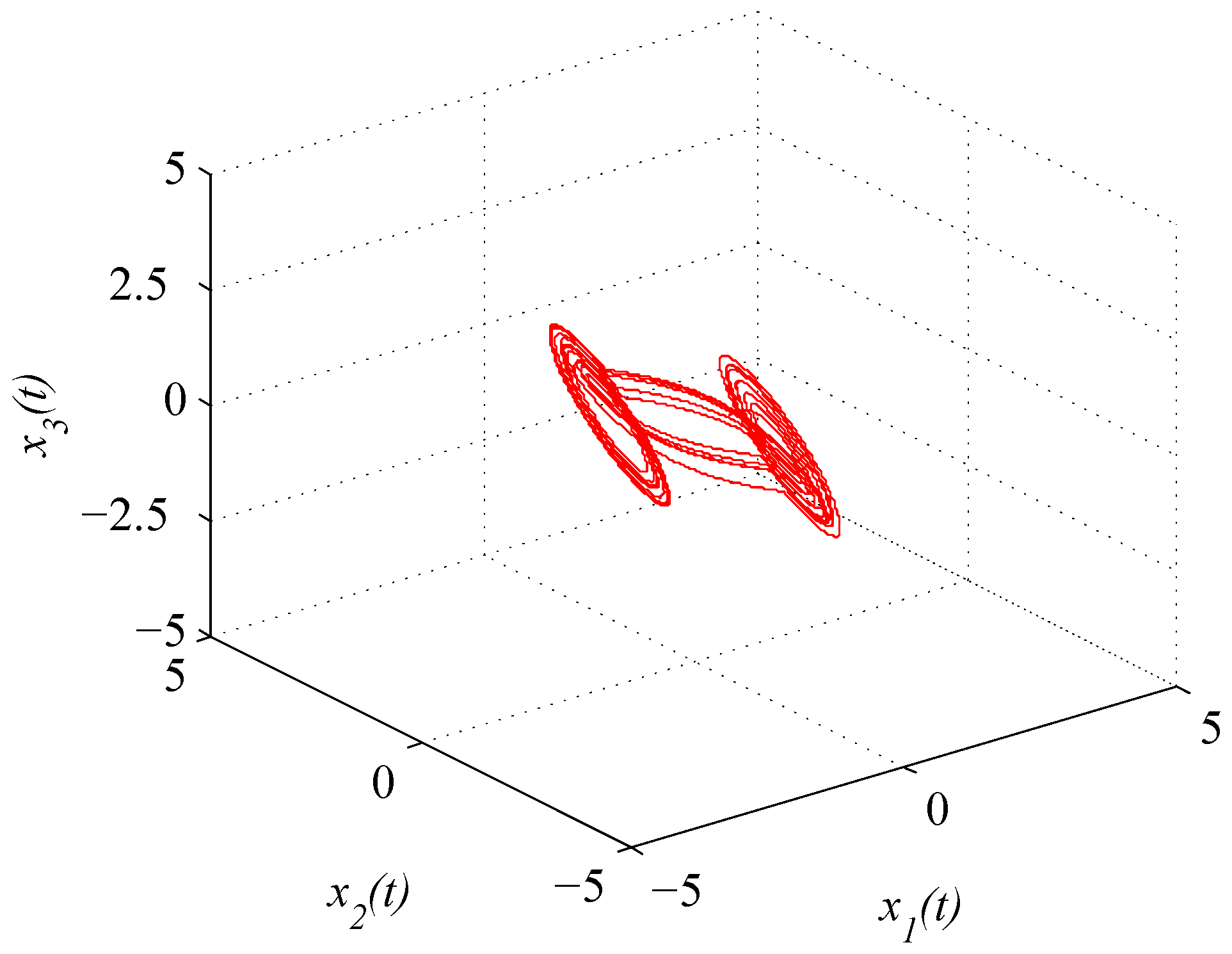
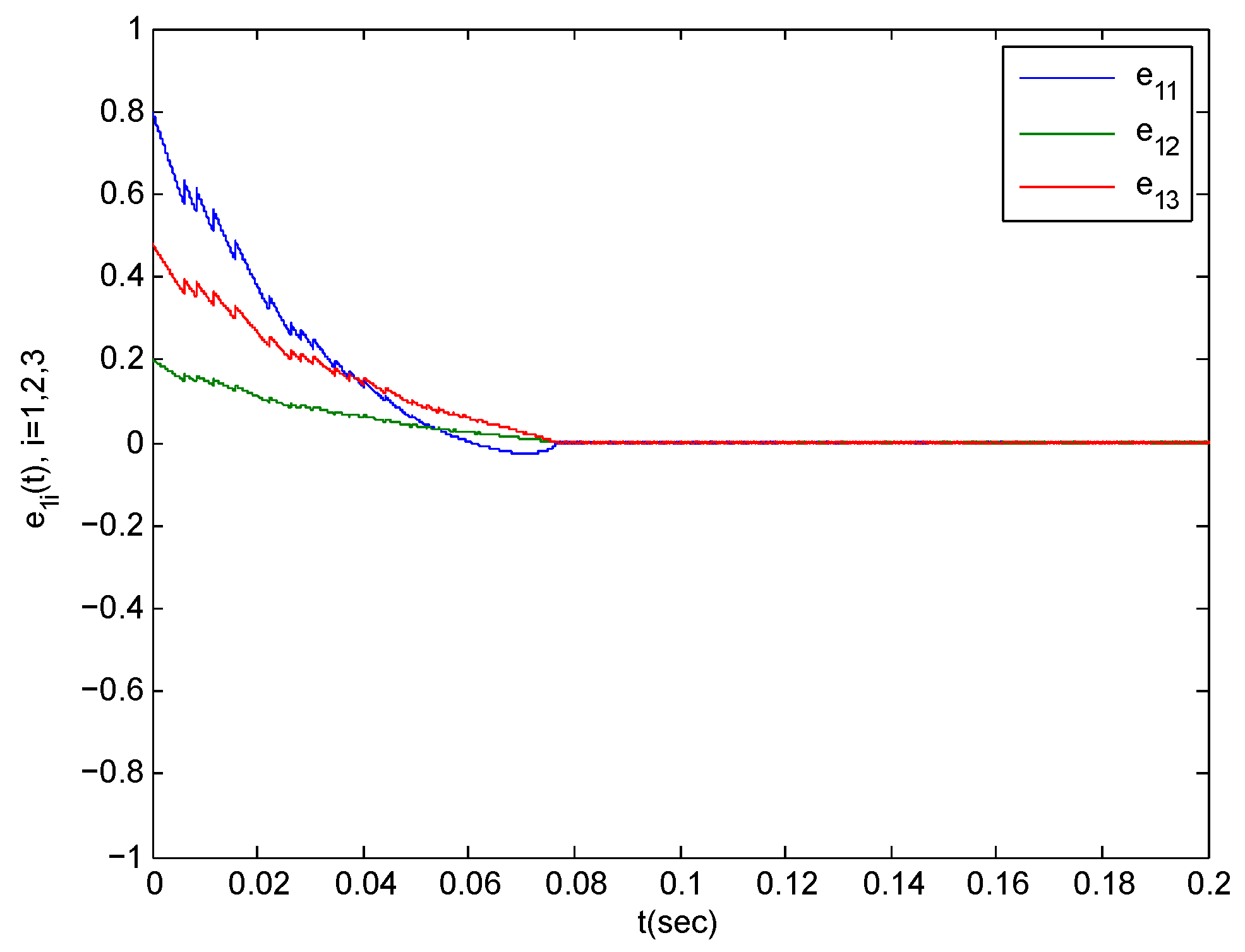
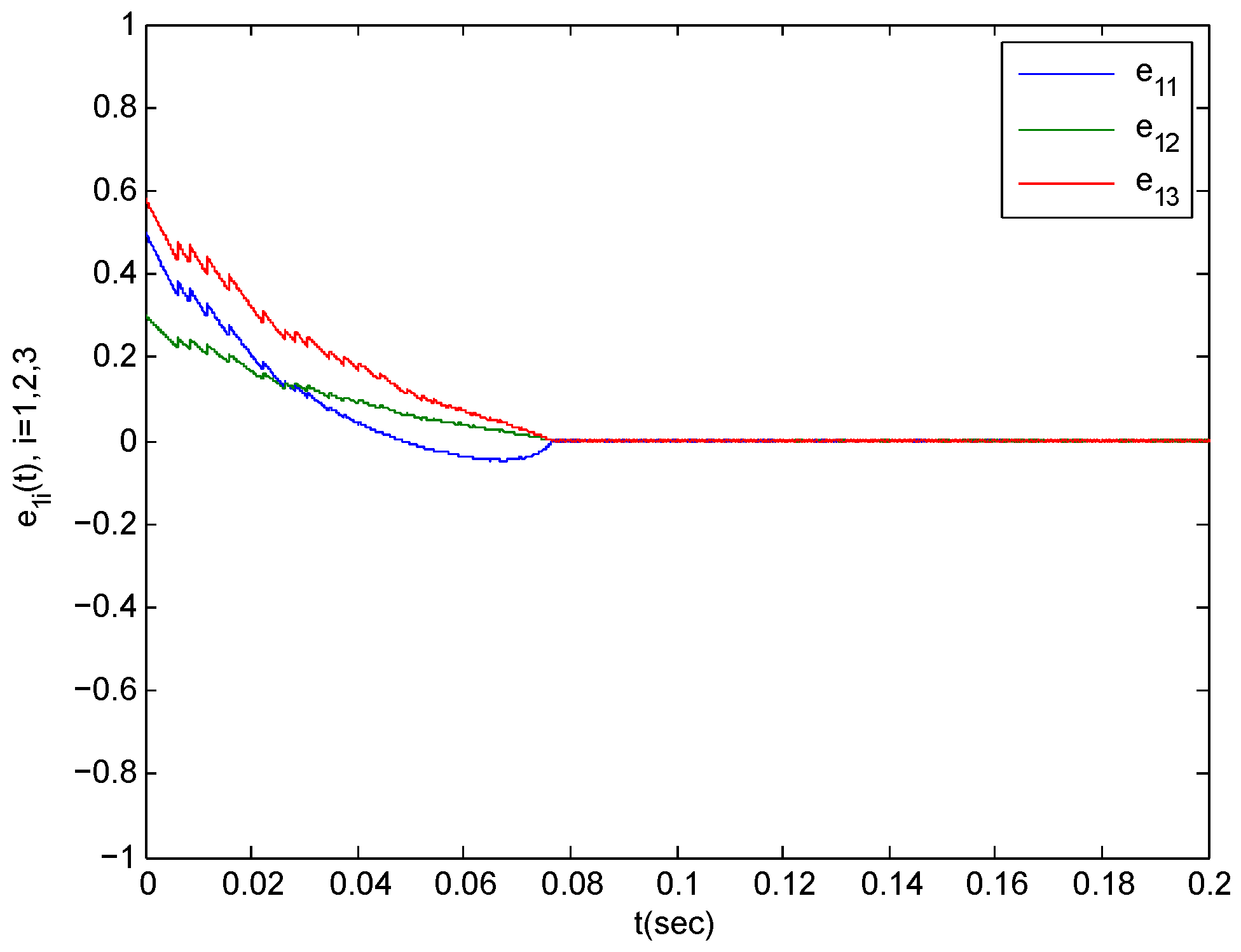
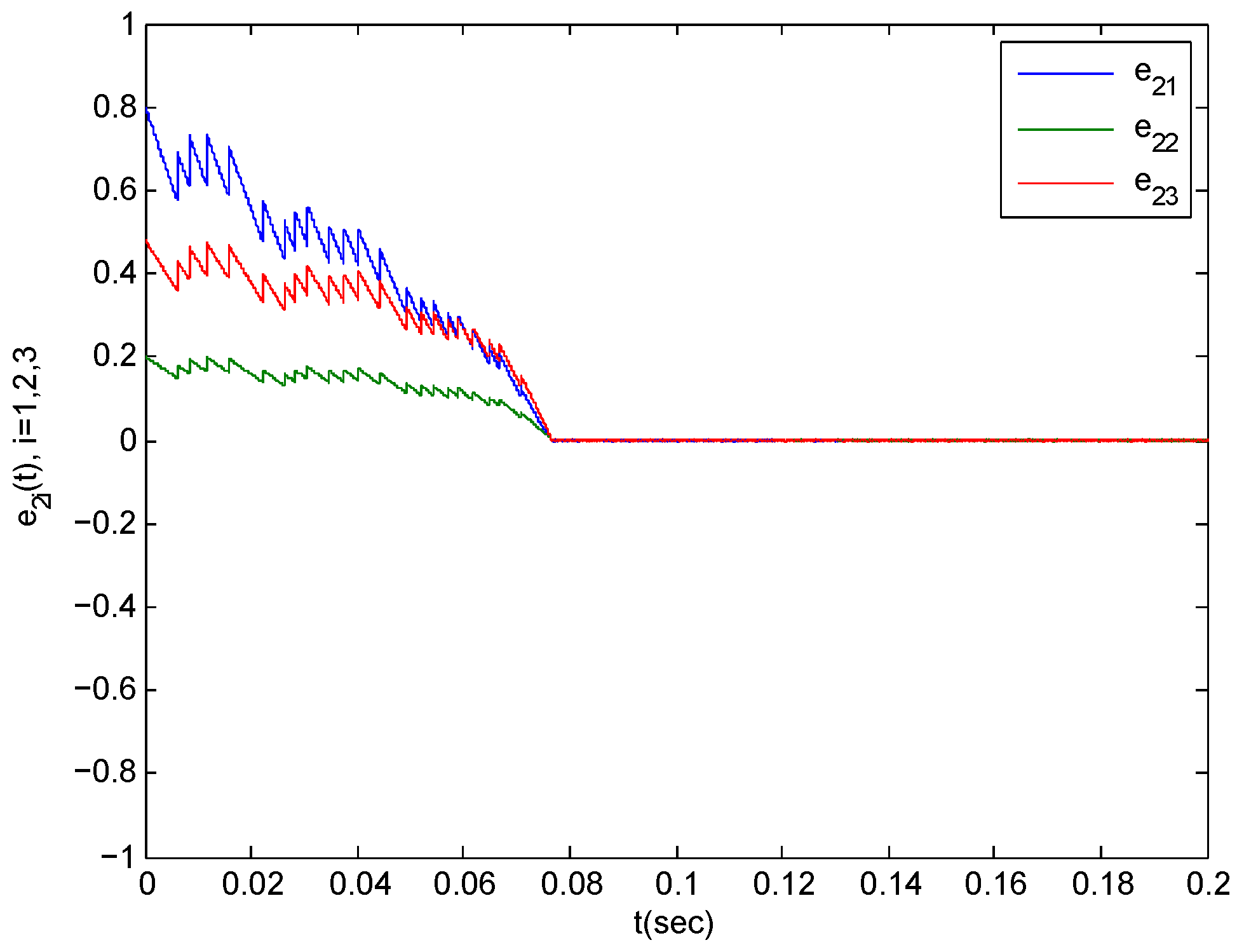
4. Numerical Examples
5. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Yang, T.; Feng, Q.; Gao, H.; Kurths, J. Synchronization in complex networks and its application—A survey of recent advances and challenges. Annu. Rev. Control 2014, 38, 184–198. [Google Scholar]
- Yu, W.; Chen, G.; Lü, J. On pinning synchronization of complex dynamical networks. Automatica 2009, 45, 429–435. [Google Scholar] [CrossRef]
- Stella, M.; De Domenico, M. Distance Entropy Cartography Characterises Centrality in Complex Networks. Entropy 2018, 20, 268. [Google Scholar] [CrossRef]
- Wu, X. Exponential Synchronization of Two Complex Dynamical Networks of Random Disturbance with Both Mixed Coupled and Time-Varying Delay by Pinning Control. Entropy 2015, 17, 6937–6953. [Google Scholar] [CrossRef]
- Ma, W.; Wu, Y.; Li, C. Pinning Synchronization between Two General Fractional Complex Dynamical Networks With External Disturbances. IEEE/CAA J. Autom. Sin. 2017, 4, 332–339. [Google Scholar] [CrossRef]
- Wang, J.L.; Wu, H.N.; Huang, T. Passivity-based synchronization of a class of complex dynamical networks with time-varying delay. Automatica 2015, 56, 105–112. [Google Scholar] [CrossRef]
- Ahmed, M.A.A.; Liu, Y.; Zhang, W.; Alsaadi, F.E. Exponential synchronization via pinning adaptive control for complex networks of networks with time delays. Neurocomputing 2017, 225, 198–204. [Google Scholar] [CrossRef]
- Yang, X. Can neural networks with arbitrary delays be finite-timely synchronized? Neurocomputing 2014, 143, 275–281. [Google Scholar] [CrossRef]
- Zhou, J.; Lu, J.A. Pinning adaptive synchronization of a general complex dynamical network. Automatica 2008, 44, 996–1003. [Google Scholar] [CrossRef]
- Khadra, A.; Liu, X.Z.; Shen, X. Analyzing the Robustness of Impulsive Synchronization Coupled by Linear Delayed Impulses. IEEE Trans. Autom. Control 2009, 54, 923–928. [Google Scholar] [CrossRef]
- Lu, J.; Chen, G. A Time-Varying Complex Dynamical Network Model And Its Controlled Synchronization Criteria. IEEE Trans. Autom. Control 2005, 50, 841–846. [Google Scholar]
- Yang, X.; Cao, J.; Lu, J. Synchronization of delayed complex dynamical networks with impulsive and stochastic effects. Nonlinear Anal. Real World Appl. 2011, 12, 2252–2266. [Google Scholar] [CrossRef]
- Liu, T.; Hill, D.J.; Zhao, J. Synchronization of Dynamical Networks by Network Control. IEEE Trans. Autom. Control 2012, 57, 1574–1580. [Google Scholar] [CrossRef]
- Yang, X.; Zhu, Q.; Huang, C. Generalized lag-synchronization of chaotic mix-delayed systems with uncertain parameters and unknown perturbations. Nonlinear Anal. Real World Appl. 2011, 12, 93–105. [Google Scholar] [CrossRef]
- Tan, W.; Jiang, F.; Huang, C.; Zhou, L. Synchronization for a Class of Fractional-Order Hyperchaotic System and Its Application. J. Appl. Math. 2012, 2012, 974639. [Google Scholar] [CrossRef]
- Perruquetti, W.; Floquet, T.; Moulay, E. Finite-Time Observers: Application to Secure Communication. IEEE Trans. Autom. Control 2008, 53, 356–360. [Google Scholar] [CrossRef]
- Li, L.; Jian, J. Finite-Time Synchronization of Chaotic Complex Networks with Stochastic Disturbance. Entropy 2014, 17, 39–51. [Google Scholar] [CrossRef]
- Aghababa, M.P.; Aghababa, H.P. Synchronization of mechanical horizontal platform systems in finite time. Appl. Math. Model. 2012, 36, 4579–4591. [Google Scholar] [CrossRef]
- Yang, X.; Cao, J. Finite-time stochastic synchronization of complex networks. Appl. Math. Model. 2010, 34, 3631–3641. [Google Scholar] [CrossRef]
- Khadra, A.; Liu, X.; Shen, X. Application of impulsive synchronization to communication security. IEEE Trans. Circuits Syst. I Fundam. Theory Appl. 2003, 50, 341–351. [Google Scholar] [CrossRef]
- Aghababa, M.P.; Khanmohammadi, S.; Alizadeh, G. Finite-time synchronization of two different chaotic systems with unknown parameters via sliding mode technique. Appl. Math. Model. 2011, 35, 3080–3091. [Google Scholar] [CrossRef]
- Vincent, U.E.; Guo, R. Finite-time synchronization for a class of chaotic and hyperchaotic systems via adaptive feedback controller. Phys. Lett. A 2011, 375, 2322–2326. [Google Scholar] [CrossRef]
- Lu, J.; Ho, D.W.C.; Cao, J. A unified synchronization criterion for impulsive dynamical networks. Automatica 2010, 46, 1215–1221. [Google Scholar] [CrossRef]
- Yang, X.; Huang, C.; Zou, X. Effect of impulsive controls in a model system for age-structured population over a patchy environment. J. Math. Biol. 2018, 76, 1387–1419. [Google Scholar] [CrossRef] [PubMed]
- Wu, Y.; Fu, S.; Li, W. Exponential synchronization for coupled complex networks with time-varying delays and stochastic perturbations via impulsive control. J. Frankl. Inst. 2019, 356, 492–513. [Google Scholar] [CrossRef]
- Jianquan, L.; Ho, D.W.C.; Jinde, C.; Jürgen, K. Exponential synchronization of linearly coupled neural networks with impulsive disturbances. IEEE Trans. Neural Netw. 2011, 22, 329–336. [Google Scholar]
- Ren, H.; Deng, F.; Peng, Y. Finite time synchronization of Markovian jumping stochastic complex dynamical systems with mix delays via hybrid control strategy. Neurocomputing 2018, 22, 683–693. [Google Scholar] [CrossRef]
- Wang, X.; Guo, Y. H-infinity Control for Markov Jump Systems with Nonlinear Noise Intensity Function and Uncertain Transition Rates. Entropy 2015, 17, 4762–4774. [Google Scholar] [CrossRef]
- Yurong, L.; Zidong, W.; Jinling, L.; Xiaohui, L. Stability and synchronization of discrete-time Markovian jumping neural networks with mixed mode-dependent time delays. IEEE Trans. Neural Netw. 2009, 20, 1102–1116. [Google Scholar] [CrossRef]
- Li, Z.X.; Ju, H.P.; Wu, Z.G. Synchronization of complex networks with nonhomogeneous Markov jump topology. Nonlinear Dyn. 2013, 74, 65–75. [Google Scholar] [CrossRef]
- Yang, X.; Cao, J.; Lu, J. Synchronization of Randomly Coupled Neural Networks with Markovian Jumping and Time-Delay. IEEE Trans. Circuits Syst. I Regul. Pap. 2013, 60, 363–376. [Google Scholar] [CrossRef]
- Zhu, J.; Ding, Q.; Spiryagin, M.; Xie, W. State and mode feedback control for discrete-time Markovian jump linear systems with controllable MTPM. IEEE/CAA J. Autom. Sin. 2019, 6, 830–837. [Google Scholar] [CrossRef]
- Zhang, W.; Li, C.; He, X.; Li, H. Finite-time synchronization of complex networks with non-identical nodes and impulsive disturbances. Mod. Phys. Lett. B 2018, 32, 1850002. [Google Scholar] [CrossRef]
- Yu, T.; Cao, D.; Yang, Y.; Liu, S.; Huang, W. Robust synchronization of impulsively coupled complex dynamical network with delayed nonidentical nodes. Chaos Solitons Fractals 2016, 87, 92–101. [Google Scholar] [CrossRef]
- Zhao, J.; Hill, D.J.; Liu, T. Synchronization of Dynamical Networks with Nonidentical Nodes: Criteria and Control. IEEE Trans. Circuits Syst. I Regul. Pap. 2011, 58, 584–594. [Google Scholar] [CrossRef]
- Yang, X.; Wu, Z.; Cao, J. Finite-time synchronization of complex networks with nonidentical discontinuous nodes. Nonlinear Dyn. 2013, 73, 2313–2327. [Google Scholar] [CrossRef]
- Yang, X.; Lu, J. Finite-Time Synchronization of Coupled Networks with Markovian Topology and Impulsive Effects. IEEE Trans. Autom. Control 2016, 61, 2256–2261. [Google Scholar] [CrossRef]
- Horn, R.A.; Johnson, C.R. Topics in Matrix Analysis; Cambridge University Press: Cambridge, UK, 1994. [Google Scholar]


© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, T.; Peng, S.; Zhang, Z. Finite-Time Synchronization of Markovian Jumping Complex Networks with Non-Identical Nodes and Impulsive Effects. Entropy 2019, 21, 779. https://doi.org/10.3390/e21080779
Chen T, Peng S, Zhang Z. Finite-Time Synchronization of Markovian Jumping Complex Networks with Non-Identical Nodes and Impulsive Effects. Entropy. 2019; 21(8):779. https://doi.org/10.3390/e21080779
Chicago/Turabian StyleChen, Tao, Shiguo Peng, and Zhenhua Zhang. 2019. "Finite-Time Synchronization of Markovian Jumping Complex Networks with Non-Identical Nodes and Impulsive Effects" Entropy 21, no. 8: 779. https://doi.org/10.3390/e21080779
APA StyleChen, T., Peng, S., & Zhang, Z. (2019). Finite-Time Synchronization of Markovian Jumping Complex Networks with Non-Identical Nodes and Impulsive Effects. Entropy, 21(8), 779. https://doi.org/10.3390/e21080779




