Chaotic entanglement demonstrates a new way for chaotic systems to interact and thus signals a new parallel between quantum and classical mechanics. We now further explore this connection by discussing other properties of chaotic systems that quantum systems also exhibit. We also address several concerns that invariably arise when examining entangled quantum systems and how these concerns relate to chaotic systems. Key to our discussion is the important role that the cupolets and UPOs of chaotic systems play in determining the dynamical properties of chaotic systems.
4.1. Hilbert Space Considerations
Formulating a Hilbert space of states is taken as a starting point in many quantum studies. This allows one to express an associated wave function as a linear combination of orthonormal state vectors that satisfies the Schrödinger equation. For instance, one way to formulate the Hilbert space of a quantum system is via the Fourier modes of the system: one can assemble linear combinations of sinusoids in order to define the state vectors as is done with the infinite square well [
40].
Constructing a Hilbert space on a chaotic system is not as straightforward because the governing equations are nonlinear and prevent linear combinations of states from also being solutions. Cupolets are highly-accurate approximations to the periodic solutions of chaotic systems, and so one could designate cupolets (e.g., UPOs) as the state vectors, except that cupolets and UPOs do not satisfy any simple orthogonality principles. Moreover, chaotic systems generally admit a countably infinite number of these periodic orbits on their attractors [
41], and so cupolets and UPOs would form an overdetermined set of basis elements. In fact, the Fourier spectra obtained from any large collection of cupolets is also overdetermined: although the simplest cupolets consist of just one or two spectral peaks, the higher period cupolets exhibit tens or hundreds of significant peaks in their spectra [
15,
16].
However, cupolets and UPOs are still regarded as the states of chaotic systems, even if superpositions of these orbits are unable to satisfy the underlying equations. This is because ergodicity guarantees that a free-running chaotic system ultimately realizes all possible non-equilibrium states and visits arbitrarily small neighborhoods of its periodic solutions infinitely often. Even though chaotic systems evolve aperiodically for all time, the dynamics of these systems are ultimately confined to their attractors, which means that a wandering chaotic trajectory undergoes a series of close encounters with the embedded UPOs and cupolets.
Furthermore, many characteristics of chaotic systems, such as Lyapunov exponents, the natural measure, dimension, topological entropy, and several orbit expansions, can all be expressed in terms of UPOs [
14,
17,
42,
43,
44]. In particular, the natural measure, which is roughly interpreted as the probability of a chaotic system visiting a given region of its attactor over time, is often described as being concentrated on the UPOs. In other words, as it evolves, a chaotic system visits regions populated by UPOs with greater frequency and will dwell alongside an UPO for an extended amount of time after which the trajectory begins shadowing other UPOs. Since UPOs are solutions to the governing differential equations, uniqueness properties imply that these orbits cannot be crossed in phase space. This is easier to visualize in many low-dimensional chaotic systems, such as the double scroll, Lorenz, and Rössler systems, where the attractors are locally ribbon-like in at least part of their domains. Cupolets are generated in such a way that uniqueness considerations also apply, except possibly at certain locations along a control plane where the controls are applied [
29]. Chaotic trajectories are thus restricted to evolving along unique paths that are locally bounded by UPOs and cupolets, which means that the dynamics of chaotic systems are locally dependent on these orbits.
4.2. Functional Representation of Cupolets
While it is not straightforward to establish a superposition of Hilbert space basis elements for nonlinear dynamical systems, the periodic nature of cupolets does allow for functional representations of these orbits to be derived and used as (approximate) solutions to the nonlinear differential equations. This results in a low complexity approximation of the UPO solutions of the nonlinear differential equations. To demonstrate this, we will now derive the functional form of two cupolets and then show how well the functional form approximates the corresponding true periodic orbits obtained through numerical integration.
Since cupolets are periodic over the attractor, they play the role of eigenfunctions for the differential equations, and because of their periodicity, the Fourier decompositions of cupolets converge rapidly. Hence, one can use the Fourier representation of a cupolet—itself a finite dimensional expansion over a discrete Hilbert space—to create a functional form that can be used in symbolic computational systems like Mathematica (Version 11.3, Wolfram Research: Champaign, IL, USA). Furthermore, one can look at the fast Fourier transform (FFT) of sampled cupolet data to determine which Fourier coefficients are significant, and then truncate the representation so that only the significant Fourier modes are retained. As we demonstrate below, the functional form of a cupolet compares favorably to its numerical solution which is obtained directly from the uncontrolled differential equations of the double scroll system.
In order to create a functional representation of a cupolet, the time domain data from the numerical simulation of the cupolet is preemptively stored in a vector of 1024 samples. A cupolet’s period,
T, in simulated time varies among the cupolets, and this value needs to be initially recorded as well. The numerical integration of the system must be carefully managed so as to maintain accurate time steps even as the system passes through the control planes and is subjected to the perturbations of the control scheme described in
Section 2 [
22]. Even so, the cupolets are often extremely close to the true UPOs of the system. The vector of samples is then passed through the FFT to create a vector of
frequency components. Of these components, one is a constant term, 511 are designated as “positive spinning” components, 511 are designated as “negative spinning” components, and one is associated with the Nyquist frequency that is neither positive- nor negative-spinning. The term “negative spinning” simply means that the complex sinusoids are sampled by moving in the negative angle (e.g., clockwise direction) around the complex unit circle.
The derivation of the functional representation of a cupolet proceeds as follows. We let the vector of 1024 samples of the cupolet be represented as
, with individual entries designated
, and the corresponding frequency domain coefficients as
. The initial FFT is calculated as
where
[
45]. Note that the Nyquist frequency coefficient is
. It is now useful to relabel the
f index because half of these coefficients are negative and reflect the “negative spinning” aspect of the complex sinusoids. The relabeling is done for all indices
, in which case
. Now that the negative indices represent negative spinning oscillators, they can be grouped with the correspondingly-labeled positive spinning oscillators in complex conjugate pairs. Under this relabeling, the original sampled values can each be recovered exactly from Equation (
2) via the inverse FFT calculation:
It is the inverse form of the FFT that allows for the conversion from discrete form to functional form, since each index
f corresponds to an integer period complex sinusoid taken over the cupolet period
T. Each
W term in the sum corresponds to a discretely-sampled complex exponential, which has now been converted to a continuous-time complex exponential function. If we let
be a placeholder for the continuous time component, we can take advantage of the complex conjugate pairing of the complex sinusoids and the corresponding coefficients
and
in order to obtain a (real) functional form
, since the imaginary parts drop out. Next, we make explicit the integer period nature of the sinusoids and the period of the cupolet
T by setting
, where
t represents continuous time. Consequently, we have defined the complex sinusoids to be periodic over a continuous time variable that naturally encodes both the period of the cupolet and the exact integer periods of the Fourier representation. This results in the (full) functional form of a given cupolet:
Equation (
4) can also be expressed in an equivalent form that shows the complex conjugate pairing along with the constant term and the (real) Nyquist term:
Once a cupolet’s functional form has been created, it can be used in a software package like Mathematica that allows for symbolic manipulation of mathematical equations. In addition, since many of the cupolets have rapidly decaying magnitudes for the Fourier/FFT coefficients, it is possible to keep only a subset of the coefficients in order to get a convenient functional form. In the examples presented below, we have
, so there are 511 positive and negative frequency components (plus the constant and Nyquist terms). We can truncate the representation in Equation (
4) to retain only
Q-many components, where
, giving
and in the examples below we will take
and
.
To utilize this representation, the Mathematica software package can be used to compare the functional representation of the dynamical variables with the Mathematica numerical solution of the uncontrolled double scroll equations.
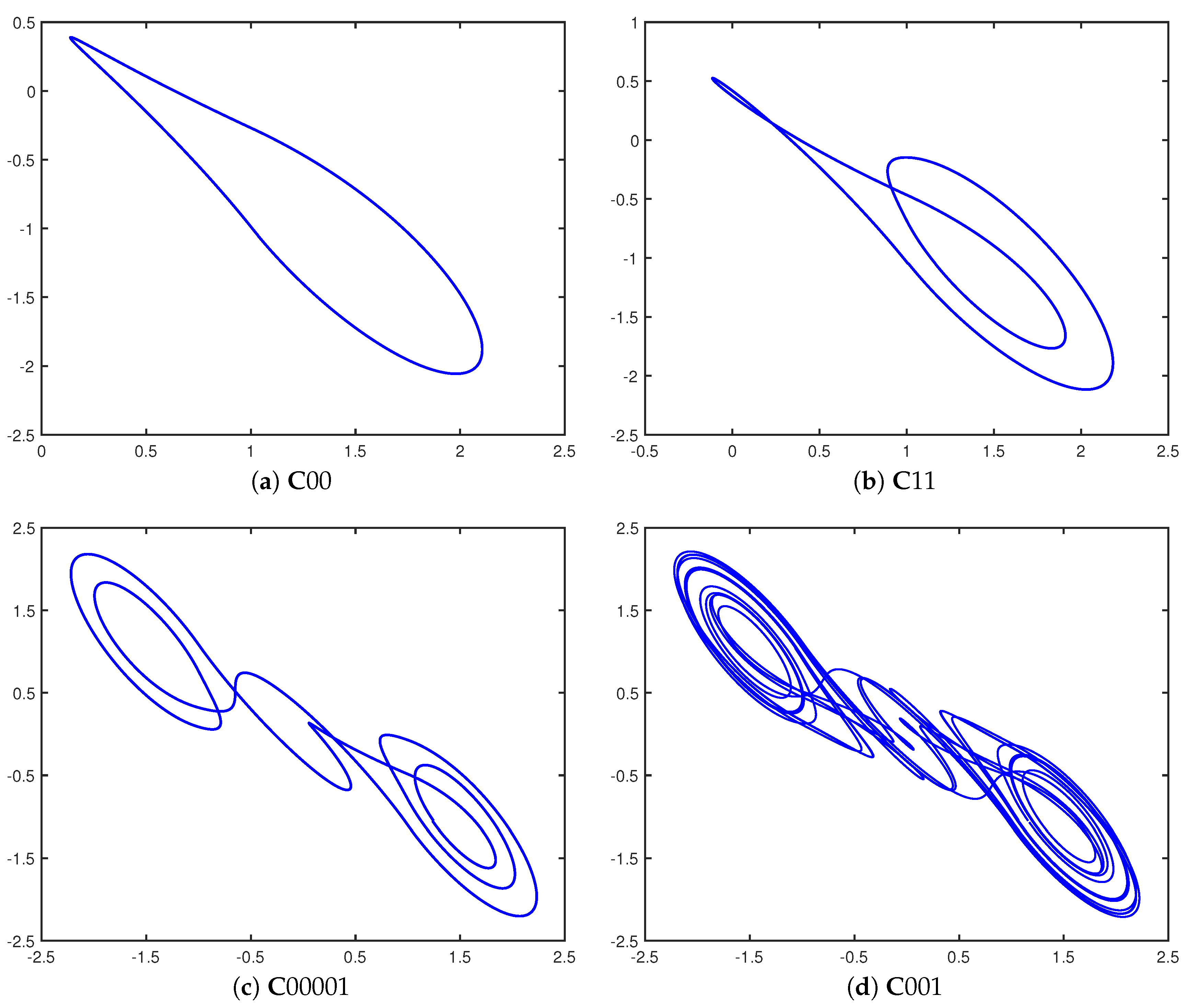
Figure 5 shows the comparisons between the numerical solution and the full and truncated functional forms of two cupolets. The first cupolet is the simplest of all, cupolet
, with the truncated version using
coefficients. The second example uses the 5-loop cupolet
, and the truncated version uses
. In each case, the magnitude of the Fourier coefficients has diminished by over two orders of magnitude at the point where the series is truncated.
Figure 5 also depicts the comparison between the
-component of the numerical solution and the corresponding truncated functional form for these cupolets. In all of these figures, the numerical data appear to be superimposed with the data obtained from the cupolets’ functional forms. This is because of how closely the functional forms approximate the cupolet’s true periodic orbit. Note that the orbits of these two cupolets have been seen previously in
Figure 2.
While the cupolets provide useful approximations of UPO solutions of the nonlinear chaotic differential equations, there are still perturbations to a natural orbit from the controls applied on the control plane, so when there are larger perturbations, the fit to the uncontrolled solution will not be as close. Even so, a truncated cupolet expansion would provide a good starting point for a perturbative solution method, such as an improved Poincaré–Lindstedt solution, or a similar approach to developing a higher accuracy solution. In conclusion, while there is not a natural generic Hilbert space basis that satisfies the nonlinear dynamical equations of chaos, it is possible to use related techniques to develop functional approximations for the cupolets and UPOs that satisfy the constraint of being restricted to the chaotic attractor while also providing an approximate periodic solution.
4.3. Superposition of States
To represent the state of a given chaotic system as a superposition of cupolet states, let
denote the state space coordinates of the system’s
cupolet at time
, where
. The state of the chaotic system,
, can then be expressed as a weighted sum of its cupolets:
where each weight,
, represents the contribution to
from cupolet
at time
t with respect to the natural measure c.f., [
17,
43]. As the chaotic system evolves in time, each
varies according to the proximity of the system to that cupolet. A chaotic system’s
state vector,
, is thereby formulated by collecting the weights of each cupolet:
The set of
will have local compact support because the cupolets that provide a nonzero contribution to the overall state of the system are those that are found within a local neighborhood of the current state of the system, whereas cupolets located farther away will contribute negligibly. In other words, when the system is dwelling near its
kth cupolet, then
because at this moment
and
for all
. Similarly, as the chaotic system deviates away from the
kth cupolet, the dynamics are well approximated by nearby cupolets, say
,
, and
, while
for more distant cupolets. To carry out an explicit calculation along these lines, there are several options. One can select a point on the attractor and then select segments of neighboring cupolets and use them to construct a model of the local dynamics [
46,
47], or one can adopt an approach like that of matching pursuit [
48] and use the set of cupolets as a dictionary of states. Future work may compare a variety of methods of determining
for a set or subset of cupolets.
In quantum mechanics, the wave function is of fundamental importance since it provides a probabilistic description of the state of a quantum system. The analog for a chaotic system is its state vector, , which provides a complete and evolving description for the state of a chaotic system in terms of its cupolets (or equivalently, its UPOs). In this way, a freely evolving chaotic system is viewed as evolving in a “mixed state” that is a superposition of cupolet states. In a mixed state, the contributions to the associated state vector come primarily from the cupolets in between which the chaotic trajectory is evolving and is nearest to at that moment.
4.4. Wave Function Collapse
Another fundamental concept in quantum mechanics is the idea that making a measurement induces the collapse of a quantum system’s associated wave function onto a specific state. Prior to the disturbance, the wave function is suspended in a superposition of state vectors, which inhibits the quantum system from being unambiguously described.
Similar behavior is supported by chaotic systems. When controls are repetitively applied to a chaotic system, cupolets form because of two key properties: the system stabilizes uniquely onto a periodic orbit under the influence of a set of repeating perturbations, and this stabilization occurs independently of initial conditions. These properties allow a chaotic system to be collapsed onto a specific cupolet from any initial state. The repeated action of the controls acts as the measurement process that induces wave function collapse. This occurs precisely when the chaotic system stabilizes onto a cupolet, say
. Via Equation (
7), when this happens,
and
for all
, which gives
as expected. The state vector given by Equation (
8) reduces as well to
, whose only nonzero component is its
. Until the collapse occurs, a chaotic system cannot be definitively described as a single cupolet state because it is instead locally dependent on a superposition of cupolet states.
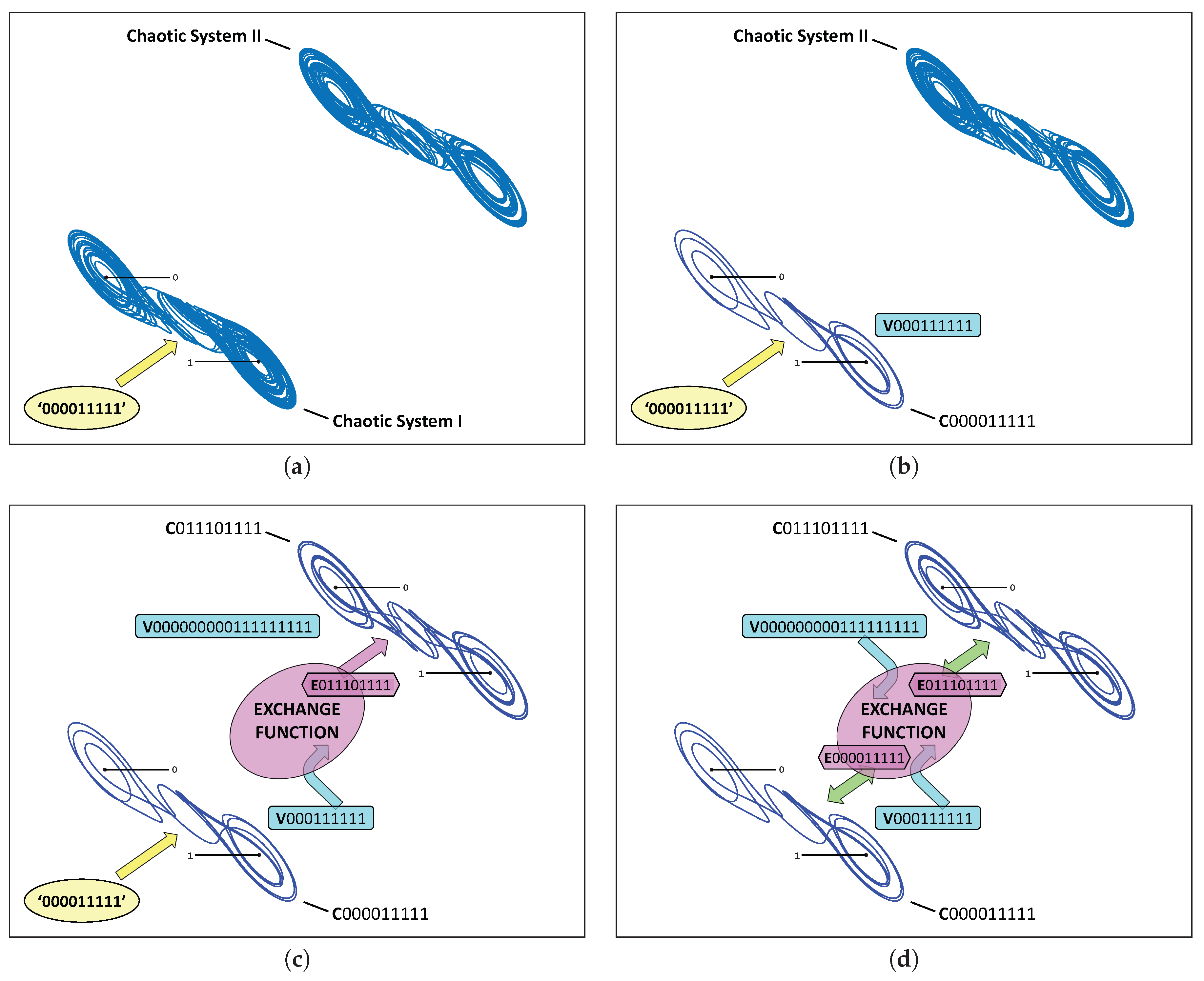
It is important to stress that the interactions between chaotic systems that support chaotic entanglement would be such that the interaction could have the same effect as a measurement, so that the system in a chaotic state would collapse onto a periodic cupolet state. Thus, in chaotic entanglement, it may be fair to say that interaction equals measurement.
4.5. Natural Chaotic Entanglement
In chaotic systems, the concepts of measurement and state vector collapse are not only induced by external measurements or user-implemented controls, but are able to arise naturally in chaotic entanglement. Because their periodic orbits are unstable, isolated chaotic systems evolve aperiodically, yet a chaotic system tends to dwell significantly longer on its UPOs than on any other states or regions of phase space. By extension, an ensemble of independent chaotic systems would also each be dwelling along their UPOs and cupolets infinitely often. If one of these chaotic systems happens to dwell on a cupolet that exhibits the ability to entangle, and that can also communicate control information to a second nearby chaotic system, and if this interaction is as successful in the reverse direction, then the two interacting systems would entangle naturally.
In the context of two arbitrary cupolets,
and
, this situation implies that the parent system of cupolet
will approach and dwell on
infinitely often. If a second chaotic system is at the same time dwelling near
, then entanglement would form naturally between the two systems, provided that the symbolic dynamics of the cupolets can be used to maintain their periodic behavior. In this way, isolated and independently-evolving chaotic systems would be perturbing each other with the interactions themselves playing the role of the controls or measurements. This makes it possible for entanglement to occur naturally, as has been emphasized both in
Section 3.3 and in the recent studies of macroscopic systems examined in [
37,
38,
39]. As we discuss below, the potential for natural chaotic entanglement plays a key role in the interpretation of making measurements on individual members of entangled cupolet pairs.
4.6. Measurement Problem
It is first worthwhile to compare the effects of a knowledgeable measurement on a chaotic system, as opposed to a blind measurement. For instance, if one has both knowledge of the control scheme and access to measurement tools that are smaller than the scale of the control bins, then one could monitor the state of a chaotic system without disturbing its trajectory. That is, one could design a measurement whose effects would not be strong enough to perturb an evolving cupolet to a new bin center on a control plane. The slight deviation from the original orbit could be small enough to be corrected the next time the cupolet intersects a control plane via the implementation of the microcontrols. This we define as a knowledgeable measurement because it permits one to not only study a cupolet without compromising its stability, but to also probe two entangled systems without compromising their entanglement.
If a measurement is not implemented as carefully, the repercussions would be more pronounced. Consider the effects of the measurement described earlier in
Section 3.2, whereby a single ‘1’ control bit in a given cupolet’s control sequence is altered to a ‘0’ control bit. Such a disturbance would destabilize the cupolet and cause the parent system to either revert to chaotic behavior or to stabilize again after a potentially long transient period. This disturbance is known as a blind measurement, and it would cause the destablized orbit to begin generating a new visitation sequence. Had this cupolet been entangled with another cupolet, then the effects of the blind measurement would transfer to the partner cupolet by way of the exchange function. This is because the exchange function would begin producing a different emitted sequence that no longer matches the control sequence required to maintain the stability of the partner cupolet. The cupolets’ entanglement would then be lost.
Regarding the measurement problem, consider the situation in which a pair of cupolets has entangled, either through the deliberate preparation of an entangled state, or naturally through pure entanglement. If a knowledgeable measurement is conducted on one member of the entangled pair, then the state of the other member would be known with certainty (with the proviso that we have only found unique pairings at this point). Should the measurement process involve blind measurements, then the disrupted communication between the members of the entangled pair would induce the two parent systems to begin evolving independently. Similarly, if the interaction between members of an entangled pair is limited by distance, and if the entangled cupolets become too far separated, then their entanglement would decay as their communication wanes. This decay would not necessarily be very rapid, but would be determined by the local Lyapunov exponents of the two cupolets [
14]. In these situations, the history of the previous entanglement would not be immediately erased because a measurement conducted on one member of the entangled pair would be predictive of the state of the second system, although the accuracy of the prediction would diminish over time.
In contrast, the principles of quantum mechanics dictate that making any measurement on a system immediately alters its state. This is problematic for researchers for whom knowing the actual state of a quantum system is important [
49,
50]. As indicated by Isham,
“… quantum theory encounters questions that need to be answered, one of the most important of which is what it means to say, and how it can be ensured that the individual systems on which the repeated measurements are to be made are all in the ‘same’ state immediately before the measurement. This crucial problem of state preparation is closely related to the idea of a reduction of the state vector.”
When combined with knowledgeable measurements, the cupolet-stabilizing control scheme could aid in state preparation for experiments. Cupolets are generated regardless of the current or initial state of the system, which means that if chaotic control methods are designed to stabilize cupolets from physical systems, then two systems could be synchronized to be in the same state prior to making experimental measurements. In other words, chaotic entanglement could allow for experimenters to probe further into the classical–quantum transition without interrupting an entanglement state.
4.7. Entropy
In quantum mechanics, entropy is used to assess the strength of an entanglement [
5]. In classical systems, entropy is a quantity that has been long associated with thermodynamics given that it measures statistical uncertainty. However, entropy is now understood to be deeply related to information theory because it is used to quantify the rate at which evolving classical systems generate information over time. Information in chaotic systems is typically encoded in their symbolic dynamics, in which case entropy specifically measures the growth rate of new symbol sequences as they are produced by an evolving system. The visitation sequences of the double scroll system are an example of such a symbolic dynamics.
In this interpretation, entropy is used to distinguish chaotic behavior from random or (quasi–)periodic behavior. This is achieved by calculating the Kolmogorov, or metric, entropy of a classical system. Denoted by
K, Kolmogorov entropy ranges from
for periodic or quasiperiodic systems to
for random systems. In between are the chaotic trajectories for which
[
42,
52]. This last result follows from the fact that, although the dynamics of chaotic systems are deterministic, their aperiodicity and the geometry of their attractors are such that chaotic trajectories are confined to evolving along unique and non-stochastic paths on an attractor.
Entropy is particularly relevant to chaotic entanglement. Every freely-evolving chaotic system generates symbolic information at a positive and finite rate, but the entropy decays to zero whenever the chaotic behavior is controlled to become periodic. This would occur whenever a chaotic system is directed onto a periodic or cupolet state by the control scheme described in
Section 2, or whenever two interacting chaotic systems stabilize each other through entanglement. Both scenarios trigger the collapse of chaotic to periodic behavior, during which time the entropy decreases to zero. Chaotic entanglement and stabilization are thus entropy-reversing events.
Entropy-reversing events are unusual in classical mechanics given that the Second Law of Thermodynamics states that the entropy of any closed or isolated system is never decreasing. However, in its information interpretation, decreasing entropy is not unusual because this would occur anytime periodic orbits are stabilized from chaotic systems. As mentioned previously, chaotic entanglement is so far established only at the information-theoretic stage, but it is compelling to envision the implications of detecting chaotic entanglement in physical systems. If physical systems are found to interact in this manner, then their interaction would coincide with a decrease in the (information) entropy and would potentially induce the systems into chaotic entanglement.
4.8. Differences with Quantum Entanglement
We have discussed several properties of chaotic systems that evoke parallels between chaotic and quantum entanglement. It is just as interesting to discuss their differences. First, superposition in a purely quantum sense refers to linear combinations of state vectors that collectively describe the state of a quantum system and that satisfy the Schrödinger equation. Conventional superposition is not supported in chaotic systems due to their nonlinear governing equations. Superposition instead refers to a chaotic system existing as a mixture of its cupolets, or more precisely, its UPOs. In this framework, the state of a chaotic system is well-represented as a linear combination of the states of these periodic orbits. As the chaotic system evolves in time, so too does its state vector because each
in Equation (
8) represents the contribution of an associated cupolet to the current state of the chaotic system.
A second difference between quantum and chaotic entanglement concerns how expectedly entanglement can arise. Quantum entanglement is typically created deliberately between subatomic particles via direct interactions like atomic cascades or by spontaneous parametric down-conversions [
53,
54]. Interaction is also required for chaotic entanglement to arise because two chaotic systems must interactively communicate control information to each other, or the physical analog of control information. However, chaotic entanglement can also form naturally and without the aid of external preparation or control. As discussed in
Section 3.3, this is known as pure entanglement, and it arises because evolving chaotic systems are constantly visiting neighborhoods of their periodic orbits. This increases the likelihood that two interacting chaotic systems are concurrently shadowing periodic orbits that could induce entanglement.
Third, unlike quantum entanglement, which allows for spontaneous nonlocal correlations, chaotic entanglement is neither distance-independent nor instantaneous in its response to measurements. Although chaotic entanglement currently exists strictly at the information-theoretic stage, there is much potential for it to manifest in physical systems. Either these physical systems must be evolving in close proximity, or there must be a channel available for information exchange in order for their communication to induce an entanglement. Should two chaotically-entangled systems become spatially separated, or simply lose the ability to communicate, then the efficacy of their interaction would diminish to zero, leading to a loss of entanglement. Each system would transition to chaotic behavior because their trajectories are no longer being directed along periodic orbits. Unlike what transpires in quantum entanglement, this transition would not be instantaneous. The previously-entangled systems would continue to evolve in close proximity of its cupolet for a period of time proportional to the cupolet’s local Lyapunov exponent. As a consequence, chaotic entanglement does not exhibit instantaneous action at a distance.
This delayed response to measurement would allow for entanglement to be reacquired between two previously-entangled chaotic systems, provided that the correct control sequences are reinstated. If the interaction is restored quickly enough between the two systems, either by shortening their spatial separation or by removing any communication barriers, then the two systems would not have drifted too far from their previously-stabilized periodic orbits. With their interaction reinstated, the two systems would redirect each other back onto their respective periodic orbits and resume their entanglement.
To summarize, the key ingredients missing from our list of classical analogs to quantum mechanical characteristics are superposition in a conventional sense, nonlocality, and the instantaneous response to measurement. In quantum mechanics, when a measurement is applied to one of two entangled particles, the state vectors of both particles each instantly collapse onto a specific state vector regardless of spatial separation. Chaotic entanglement, in contrast, is limited by physical distances, and it exhibits delayed and resilient responses to measurement due to the influence that UPOs have on the dynamics of chaotic systems.