Probabilistic Resumable Quantum Teleportation of a Two-Qubit Entangled State
Abstract
1. Introduction
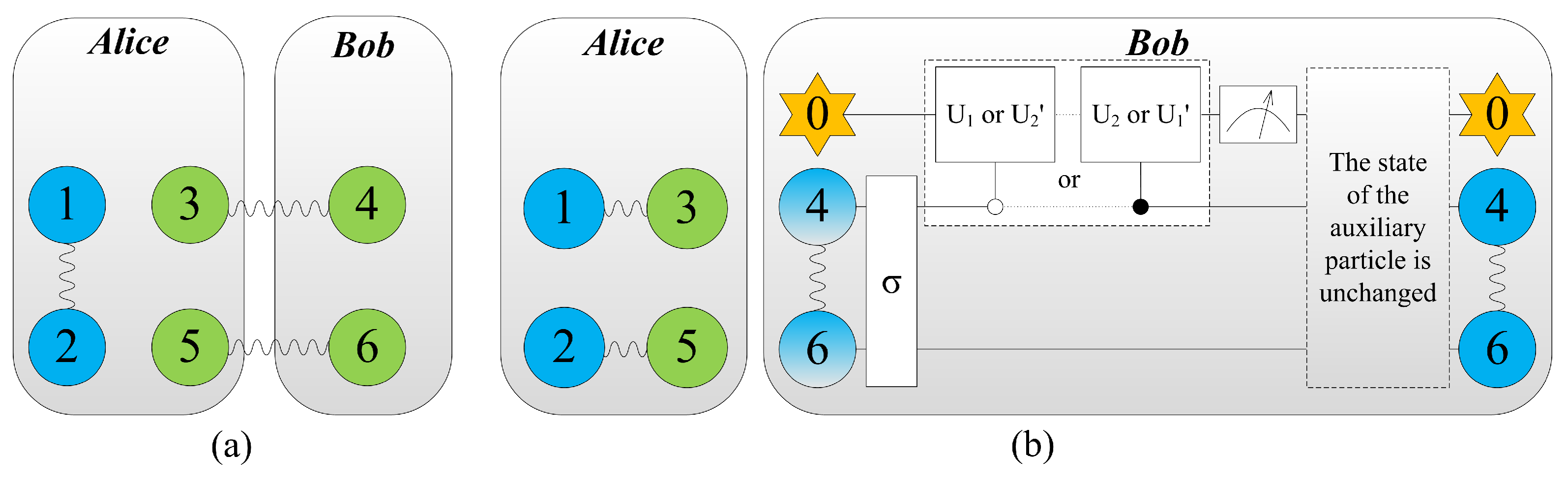
2. Probabilistic Teleportation of a Two-Qubit Entangled State
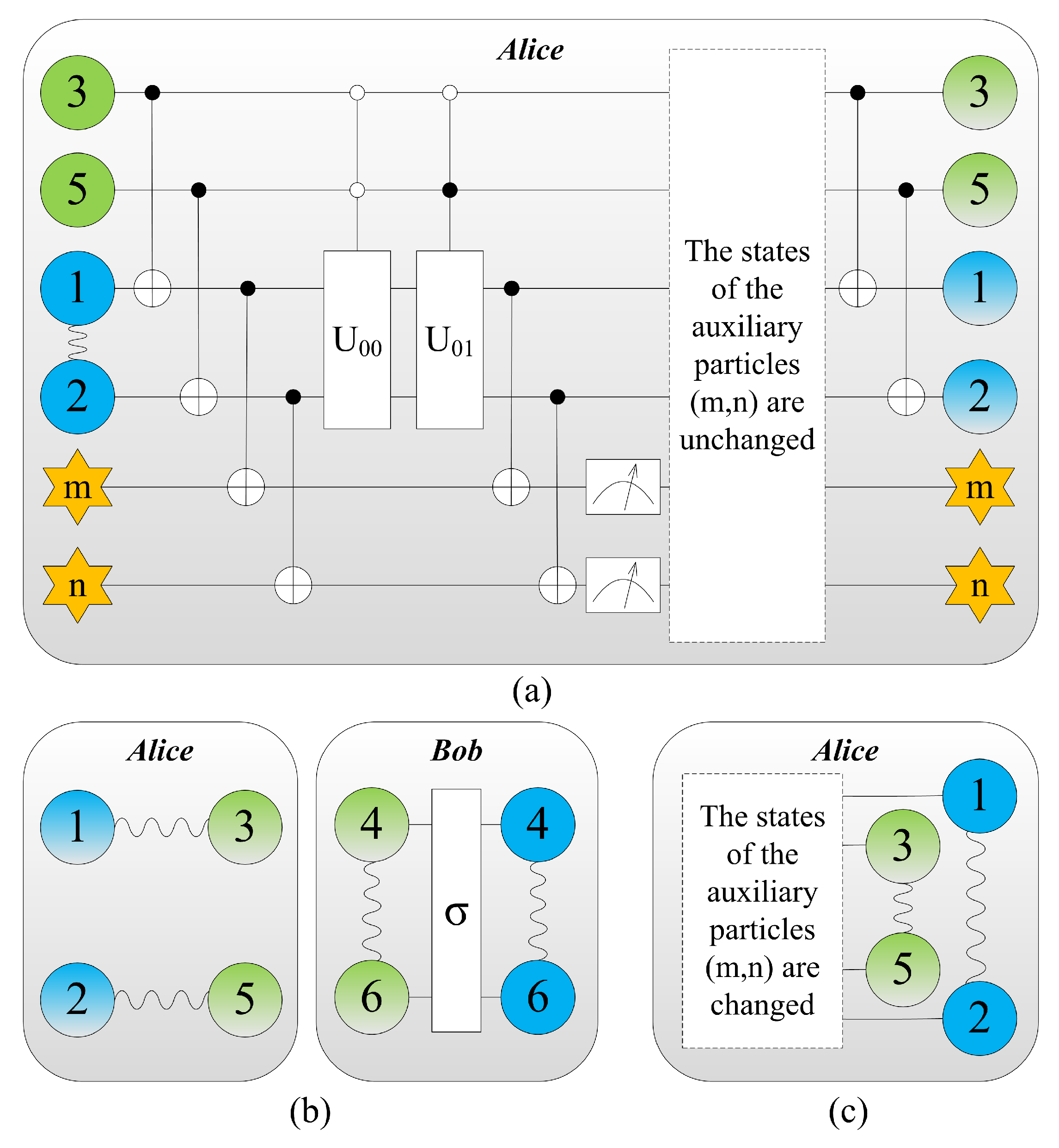
3. Resumable Quantum Teleportation of a Two-Qubit Entangled Sstate
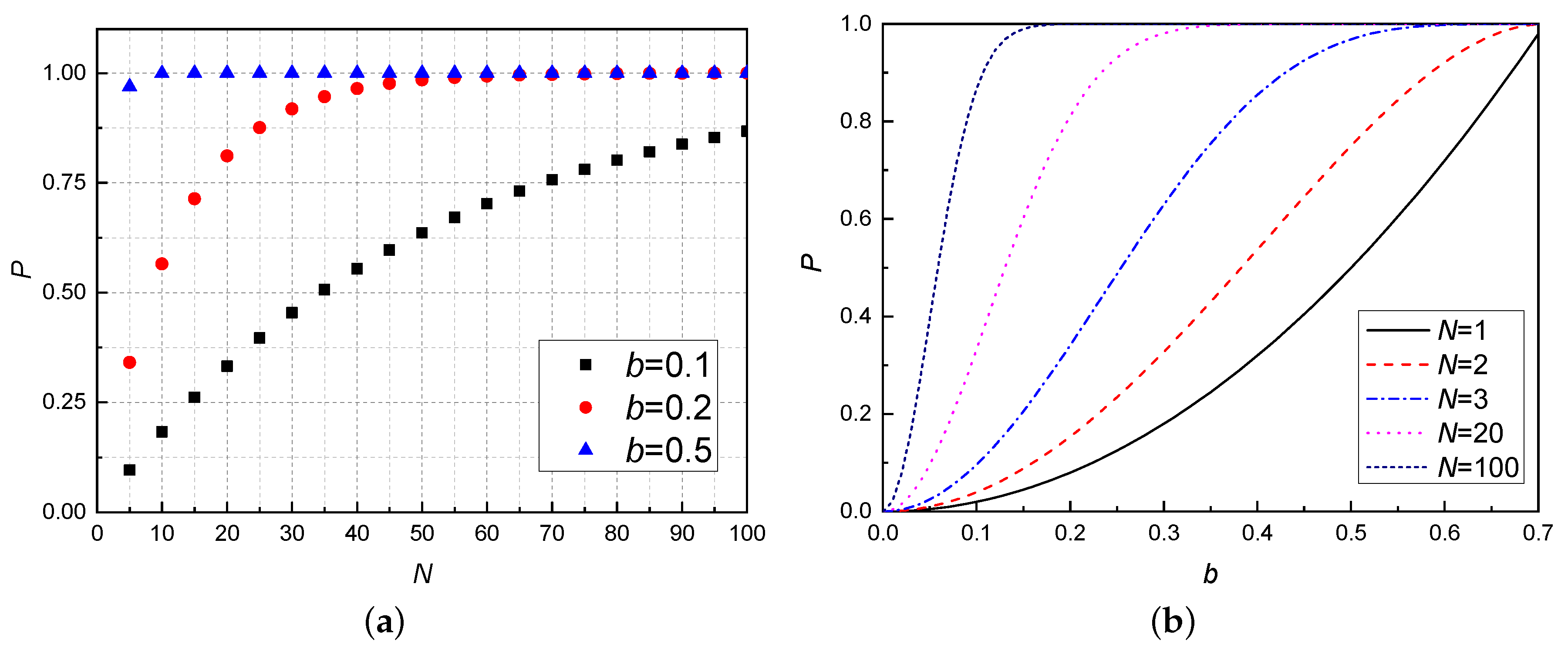
4. Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Bennett, C.H.; Brassard, G.; Crepeau, C.; Jozsa, R.; Peres, A.; Wootters, W.K. Teleporting an unknown quantum state via dual classical and Einstein-Podolsky-Rosen channels. Phys. Rev. Lett. 1993, 70, 1895–1899. [Google Scholar] [CrossRef] [PubMed]
- Einstein, A.; Podolsky, B.; Rosen, N. Can quantum-mechanical description of physical reality be considered complete? Phys. Rev. 1935, 47, 777. [Google Scholar] [CrossRef]
- Bouwmeester, D.; Pan, J.W.; Mattle, K.; Eibl, M.; Weinfurter, H.; Zeilinger, A. Experimental quantum teleportation. Nature 1997, 390, 575–579. [Google Scholar] [CrossRef]
- Boschi, D.; Branca, S.; De Martini, F.; Hardy, L.; Popescu, S. Experimental realization of teleporting an unknown pure quantum state via dual classical and Einstein-Podolsky-Rosen channels. Phys. Rev. Lett. 1998, 80, 1121–1125. [Google Scholar] [CrossRef]
- Furusawa, A.; Sørensen, J.L.; Braunstein, S.L.; Fuchs, C.A.; Kimble, H.J.; Polzik, E.S. Unconditional quantum teleportation. Science 1998, 282, 706–709. [Google Scholar] [CrossRef] [PubMed]
- Banaszek, K. Optimal quantum teleportation with an arbitrary pure state. Phys. Rev. A 2000, 62, 024301. [Google Scholar] [CrossRef]
- Yu, K.F.; Yang, C.W.; Liao, C.H.; Hwang, T. Authenticated semi-quantum key distribution protocol using Bell states. Quantum Inf. Process. 2014, 13, 1457–1465. [Google Scholar] [CrossRef]
- Deng, F.G.; Li, C.Y.; Li, Y.S.; Zhou, H.Y.; Wang, Y. Symmetric multiparty-controlled teleportation of an arbitrary two-particle entanglement. Phys. Rev. A 2005, 72, 022338. [Google Scholar] [CrossRef]
- Gottesman, D.; Chuang, I.L. Demonstrating the viability of universal quantum computation using teleportation and single-qubit operations. Nature 1999, 402, 390–393. [Google Scholar] [CrossRef]
- Thapliyal, K.; Verma, A.; Pathak, A. A general method for selecting quantum channel for bidirectional controlled state teleportation and other schemes of controlled quantum communication. Quantum Inf. Process. 2015, 14, 4601–4614. [Google Scholar] [CrossRef]
- Takeda, S.; Mizuta, T.; Fuwa, M.; van Loock, P.; Furusawa, A. Deterministic quantum teleportation of photonic quantum bits by a hybrid technique. Nature 2013, 500, 315–318. [Google Scholar] [CrossRef]
- Kim, Y.H.; Kulik, S.P.; Shih, Y. Quantum teleportation of a polarization state with a complete Bell state measurement. Phys. Rev. Lett. 2001, 86, 1370. [Google Scholar] [CrossRef]
- Prakash, H.; Verma, V. Minimum assured fidelity and minimum average fidelity in quantum teleportation of single qubit using non-maximally entangled states. Quantum Inf. Process. 2012, 11, 1951–1959. [Google Scholar] [CrossRef]
- Li, G.; Ye, M.Y.; Lin, X.M. Entanglement fidelity of the standard quantum teleportation channel. Phys. Lett. A 2013, 377, 1531–1533. [Google Scholar] [CrossRef]
- Linden, N.; Massar, S.; Popescu, S. Purifying noisy entanglement requires collective measurements. Phys. Rev. Lett. 1998, 81, 3279. [Google Scholar] [CrossRef]
- Werner, R.F. Quantum states with Einstein-Podolsky-Rosen correlations admitting a hidden-variable model. Phys. Rev. A 1989, 40, 4277. [Google Scholar] [CrossRef]
- Bennett, C.H.; Brassard, G.; Popescu, S.; Schumacher, B.; Smolin, J.A.; Wootters, W.K. Purification of noisy entanglement and faithful teleportation via noisy channels. Phys. Rev. Lett. 1996, 76, 722. [Google Scholar] [CrossRef]
- Bowen, G.; Bose, S. Teleportation as a Depolarizing Quantum Channel, Relative Entropy, and Classical Capacity. Phys. Rev. Lett. 2001, 87, 267901–267904. [Google Scholar] [CrossRef]
- Albeverio, S.; Fei, S.M.; Yang, W.L. Optimal teleportation based on bell measurements. Phys. Rev. A 2002, 66, 012301. [Google Scholar] [CrossRef]
- Li, W.L.; Li, C.F.; Guo, G.C. Probabilistic teleportation and entanglement matching. Phys. Rev. A 2000, 61, 034301. [Google Scholar] [CrossRef]
- Li, D.C.; Shi, Z.K. Probabilistic teleportation via entanglement. Int. J. Theor. Phys. 2008, 47, 2645–2654. [Google Scholar] [CrossRef]
- Agrawal, P.; Pati, A.K. Probabilistic quantum teleportation. Phys. Lett. A 2002, 305, 12–17. [Google Scholar] [CrossRef]
- An, N.B. Probabilistic teleportation of an M-qubit state by a single non-maximally entangled qubit-pair. Phys. Lett. A 2008, 372, 3778–3783. [Google Scholar] [CrossRef]
- Gou, Y.T.; Shi, H.L.; Wang, X.H.; Liu, S.Y. Probabilistic resumable bidirectional quantum teleportation. Quantum Inf. Process. 2017, 16, 1–13. [Google Scholar] [CrossRef]
- Liu, D.S.; Huang, Z.P.; Guo, X.J. Probabilistic Teleportation via Quantum Channel with Partial Information. Entropy 2015, 17, 3621–3630. [Google Scholar] [CrossRef]
- Wang, K.; Yu, X.T.; Cai, X.F.; Zhang, Z.C. Probabilistic Teleportation of Arbitrary Two-Qubit Quantum State via Non-Symmetric Quantum Channel. Entropy 2018, 20, 238. [Google Scholar] [CrossRef]
- Gorbachev, V.N.; Trubilko, A.I. Quantum teleportation of an Einstein-Podolsy-Rosen pair using an entangled three-particle state. J. Exp. Theor. Phys. 2000, 91, 894–898. [Google Scholar] [CrossRef]
- Shi, B.S.; Jiang, Y.K.; Guo, G.C. Probabilistic teleportation of two-particle entangled state. Phys. Lett. A 2000, 268, 161–164. [Google Scholar] [CrossRef]
- Lu, H.; Guo, G.C. Teleportation of a two-particle entangled state via entanglement swapping. Phys. Lett. A 2000, 276, 209–212. [Google Scholar] [CrossRef]
- Wootters, W.K.; Zurek, W.H. A single quantum cannot be cloned. Nature 1982, 299, 802–803. [Google Scholar] [CrossRef]
- Bužek, V.; Hillery, M. Universal optimal cloning of arbitrary quantum states: from qubits to quantum registers. Phys. Rev. Lett. 1998, 81, 5003. [Google Scholar] [CrossRef]
- Brune, M.; Hagley, E.; Dreyer, J.; Maître, X.; Maali, A.; Wunderlich, C.; Raimond, J.M.; Haroche, S. Observing the progressive decoherence of the meter in a quantum measurement. Phys. Rev. Lett. 1996, 77, 4887. [Google Scholar] [CrossRef]
- Ohya, M. Some aspects of quantum information theory and their applications to irreversible processes. Rep. Math. Phys. 1989, 27, 19–47. [Google Scholar] [CrossRef]
- Roa, L.; Groiseau, C. Probabilistic teleportation without loss of information. Phys. Rev. A 2015, 91, 012344. [Google Scholar] [CrossRef]
- Lütkenhaus, N.; Calsamiglia, J.; Suominen, K.A. Bell measurements for teleportation. Phys. Rev. A 1999, 59, 3295. [Google Scholar] [CrossRef]
- Bai, C.H.; Wang, D.Y.; Hu, S.; Cui, W.X.; Jiang, X.X.; Wang, H.F. Scheme for implementing multitarget qubit controlled-NOT gate of photons and controlled-phase gate of electron spins via quantum dot-microcavity coupled system. Quantum Inf. Process. 2016, 15, 1485–1498. [Google Scholar] [CrossRef]
- De Martini, F.; Bužek, V.; Sciarrino, F.; Sias, C. Experimental realization of the quantum universal NOT gate. Nature 2002, 419, 815–818. [Google Scholar] [CrossRef] [PubMed]
- DiVincenzo, D.P. Two-bit gates are universal for quantum computation. Phys. Rev. A 1995, 51, 1015. [Google Scholar] [CrossRef]
- Sleator, T.; Weinfurter, H. Realizable universal quantum logic gates. Phys. Rev. Lett. 1995, 74, 4087. [Google Scholar] [CrossRef] [PubMed]
- Schlosshauer, M. Decoherence, the measurement problem, and interpretations of quantum mechanics. Rev. Mod. Phys. 2005, 76, 1267. [Google Scholar] [CrossRef]
| Alice’s Measurement Results | Probability | Bob’s Unitary Operation |
|---|---|---|
| Alice’ Result | Probability | Operation |
|---|---|---|
| Alice’s Measurement Results | the State of Particles () | Bob’s Unitary Operation |
|---|---|---|
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Z.-Y.; Gou, Y.-T.; Hou, J.-X.; Cao, L.-K.; Wang, X.-H. Probabilistic Resumable Quantum Teleportation of a Two-Qubit Entangled State. Entropy 2019, 21, 352. https://doi.org/10.3390/e21040352
Wang Z-Y, Gou Y-T, Hou J-X, Cao L-K, Wang X-H. Probabilistic Resumable Quantum Teleportation of a Two-Qubit Entangled State. Entropy. 2019; 21(4):352. https://doi.org/10.3390/e21040352
Chicago/Turabian StyleWang, Zhan-Yun, Yi-Tao Gou, Jin-Xing Hou, Li-Ke Cao, and Xiao-Hui Wang. 2019. "Probabilistic Resumable Quantum Teleportation of a Two-Qubit Entangled State" Entropy 21, no. 4: 352. https://doi.org/10.3390/e21040352
APA StyleWang, Z.-Y., Gou, Y.-T., Hou, J.-X., Cao, L.-K., & Wang, X.-H. (2019). Probabilistic Resumable Quantum Teleportation of a Two-Qubit Entangled State. Entropy, 21(4), 352. https://doi.org/10.3390/e21040352




