Influence of the Coupling between Two Qubits in an Open Coherent Cavity: Nonclassical Information via Quasi-Probability Distributions
Abstract
:1. Introduction
2. Physical Model and Density Matrix
3. Quasi-Probability Distributions
3.1. Wigner Function
3.2. Q-Function and Partial Wehrl Entropies
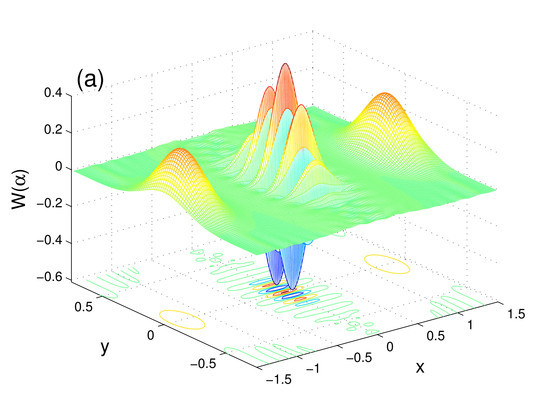
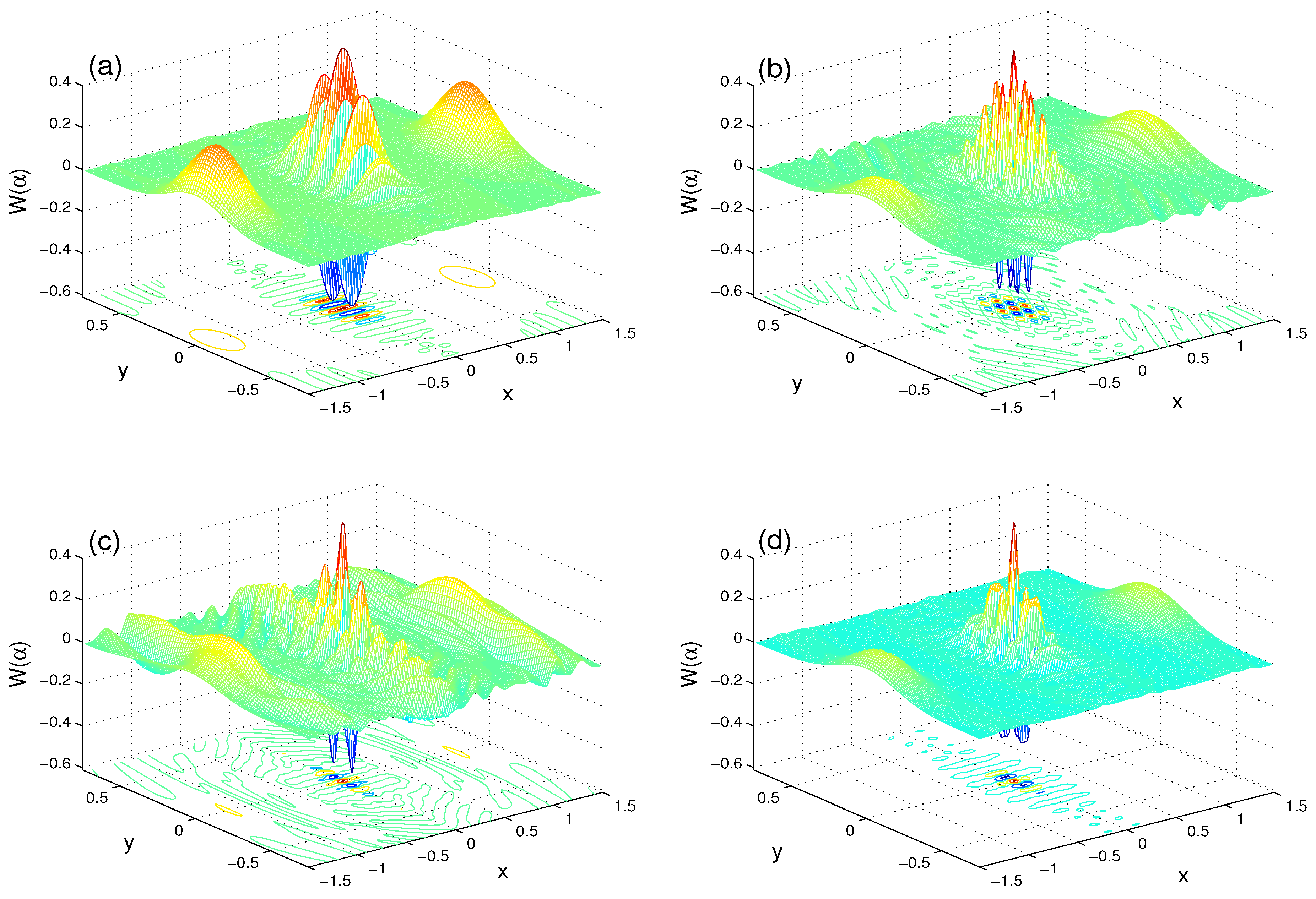
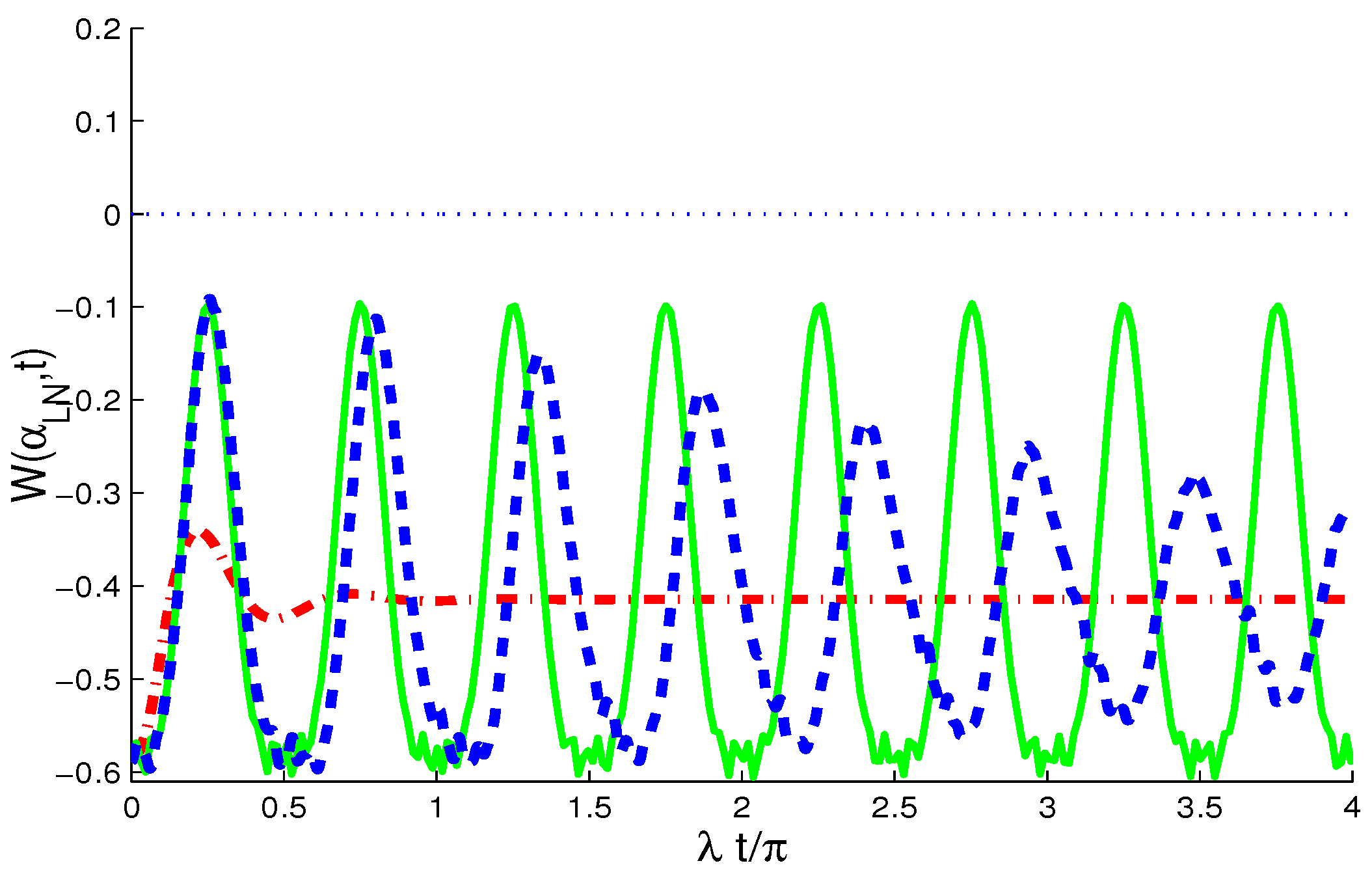
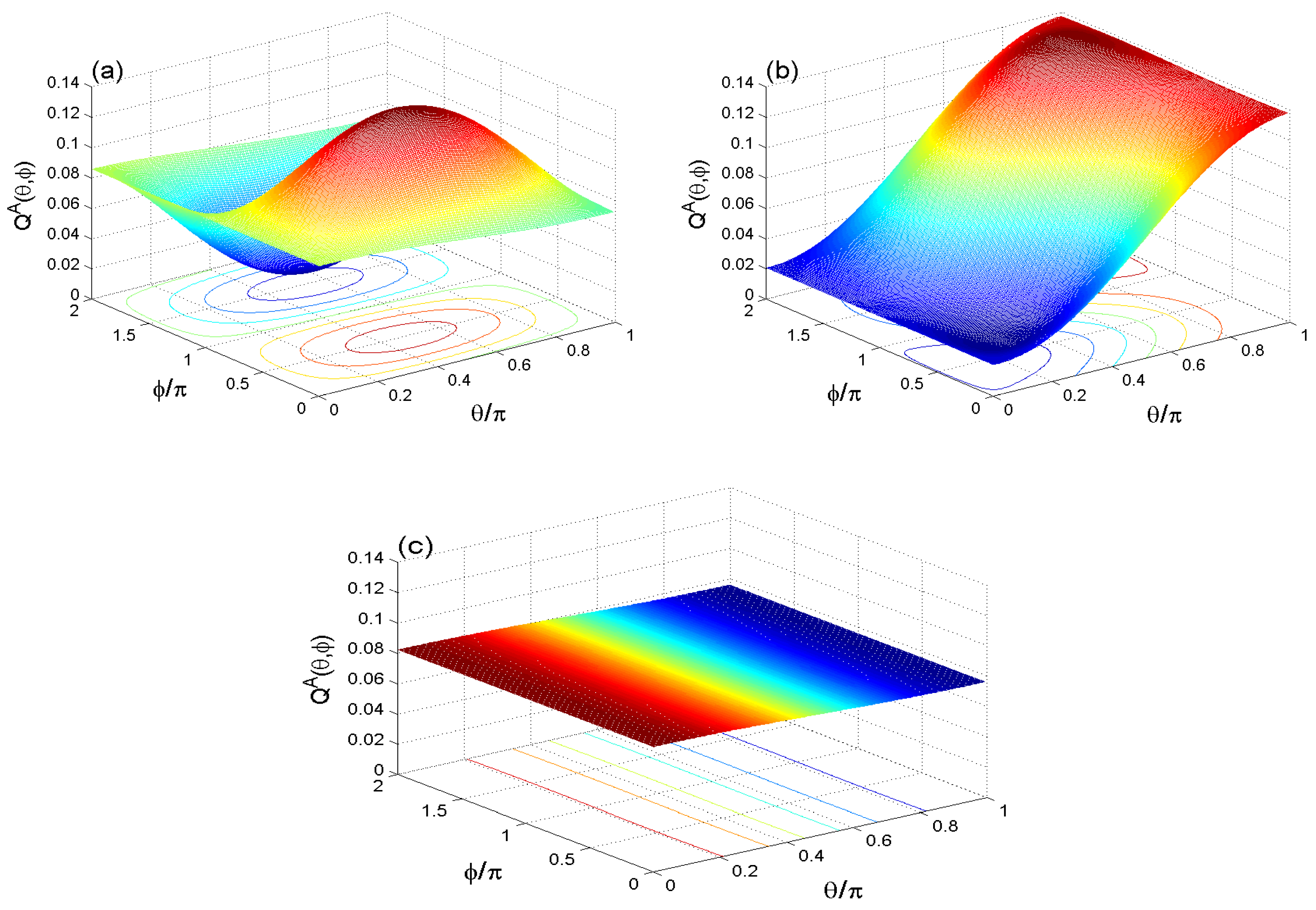
4. Numerical Analysis of Quasi-Probability Functions
4.1. Numerical Analysis of WF
4.2. Numerical Analysis of QF
4.3. Coherence Loss of Wehrl Entropy
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Shore, B.W. The Theory of Coherent Atomic Excitation; Wiley: New York, NY, USA, 1990. [Google Scholar]
- Li, D.X.; Shao, X.Q. Rapid population transfer of a two-level system by a polychromatic driving field. Sci. Rep. 2019, 9, 9023. [Google Scholar] [CrossRef] [PubMed]
- Nakamura, Y.; Pashkin, Y.A.; Tsai, J.S. Coherent control of macroscopic quantum states in a single-Cooper-pair box. Nature 1999, 398, 786. [Google Scholar] [CrossRef]
- Treutlein, P.; Genes, C.; Hammerer, K.; Poggio, M.; Rabl, P. Hybrid Mechanical Systems; Springer: Berlin/Heidelberg, Germany, 2014. [Google Scholar]
- You, J.Q.; Nori, F. Quantum information processing with superconducting qubits in a microwave field. Phys. Rev. B 2003, 68, 064509. [Google Scholar] [CrossRef]
- Liu, Y.-X.; Wei, L.F.; Nori, F. Measuring the quality factor of a microwave cavity using superconducting qubit devices. Phys. Rev. A 2005, 72, 033818. [Google Scholar] [CrossRef]
- Hime, T.; Reichardt, P.A.; Plourde, B.L.T.; Robertson, T.L.; Wu, C.E.; Ustinov, A.V.; Clarke, J. Solid-state qubits with current-controlled coupling. Science 2006, 314, 1427–1429. [Google Scholar] [CrossRef]
- Khan, S.; Tureci, H.E. Frequency Combs in a Lumped-Element Josephson-Junction Circuit. Phys. Rev. Lett. 2018, 120, 153601. [Google Scholar] [CrossRef]
- Obada, A.-S.F.; Hessian, H.A.; Mohamed, A.-B.A.; Homid, A.H. Implementing discrete quantum Fourier transform via superconducting qubits coupled to a superconducting cavity. J. Opt. Soc. Am. B 2013, 30, 1178–1185. [Google Scholar] [CrossRef]
- Nilsen, A.M.; Chuang, L.I. Quantum Computation and Quantum Information; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Wigner, E.P. On the Quantum Correction For Thermodynamic Equilibrium. Phys. Rev. 1932, 47, 749. [Google Scholar] [CrossRef]
- Husimi, K. Some Formal Properties of the Density Matrix. Proc. Phys. Math. Soc. Jpn. 1940, 22, 264–314. [Google Scholar]
- Hillery, M.; O’Connell, R.F.; Scully, M.O.; Wigner, E.P. Distribution functions in physics: Fundamentals. Phys. Rep. 1984, 106, 121. [Google Scholar] [CrossRef]
- Miranowicz, A.; Leoński, W.; Imoto, N. Quantum-Optical States in Finite-Dimensional Hilbert Space. I. General Formalism. Adv. Chem. Phys. 2001, 119, 155–194. [Google Scholar]
- Banerji, A.; Singh, R.P.; Bandyopadhyay, A. Entanglement measure using Wigner function: Case of generalized vortex state formed by multiphoton subtraction. Opt. Commun. 2014, 330, 85–90. [Google Scholar] [CrossRef]
- Mohamed, A.-B.; Eleuch, H. Non-classical effects in cavity QED containing a nonlinear optical medium and a quantum well: Entanglement and non-Gaussanity. Eur. Phys. J. D 2015, 69, 191. [Google Scholar] [CrossRef]
- Ghorbani, M.; Faghihi, M.J.; Safari, H. Wigner function and entanglement dynamics of a two-atom two-mode nonlinear Jaynes-Cummings model. J. Opt. Soc. Am. B 2017, 34, 1884–1893. [Google Scholar] [CrossRef]
- Ren, G.; Zhang, W. Nonclassicality of superposition of photon-added two-mode coherent states. Optik 2019, 181, 191–201. [Google Scholar] [CrossRef]
- Bolda, E.L.; Tan, S.M.; Walls, D. Measuring the quantum state of a Bose-Einstein condensate. Phys. Rev. A 1998, 57, 4686. [Google Scholar] [CrossRef]
- Yazdanpanah, N.; Tavassoly, M.K.; Juárez-Amaro, R.; Moya-Cessa, H.M. Reconstruction of quasiprobability distribution functions of the cavity field considering field and atomic decays. Opt. Commun. 2017, 400, 69–73. [Google Scholar] [CrossRef]
- Mohamed, A.-B.A.; Eleuch, H.; Hessian, H.A. Stationary phase-space information in a qubit interacting non-linearly with a lossy single-mode field in the offresonant case. Opt. Quantum Electron. 2017, 49, 84. [Google Scholar] [CrossRef]
- Obada, A.-S.F.; Mohamed, A.-B.A. Erasing information and purity of a quantum dot via its spontaneous decay. Solid State Commun. 2011, 151, 1824–1827. [Google Scholar] [CrossRef]
- Mohamed, A.-B.A.; Eleuch, H. Coherence and information dynamics of a Λ-type three-level atom interacting with a damped cavity field. Eur. Phys. J. Plus 2017, 132, 75. [Google Scholar] [CrossRef]
- Von Neumann, J. Mathematical Foundations of Quantum Mechanics; Princeton University Press: Princeton, NJ, USA, 1955. [Google Scholar]
- Shannon, C.E.; Weaver, W. Mathematical Theory of Communication; Urbana University Press: Chicago, IL, USA, 1949. [Google Scholar]
- Mohamed, A.-B.A.; Eleuch, H. Generation and robustness of bipartite non-classical correlations in two nonlinear microcavities coupled by an optical fiber. J. Opt. Soc. Am. B 2018, 35, 47–53. [Google Scholar] [CrossRef]
- Mohamed, A.-B.A.; Joshi, A.; Hassan, S.S. Bipartite non-local correlations in a double-quantum-dot excitonic system. J. Phys. A Math. Theor. 2014, 47, 335301. [Google Scholar] [CrossRef]
- Milburn, G.J. Intrinsic decoherence in quantum mechanics. Phys. Rev. A 1991, 44, 5401. [Google Scholar] [CrossRef] [PubMed]
- Mohamed, A.-B.A.; Metwally, N. Nonclassical features of two SC-qubit system interacting with a coherent SC-cavity. Physica E 2018, 102, 1–7. [Google Scholar] [CrossRef]
- Nambu, Y.; Jona-Lasinio, G. Dynamical Model of Elementary Particles Based on an Analogy with Superconductivity. I. Phys. Rev. 1961, 122, 345–358. [Google Scholar] [CrossRef]
- Nambu, Y.; Jona-Lasinio, G. Dynamical Model of Elementary Particles Based on an Analogy with Superconductivity. II. Phys. Rev. 1961, 124, 246–254. [Google Scholar] [CrossRef]
- Arraut, I. The Quantum Yang-Baxter Conditions: The Fundamental Relations behind the Nambu-Goldstone Theorem. Symmetry 2019, 11, 803. [Google Scholar] [CrossRef]
- Prähofer, M.; Spohn, H. Exact Scaling Functions for One-Dimensional Stationary KPZ Growth. J. Stat. Phys. 2004, 115, 255–279. [Google Scholar] [CrossRef]
- Moya-Cessa, H.; Knight, P.L. Series representation of quantum-field quasiprobabilities. Phys. Rev. A 1993, 48, 2479. [Google Scholar] [CrossRef]
- Hessian, H.A.; Mohamed, A.-B.A. Quasi-Probability Distribution Functions for a Single Trapped Ion Interacting with a Mixed Laser Field. Laser Phys. 2008, 18, 1217. [Google Scholar] [CrossRef]
- Cahill, K.E.; Glauber, R.J. Ordered Expansions in Boson Amplitude Operators. Phys. Rev. 1969, 177, 1857. [Google Scholar] [CrossRef]
- Vieira, V.R.; Sacramento, P.D. Generalized phase-space representatives of spin-J operators in terms of Bloch coherent states. Ann. Phys. 1995, 242, 188–231. [Google Scholar] [CrossRef]
- Wehrl, A. General properties of entropy. Rev. Mod. Phys. 1978, 50, 221. [Google Scholar] [CrossRef]
- Obada, A.-S.; Abdel-Khalek, S. New features of the atomic Wehrl entropy and its density in multi-quanta two-level system. J. Phys. A: Math. Gen. 2004, 37, 6573. [Google Scholar] [CrossRef]
- van Enk, S.J.; Kimble, H.J. On the classical character of control fields in quantum information processing. Quantum Inform. Comput. 2002, 2, 1–13. [Google Scholar]


© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mohamed, A.-B.A.; Eleuch, H.; Obada, A.-S.F. Influence of the Coupling between Two Qubits in an Open Coherent Cavity: Nonclassical Information via Quasi-Probability Distributions. Entropy 2019, 21, 1137. https://doi.org/10.3390/e21121137
Mohamed A-BA, Eleuch H, Obada A-SF. Influence of the Coupling between Two Qubits in an Open Coherent Cavity: Nonclassical Information via Quasi-Probability Distributions. Entropy. 2019; 21(12):1137. https://doi.org/10.3390/e21121137
Chicago/Turabian StyleMohamed, Abdel-Baset A., Hichem Eleuch, and Abdel-Shafy F. Obada. 2019. "Influence of the Coupling between Two Qubits in an Open Coherent Cavity: Nonclassical Information via Quasi-Probability Distributions" Entropy 21, no. 12: 1137. https://doi.org/10.3390/e21121137
APA StyleMohamed, A.-B. A., Eleuch, H., & Obada, A.-S. F. (2019). Influence of the Coupling between Two Qubits in an Open Coherent Cavity: Nonclassical Information via Quasi-Probability Distributions. Entropy, 21(12), 1137. https://doi.org/10.3390/e21121137