A New Belief Entropy Based on Deng Entropy
Abstract
1. Introduction
2. Preliminaries
2.1. Dempster–Shafer Evidence Theory
2.2. Shannon Entropy
2.3. Some Uncertainty Measures for Dempster–Shafer Framework
2.3.1. Hohle’s Confusion Measure
2.3.2. Yager’s Dissonance Measure
2.3.3. Dubois and Prade’s Weighted Hartley Entropy
2.3.4. Klir and Ramer’s Discord Measure
2.3.5. Klir and Parviz’s Strife Measure
2.3.6. George and Pal’s Conflict Measure
2.4. Deng Entropy and Its Modified Entropy
2.4.1. Zhou et al.’s Entropy
2.4.2. Pan et al.’s Entropy
2.4.3. Cui et al.’s Entropy
3. The Proposed Method
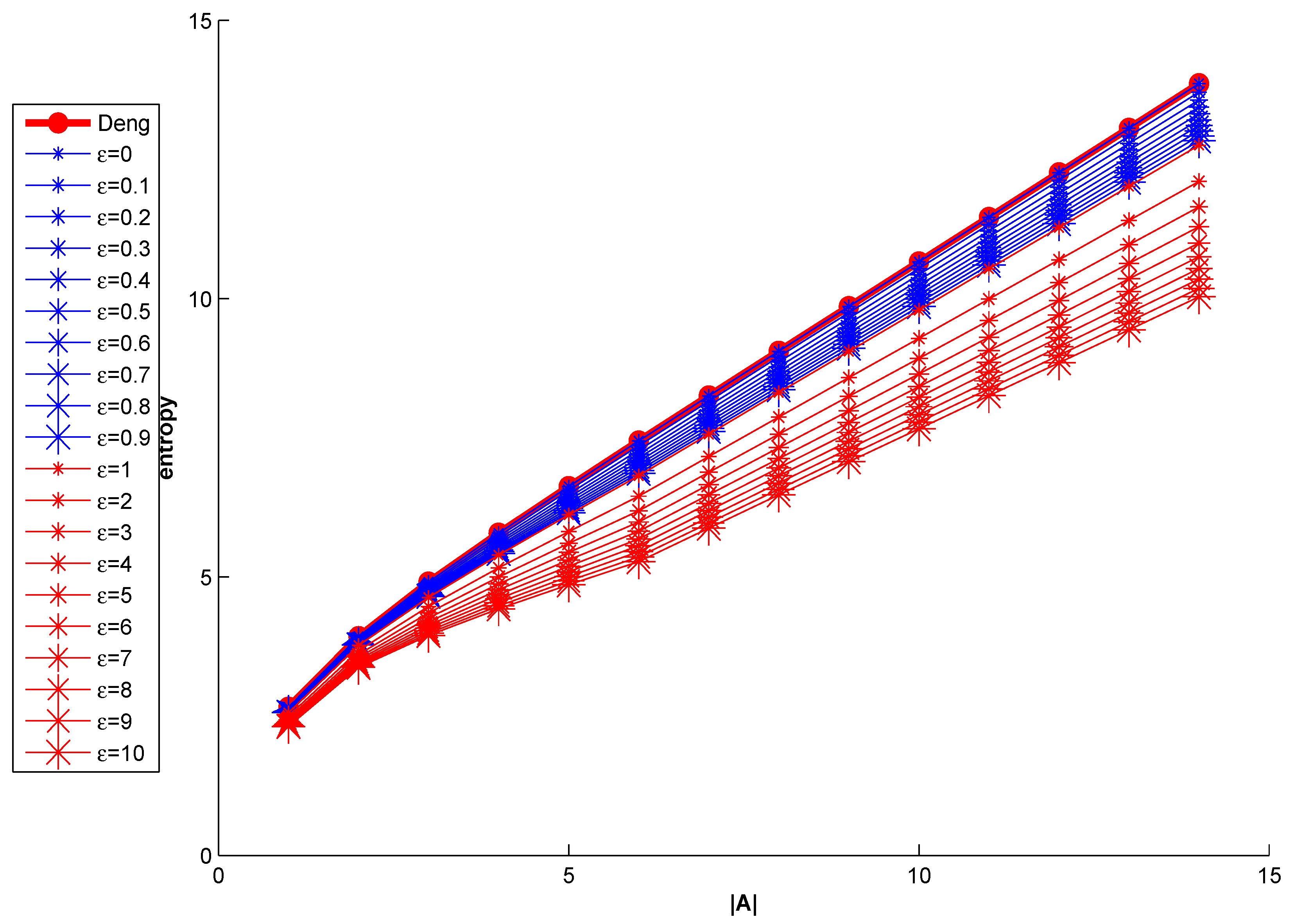
4. Numerical Examples
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Gordon, J.; Shortliffe, E.H. The Dempster–Shafer theory of evidence. Rule-Based Expert Systems: The MYCIN Experiments of the Stanford Heuristic Programming Project. Available online: https://dl.acm.org/citation.cfm?id=85351 (accessed on 9 October 2019).
- Zadeh, L.A. A simple view of the Dempster–Shafer theory of evidence and its implication for the rule of combination. AI Mag. 1986, 7, 85. [Google Scholar]
- Charnes, A.; Cooper, W.W.; Rhodes, E. Measuring the efficiency of decision-making units. Eur. J. Oper. Res. 1978, 2, 429–444. [Google Scholar] [CrossRef]
- Bell, D.E. Regret in decision-making under uncertainty. Oper. Res. 1982, 30, 961–981. [Google Scholar] [CrossRef]
- Polikar, R. Ensemble based systems in decision-making. IEEE Circuits Syst. Mag. 2006, 6, 21–45. [Google Scholar] [CrossRef]
- Edwards, W. The theory of decision-making. Psychol. Bull. 1954, 51, 380. [Google Scholar] [CrossRef]
- Spekman, R.E.; Kamauff, J.; Spear, J. Towards more effective sourcing and supplier management. Eur. J. Purch. Supply Manag. 1999, 5, 103–116. [Google Scholar] [CrossRef]
- Reuter, C.; Foerstl, K.; Hartmann, E.; Blome, C. Sustainable global supplier management: The role of dynamic capabilities in achieving competitive advantage. J. Supply Chain Manag. 2010, 46, 45–63. [Google Scholar] [CrossRef]
- Choi, T.Y.; Kim, Y. Structural embeddedness and supplier management: A network perspective. J. Supply Chain Manag. 2008, 44, 5–13. [Google Scholar] [CrossRef]
- Hu, M.K. Visual pattern recognition by moment invariants. IRE Trans. Inf. Theory 1962, 8, 179–187. [Google Scholar]
- Brose, M.S.; Rebbeck, T.R.; Calzone, K.A.; Stopfer, J.E.; Nathanson, K.L.; Weber, B.L. Cancer risk estimates for BRCA1 mutation carriers identified in a risk evaluation program. J. Natl. Cancer Inst. 2002, 94, 1365–1372. [Google Scholar] [CrossRef]
- Klinke, A.; Renn, O. A New Approach to Risk Evaluation and Management: Risk-Based, Precaution-Based, and Discourse-Based Strategies 1. Risk Anal. Int. J. 2002, 22, 1071–1094. [Google Scholar] [CrossRef]
- Nashef, S.A.; Roques, F.; Michel, P.; Gauducheau, E.; Lemeshow, S.; Salamon, R.; Group, E.S. European system for cardiac operative risk evaluation (Euro SCORE). Eur. J. Cardiothorac. Surg. 1999, 16, 9–13. [Google Scholar] [CrossRef]
- Epanechnikov, V.A. Non-parametric estimation of a multivariate probability density. Theory Probab. Appl. 1969, 14, 153–158. [Google Scholar] [CrossRef]
- Rubinov, M.; Sporns, O. Complex network measures of brain connectivity: Uses and interpretations. Neuroimage 2010, 52, 1059–1069. [Google Scholar] [CrossRef] [PubMed]
- Sporns, O. The human connectome: a complex network. Ann. N. Y. Acad. Sci. 2011, 1224, 109–125. [Google Scholar] [CrossRef]
- Deng, Y. Generalized evidence theory. Appl. Intell. 2015, 43, 530–543. [Google Scholar] [CrossRef]
- Wanga, N.; Liua, F.; Weia, D. A modified combination rule for D numbers theory. Math. Prob. Eng. 2016, 2016, 1–10. [Google Scholar] [CrossRef]
- Wang, N.; Liu, X.; Wei, D. A Modified D Numbers’ Integration for Multiple Attributes Decision Making. Int. J. Fuzzy Syst. 2017, 20, 1–12. [Google Scholar] [CrossRef]
- Boudraa, A.O.; Bentabet, L.; Salzenstein, F. Dempster–Shafer’s basic probability assignment based on fuzzy membership functions. Electron. Lett. Comput. Vision Image Anal. 2004, 4, 1–10. [Google Scholar] [CrossRef]
- Deng, X.; Liu, Q.; Deng, Y.; Mahadevan, S. An improved method to construct basic probability assignment based on the confusion matrix for classification problem. Inf. Sci. 2016, 340, 250–261. [Google Scholar] [CrossRef]
- Yager, R.R. Entropy and specificity in a mathematical theory of evidence. Int. J. Gen. Syst. 1983, 9, 249–260. [Google Scholar] [CrossRef]
- De Mántaras, R.L. A distance-based attribute selection measure for decision tree induction. Mach. Learn. 1991, 6, 81–92. [Google Scholar] [CrossRef]
- Morgan, B.S. An Alternate Approach to the Development of a Distance-Based Measure of Racial Segregation. Am. J. Sociol. 1983, 88, 1237–1249. [Google Scholar] [CrossRef]
- Tang, Y.; Zhou, D.; Xu, S.; He, Z. A weighted belief entropy-based uncertainty measure for multi-sensor data fusion. Sensors 2017, 17, 928. [Google Scholar] [CrossRef] [PubMed]
- Pal, N.R.; Bezdek, J.C.; Hemasinha, R. Uncertainty measures for evidential reasoning I: A review. Int. J. Approximate Reason. 1992, 7, 165–183. [Google Scholar] [CrossRef]
- George, T.; Pal, N.R. Quantification of conflict in the Dempster–Shafer framework: A new approach. Int. J. Gen. Syst. 1996, 24, 407–423. [Google Scholar] [CrossRef]
- Lin, J. Divergence measures based on the Shannon entropy. IEEE Trans. Inf. Theory 1991, 37, 145–151. [Google Scholar] [CrossRef]
- Rai, A.; Al-Hindi, H. The effects of development process modeling and task uncertainty on development quality performance. Inf. Manag. 2000, 37, 335–346. [Google Scholar] [CrossRef]
- Zhou, D.; Tang, Y.; Jiang, W. A modified belief entropy in dempster-shafer framework. PLoS ONE 2017, 12, e0176832. [Google Scholar] [CrossRef]
- Chen, X.H.; Dempster, A.P.; Liu, J.S. Weighted finite population sampling to maximize entropy. Biometrika 1994, 81, 457–469. [Google Scholar] [CrossRef]
- Deng, Y. Deng entropy. Chaos Solitons Fractals 2016, 91, 549–553. [Google Scholar] [CrossRef]
- Li, Z.; Pradeep, K.G.; Deng, Y.; Raabe, D.; Tasan, C.C. Metastable high-entropy dual-phase alloys overcome the strength-ductility trade-off. Nature 2016, 534, 227. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.; Xiao, F.; Deng, X.; Fei, L.; Deng, Y. Weighted evidence combination based on distance of evidence and entropy function. Int. J. Distrib. Sens. Netw. 2016, 12, 3218784. [Google Scholar] [CrossRef]
- Abellán, J. Analyzing properties of Deng entropy in the theory of evidence. Chaos Solitons Fractals 2017, 95, 195–199. [Google Scholar] [CrossRef]
- Ng, M.; Fleming, T.; Robinson, M.; Thomson, B.; Graetz, N.; Margono, C.; Mullany, E.C.; Biryukov, S.; Abbafati, C.; Abera, S.F.; et al. Global, regional, and national prevalence of overweight and obesity in children and adults during 1980–2013: A systematic analysis for the Global Burden of Disease Study 2013. Lancet 2014, 384, 766–781. [Google Scholar] [CrossRef]
- Pan, L.; Deng, Y. A new belief entropy to measure uncertainty of basic probability assignments base on belief function and plausibility function. Entropy 2018, 20, 842. [Google Scholar] [CrossRef]
- Cui, H.; Liu, Q.; Zhang, J.; Kang, B. An improved Deng entropy and its application in pattern recognition. IEEE Access 2019, 7, 18283–18292. [Google Scholar] [CrossRef]
- Florea, M.C.; Jousselme, A.L.; Grenier, D.; Bossé, É. An unified approach to the fusion of imperfect data? In Proceedings of SPIE—The International Society for Optical Engineering; SPIE: Bellingham, WA, USA, 2002; pp. 75–85. [Google Scholar]

| Fusion Method Focus | A | B | A, B |
|---|---|---|---|
| Dempster’s combination rule | 0 | 0 | 1 |
| The proposed method | 0.4999 | 0.4999 | 0.0002 |
| Cases | Deng Entropy | Cui’s Entropy | W Entropy |
|---|---|---|---|
| 2.6623 | 2.6622 | 2.5623 | |
| 3.9303 | 3.9301 | 3.7703 | |
| 4.9082 | 4.908 | 4.6315 | |
| 5.7878 | 5.7876 | 5.3945 | |
| 6.6256 | 6.6254 | 6.1156 | |
| 7.4441 | 7.4438 | 6.8174 | |
| 8.2532 | 8.2529 | 7.5666 | |
| 9.0578 | 9.0574 | 8.3111 | |
| 9.8600 | 9.8596 | 9.0534 | |
| 10.6612 | 10.6607 | 9.7945 | |
| 11.4617 | 11.4613 | 10.5351 | |
| 12.2620 | 12.2615 | 11.2753 | |
| 13.0622 | 13.0616 | 12.0155 | |
| 13.8622 | 13.8616 | 12.7556 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, D.; Gao, J.; Wei, D. A New Belief Entropy Based on Deng Entropy. Entropy 2019, 21, 987. https://doi.org/10.3390/e21100987
Wang D, Gao J, Wei D. A New Belief Entropy Based on Deng Entropy. Entropy. 2019; 21(10):987. https://doi.org/10.3390/e21100987
Chicago/Turabian StyleWang, Dan, Jiale Gao, and Daijun Wei. 2019. "A New Belief Entropy Based on Deng Entropy" Entropy 21, no. 10: 987. https://doi.org/10.3390/e21100987
APA StyleWang, D., Gao, J., & Wei, D. (2019). A New Belief Entropy Based on Deng Entropy. Entropy, 21(10), 987. https://doi.org/10.3390/e21100987




