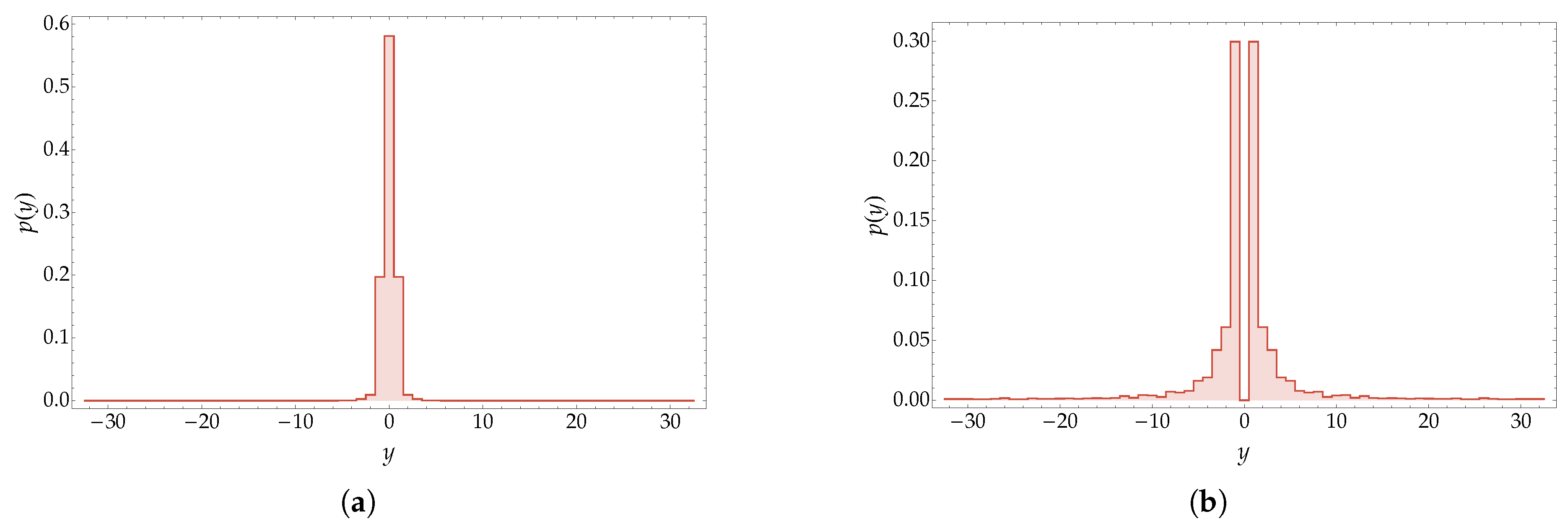1. Introduction
Quantum walks (QWs) describe the evolution of one-particle quantum states on a lattice, or, more generally, on a graph. The quantum walk evolution is linear in the quantum state and the quantum aspect of the evolution occurs in the interference between the different paths available to the walker. There are two kinds of quantum walks: continuous time QWs, where the evolution operator of the system given in terms of an Hamiltonian can be applied at any time (see Farhi et al. [
1]), and discrete-time QWs, where the evolution operator is applied in discrete unitary time-steps. The discrete-time model, which appeared already in the Feynman discretization of the Dirac equation [
2], was later rediscovered in quantum information [
3,
4,
5,
6,
7], and proved to be a versatile platform for various scopes. For example, QWs have been used for empowering quantum algorithms, such as database search [
8,
9], or graph isomorphism [
10,
11]. Moreover, quantum walks have been studied as a simulation tool for relativistic quantum fields [
12,
13,
14,
15,
16,
17,
18,
19,
20,
21,
22,
23,
24,
25,
26,
27,
28], and they have been used as discrete models of spacetime [
29,
30,
31,
32].
QWs are among the most promising quantum simulators with possible realizations in a variety of physical systems, such as nuclear magnetic resonance [
33,
34], trapped ions [
35], integrated photonics, and bulk optics [
36,
37,
38,
39].
New research perspectives are unfolding in the scenario of multi-particle interacting quantum walks where two or more walking particles are coupled via nonlinear (in the field) unitary operators. The properties of these systems are still largely unexplored. Both continuous-time [
40] and discrete-time [
41] quantum walks on sparse unweighted graphs are equivalent in power to the quantum circuit model. However, it is highly non-trivial to design a suitable architecture for universal quantum computation based on quantum walks. Within this perspective, a possible route has been suggested in [
42] based on interacting multi-particle quantum walks with indistinguishable particles (Bosons or Fermions), proving that “almost any interaction” is universal. Among the universal interacting many-body systems are the models with coupling term of the form
, with
the number operator at site
x. The latter two-body interaction lies at the basis of notable integrable quantum systems in one space dimension such as the Hubbard and the Thirring Hamiltonian models.
The first attempts at the analysis of interacting quantum walks were carried out in [
43,
44]. More recently, in [
45], the authors proposed a discrete-time analogue of the Thirring model, which is indeed a Fermionic quantum cellular automaton, whose dynamics in the two-particle sector reduces to an interacting two-particle quantum walk. As for its Hamiltonian counterpart, the discrete-time interacting walk has been solved analytically in the case of two Fermions. Analogously to any Hamiltonian integrable system, also in the discrete-time case the solution is based on the Bethe Ansatz technique. However, discreteness of the evolution prevents the application of the usual Ansatz, and a new Ansatz has been introduced successfully [
45].
In this paper, we present an original simplified derivation of the solution of [
45], which exploits the symmetries of the interacting walk. We present the diagonalization of the evolution operator and the characterization of its spectrum. We explicitly write the two particle states corresponding to the scattering solutions of the system, having eigenvalues in the continuous spectrum of the evolution operator. We then show how the present model predicts the formation of bound states, which are eigenstates of the interacting walk corresponding to the discrete spectrum. We provide also in this case the analytic expression of such molecular states.
We comment on the phenomenological differences between the Hamiltonian model and the discrete-time one. First, we see that the set of possible scattering solutions is larger in the discrete-time case: for a fixed value total momentum, a non trivial transfer of relative momentum can occur besides the simple exchange of momentum between the two particles, differently from the Hamiltonian case. In addition, the family of bound states appearing in the discrete-time scenario is larger than the corresponding Hamiltonian one. Indeed, for any fixed value of the coupling constant, a bound state exists with any possible value of the total momentum, while, for Hamiltonian systems, bound states cannot have arbitrary total momentum.
Finally, we show that, in the set of solutions for the interacting walk, there are perfectly localized states (namely, states that lie on a finite number of lattice sites). Moreover, differently from the Hamiltonian systems, bound states exist also for null coupling constants; however, this is true only for finitely many isolated values of the total momentum. In addition to the exact analytical solution of the dynamics, we show the simulation of some significant initial states.
2. The Dirac Quantum Walk
In this section, we review the Dirac quantum cellular automaton on the line describing the free evolution of a two-component Fermionic field. The single particle Hilbert space is given by
for which we employ the factorized basis
, with
and
. The Dirac automaton describes an arbitrary number of Fermions whose evolution is linear in the field:
where
is a unitary operator. In the single particle sector, the automaton can be regarded as a quantum walk on the single-particle Hilbert space
whose evolution unitary operator
W is given by
where
denotes the translation operator on
, defined by
.
Since the walk
W is translation invariant (it commutes with the translation operator), it can be diagonalized in momentum space. In the momentum representation, defining
, with
, the walk operator can be written as
where
. The spectrum of the walk is given by
, where the dispersion relation
is given by
where Arccos denotes the principal value of the arccosine function. The single-particle eigenstates, solving the eigenvalue problem
can be conveniently written as
with
,
.
3. The Thirring Quantum Walk
In this section, we present a Fermionic cellular automaton in one spatial dimension with an on-site interaction, namely two particles interact only when they lie at the same lattice site. The linear part corresponds to the Dirac QW [
17] and the interaction term is the most general number-preserving coupling in one dimension [
46]. The same kind of interaction characterizes also the most studied integrable quantum systems, such as the Thirring [
47] and the Hubbard [
48] models.
The linear part of the automaton is given by the Dirac automaton, describing the free evolution of the particles. In order to introduce an interaction, we modify the evolution operator adding an extra unitary step of the form:
where
,
, represents the particle number at site
x, namely
, and
is a real coupling constant. Since the interaction term preserves the total number operator, we can study the automaton for a fixed number of particles. For
N interacting particles, we can describe the evolution in terms of an interacting quantum walk over
with the free evolution given by
.
In this work, we focus on the two-particle sector whose solutions has been derived in [
45]. As we will see, the Thirring walk features molecule states besides scattering solutions. This features is shared also by the Hadamard walk with the same on-site interaction [
44].
, acting on the Hilbert space
and describing the free evolution of the particles. In order to introduce an interaction, we modify the update rule of the walk with an extra step
:
. In the present case, the term
has the form
Since we focus on the solutions involving the interaction of two particles, it is convenient to write the walk in the centre of mass basis , with , and . Therefore, on this basis, the generic Fermionic state is with . Notice that only the pairs with y and w, both even or odd, correspond to physical points in the original basis .
We define the two-particle walk with both
y and
w in
, so that the linear part of walk can be written as
where
represents the translation operator in the relative coordinate
y, and
the translation operator in the centre of mass coordinate
w, whereas the interacting term reads
This definition gives a walk that can be decomposed into two identical copies of the original walk. Indeed, defining as C the projector on the physical center of mass coordinates, one has , where and are unitarily equivalent. We will then diagonalize the operator , reminding readers that the physical solutions will be given by projecting the eigenvectors with C.
Introducing the (half) relative momentum
and the (half) total momentum
, the free evolution of the two particles is written in the momentum representation as
where the matrix
is given by
Furthermore, we introduce the vectors
, with
, such that
where
is the dispersion relation of the two-particle walk. Explicitly, the vectors
are given by
We focus in this work on Fermionic solutions satisfying the eigenvalue equation
with
. In the centre of mass basis, the antisymmetry condition reads
E being the exchange matrix
4. Symmetries of the Thirring Quantum Walk
The Thirring walk manifests some symmetries that allow for simplifying the derivation and the study of the solutions. First of all, as we already mentioned, one can show that the interaction commutes with the total number operator. This means that one can study the walk dynamics separately for each fixed number of particles. We focus here on the two-particle walk , where and .
Since the interacting walk
commutes with the translations in the centre of mass coordinate
w, the total momentum is a conserved quantity, so it is convenient to study the walk parameterized by the total momentum
p. To this end, we consider the basis
, so that, for fixed values of
p, the interacting walk of two particles can be expressed in terms of a one-dimensional QW
with a four-dimensional coin:
Although the range of the variable
p is the interval
, it is possible to show that one can restrict the study of the walk to the interval
. On the one hand, the two-particle walk transforms unitarily under a parity transformation in the momentum space. Starting from the single particle walk,
transforms under a parity transformation as
so that, for the two-particle walk, we have the relation
On the other hand, a translation of
of the total momentum
p entails that
while the interaction term remains unaffected in both cases.
The Thirring walk features also another symmetry that can be exploited to simplify the derivation of the solutions. It is easy to check that the walk operator
commutes with the projector defined by
where
and
are the projectors on the even and the odd subspaces, respectively:
The projector P induces a splitting of the total Hilbert space in two subspaces P and , with the interaction term acting non-trivially only in the subspace P. In the complementary subspace , the evolution is free for Fermionic particles. This means that solutions of the free theory are also solutions of the interacting one, as opposed to the Bosonic case for which the interaction is non-trivial also in .
5. Review of the Solutions
We focus in this section on the antisymmetric solutions of the Thirring walk that actually feel the interaction. From the remarks that we have made in the previous section, such solutions can only be found in the subspace
P. Formally, we have to solve the eigenvalue equation
, with
. Conveniently, we write a vector
in the form
and the antisymmetry condition becomes:
The restriction of the walk to the subspace
P entails that the eigenvalue problem is equivalent to the following system of equations:
The most general solution of Equation (
28) for
has two forms:
and
with
,
, and
satisfying the condition
Solving Equation (
28) corresponds now to find the function
. Let us now study the equation
Since
has to be an eigenvalue of
,
must be real and thus
or
with
, so we conveniently define the sets:
It is easy to see that
for all
s,
r and
l, and the range of the function
covers the entire unit circle except for the points
. Therefore, we can discuss separately the case
and the case
. A solution with
actually exists, corresponding to the function of Equation (
29), and it will be discussed in
Section 5.3.
Let us start with the case , which will lead to the characterization of the continuous spectrum of the Thirring walk and of the scattering solutions.
5.1. Scattering Solutions
In this section, we assume
with
. This implies that
: indeed, as one can notice from
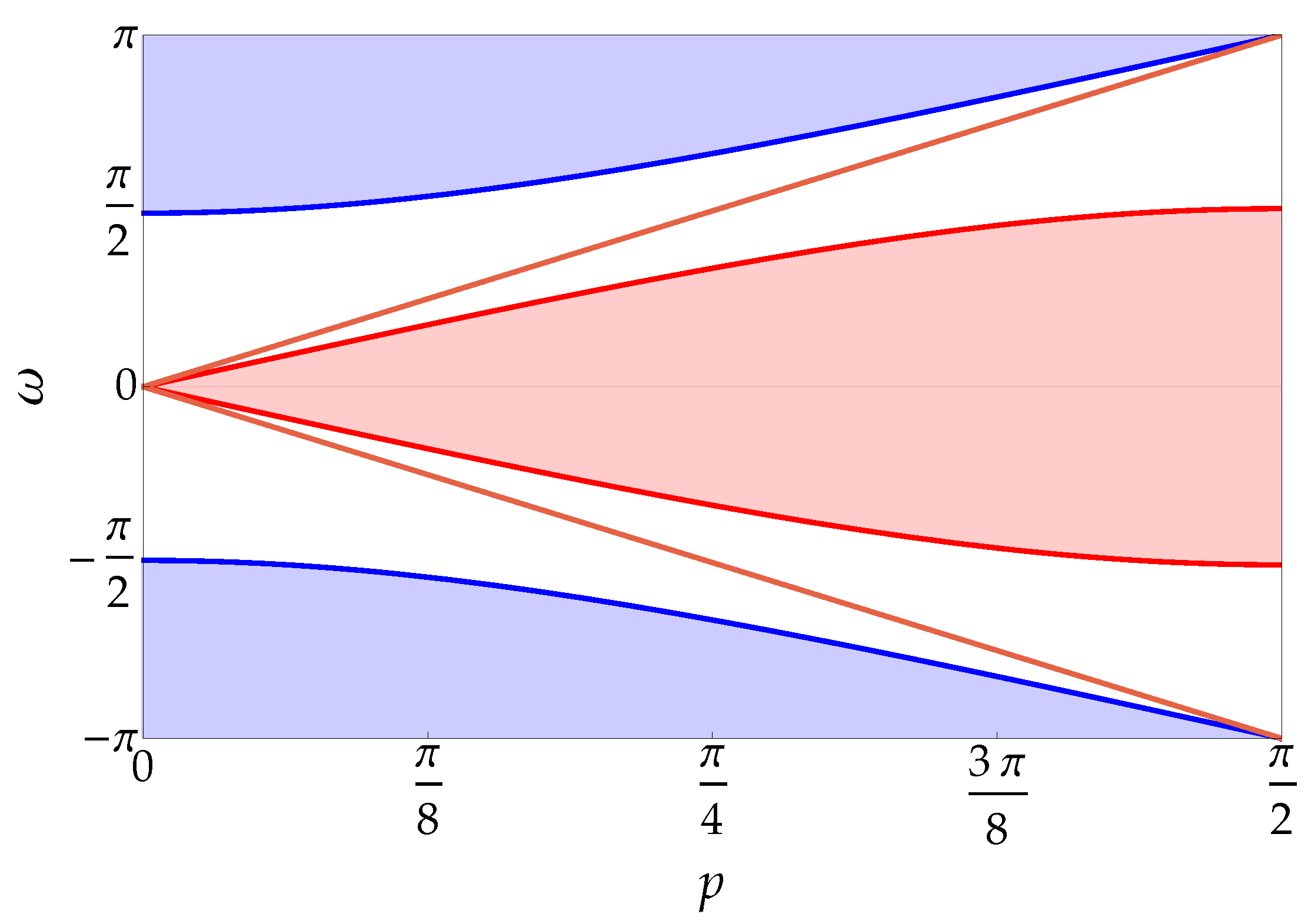
Figure 1, the lines
lie entirely in the gaps between the curves
and
. The solution is thus the one given in Equation (
30). One can prove that
and
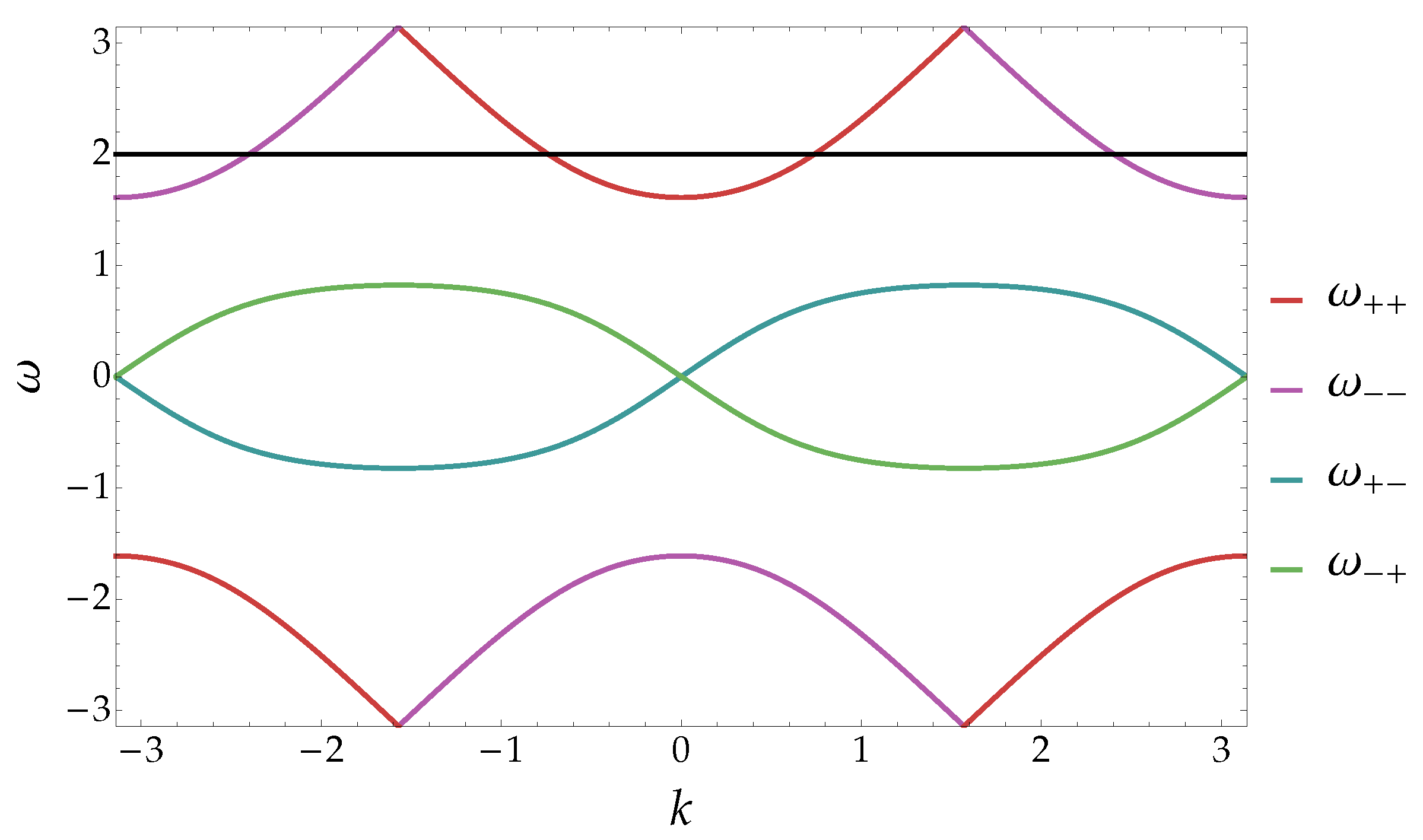
. Furthermore, as one can notice from
Figure 2, there are four values of the triple
such that
for a given value of
: if the triple
is a solution, so are
,
and
; and if
is a solution, then also
,
and
are solutions. This result greatly simplifies Equation (
30). Indeed, the sum over
and the integral over
k reduces to the sum of four terms:
As we will see, the original problem can be simplified in this way to an algebraic problem with a finite set of equations. We note that the fact that the equation has a finite number of solutions is a consequence of the fact that we are considering a model in one spatial dimension. However, in analogous one-dimensional Hamiltonian models (e.g., the Hubbard model), the degeneracy of the eigenvalues is two.
Let us consider for the sake of simplicity the solution of the kind
, since the other one can be analysed in a similar way. Using the notation of
Appendix, Equation (
35) reduces to the expressions (dropping the + superscript)
We notice that now the number of unknown parameters is further reduced to three, namely
,
, and
. Clearly, one of the parameters can be fixed by choosing arbitrarily the normalization. From now on, we fix
and define
. Equation (
36) has to satisfy the recurrence relations of Equation (
28) for
and
, while, for
it is automatically satisfied. For
, Equation (
28) becomes
Starting from Equation (
37), we can notice that
, where we employed the notation of
Appendix A, so that we obtain
. We can then substitute this expression in Equation (
39) and use the relations
to obtain the expression
and thus
For these values of
and
one can verify that Equation (
28) is satisfied also for
, thus concluding the derivation. For the solution of the kind
we can follow a similar reasoning, obtaining the analogous quantity
:
It is worth noticing that is of unit modulus for .
The final form of the solution results in being:
which in terms of the relative coordinate
y can be written as
We can interpret such a solution as a scattering of plane waves for which the coefficient
plays the role of the transmission coefficient. Being the total momentum a conserved quantity, the two particles can only exchange their momenta, as expected from a theory in one dimension. Furthermore, for each value
k of the relative momentum, the two particles can also acquire an additional phase of
. As the interaction is a compact perturbation of the free evolution, the continuous spectrum is the same as that of the free walk. Equation (
46) provides the generalized eigenvector if
corresponding to the continuous spectrum
.
5.2. Bound States
In the previous section, we derived the solutions in the continuous spectrum, which can be interpreted as scattering plane waves in one spatial dimension. We seek now the solutions corresponding to the discrete spectrum, namely solutions with eigenvalues in any one of the sets
. The derivation of the solution follows similar steps as for the scattering solutions. In particular, the degeneracy in
k is the same: there are four solutions to the equation
even in this case, as proved in [
45]. Therefore, the general form of the solution in this case can be written again as in Equation (
35) and, following the same reasoning, one obtains the same set of solutions as in Equation (
46). At this stage, we did not impose that the solution is a proper eigenvector in the Hilbert space
. To this end, we have to set
to eliminate the exponentially-divergent terms in Equation (
46). As one can prove, the equation
has only one solution for fixed values of
and
p. More precisely, there is a unique
, with
and
, such that either
or
.
In other words, for each pair of values
the walk
has one and only one eigenvector corresponding to an eigenvalue in the point spectrum. Such eigenvector can be written as
where
is the solution of
or
and ± chosen accordingly. More compactly, in the
y coordinate, the solution can be written as
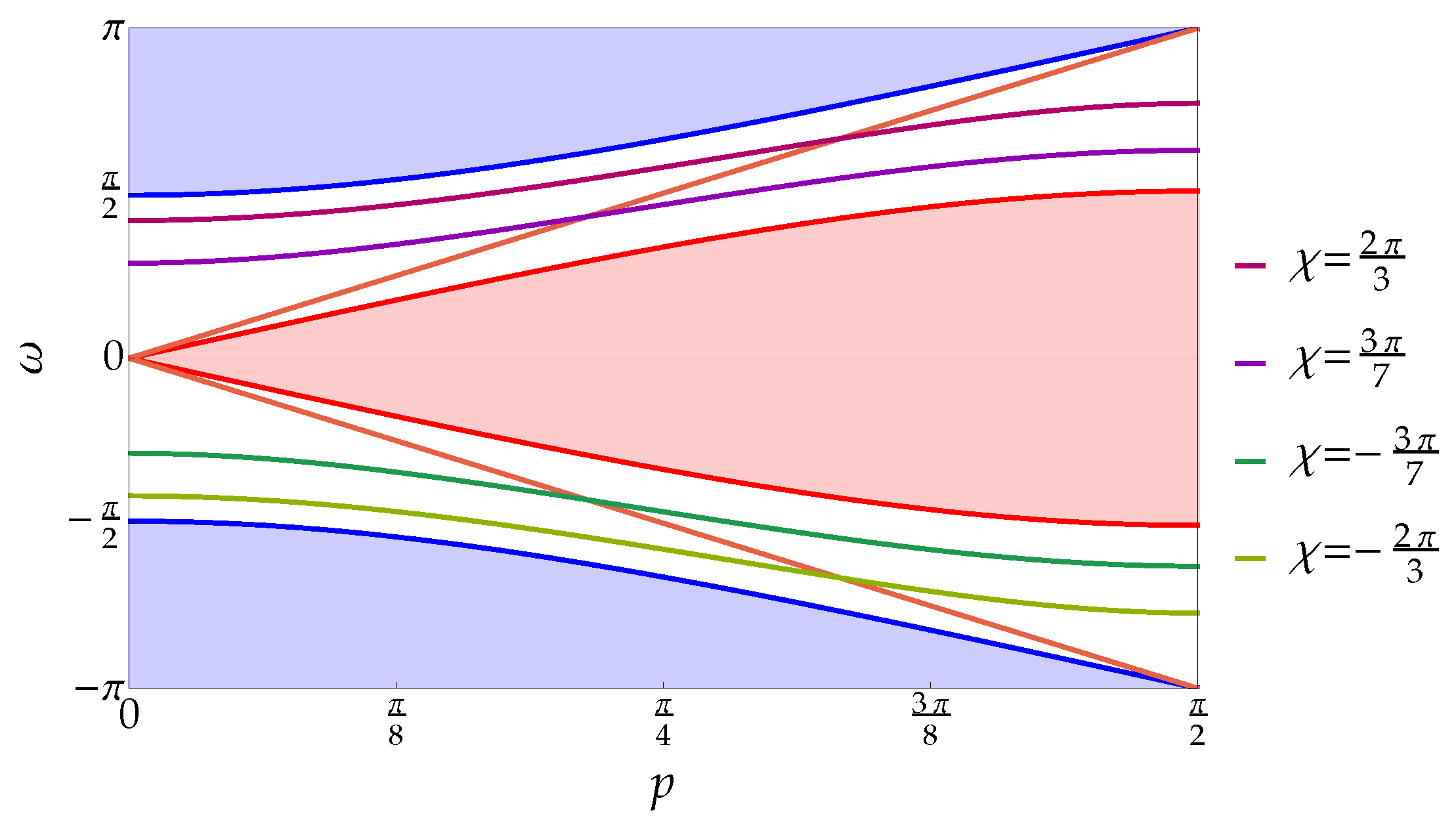
In
Figure 3, the discrete spectrum of the interacting walk together with the continuous spectrum as a function of the total momentum
p is depicted. The solid curves in the gaps between the continuous bands denote the discrete spectrum for different values of the coupling constant
. Molecule states appear also in the Hadamard walk with the same on-site interaction [
44].
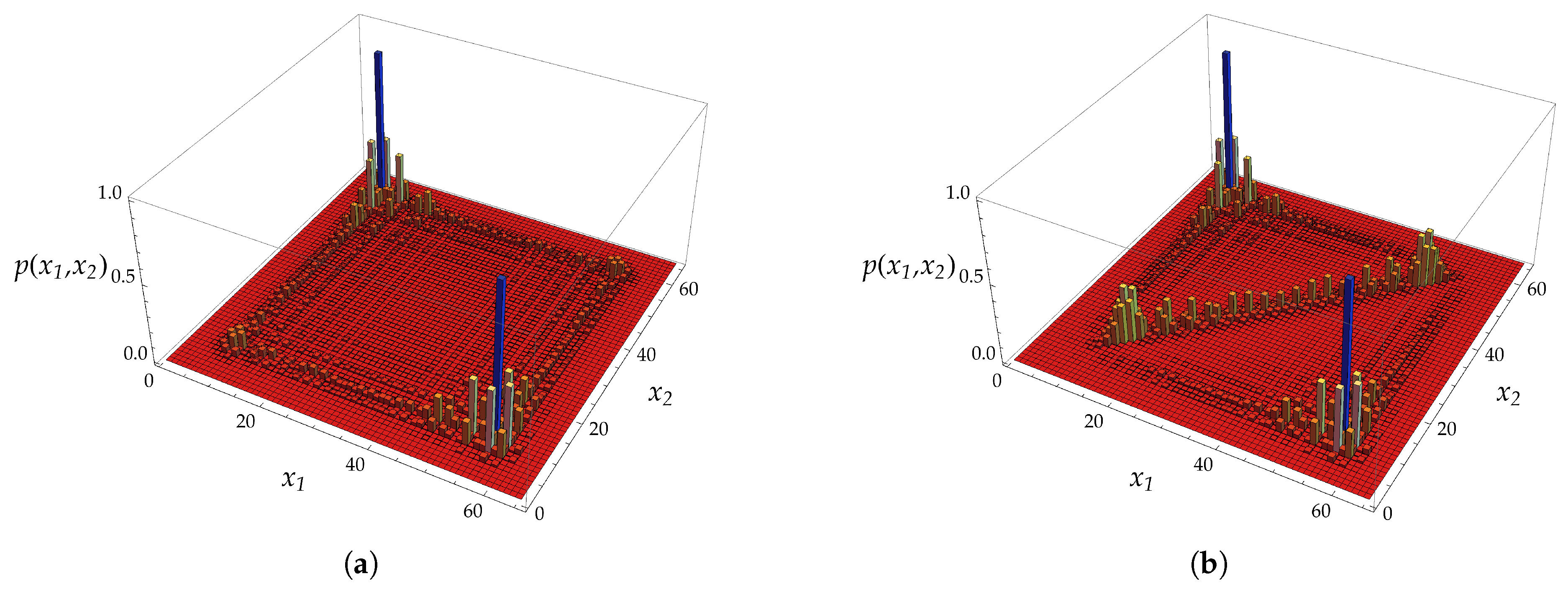
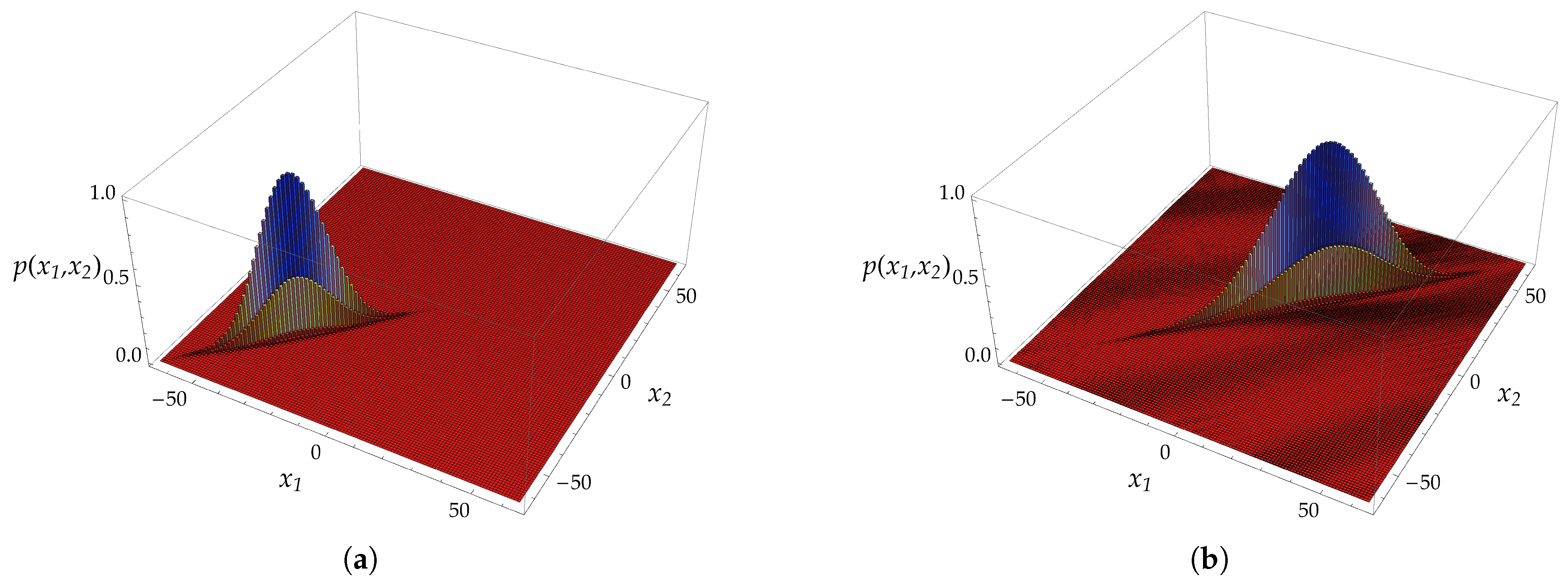
Referring to
Figure 4, we show the evolution of two particles initially prepared in a singlet state localized at the origin. From the figure, one can appreciate the appearance of the bound state component that has non-vanishing overlapping with the initial state. The bound state, being exponentially decaying in the relative coordinate
y, is localized on the diagonal of the plot, that is when the two particles lie at the same point.
In
Figure 5, the probability distribution of the bound state corresponding to a choice of parameters
and
is depicted. The plot highlights the exponential decay of the tails, which is the characterizing feature of the bound state.
5.3. Solution for
Thus far, we have studied proper eigenvectors that decay exponentially as the two particles are further apart. However, the previous analysis failed to cover the particular case when , since the range of does not include the two points of the unit circle .
We now study the solutions with
having the form given in Equation (
29). One can prove that such solutions are non-vanishing only for
on
P, namely we look for a solution of the form
Subtracting the first and the last equations of (
28) using (
50), we obtain the following equation:
If both
and
are non-zero, one can prove that a solution does not exist and thus we have to consider the two cases
and
separately. Starting from
, Equation (
51) imposes that
, meaning that, if a solution exists in this case, it is an eigenvector corresponding to the eigenvalue
. From the second equation of (
28), we obtain the relation
and, using the first equation of (
28), it turns out that a solution exists only if
, as expected, since, otherwise, the case of
Section 5.2 would have held. The other case, namely
, can be studied analogously. Let us, then, denote as
such proper eigenvectors with eigenvalue
for
and, choosing
as the value for the free parameter
, we obtain the following expression for
:
Such solutions provide a special case of molecule states (namely, proper eigenvectors of ), being localized on few sites, and differ from the previous solutions showing an exponential decay in the relative coordinate.
5.4. Solutions for
The solutions that we presented in the previous discussion do not cover the extreme values
(see [
45] for a reference). Let us consider for definiteness the case
, since the other case is obtained in a similar way. For
, the previous analysis still holds. Indeed, noticing that
, we have
and
if and only if
, whereas
for all
. This means that the solutions
of Equation (
46) are actually eigenvectors of
. Thus, the spectrum is made by a continuous part, given by the arc of the unit circle containing
and having
as extremes, and a point spectrum with two points:
, where
is the solution of
for
, and 1. As shown in [
45], 1 is a separated part of the spectrum of
and the corresponding eigenspace is a separable Hilbert space of stationary bound states. This fact underlines an important feature of the Thirring walk not shared by analogous Hamiltonian models. It is remarkable that this behaviour occurs also for the free walk with
. In
Figure 6, we show the probability distribution of two states having the properties hereby discussed. It is worth noticing that all the states
with
are eigenvectors relative to the eigenvalue 1, and thus they generate a subspace on which the walk acts identically. We remark that this behaviour relies on the fact that the dispersion relation in one dimension is an even function of
k.
6. Conclusions
In this work, we reviewed the Thirring quantum walk [
45], providing a simplified derivation of its solutions for Fermionic particles. The simplified derivation relies on the symmetric properties of the walk evolution operator, allowing for separating the subspace of solutions affected by the interaction from the subspace where the interaction step acts trivially. The interaction term is the most general number-preserving interaction in one dimension, whereas the free evolution is provided by the Dirac QW [
17].
We showed the explicit derivation of the scattering solutions (solutions for the continuous spectrum) as well as for the bound-state solutions. The Thirring walk features also localized bound states (namely, states whose support is finite on the lattice) when
. Such solutions exist only when the coupling constant is
.
Figure 4 depicts the evolution of a perfectly localized state showing the overlapping with bound state components. In
Figure 5, we reported the evolution of a bound state of the two particles peaking around a certain value of the total momentum: one can appreciate that the probability distribution remains localized on the main diagonal during the evolution.
Finally, we showed that bound states exist also for a vanishing coupling constant—even though this is true only for a finite set of values of the total momentum p—which is a striking difference between the discrete model of the present work and corresponding Hamiltonian systems.