Relationship between Entropy and Dimension of Financial Correlation-Based Network
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
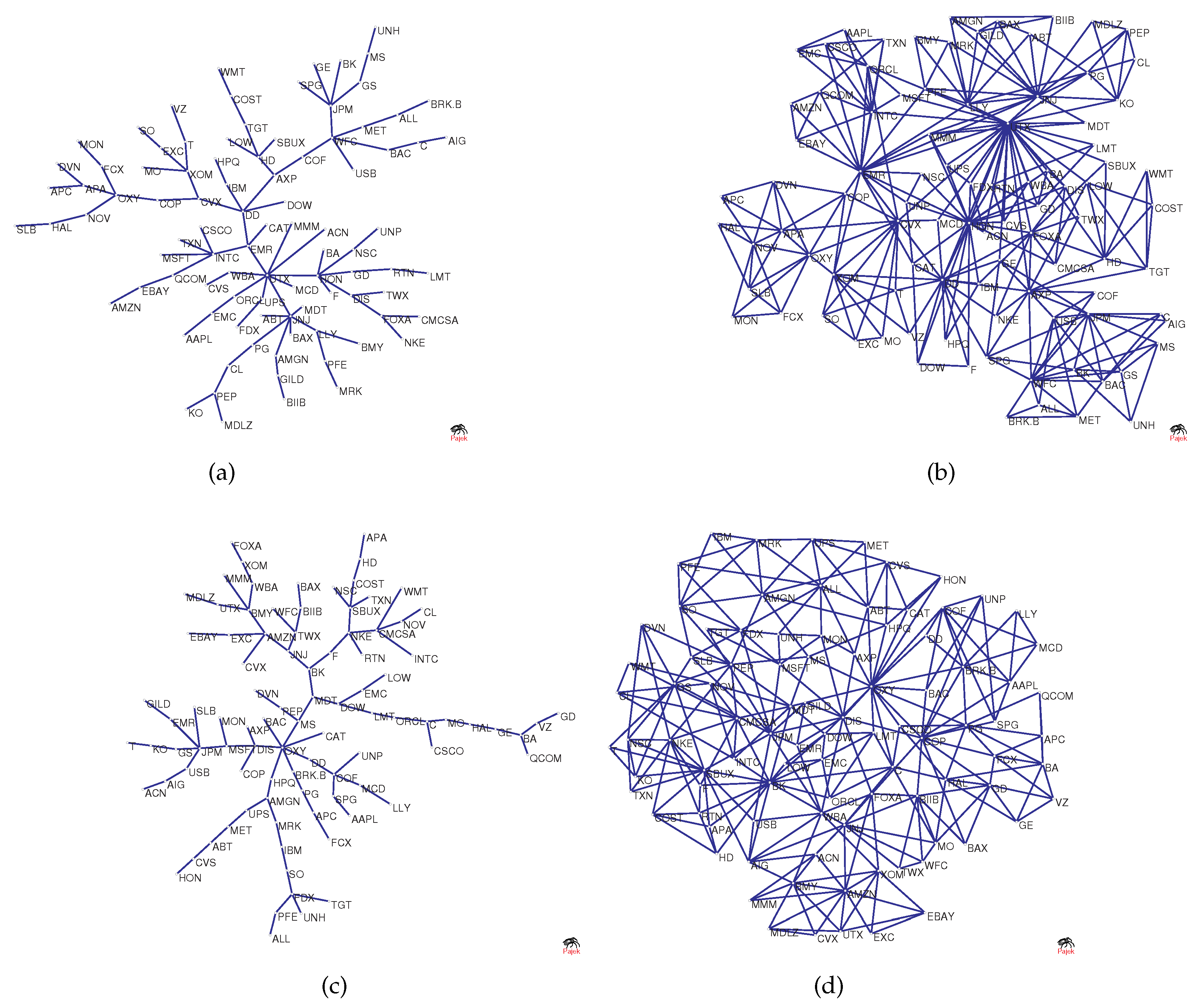
2.2. MST and PMFG
- Pearson’s correlation coefficient between any two stocks i and j is calculated and denoted as (Equation (2)).
- We extract elements of the upper triangular matrix of correlation coefficient matrix and arrange them in ascending order, denoted by .
- In order of , we add a link between the pairs of nodes of an element in when the resulting graph is a planar graph.
- The above step is repeated until a planar graph with edges is generated.
2.3. Rényi Index
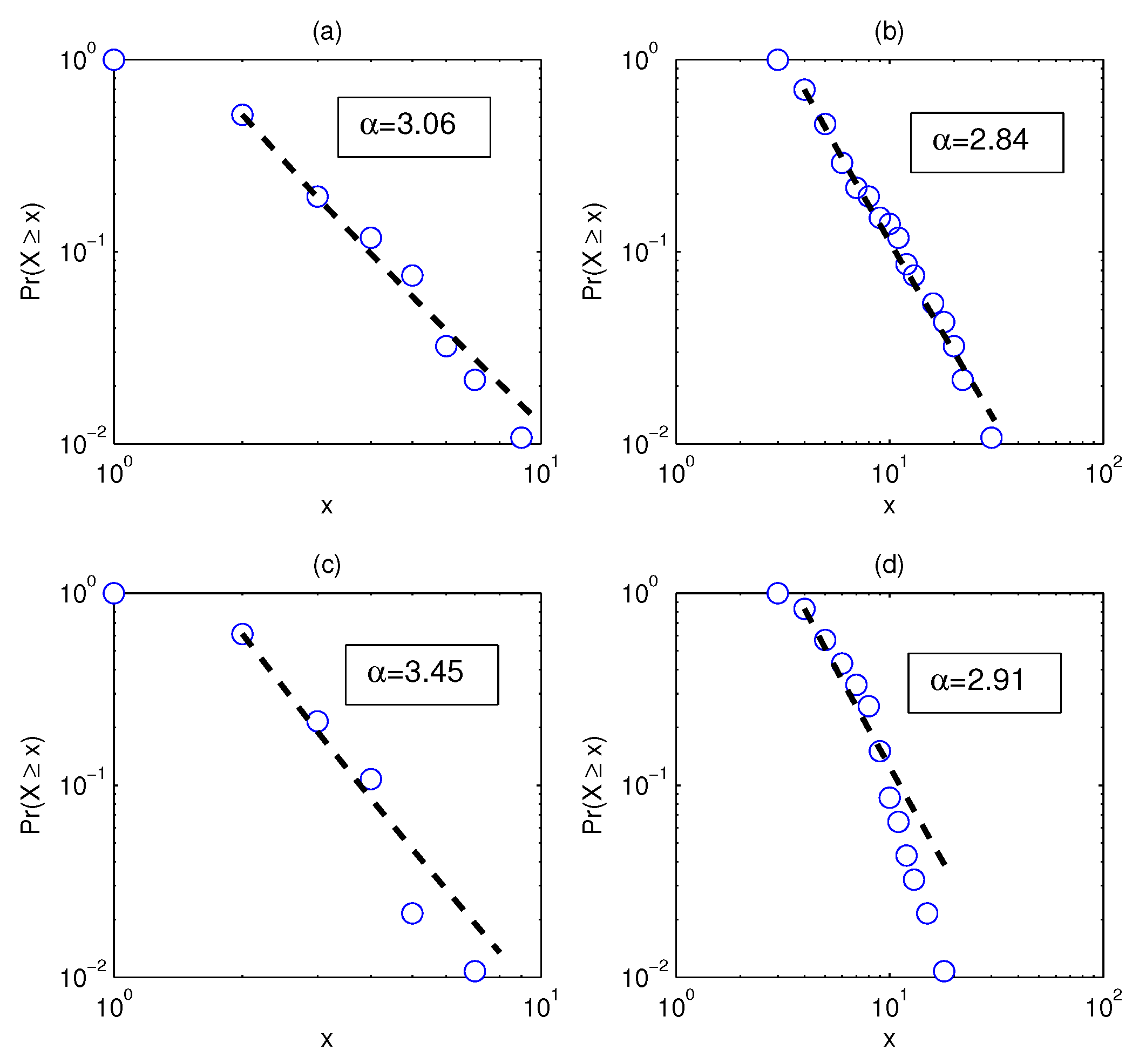
2.4. Dimension
- We calculate MST or PMFG based on distance matrix or correlation coefficient matrix. Here, the correlation-based network is denoted as , where is a node set and is an adjacency matrix.
- The shortest distance matrix is calculated by the adjacency matrix T.
- We set the threshold set and then compute threshold network for , where the elements of .
- The number of non-zero elements in the i-th row of matrix is the volume of node i with distance . That is, the volume of node i is its degree in the threshold network . Further, the volume is calculated usingthat is, the average is calculated.
- If the scaling relationship is asthe volume dimension is defined, where C is a constant and r is the distance.
2.5. Generalized Volume-Based Dimensions
3. Results
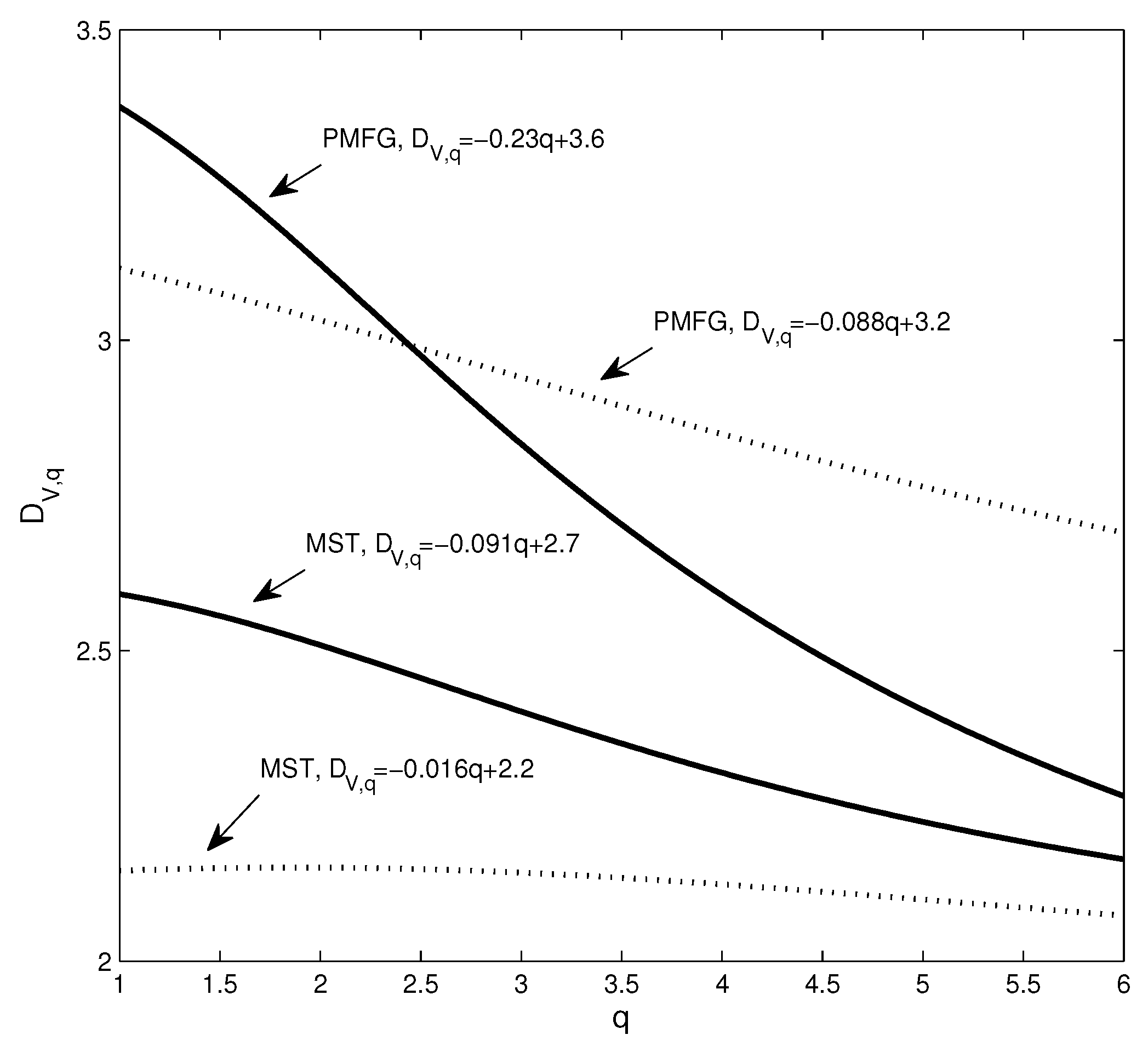
3.1. Generalized Volume-Based Dimensions
3.2. Relationship between the Dimension and the Rényi Index of the Threshold Network
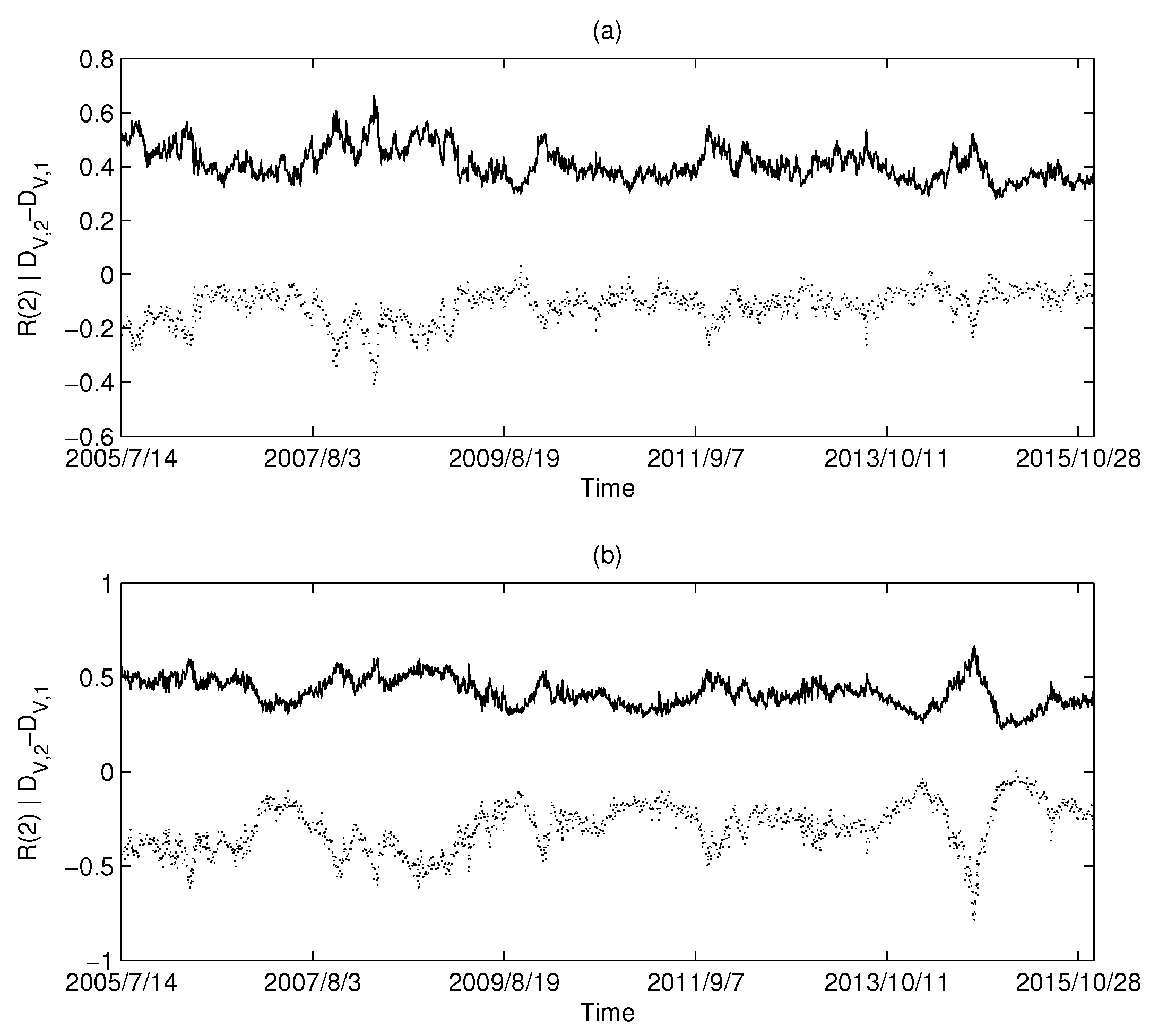
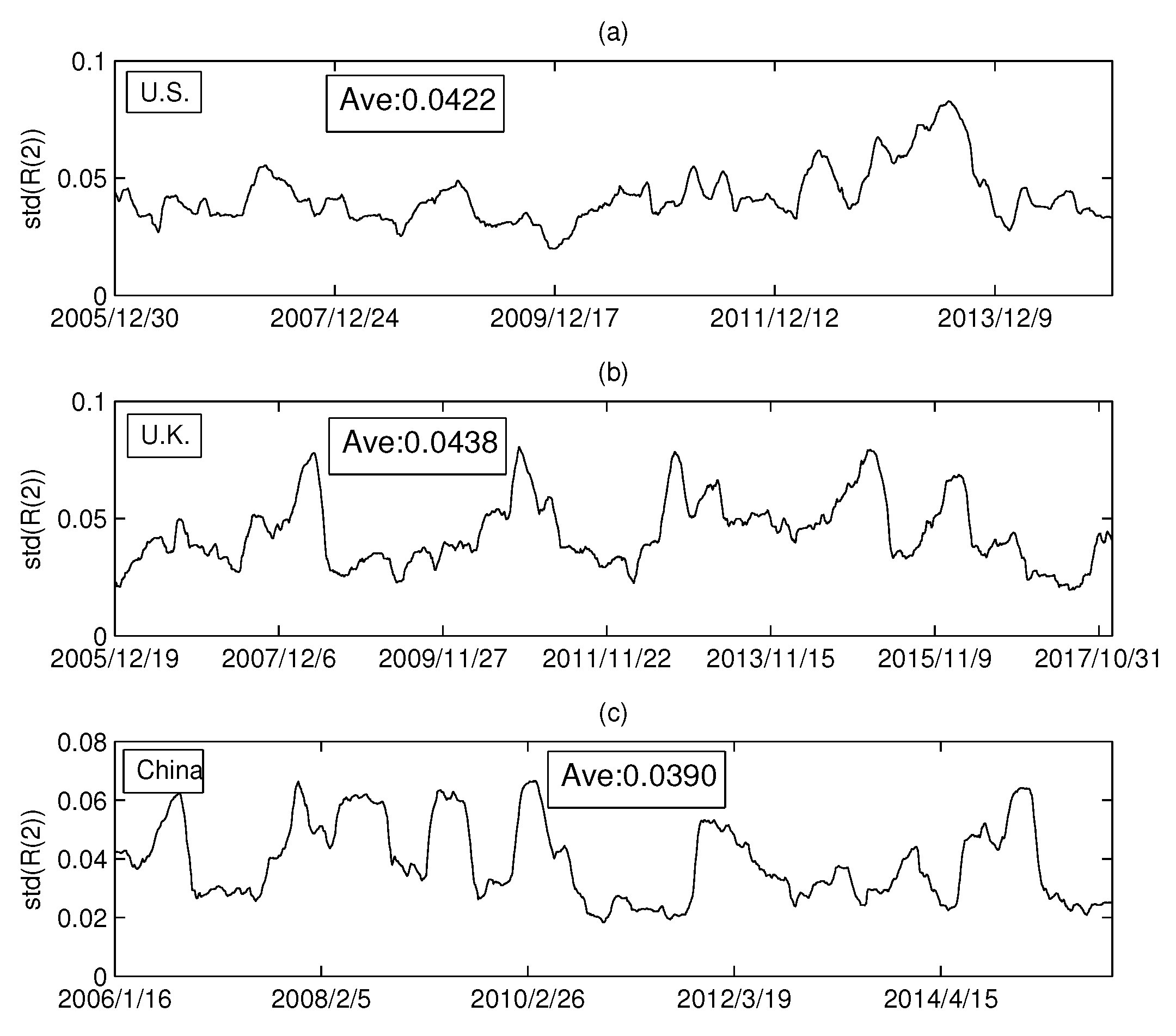
3.3. Empirical Analysis Based on Different Countries
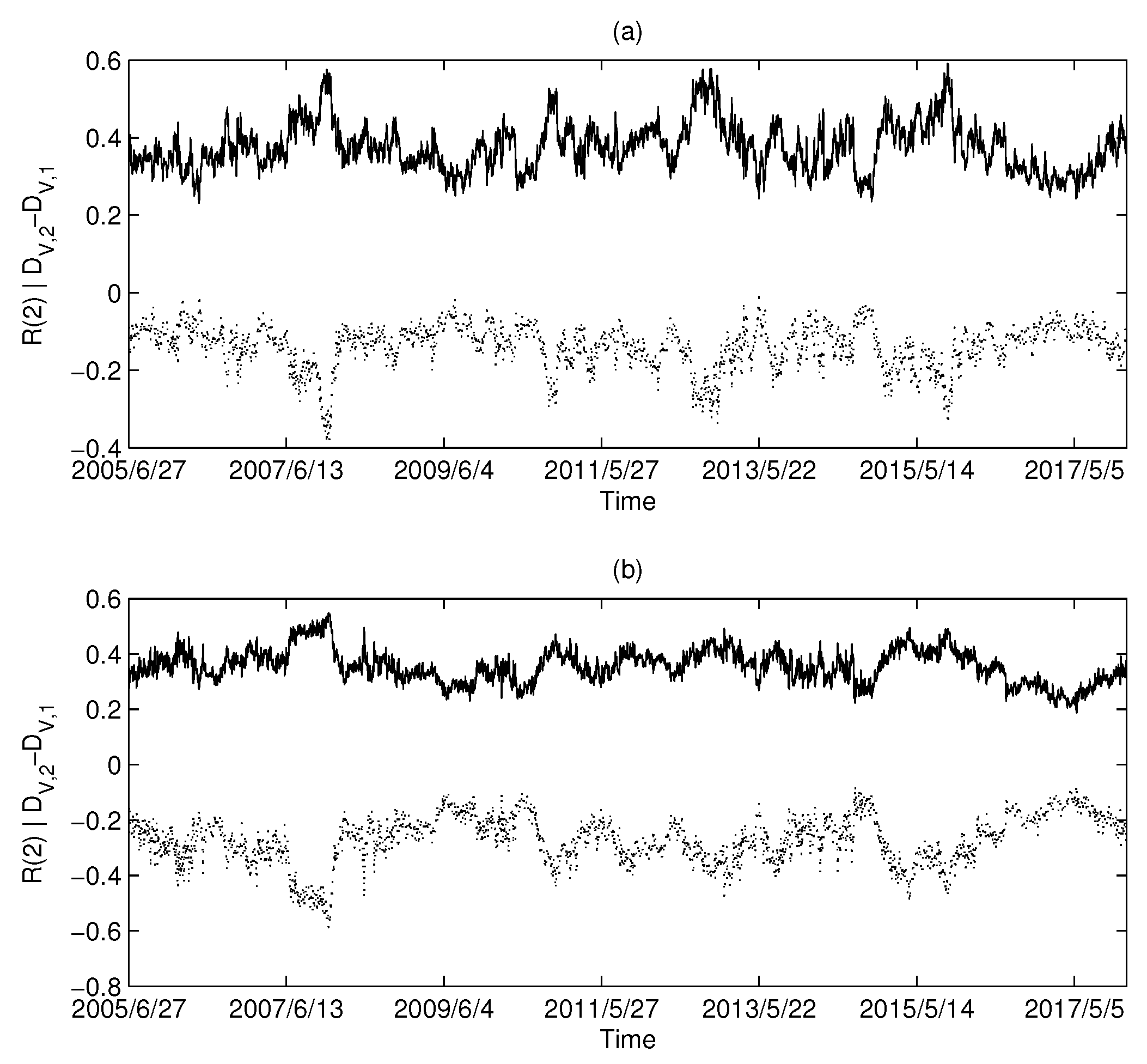
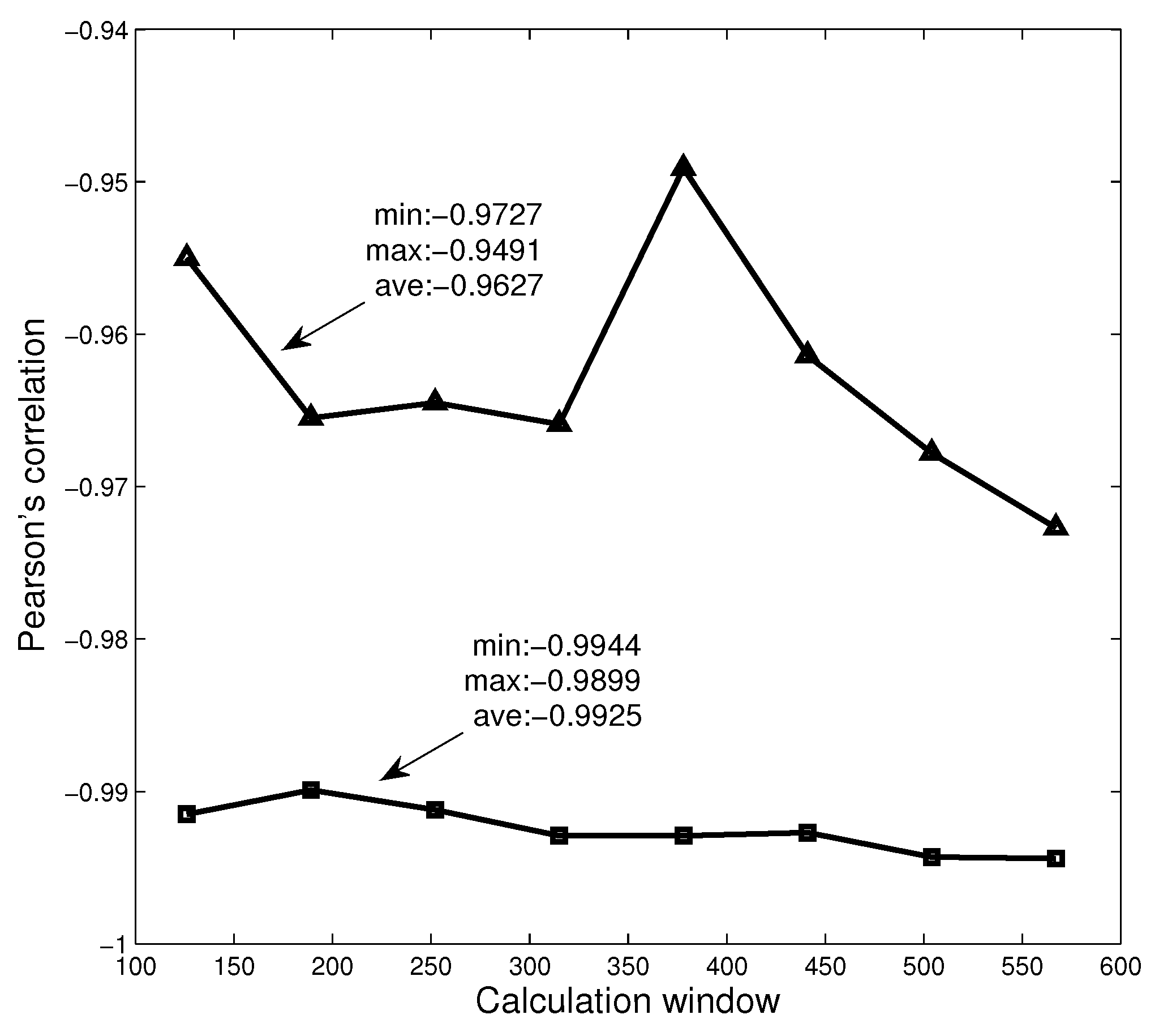
3.4. Robust Analysis of Calculation Window
3.5. The Dynamics of the Rényi Index
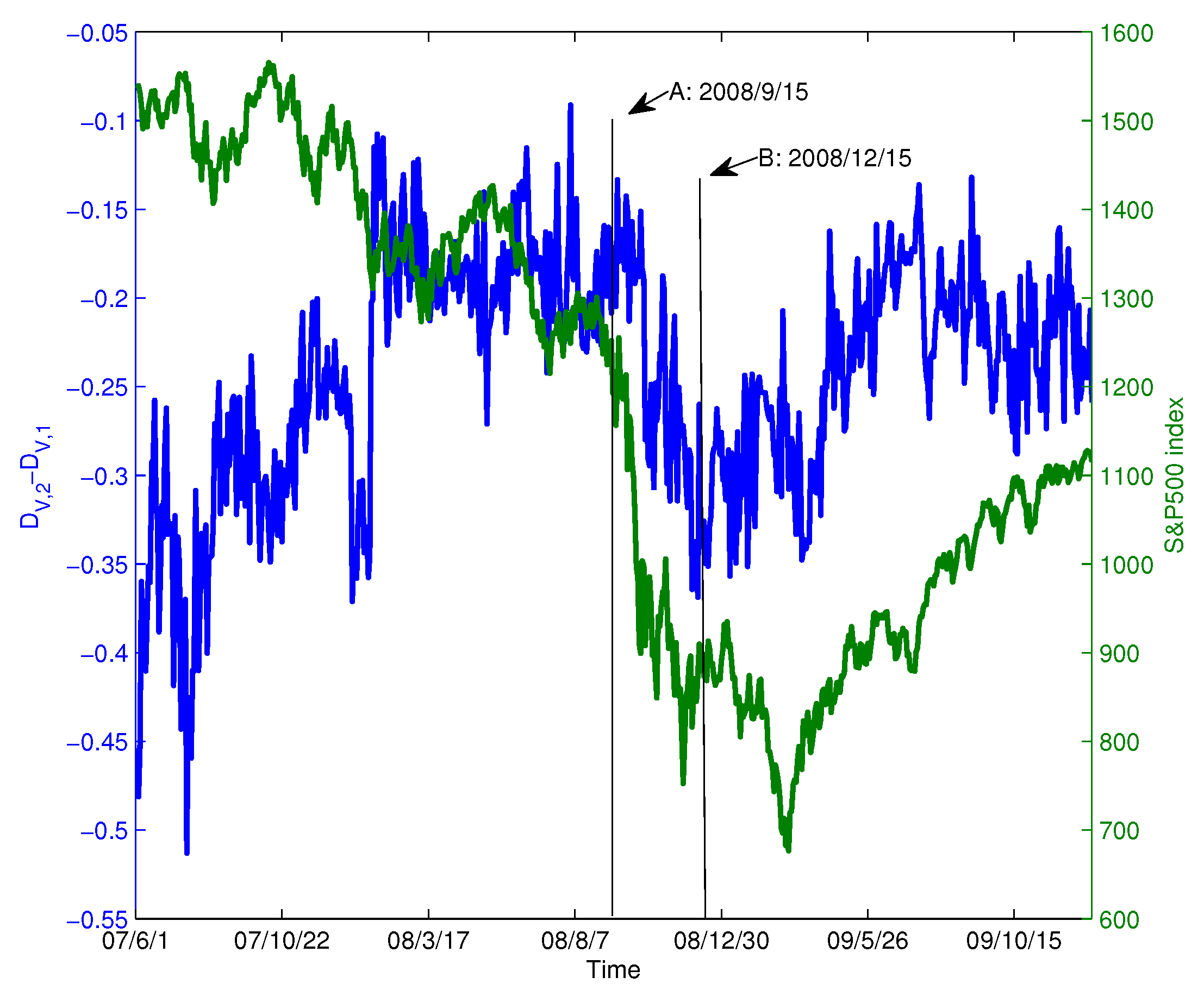
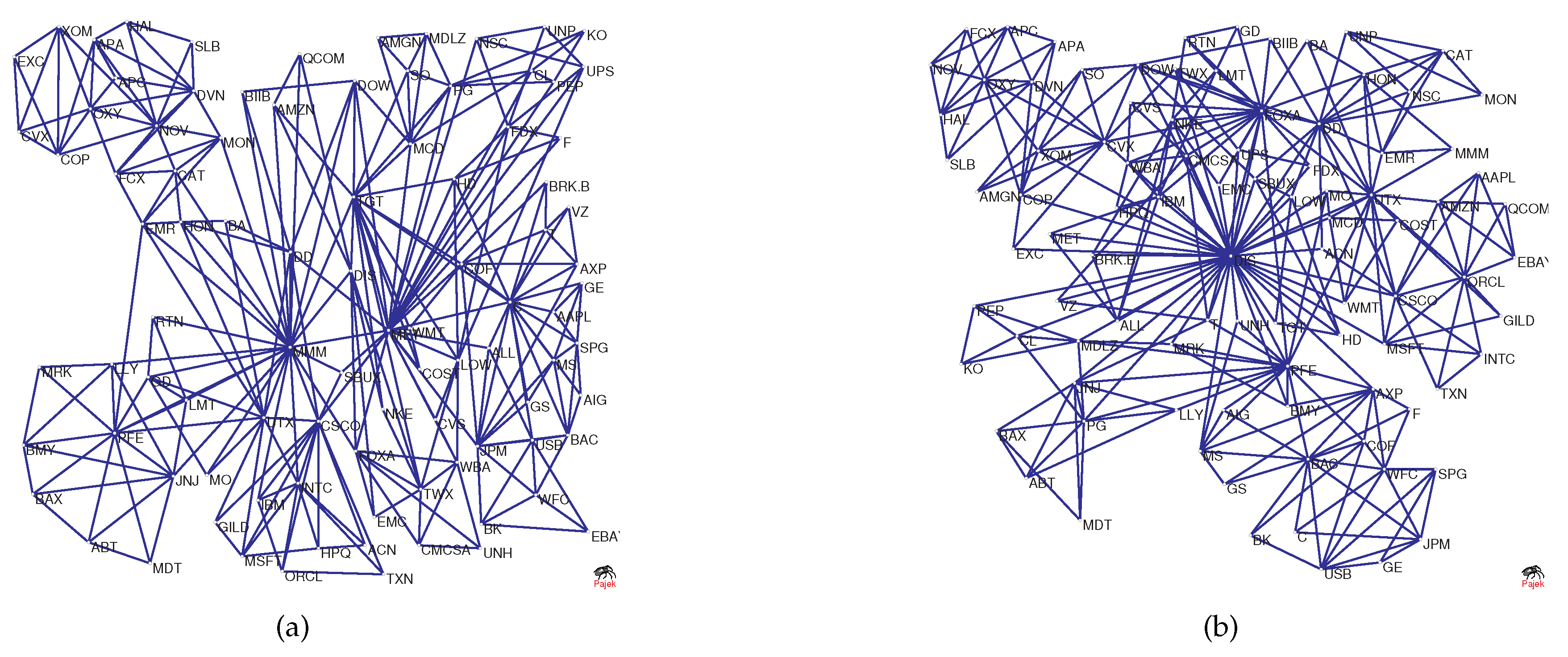
3.6. Example of Volume-Based Dimension Analysis
4. Discussion and Conclusions
4.1. Discussion
4.2. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Boccaletti, S.; Latora, V.; Moreno, Y.; Chavez, M.; Hwang, D.-U. Complex networks: Structure and dynamics. Phys. Rep. 2006, 424, 175–308. [Google Scholar] [CrossRef]
- Newman, M.E.J. The structure and function of complex networks. SIAM Rev. 2006, 45, 167–256. [Google Scholar] [CrossRef]
- Fortunato, S. Community detection in graphs. Phys. Rep. 2010, 486, 75–174. [Google Scholar] [CrossRef]
- Malliaros, F.D.; Vazirgiannis, M. Clustering and community detection in directed networks: A survey. Phys. Rep. 2013, 533, 95–142. [Google Scholar] [CrossRef]
- Zanin, M.; Papo, D.; Sousa, P.A.; Menasalvas, E.; Nicchi, A.; Kubik, E.; Boccaletti, S. Combining complex networks and data mining: Why and how. Phys. Rep. 2016, 635, 1–44. [Google Scholar] [CrossRef]
- Barabási, A.L.; Albert, R. Emergence of Scaling in Random Networks. Science 1999, 286, 509–512. [Google Scholar] [CrossRef] [PubMed]
- Clauset, A.; Shalizi, C.R.; Newman, M.E. Power-law distributions in empirical data. SIAM Rev. 2009, 51, 661–703. [Google Scholar] [CrossRef]
- Song, C.; Havlin, S.; Makse, H.A. Self-similarity of complex networks. Nature 2005, 433, 392–395. [Google Scholar] [CrossRef] [PubMed]
- Wei, D.; Wei, B.; Hu, Y.; Zhang, H.; Deng, Y. A new information dimension of complex networks. Phys. Lett. A 2014, 378, 1091–1094. [Google Scholar] [CrossRef]
- Zhang, Q.; Luo, C.; Li, M.; Deng, Y.; Mahadevan, S. Tsallis information dimension of complex networks. Physica A 2015, 419, 707–717. [Google Scholar] [CrossRef]
- Rosenberg, E. Maximal entropy coverings and the information dimension of a complex network. Phys. Lett. A 2017, 381, 574–580. [Google Scholar] [CrossRef]
- Shanker, O. Defining dimension of a complex network. Modern Phys. Lett. B 2007, 21, 321–326. [Google Scholar] [CrossRef]
- Shanker, O. Graph zeta function and dimension of complex network. Mod. Phys. Lett. B 2007, 21, 639–644. [Google Scholar] [CrossRef]
- Long, G.U.O.; Cai, X.U. The Fractal Dimensions of Complex Networks. Chin. Phys. Lett. 2009, 26, 088901. [Google Scholar] [CrossRef]
- Wei, D.J.; Liu, Q.; Zhang, H.X.; Hu, Y.; Deng, Y.; Mahadevan, S. Box-covering algorithm for fractal dimension of weighted networks. Sci. Rep. 2013, 3, 3049. [Google Scholar] [CrossRef] [PubMed]
- Wang, D.-L.; Yu, Z.-G.; Anh, V. Multifractal analysis of complex networks. Chin. Phys. B 2012, 21, 080504. [Google Scholar] [CrossRef]
- Furuya, S.; Yakubo, K. Multifractality of complex networks. Phys. Rev. E 2011, 84, 036118. [Google Scholar] [CrossRef] [PubMed]
- Liu, J.-L.; Yu, Z.-G.; Anh, V. Determination of multifractal dimensions of complex networks by means of the sandbox algorithm. Chaos 2015, 25, 023103. [Google Scholar] [CrossRef] [PubMed]
- Jalan, S.; Yadav, A.; Sarkar, C.; Boccaletti, S. Unveiling the multi-fractal structure of complex networks. Chaos Solitons Fractals 2017, 97, 11–14. [Google Scholar] [CrossRef]
- Lacasa, L.; Gámez-Gardenes, J. Correlation Dimension of Complex Networks. Phys. Rev. Lett. 2013, 110, 168703. [Google Scholar] [CrossRef] [PubMed]
- Zhao, L.; Li, W.; Cai, X. Structure and dynamics of stock market in times of crisis. Phys. Lett. A 2016, 380, 654–666. [Google Scholar] [CrossRef]
- Birch, J.; Pantelous, A.A.; Soramäki, K. Analysis of correlation based networks representing DAX 30 stock price returns. Comput. Econ. 2016, 47, 501–525. [Google Scholar] [CrossRef]
- Mantegna, R.N. Hierarchical structure in financial markets. Eur. Phys. J. B Condens. Matter Complex Syst. 1999, 11, 193–197. [Google Scholar] [CrossRef]
- Tumminello, M.; Aste, T.; Di Matteo, T.; Mantegna, R.N. A tool for filtering information in complex systems. Proc. Natl. Acad. Sci. USA 2005, 102, 10421–10426. [Google Scholar] [CrossRef] [PubMed]
- Yang, Y.; Yang, H. Complex network-based time series analysis. Physica A 2008, 387, 1381–1386. [Google Scholar] [CrossRef]
- Chi, K.T.; Liu, J.; Lau, F.C. A network perspective of the stock market. J. Empir. Financ. 2010, 17, 659–667. [Google Scholar] [CrossRef]
- Tumminello, M.; Di Matteo, T.; Aste, T.; Mantegna, R.N. Correlation based networks of equity returns sampled at different time horizons. Eur. Phys. J. B 2007, 55, 209–217. [Google Scholar] [CrossRef]
- Buccheri, G.; Marmi, S.; Mantegna, R.N. Evolution of correlation structure of industrial indices of US equity markets. Phys. Rev. E 2007, 88, 012806. [Google Scholar] [CrossRef] [PubMed]
- Matesanz, D.; Ortega, G.J. Network analysis of exchange data: Interdependence drives crisis contagion. Qual. Quant. 2014, 48, 1835–1851. [Google Scholar] [CrossRef] [Green Version]
- Vodenska, I.; Becker, A.P.; Zhou, D.; Kenett, D.Y.; Stanley, H.E.; Havlin, S. Community analysis of global financial markets. Risks 2016, 4, 13. [Google Scholar] [CrossRef]
- Naylor, M.J.; Rose, L.C.; Moyle, B.J. Topology of foreign exchange markets using hierarchical structure methods. Physica A 2007, 382, 199–208. [Google Scholar] [CrossRef]
- Garas, A.; Argyrakis, P. Correlation study of the athens stock exchange. Physica A 2007, 380, 199–208. [Google Scholar] [CrossRef]
- Onnela, J.P.; Chakraborti, A.; Kaski, K.; Kertesz, J.; Kanto, A. Dynamics of market correlations: Taxonomy and portfolio analysis. Phys. Rev. E 2003, 68, 056110. [Google Scholar] [CrossRef] [PubMed]
- Wiliński, M.; Sienkiewicz, A.; Gubiec, T.; Kutner, R.; Struzik, Z.R. Structural and topological phase transitions on the German Stock Exchange. Physica A 2013, 392, 5963–5973. [Google Scholar] [CrossRef]
- Górski, A.Z.; Drożdż, S.; Kwapień, J. Scale free effects in world currency exchange network. Eur. Phys. J. B 2008, 66, 91–96. [Google Scholar] [CrossRef]
- Nie, C.X.; Song, F.T.; Li, S.P. Rényi indices of financial minimum spanning trees. Physica A 2016, 444, 883–889. [Google Scholar] [CrossRef]
- Eliazar, I. Randomness, evenness, and Rényi’s index. Physica A 2011, 390, 1982–1990. [Google Scholar] [CrossRef]
- Eliazar, I.I.; Sokolov, I.M. Measuring statistical evenness: A panoramic overview. Physica A 2012, 391, 1323–1353. [Google Scholar] [CrossRef]
- Maasoumi, E. A compendium to information theory in economics and econometrics. Econ. Rev. 1993, 12, 137–181. [Google Scholar] [CrossRef]
- Zhou, R.; Cai, R.; Tong, G. Applications of entropy in finance: A review. Entropy 2013, 15, 4909–4931. [Google Scholar] [CrossRef]
- Bekiros, S.D. Timescale analysis with an entropy-based shift-invariant discrete wavelet transform. Comput. Econ. 2014, 44, 231–251. [Google Scholar] [CrossRef]
- Gençay, R.; Gradojevic, N. Crash of ’87—Was it expected? Aggregate market fears and long-range dependence. J. Empir. Financ. 2011, 17, 270–282. [Google Scholar] [CrossRef]
- Stutzer, M.J. Simple entropic derivation of a generalized Black-Scholes option pricing model. Entropy 2000, 2, 70–77. [Google Scholar] [CrossRef]
- Kitamura, Y.; Stutzer, M. Connections between entropic and linear projections in asset pricing estimation. J. Econ. 2002, 107, 159–174. [Google Scholar] [CrossRef]
- Bowden, R.J. Directional entropy and tail uncertainty, with applications to financial hazard. Quant. Financ. 2011, 11, 437–446. [Google Scholar] [CrossRef]
- Gradojevic, N.; Caric, M. Predicting systemic risk with entropic indicators. J. Forecast. 2017, 36, 16–25. [Google Scholar] [CrossRef]
- Billio, M.; Casarin, R.; Costola, M.; Pasqualini, A. An entropy-based early warning indicator for systemic risk. J. Int. Financ. Markets Inst. Money 2016, 45, 42–59. [Google Scholar] [CrossRef]
- Maasoumi, E.; Racine, J. Entropy and predictability of stock market returns. J. Econ. 2002, 107, 291–312. [Google Scholar] [CrossRef]
- Sandhu, R.S.; Georgiou, T.T.; Tannenbaum, A.R. Ricci curvature: An economic indicator for market fragility and systemic risk. Sci. Adv. 2016, 2, e1501495. [Google Scholar] [CrossRef] [PubMed]
- Yang, J.; Qiu, W. A measure of risk and a decision-making model based on expected utility and entropy. J. Oper. Res. 2005, 164, 792–799. [Google Scholar] [CrossRef]
- Zeng, Z.J.; Xie, C.; Yan, X.G.; Hu, J.; Mao, Z. Are stock market networks non-fractal? Evidence from New York Stock Exchange. Financ. Res. Lett. 2016, 17, 97–102. [Google Scholar] [CrossRef]
- Prim, R.C. Shortest connection networks and some generalizations. Bell Syst. Tech. J. 1957, 36, 1389–1401. [Google Scholar] [CrossRef]
- Campbell, J.Y.; Lo, A.W.C.; MacKinlay, A.C. (Eds.) Multifactor Pricing Models. In The Econometrics of Financial Markets; Princeton University Press: Princeton, NJ, USA, 1997; pp. 219–251. [Google Scholar]
- Fama, E.F.; French, K.R. Common risk factors in the returns on stocks and bonds. J. Financ. Econ. 1993, 33, 3–56. [Google Scholar] [CrossRef]
- Tumminello, M.; Lillo, F.; Mantegna, R.N. Hierarchically nested factor model from multivariate data. Europhys. Lett. 2007, 78, 30006. [Google Scholar] [CrossRef]
- Ma, J.; Yang, J.; Zhang, X.; Huang, Y. Analysis of Chinese stock market from a complex network perspective: Better to invest in the central. In Proceedings of the 2015 34th Chinese Control Conference (CCC), Hangzhou, China, 28–30 July 2015; Volume 34, pp. 8606–8611. [Google Scholar]
- Bun, J.; Bouchaud, J.P.; Potters, M. Cleaning large correlation matrices: Tools from random matrix theory. Phys. Rep. 2017, 666, 1–109. [Google Scholar] [CrossRef]
- Kenett, D.Y.; Tumminello, M.; Madi, A.; Gur-Gershgoren, G.; Mantegna, R.N.; Ben-Jacob, E. Dominating clasp of the financial sector revealed by partial correlation analysis of the stock market. PLoS ONE 2010, 5, e15032. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Billio, M.; Getmansky, M.; Lo, A.W.; Pelizzon, L. Econometric measures of connectedness and systemic risk in the finance and insurance sectors. J. Financ. Econ. 2012, 104, 535–559. [Google Scholar] [CrossRef]
- Papana, A.; Kyrtsou, C.; Kugiumtzis, D.; Diks, C. Financial networks based on Granger causality: A case study. Physica A 2017, 482, 65–73. [Google Scholar] [CrossRef]
- Isogai, T. Dynamic correlation network analysis of financial asset returns with network clustering. Appl. Netw. Sci. 2017, 2, 8. [Google Scholar] [CrossRef]
- Yin, K.; Liu, Z.; Liu, P. Trend analysis of global stock market linkage based on a dynamic conditional correlation network. J. Bus. Econ. Manag. 2017, 18, 779–800. [Google Scholar] [CrossRef]





© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nie, C.-x.; Song, F.-t. Relationship between Entropy and Dimension of Financial Correlation-Based Network. Entropy 2018, 20, 177. https://doi.org/10.3390/e20030177
Nie C-x, Song F-t. Relationship between Entropy and Dimension of Financial Correlation-Based Network. Entropy. 2018; 20(3):177. https://doi.org/10.3390/e20030177
Chicago/Turabian StyleNie, Chun-xiao, and Fu-tie Song. 2018. "Relationship between Entropy and Dimension of Financial Correlation-Based Network" Entropy 20, no. 3: 177. https://doi.org/10.3390/e20030177
APA StyleNie, C.-x., & Song, F.-t. (2018). Relationship between Entropy and Dimension of Financial Correlation-Based Network. Entropy, 20(3), 177. https://doi.org/10.3390/e20030177






