Finding a Hadamard Matrix by Simulated Quantum Annealing
Abstract
:1. Introduction
1.1. Background
1.2. Finding A Hadamard Matrix
2. Methods
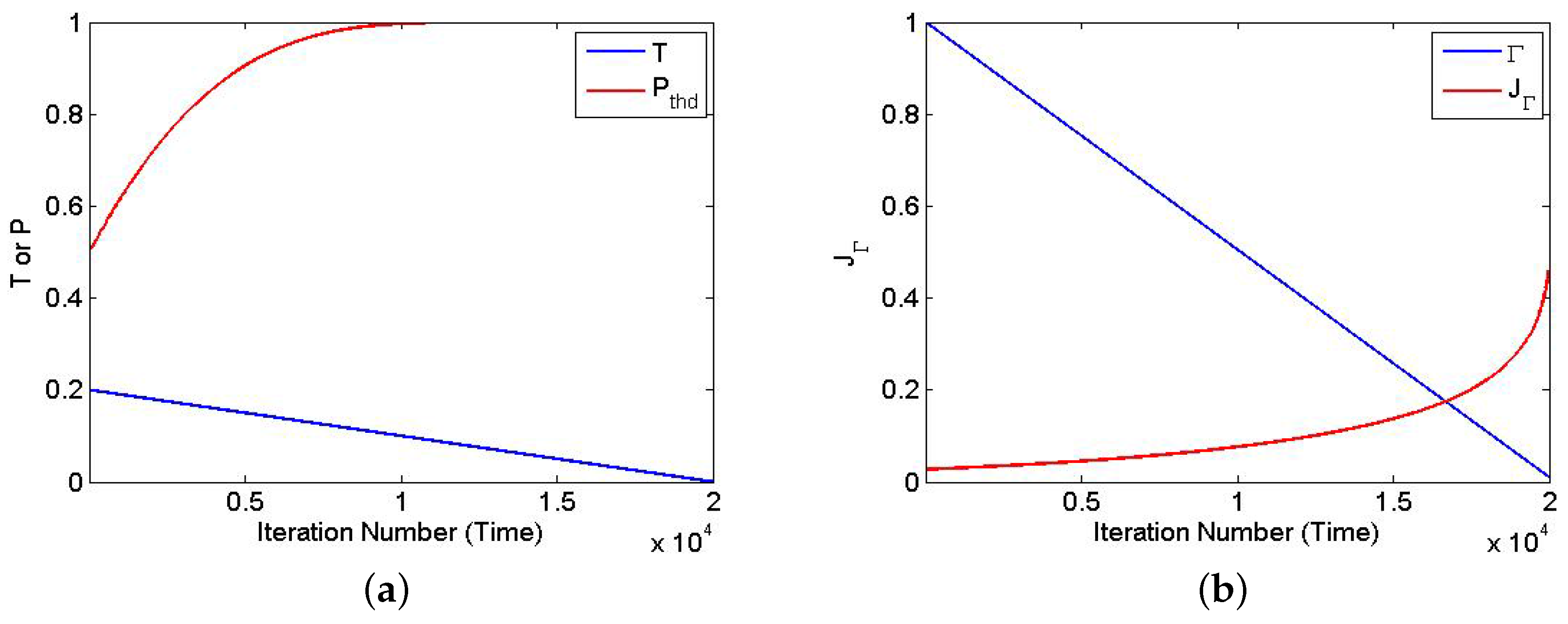
2.1. Simulated Quantum Annealing
2.2. SQA Formulation of the SH Spin Vector
| Algorithm 1 Finding an H-Matrix via Simulated Quantum Annealing |
|
3. Numerical Experiments and Analysis
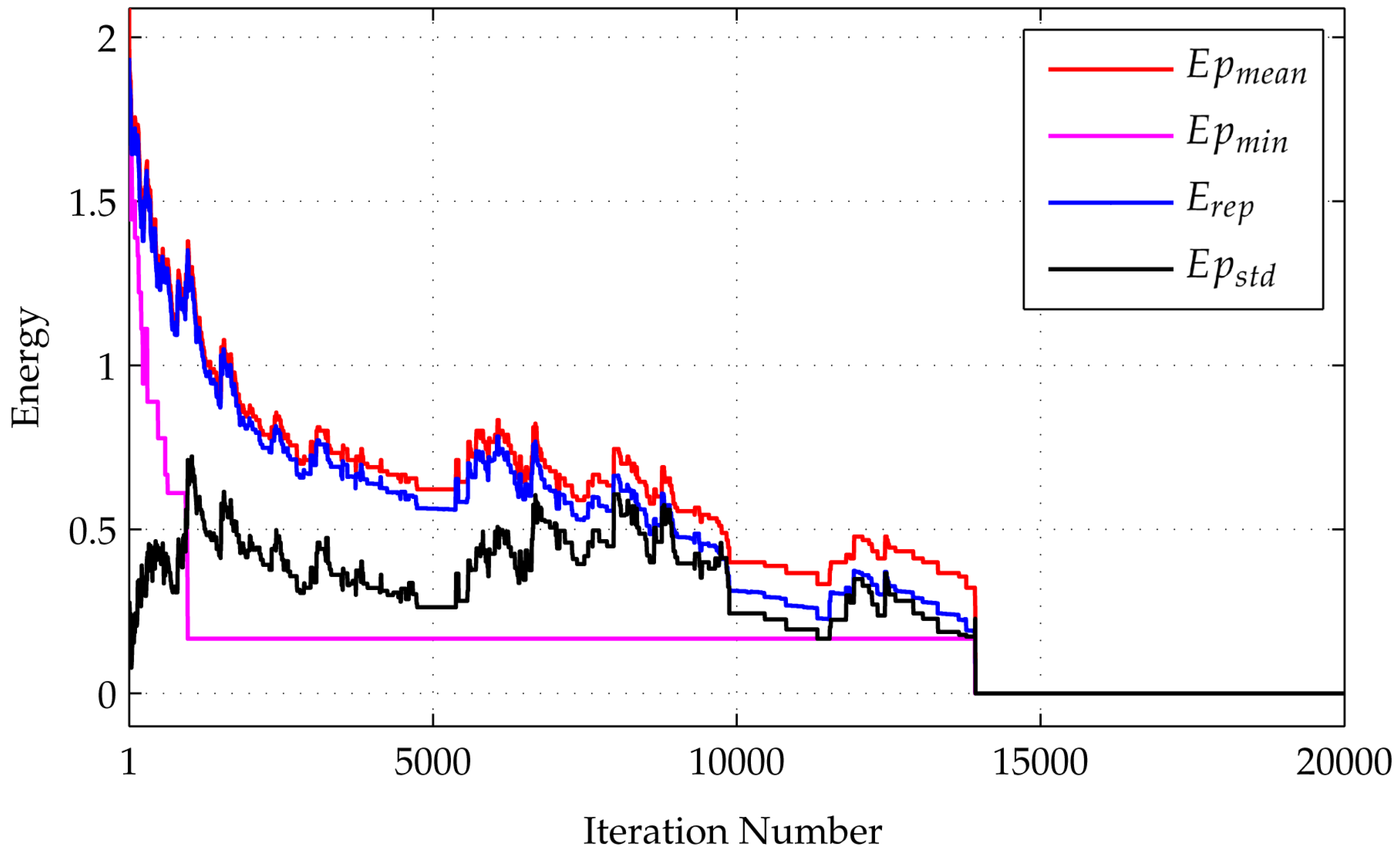
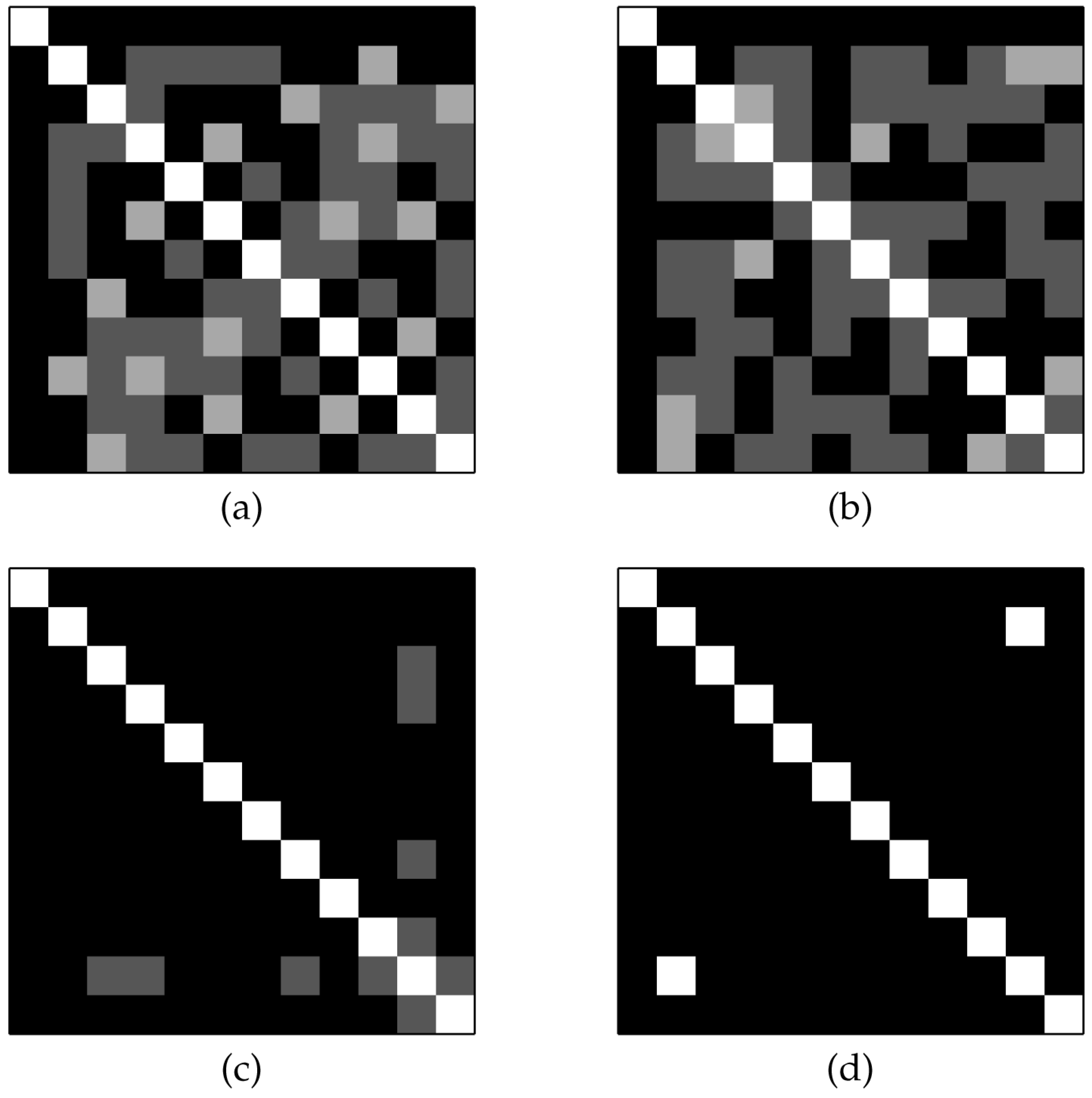
3.1. Finding a 12-Order SH-Matrix Using SQA
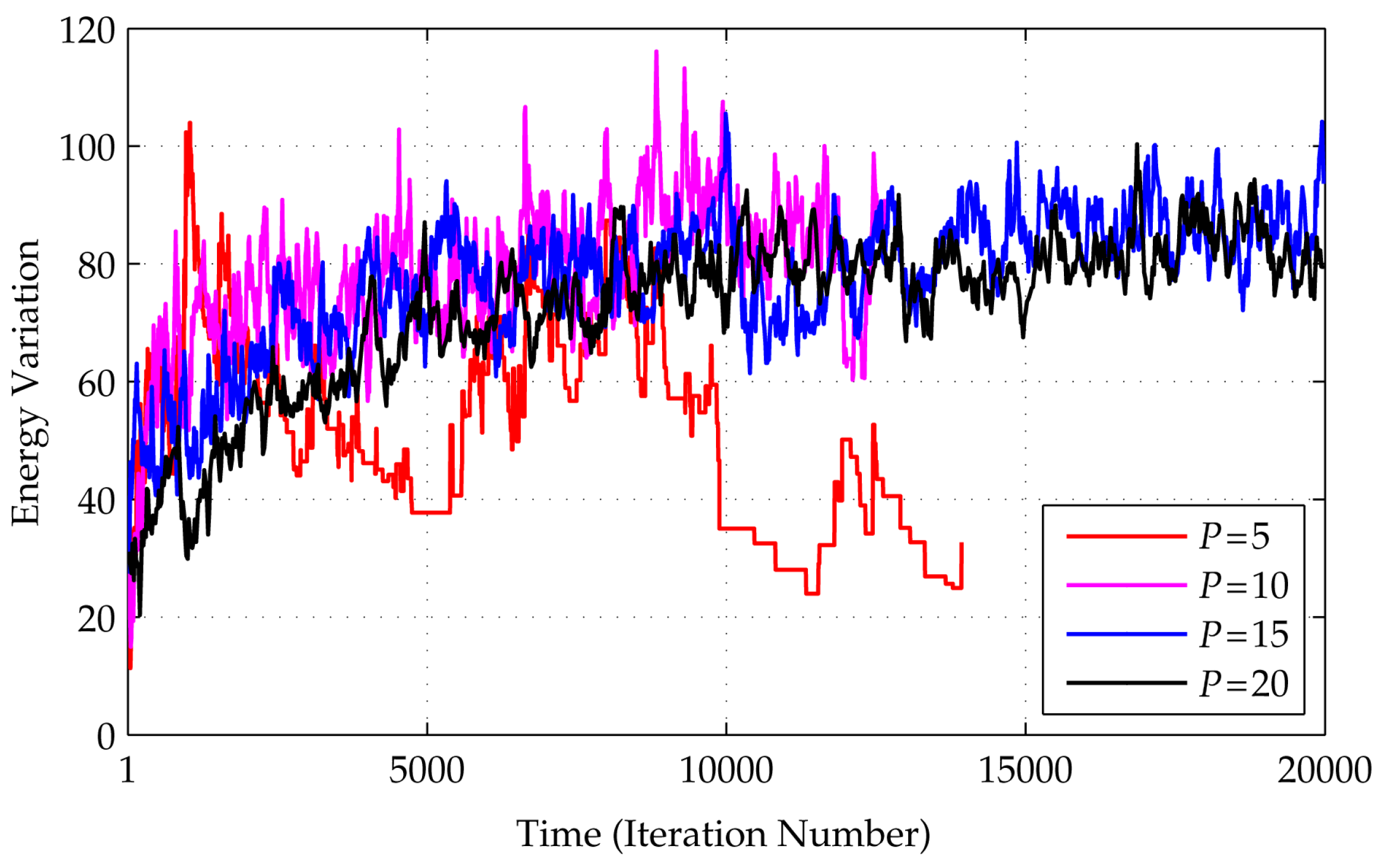
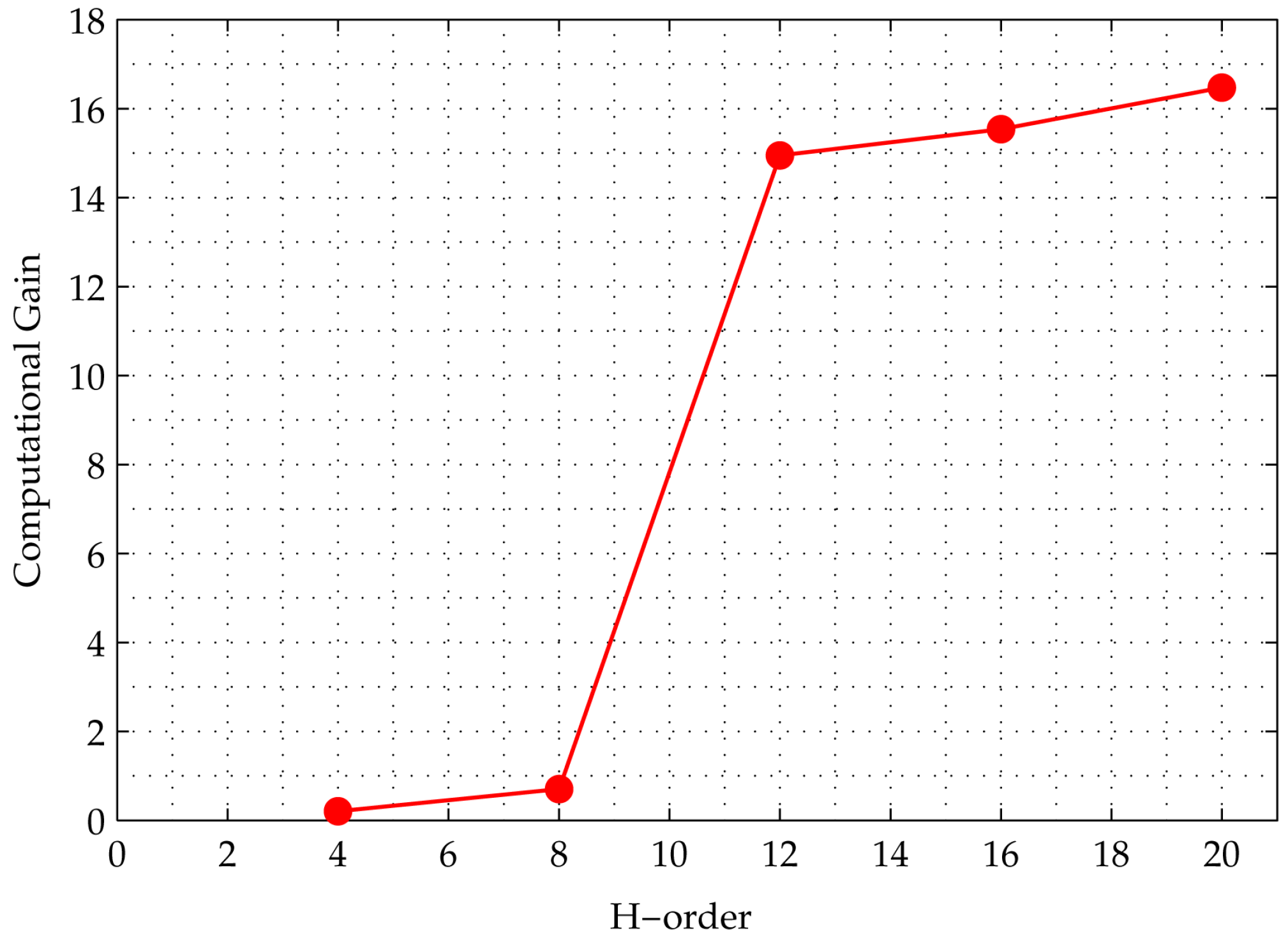
3.2. The Number of The Replicas and Convergence Issue
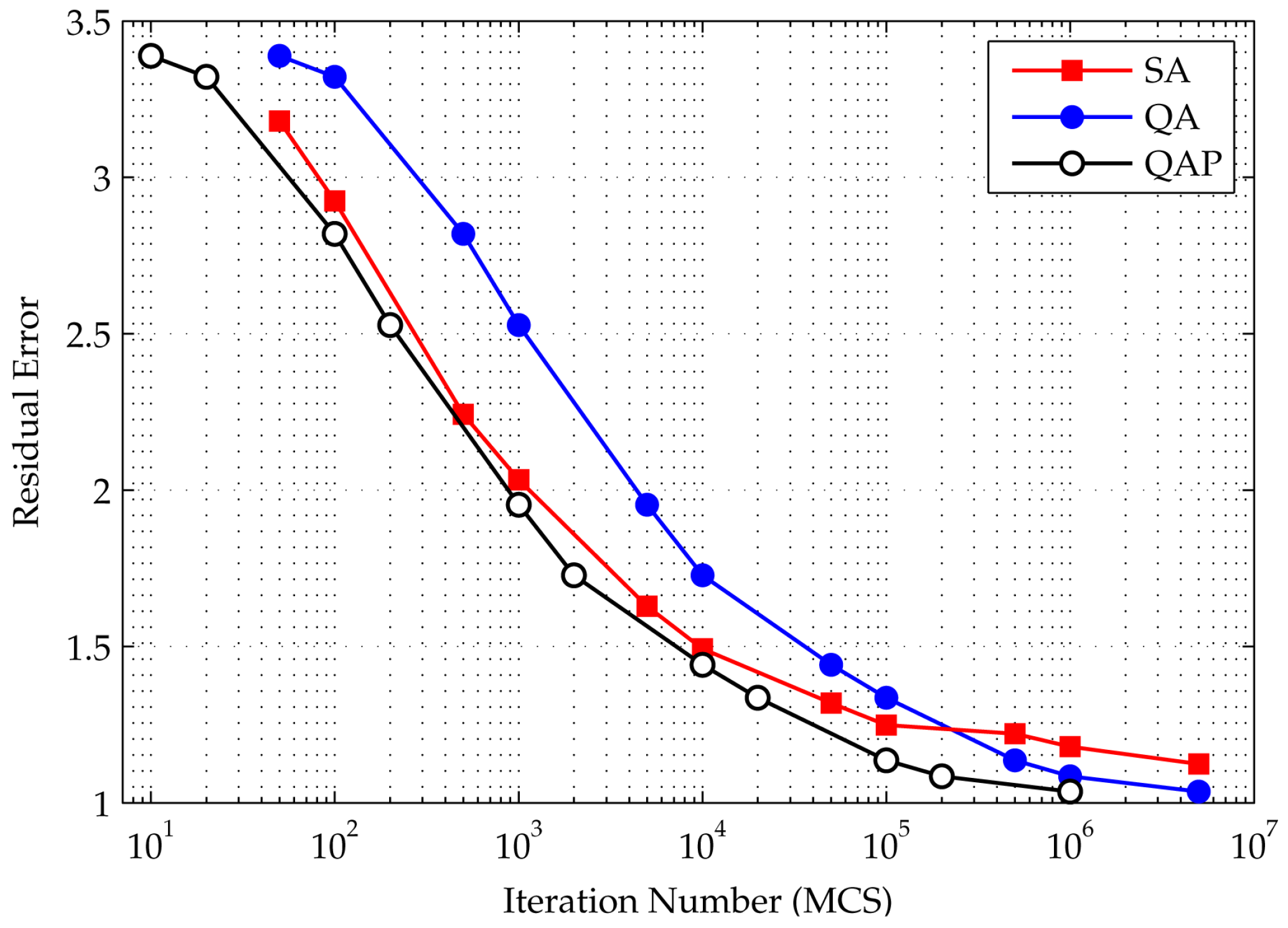
3.3. Performance Comparison: SQA vs. SA
4. Conclusions
Acknowledgments
Conflicts of Interest
References
- Lucas, A. Ising formulations of many NP problem. Front. Phys. 2014, 2, 5. [Google Scholar] [CrossRef]
- Metropolis, N.; Rosenbluth, A.W.; Rosenbluth, M.N.; Teller, A.H. Equation of state calculations by fast computing machines. J. Chem. Phys. 1953, 21, 1087. [Google Scholar] [CrossRef]
- Kirkpatrick, S.; Gelatt, C.D., Jr.; Vecchi, M.P. Optimization by simulated annealing. Science 1983, 220, 671–680. [Google Scholar] [CrossRef] [PubMed]
- Cerny, V. Thermodynamical approach to the traveling salesman problem: An efficient simulation algorithm. J. Optim. Theory Appl. 1985, 45, 41–51. [Google Scholar] [CrossRef]
- Kadowaki, T.; Nishimori, H. Quantum annealing in the transverse Ising model. Phys. Rev. E 1988, 58, 5355. [Google Scholar] [CrossRef]
- Santoro, G.E.; Martonak, R.; Tosatti, E.; Car, E. Theory of quantum annealing of an Ising spin glass. Science 2002, 295, 2427–2730. [Google Scholar] [CrossRef] [PubMed]
- Boixo, S.; Rønnow, T.F.; Isakov, S.V.; Wang, Z.; Wecker, D.; Lidar, D.A.; Martinis, J.M.; Troyer, M. Evidence for quantum annealing with more than one hundred qubits. Nat. Phys. 2014, 10, 218–224. [Google Scholar] [CrossRef]
- Heim, B.; Rønnow, T.F.; Isakov, S.V.; Troyer, M. Quantum versus classical annealing of Ising spin glasses. Science 2015, 348, 215–217. [Google Scholar] [CrossRef] [PubMed]
- Rønnow, T.F.; Wang, Z.; Job, J.; Boixo, S.; Isakov, S.V.; Wecker, D.; Martinis, J.M.; Lidar, D.A.; Troyer, M. Defining and detecting quantum speedup. Science 2014, 345, 420–424. [Google Scholar] [CrossRef] [PubMed]
- Isakov, S.V.; Mazzola, G.; Smelyanskiy, V.N.; Jiang, Z.; Boixo, S.; Neven, H.; Troyer, M. Understanding Quantum Tunneling through Quantum Monte Carlo Simulation. Phys. Rev. Lett. 2016, 117, 180402. [Google Scholar] [CrossRef] [PubMed]
- Mazzola, G.; Smelyanskiy, V.N.; Troyer, M. Quantum Monte Carlo Tunneling from quantum chemistry to quantum annealing. Phys. Rev. B 2017, 96, 134305. [Google Scholar] [CrossRef]
- Martonak, R.; Santoro, G.E.; Tosatti, E. Quantum annealing of the traveling-salesman problem. Phys. Rev. E 2004, 70. [Google Scholar] [CrossRef] [PubMed]
- Titiloye, O.; Crispin, A. Quantum annealing of the graph coloring problem. Discret. Optim. 2011, 8, 376–384. [Google Scholar] [CrossRef]
- Zick, K.M.; Shehab, O.; French, M. Experimental quantum annealing: Case study involving the graph isomorphism problem. Sci. Rep. 2015, 5, 11168. [Google Scholar] [CrossRef] [PubMed]
- Suksmono, A.B. Finding a Hadamard matrix by simulated annealing of spin-vectors. J. Phys. Conf. Ser. 2012, 856, 012012. [Google Scholar] [CrossRef]
- Suzuki, M. Relationship between d-dimensional quantal spin systems and (d+1)-dimensional Ising systems: Equivalence, critical exponents and systematic approximants of the partition function and spin correlations. Prog. Theor. Phys. 1976, 56, 1454–1469. [Google Scholar] [CrossRef]
- Trotter, H.F. On the product of semi-groups of operators. Proc. Am. Math. Soc. 1959, 10, 545–551. [Google Scholar] [CrossRef]
- Sylvester, J.J. Thoughts on inverse orthogonal matrices, simultaneous sign successions, and tessellated pavements in two or more colours, with applications to Newton’s Rule, ornamental tile-work, and the theory of numbers. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1867, 34, 461–475. [Google Scholar] [CrossRef]
- Hadamard, J. Resolution d’une question relative aux determinants. Bull. Sci. Math. 1893, 17, 240–246. [Google Scholar]
- Hedayat, A.; Wallis, W.D. Hadamard Matrices and Their Applications. Ann. Stat. 1978, 6, 1184–1238. [Google Scholar] [CrossRef]
- Horadam, K.J. Hadamard Matrices and Their Applications; Princeton University Press: Princeton, NJ, USA, 2007; ISBN 978-1-40-084290-2. [Google Scholar]
- Garg, V. Wireless Communications and Networking; Morgan-Kaufman: San Francisco, CA, USA, 2007; ISBN 978-0-12-373580-5. [Google Scholar]
- Seberry, J.; Wysocki, B.J.; Wysocki, T.A. On some applications of Hadamard matrices. Metrika 2005, 62, 221–239. [Google Scholar] [CrossRef]
- Paley, R.E.A.C. On Orthogonal Matrices. J. Math. Phys. 1933, 12, 311–320. [Google Scholar] [CrossRef]
- Dade, E.C.; Goldberg, K. The construction of Hadamard matrices. Mich. Math. J. 1959, 6, 247–250. [Google Scholar] [CrossRef]
- Williamson, J. Hadamard’s determinant theorem and the sum of four squares. Duke Math. J. 1944, 11, 65–81. [Google Scholar] [CrossRef]
- Bush, K.A. Unbalanced Hadamard matrices and finite projective planes of even order. J. Comb. Theory Ser. A 1971, 11, 38–44. [Google Scholar] [CrossRef]
- Bush, K.A. Atti del Convegno di Geometria Combinatoria e sue Applicazioni; University Perugia: Perugia, Italy, 1971; Volume 131. [Google Scholar]
- Wallis, J.S. On the existence of Hadamard matrices. J. Comb. Theory A 1976, 21, 188–195. [Google Scholar] [CrossRef]
- Battaglia, D.A.; Santoro, G.E.; Tosatti, E. Optimization by quantum annealing: Lessons from hard satisfiability problems. Phys. Rev. E 2005, 71, 066707. [Google Scholar] [CrossRef] [PubMed]



© 2018 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Suksmono, A.B. Finding a Hadamard Matrix by Simulated Quantum Annealing. Entropy 2018, 20, 141. https://doi.org/10.3390/e20020141
Suksmono AB. Finding a Hadamard Matrix by Simulated Quantum Annealing. Entropy. 2018; 20(2):141. https://doi.org/10.3390/e20020141
Chicago/Turabian StyleSuksmono, Andriyan Bayu. 2018. "Finding a Hadamard Matrix by Simulated Quantum Annealing" Entropy 20, no. 2: 141. https://doi.org/10.3390/e20020141
APA StyleSuksmono, A. B. (2018). Finding a Hadamard Matrix by Simulated Quantum Annealing. Entropy, 20(2), 141. https://doi.org/10.3390/e20020141




