Discrete Transforms and Orthogonal Polynomials of (Anti)symmetric Multivariate Sine Functions
Abstract
1. Introduction
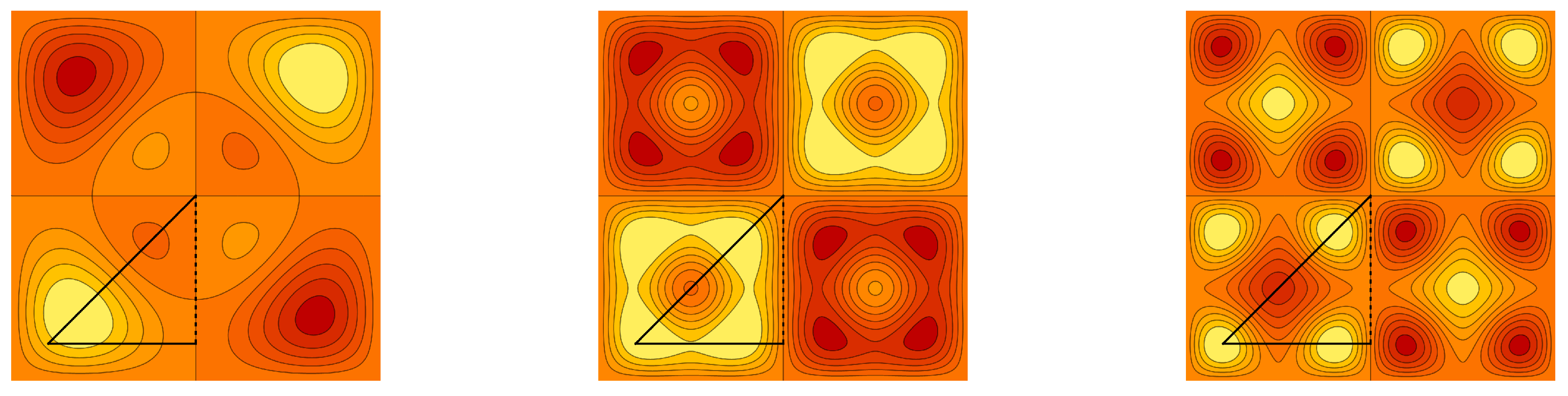
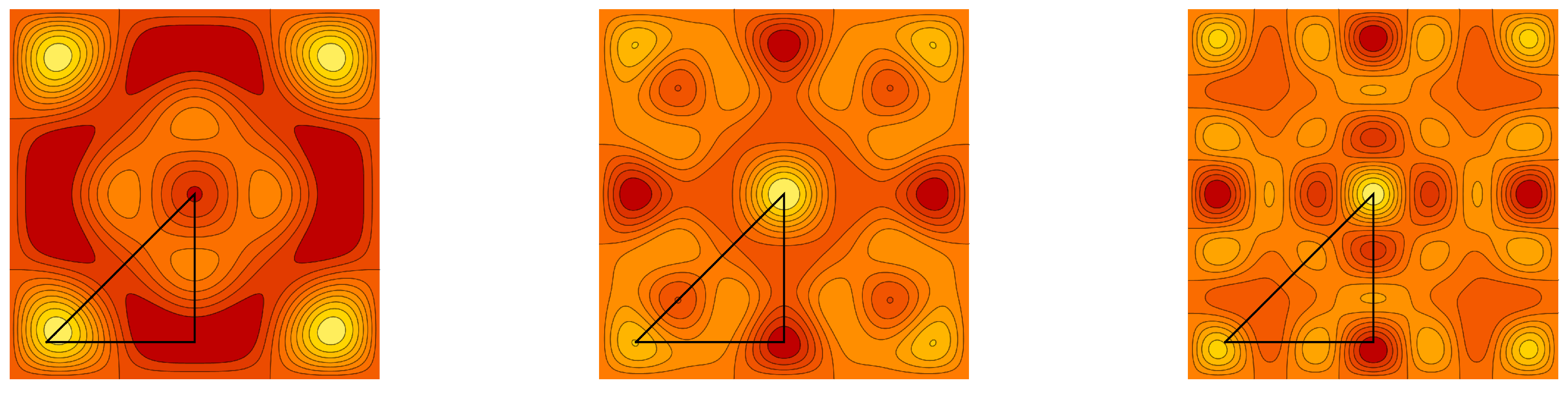
2. Multivariate (Anti)symmetric Sine Functions
2.1. Definitions and Symmetry Properties
2.2. Continuous Orthogonality
3. Discrete Transforms
3.1. Antisymmetric Multivariate Discrete Sine Transforms
3.2. Symmetric Multivariate Discrete Sine Transforms
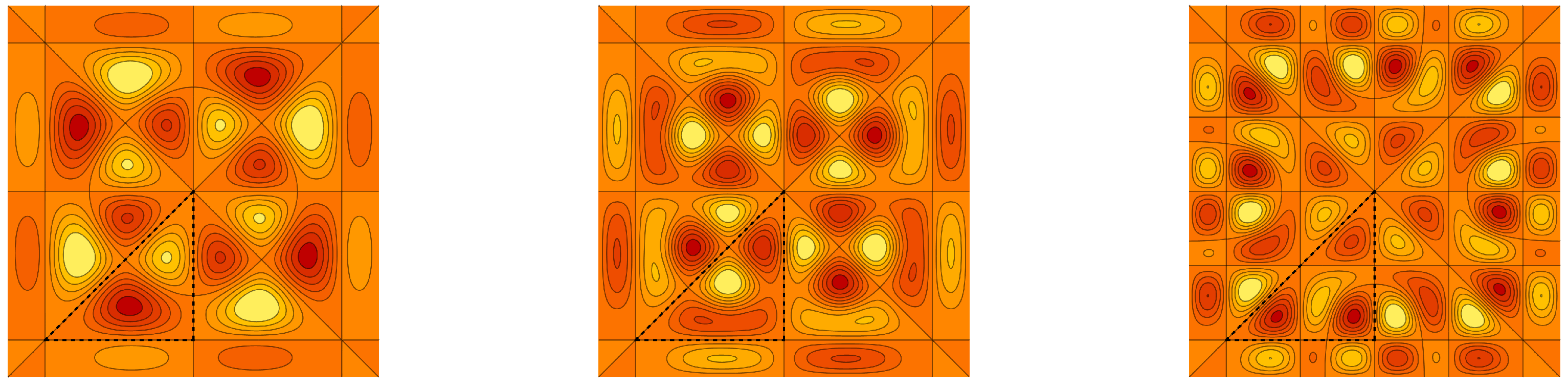
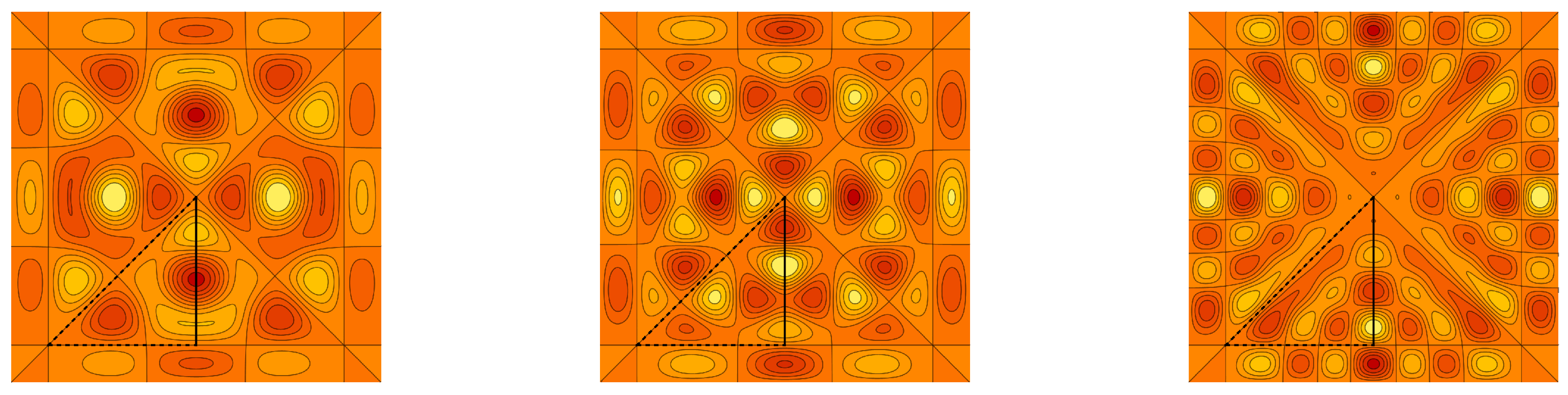
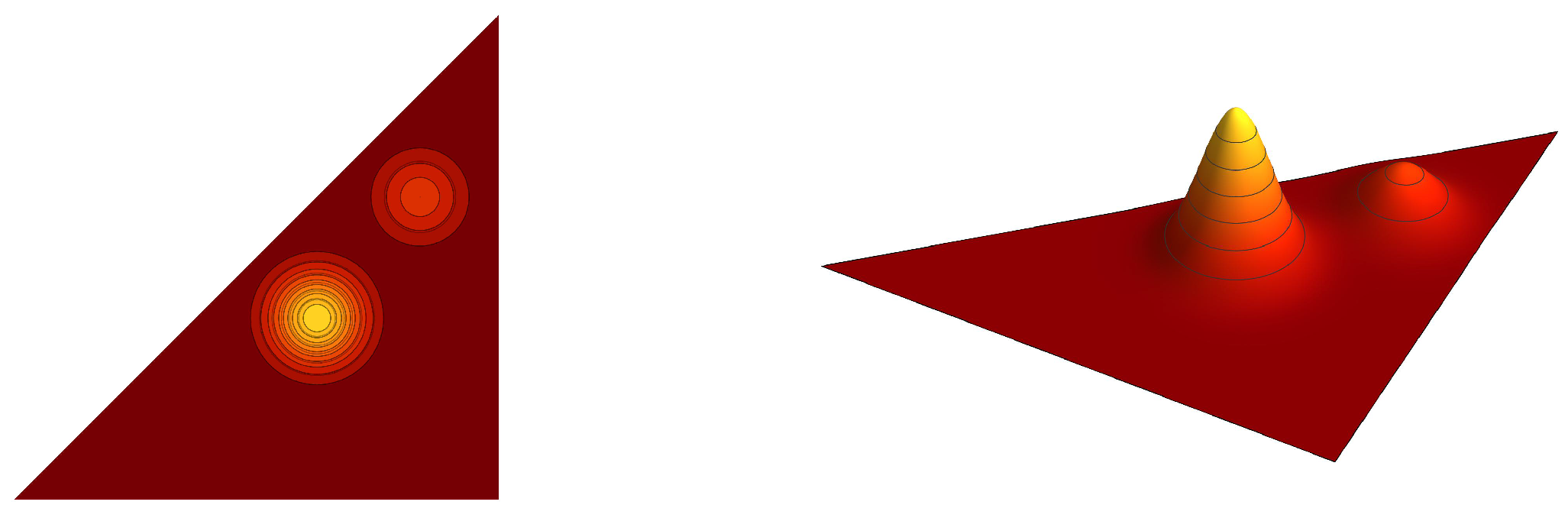
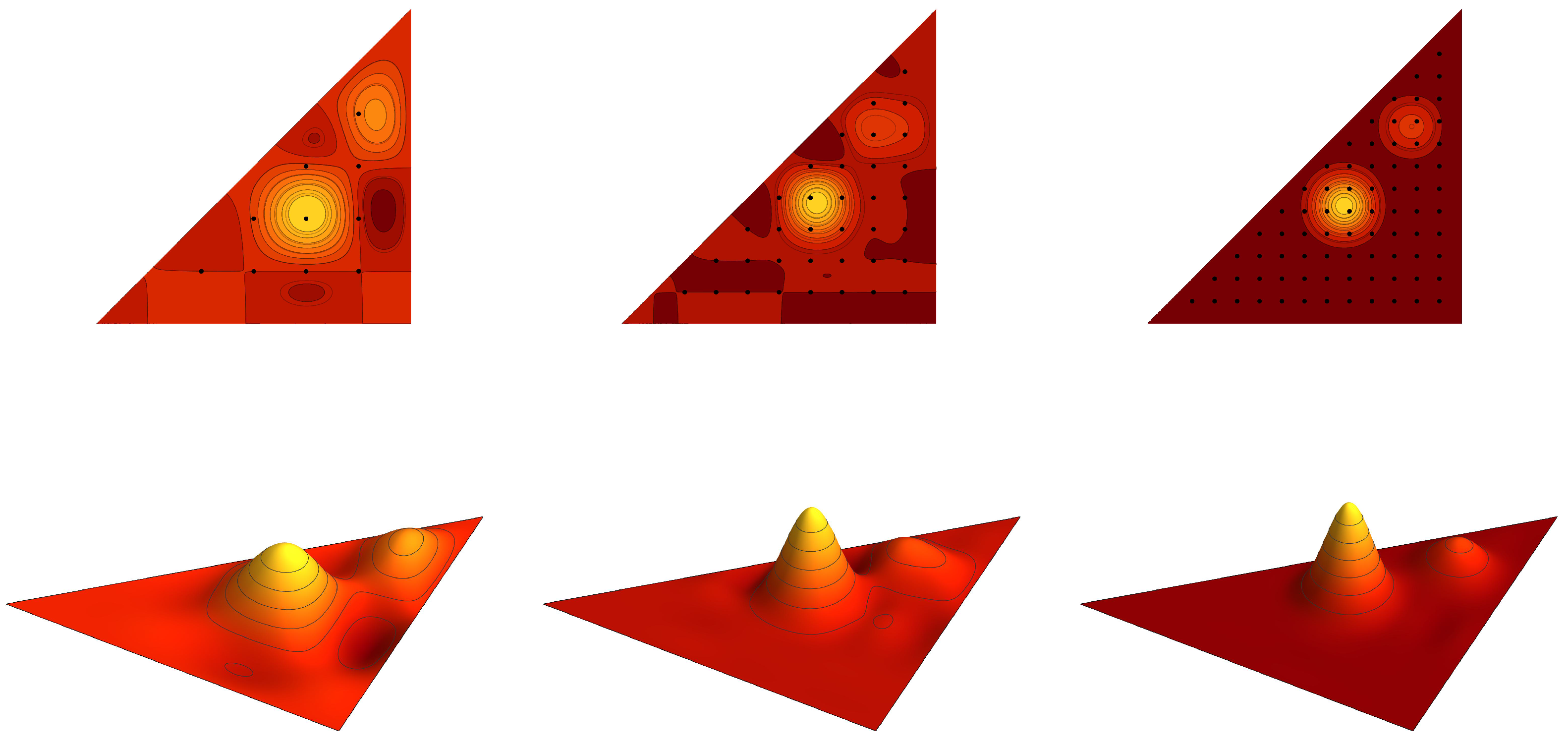
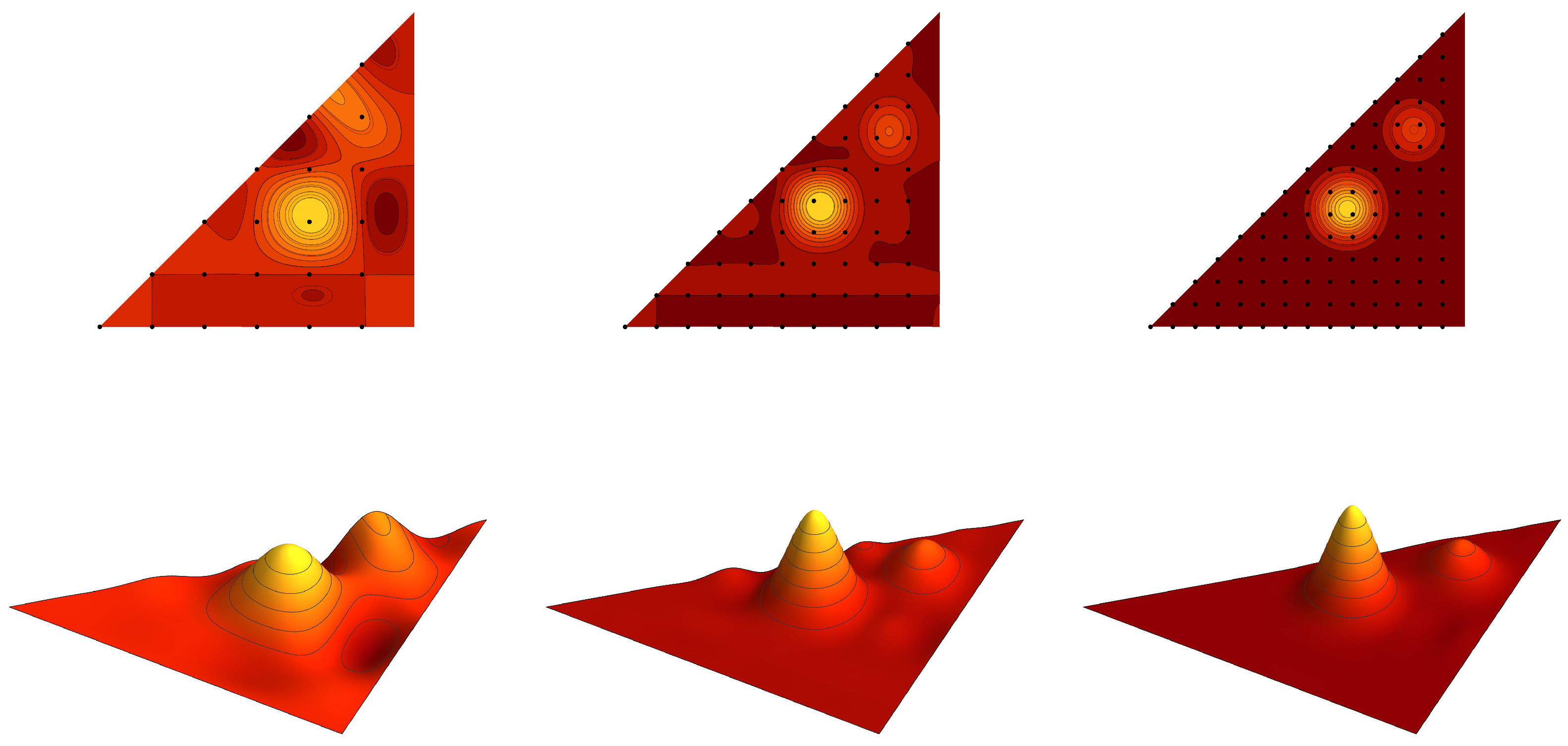
3.3. Interpolation by (Anti)symmetric Sine Functions
3.4. Matrices of the Normalized Discrete Trigonometric Transforms
4. Chebyshev-Like Multivariate Orthogonal Polynomials
4.1. Recurrence Relations
4.2. Continuous Orthogonality
4.3. Cubature Formulas
- 1.
- For , and any polynomial of degree at most , the following formula holds exactly,
- 2.
- For , and any polynomial of degree at most , the following formula holds exactly,
- 3.
- For , and any polynomial of degree at most , the following formulas hold exactly,
- 1.
- For and any polynomial of degree at most , the following formula holds exactly,
- 2.
- For , and any polynomial of degree at most , the following formula holds exactly,
- 3.
- For and any polynomial of degree at most , the following formulas hold exactly,
- 1.
- For , and any polynomial of degree at most , the following formula holds exactly,
- 2.
- For , and any polynomial of degree at most , the following formulas hold exactly,
- 3.
- For , and any polynomial of degree at most , the following formula holds exactly,
- 1.
- For and any polynomial of degree at most , the following formula holds exactly,
- 2.
- For and any polynomial of degree at most , the following formulas hold exactly,
- 3.
- For , and any polynomial of degree at most , the following formulas hold exactly,
4.4. Gaussian Cubature Formulas
- 1.
- the orthogonal polynomials of degree vanish for all nodes ,
- 2.
- the orthogonal polynomials of degree vanish for all nodes ,
- 3.
- the orthogonal polynomials of degree vanish for all nodes ,
- 4.
- the orthogonal polynomials of degree vanish for nodes .
5. Conclusions
- The present fully explicit expression of the cubature rules allows straightforward implementation of the numerical integration and approximation methods. Compared to the abstract variables of the symmetric polynomials (93) from [22], the additional relation (49) established via the fundamental symmetric cosine function connects directly, like in the classical Chebyshev polynomials, the underlying lattice with the generalized Chebyshev nodes. The antisymmetric discrete sine transforms from Table 1 are special cases of the discrete transforms derived in [14] from generalized Schur polynomials associated with Bernstein-Szegö polynomials and parametrized by . On the other hand, the symmetric discrete sine transforms from Table 1 extend the set of discrete transforms connected to the Chebyshev polynomials of the second and fourth kind.
- The symmetry group of the (anti)symmetric sine functions is isomorphic to the Weyl groups of the classical series of the simple Lie algebras and . The correspondence between the (anti)symmetric sine and cosine functions and the four types of the Weyl orbit functions is explicitly developed in [9]. The present point sets of the discrete (anti)symmetric sine transforms and the generalized Chebyshev nodes differ from the weight and dual weight lattice point sets on which the discrete transforms and cubature rules of the Weyl orbit functions are formulated. The topology of the current point sets is, however, similar for some cases to the root lattices of the series and and the explicit formulation of the comparison poses an open problem.
- The Lebesgue constant estimates of the polynomial cubatures and integral error estimates for the interpolation formulas together with criteria for the convergence of the polynomial series deserve further study. The developed cubature formulas as well as the rules from [3,8] reveal that the shifted lattice transforms carry high capacity to produce cubature formulas of Gaussian type. Versions of the Clenshaw–Curtis methods of numerical integration [36], developed for the and root systems in [37,38], also need to be further investigated. The formation of the hyperinterpolation methods [39,40], which straightforwardly employ the standard polynomial cubature rules, poses an open problem for the presented cubature rules.
- The existence and explicit forms of generating functions for the related Weyl group polynomials, developed in [41,42], further increase the relevance of the presented Chebyshev polynomial methods. The generating functions form a powerful tool for investigating symmetries and parity relations of the generated orthogonal polynomials and represent practical tool for efficient computer implementation and handling of the generated polynomials. The recurrence relations algorithms for the calculation of the trivariate polynomials are potentially superseded by explicit evaluation formulas derived from the generating functions. The form of the generating functions and the explicit evaluation formulas for the current polynomials pose open problems.
Author Contributions
Funding
Conflicts of Interest
References
- Klimyk, A.; Patera, J. (Anti)symmetric multivariate trigonometric functions and corresponding Fourier transforms. J. Math. Phys. 2007, 48, 093504. [Google Scholar] [CrossRef]
- Britanak, V.; Rao, K.; Yip, P. Discrete Cosine and Sine Transforms: General Properties, Fast Algorithms And Integer Approximation; Elsevier/Academic Press: Amsterdam, The Netherlands, 2007. [Google Scholar]
- Hrivnák, J.; Motlochová, L. Discrete transforms and orthogonal polynomials of (anti)symmetric multivariate cosine functions. SIAM J. Numer. Anal. 2010, 51, 073509. [Google Scholar] [CrossRef]
- Klimyk, A.; Patera, J. Antisymmetric Orbit Functions. SIGMA 2007, 3, 023. [Google Scholar] [CrossRef]
- Klimyk, A.; Patera, J. Orbit Functions. SIGMA 2006, 2, 006. [Google Scholar] [CrossRef]
- Hrivnák, J.; Motlochová, L.; Patera, J. Two dimensional symmetric and antisymmetric generalization of sine functions. J. Math. Phys. 2010, 51, 073509. [Google Scholar] [CrossRef]
- Hrivnák, J.; Patera, J. Two dimensional symmetric and antisymmetric generalization of exponential and cosine functions. J. Math. Phys. 2010, 51, 023515. [Google Scholar] [CrossRef]
- Moody, R.V.; Motlochová, L.; Patera, J. Gaussian cubature arising from hybrid characters of simple lie groups. J. Fourier Anal. Appl. 2014, 20, 1257–1290. [Google Scholar] [CrossRef]
- Hrivnák, J.; Motlochová, L. On connecting Weyl-orbit functions to Jacobi polynomials and multivariate (anti)symmetric trigonometric functions. Acta Polytech. 2016, 56, 283–290. [Google Scholar] [CrossRef]
- Hrivnák, J.; Patera, J. On discretization of tori compact simple Lie groups. J. Phys. A Math. Theor. 2009, 42, 385208. [Google Scholar] [CrossRef]
- Hrivnák, J.; Patera, J. On E-discretization of tori compact simple Lie groups. J. Phys. A Math. Theor. 2010, 43, 165206. [Google Scholar] [CrossRef]
- Hrivnák, J.; Walton, M.A. Weight-lattice discretization of Weyl-orbit functions. J. Math. Phys. 2016, 57, 083512. [Google Scholar] [CrossRef]
- Moody, R.V.; Patera, J. Orthogonality within Families of C-, S- and E- Functions of Any Compact Semisimple Lie Group. SIGMA 2006, 2, 076. [Google Scholar] [CrossRef]
- van Diejen, J.F.; Emsiz, E. Discrete Fourier transform associated with generalized Schur polynomials. Proc. Am. Math. Soc. 2018, 146, 3459–3472. [Google Scholar] [CrossRef]
- Handscomb, D.C.; Mason, J.C. Chebyshev Polynomials; Champman & Hall/CRC: New York, NY, USA, 2002. [Google Scholar]
- Rivlin, T.J. The Chebyshev Polynomials; John Wiley & Sons: New York, NY, USA, 1990. [Google Scholar]
- Koornwinder, T.H. Two-variable analogues of the classical orthogonal polynomials. Theory Appl. Spec. Funct. 1975, 435–495. [Google Scholar] [CrossRef]
- Cools, R. An encyklopedia of cubature formulas. J. Complex. 2003, 19, 445–453. [Google Scholar] [CrossRef]
- Dunkl, C.F.; Xu, Y. Orthogonal Polynomials of Several Variables; Cambridge University Press: Cambridge, UK, 2001. [Google Scholar]
- Li, H.; Sun, J.; Xu, Y. Discrete Fourirer analysis and Chebyshev polynomials with G2 group. SIGMA 2012, 8, 67. [Google Scholar]
- Li, H.; Sun, J.; Xu, Y. Discrete Fourirer analysis cubature and interpolation on hexagon and triangle. SIAM J. Numer. Anal. 2008, 46, 1653–1681. [Google Scholar] [CrossRef]
- Berens, H.; Schmid, H.J.; Xu, Y. Multivariate Gaussian cubature formulae. Arch. Math. 1995, 64, 26–32. [Google Scholar] [CrossRef]
- Drissi, L.B.; Saidi, E.H.; Bousmina, M. Graphene, Lattice Field Theory and Symmetries. J. Math. Phys. 2011, 52, 022306. [Google Scholar] [CrossRef]
- Hrivnák, J.; Motlochová, L.; Patera, J. Cubature formulas of multivariate polynomials arising from symmetric orbit functions. Symmetry 2016, 8, 63. [Google Scholar] [CrossRef]
- Chadzitaskos, G.; Háková, L.; Kájínek, O. Weyl Group Orbit Functions in Image Processing. Appl. Math. 2014, 5, 501–511. [Google Scholar] [CrossRef]
- Çapoğlu, I.R.; Taflove, A.; Backman, V. Computation of tightly-focused laser beams in the FDTD method. Opt. Express 2013, 21, 87–101. [Google Scholar] [CrossRef] [PubMed]
- Xu, J.; Chen, J.; Li, J. Probability density evolution analysis of engineering structures via cubature points. Comput. Mech. 2012, 50, 135–156. [Google Scholar] [CrossRef]
- Lauvergnata, D.; Nauts, A. Quantum dynamics with sparse grids: A combination of Smolyak scheme and cubature. Application to methanol in full dimensionality. Spectrochim. Acta Part A 2014, 119, 18–25. [Google Scholar] [CrossRef] [PubMed]
- Crivellini, A.; D’Alessandro, V.; Bassi, F. High-order discontinuous Galerkin solutions of three-dimensional incompressible RANS equations. Comput. Fluids 2013, 81, 122–133. [Google Scholar] [CrossRef]
- Young, J.C.; Gedney, S.D.; Adams, R.J. Quasi-Mixed-Order Prism Basis Functions for Nyström-Based Volume Integral Equations. IEEE Trans. Magn. 2012, 48, 2560–2566. [Google Scholar] [CrossRef]
- Sfevanovic, I.; Merli, F.; Crespo-Valero, P.; Simon, W.S.; Holzwarth, M.; Mattes, J.R. Mosig, Integral Equation Modeling of Waveguide-Fed Planar Antennas. IEEE Antennas Propag. Mag. 2009, 51, 82–92. [Google Scholar] [CrossRef]
- Chernyshenko, D.; Fangohr, H. Computing the demagnetizing tensor for finite difference micromagnetic simulations via numerical integration. J. Magn. Magn. Mater. 2015, 381, 440–445. [Google Scholar] [CrossRef]
- Tasinkevych, M.; Silvestre, N.M.; de Gama, M.M.T. Liquid crystal boojum-colloids. New J. Phys. 2012, 14, 073030. [Google Scholar] [CrossRef]
- Marin, M. Weak Solutions in Elasticity of Dipolar Porous Materials. Math. Probl. Eng. 2008, 2008, 158908. [Google Scholar] [CrossRef]
- Marin, M.; Florea, O. On temporal behaviour of solutions in thermoelasticity of porous micropolar bodies. An. St. Univ. Ovidius Constanta, Ser. Mat. 2014, 22, 169–188. [Google Scholar] [CrossRef]
- Clenshaw, C.W.; Curtis, A.R. A method for numerical integration on an automatic computer. Numer. Math. 1960, 2, 197–205. [Google Scholar] [CrossRef]
- Háková, L.; Hrivnák, J.; Motlochová, L. On cubature rules associated to Weyl group orbit functions. Acta Polytech. 2016, 56, 202–213. [Google Scholar] [CrossRef]
- Munthe-Kaas, H.; Ryland, B.N. On multivariate Chebyshev polynomials and spectral approximations on triangles. In Spectral and High Order Methods for Partial Differential Equations; Lecture Notes in Computational Science and Engineering; Springer: Berlin/Heidelberg, Germany, 2010; Volume 76, pp. 19–41. [Google Scholar]
- Sommariva, A.; Vianello, M.; Zanovello, R. Nontensorial Clenshaw-Curtis cubature. Numer. Algorithms 2008, 49, 409–427. [Google Scholar] [CrossRef]
- Caliari, M.; de Marchi, S.; Vianello, M. Hyperinterpolation in the cube. Comput. Math. Appl. 2008, 55, 2490–2497. [Google Scholar] [CrossRef]
- Czyżycki, T.; Hrivnák, J.; Patera, J. Generating functions for orthogonal polynomials of A2, C2 and G2. Symmetry 2018, 10, 354. [Google Scholar] [CrossRef]
- Damaskinsky, E.V.; Kulish, P.P.; Sokolov, M.A. On calculation of generating functions of Chebyshev polynomials in several variables. J. Math. Phys. 2015, 56, 063507. [Google Scholar] [CrossRef]
| ★ | ||||||
|---|---|---|---|---|---|---|
| I | 1 | |||||
| II | 1 | |||||
| III | ||||||
| IV | 1 | |||||
| V | 1 | |||||
| VI | 1 | |||||
| VII | 1 | |||||
| VIII |
| N | ||||
| 7 | ||||
| 12 | ||||
| 17 | ||||
| 22 | ||||
| 27 | ||||
| 7 | ||||
| 12 | ||||
| 17 | ||||
| 22 | ||||
| 27 |
| 1 | 1 | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 1 | ||||||||||||
| 2 | 2 | 0 | |||||||||||
| 1 | |||||||||||||
| 1 | 0 | 0 | |||||||||||
| 6 | 10 | 4 | 1 | ||||||||||
| 2 | 1 |
| 1 | |||||||||
| 1 | 0 | ||||||||
| 0 | 2 | ||||||||
| 1 | 4 | 0 |
| 1 | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 1 | ||||||||||
| 1 | 1 | |||||||||
| 3 | 1 | 2 | ||||||||
| 3 | 2 | 2 | ||||||||
| 1 | 0 | 1 | ||||||||
| 1 | 0 | 1 | 2 | |||||||
| 2 | 0 | 2 | 2 | |||||||
| 8 | 0 | 12 | 2 | 4 | ||||||
| 6 | 2 | 10 | 4 | 2 | 0 | 4 | ||||
| 2 | 3 | 0 |
| 1 | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 1 | ||||||||||
| 1 | ||||||||||
| 1 | ||||||||||
| 1 | 1 | 2 | ||||||||
| 0 | ||||||||||
| 0 | ||||||||||
| 0 | ||||||||||
| 1 | ||||||||||
| 1 | 2 | 0 | 0 | |||||||
| 1 | 1 | 4 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Brus, A.; Hrivnák, J.; Motlochová, L. Discrete Transforms and Orthogonal Polynomials of (Anti)symmetric Multivariate Sine Functions. Entropy 2018, 20, 938. https://doi.org/10.3390/e20120938
Brus A, Hrivnák J, Motlochová L. Discrete Transforms and Orthogonal Polynomials of (Anti)symmetric Multivariate Sine Functions. Entropy. 2018; 20(12):938. https://doi.org/10.3390/e20120938
Chicago/Turabian StyleBrus, Adam, Jiří Hrivnák, and Lenka Motlochová. 2018. "Discrete Transforms and Orthogonal Polynomials of (Anti)symmetric Multivariate Sine Functions" Entropy 20, no. 12: 938. https://doi.org/10.3390/e20120938
APA StyleBrus, A., Hrivnák, J., & Motlochová, L. (2018). Discrete Transforms and Orthogonal Polynomials of (Anti)symmetric Multivariate Sine Functions. Entropy, 20(12), 938. https://doi.org/10.3390/e20120938





