Second Law Analysis for Couple Stress Fluid Flow through a Porous Medium with Constant Heat Flux
Abstract
:1. Introduction
2. Model Formulation
3. Adomian Method of Solution
4. Entropy Generation Analysis
5. Discussion
6. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Nomenclature
| dimensionless lower and upper slip coefficients | |
| dimensional lower and upper slip parameters | |
| couple stress inverse parameter | |
| dimensionless fluid temperature | |
| fluid density | |
| dynamic viscosity | |
| porous permeability parameter | |
| couple stress coefficient | |
| dimensional and dimensionless fluid velocity | |
| modified Brinkman number | |
| maximum velocity | |
| Cartesian coordinates | |
| dimensionless pressure gradient | |
| characteristic velocity | |
| channel width | |
| fluid pressure | |
| specific heat capacity | |
| dimensional and referenced fluid temperatures | |
| constant heat flux | |
| porous permeability |
References
- Cimpean, D.; Pop, I.; Ingham, D.B.; Merkin, J.H. Fully Developed Mixed Convection Flow between Inclined Parallel Plates Filled with a Porous Medium. Transp. Porous Med. 2009, 77, 87–102. [Google Scholar] [CrossRef]
- Hajipour, M.; Dehkordi, A.M. Analysis of nanofluid heat transfer in parallel-plate vertical channels partially filled with porous medium. Int. J. Therm. Sci. 2012, 55, 103–113. [Google Scholar] [CrossRef]
- Mahmoudi, Y. Constant wall heat flux boundary condition in microchannels filled with a porous medium with internal heat generation under local thermal non-equilibrium condition. Int. J. Heat Mass Transf. 2015, 85, 524–542. [Google Scholar] [CrossRef]
- Mahdavi, M.; Saffar-Avval, M.; Tiari, S.; Mansoori, Z. Entropy generation and heat transfer numerical analysis in pipes partially filled with porous medium. Int. J. Heat Mass Transf. 2014, 79, 496–506. [Google Scholar] [CrossRef]
- Cimpean, D.S.; Pop, I. Fully developed mixed convection flow of a nanofluid through an inclined channel filled with a porous medium. Int. J. Heat Mass Transf. 2012, 55, 907–914. [Google Scholar] [CrossRef]
- Torabi, M.; Zhang, K.; Yang, G.; Wang, J.; Wu, P. Heat transfer and entropy generation analyses in a channel partially filled with porous media using local thermal nonequilibrium model. Energy 2015, 82, 922–938. [Google Scholar] [CrossRef]
- Neild, D.A.; Bejan, A. Convection in Porous Media, 4th ed.; Springer: New York, NY, USA, 2013. [Google Scholar]
- Bagchi, A.; Kulacki, F.A. Natural Convection in Superposed Fluid-Porous Layers; Springer: New York, NY, USA, 2014. [Google Scholar]
- Pop, I.; Ingham, D.B. Convective Heat Transfer: Mathematical and Computational Modelling of Viscous Fluids and Porous Media; Pergamon Press: Oxford, UK, 2001. [Google Scholar]
- Vadasz, P. Emerging Topics in Heat and Mass Transfer in Porous Media; Springer: Berlin, Germany, 2008; Volume 22. [Google Scholar]
- Vafai, K. Porous Media: Applications in Biological Systems and Biotechnology; CRC Press: Tokyo, Japan, 2010. [Google Scholar]
- Sahin, A.Z. Entropy generation and pumping power in a turbulent fluid flow through a smooth pipe subjected to constant heat flux. Exergy Int. J. 2002, 2, 314–321. [Google Scholar] [CrossRef]
- Stokes, V.K. Couple stresses in fluid. Phys. Fluids 1966, 9, 1709–1715. [Google Scholar] [CrossRef]
- Srinivasacharya, D.; Kaladhar, K. Analytical solution for Hall and Ion-slip effects on mixed convection flow of couple stress fluid between parallel disks. Math. Comput. Model. 2013, 57, 2494–2509. [Google Scholar]
- Ahmed, S.; Bég, O.A.; Ghosh, S.K. A couple stress fluid modelling on free convection oscillatory hydromagnetic flow in an inclined rotating channel. Ain Shams Eng. J. 2014, 5, 1249–1265. [Google Scholar] [CrossRef]
- Akhtar, S.; Shah, N.A. Exact solutions for some unsteady flows of a couple stress fluid between parallel plates. Ain Shams Eng. J. 2016. [Google Scholar] [CrossRef]
- Aksoy, Y. Effects of couple stresses on the heat transfer and entropy generation rates for a flow between parallel plates with constant heat flux. Int. J. Therm. Sci. 2016, 107, 1–12. [Google Scholar] [CrossRef]
- Srinivasacharya, D.; RamReddy, C. Soret and Dufour effects on mixed convection in a non-Darcy porous medium saturated with micropolar fluid. Nonlinear Anal. Model. Control 2011, 16, 100–115. [Google Scholar]
- Srinivasacharya, D.; Kaladhar, K. Mixed convection flow of couple stress fluid between parallel vertical plates with Hall and Ion-slip effects. Commun. Nonlinear Sci. Numer. Simul. 2012, 17, 2447–2462. [Google Scholar] [CrossRef]
- Srinivasacharya, D.; Rao, G.M. Computational analysis of magnetic effects on pulsatile flow of couple stress fluid through a bifurcated artery. Comput. Methods Programs Biomed. 2016, 137, 269–279. [Google Scholar] [CrossRef] [PubMed]
- Srinivasacharya, D.; Srinivasacharyulu, N.; Odelu, O. Flow and heat transfer of couple stress fluid in a porous channel with expanding and contracting walls. Int. Commun. Heat Mass Transf. 2009, 36, 180–185. [Google Scholar] [CrossRef]
- Srinivasacharya, D.; Srikanth, D. Effect of couple stresses on the pulsatile flow through a constricted annulus. C. R. Mec. 2008, 336, 820–827. [Google Scholar] [CrossRef]
- Bég, O.A.; Ghosh, S.K.; Ahmed, S.; Bég, T. Mathematical modelling of oscillatory magneto-convection of a couple-stress biofluid in an inclined rotating channel. J. Mech. Med. Biol. 2012, 12, 1250050. [Google Scholar] [CrossRef]
- Zueco, J.; Bég, O.A. Network numerical simulation applied to pulsatile non-Newtonian flow through a channel with couple stress and wall mass flux effects. Int. J. Appl. Math. Mech. 2009, 5, 1–16. [Google Scholar]
- Hayat, T.; Awais, M.; Safdar, A.; Hendi, A.A. Unsteady three-dimensional flow of couple stress fluid over a stretching surface with chemical reaction. Nonlinear Anal. Model. Control. 2012, 17, 47–59. [Google Scholar]
- Hina, S.; Mustafa, M.; Hayat, T. On the exact solution for peristaltic flow of couple-stress fluid with wall properties. Bulg. Chem. Commun. 2015, 47, 30–37. [Google Scholar]
- Ting, T.W.; Hung, Y.M.; Guo, N. Entropy generation of viscous dissipative nanofluid convection in asymmetrically heated porous micro channels with solid-phase heat generation. Energy Convers. Manag. 2015, 105, 731–745. [Google Scholar] [CrossRef]
- Anand, V. Entropy generation analysis of laminar flow of a nanofluid in a circular tube immersed in an isothermal external fluid. Energy 2015, 93, 154–164. [Google Scholar] [CrossRef]
- Chen, B.S.; Liu, C.C. Entropy generation in mixed convection magnetohydrodynamic nanofluid flow in vertical channel. Int. J. Heat Mass Transf. 2015, 91, 1026–1033. [Google Scholar] [CrossRef]
- López, A.; Ibáñez, G.; Pantoja, J.; Moreira, J.; Lastres, O. Entropy generation analysis of MHD nanofluid flow in a porous vertical microchannel with nonlinear thermal radiation, slip flow and convective-radiative boundary conditions. Int. J. Heat Mass Transf. 2017, 107, 982–994. [Google Scholar] [CrossRef]
- Havzali, M.; Arikoglu, A.; Komurgoz, G.; Keser, H.I.; Ozkol, I. Analytical–Numerical analysis of entropy generation for gravity-driven inclined channel flow with initial transition and entrance effects. Phys. Scr. 2008, 78, 045401. [Google Scholar] [CrossRef]
- Das, S.; Jana, R.N. Entropy generation due to MHD flow in a porous channel with Navier slip. Ain Shams Eng. J. 2014, 5, 575–584. [Google Scholar] [CrossRef]
- Adesanya, S.O. Second Law Analysis for Third-Grade Fluid with Variable Properties. J. Thermodyn. 2014, 2014, 452168. [Google Scholar] [CrossRef]
- Adesanya, S.O.; Kareem, S.O.; Falade, A.J.; Arekete, S.A. Entropy generation analysis for a reactive couple stress fluid flow through a channel saturated with porous material. Energy 2015, 93, 1239–1245. [Google Scholar] [CrossRef]
- Sheremet, M.A.; Oztop, H.F.; Pop, I.; Abu-Hamdeh, N. Analysis of entropy generation in natural convection of nanofluid inside a square cavity having hot solid block: Tiwari and Das’ model. Entropy 2016, 18, 9. [Google Scholar] [CrossRef]
- Bondareva, N.S.; Sheremet, M.A.; Oztop, H.F.; Abu-Hamdeh, N. Entropy generation due to natural convection of a nanofluid in a partially open triangular cavity. Adv. Powder Technol. 2017, 28, 244–255. [Google Scholar] [CrossRef]
- Fakoya, M.B.; van der Poll, H.M. Integrating ERP and MFCA systems for improved waste-reduction decisions in a brewery in South Africa. J. Clean. Prod. 2013, 40, 136–140. [Google Scholar] [CrossRef]
- Fakoya, M.B.; van der Poll, B. The feasibility of applying material flow cost accounting as an integrative approach to brewery waste-reduction decisions. Afr. J. Bus. Manag. 2012, 6, 9783. [Google Scholar]
- Fakoya, M.B. Adopting material flow cost accounting model for improved waste-reduction decisions in a micro-brewery. Environ. Dev. Sustain. 2015, 17, 1017–1030. [Google Scholar] [CrossRef]
- Hayat, T.; Hina, S.; Ali, N. Simultaneous effects of slip and heat transfer on the peristaltic flow. Commun. Nonlinear Sci. Numer. Simul. 2010, 15, 1526–1537. [Google Scholar] [CrossRef]
- Hayat, T.; Farooq, M.A.; Javed, T.; Sajid, M. Partial slip effects on the flow and heat transfer characteristics in a third-grade fluid. Nonlinear Anal. Real World Appl. 2009, 10, 745–755. [Google Scholar] [CrossRef]
- Hayat, T.; Javed, M.; Asghar, S. Slip effects in peristalsis. Numer. Methods Partial Differ. Equ. 2011, 27, 1003–1015. [Google Scholar] [CrossRef]
- Hayat, T.; Hussain, Q.; Ali, N. Influence of partial slip on the peristaltic flow in a porous medium. Phys. Lett. A 2008, 387, 3399–3409. [Google Scholar] [CrossRef]
- Hayat, T.; Hussain, Q.; Qureshi, M.U.; Ali, N.; Hendi, A.A. Influence of slip condition on the peristaltic transport in an asymmetric channel with heat transfer: An exact solution. Int. J. Numer. Methods Fluids 2011, 67, 1944–1959. [Google Scholar] [CrossRef]
- Ellahi, R.; Hayat, T.; Mahomed, F.M.; Asghar, S. Effects of slip on the nonlinear flow of a third-grade fluid. Nonlinear Anal. Real World Appl. 2010, 11, 139–146. [Google Scholar] [CrossRef]
- Devakar, M.; Sreenivasu, D.; Shankar, B. Analytical solutions of couple stress fluid flows with slip boundary conditions. Alex. Eng. J. 2014, 53, 723–730. [Google Scholar]
- Siddiqui, A.M.; Hameed, M.; Siddiqui, B.M.; Ghori, Q.K. Use of Adomian decomposition method in the study of parallel plate flow of a third-grade fluid. Commun. Nonlinear Sci. Numer. Simul. 2010, 15, 2388–2399. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Ganji, D.D.; Ashorynejad, H.R. Investigation of squeezing unsteady nanofluid flow using ADM. Powder Technol. 2013, 239, 259–265. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Ganji, D.D.; Ashorynejad, H.R.; Rokni, H.B. Analytical investigation of Jeffery-Hamel flow with high magnetic field and nano particle by Adomian decomposition method. Appl. Math. Mech. 2012, 33, 1553–1564. [Google Scholar] [CrossRef]
- Gray, W.G.; Miller, C.T. Thermodynamically constrained averaging theory approach for heat transport in single-fluid-Phase porous medium systems. J. Heat Transf. 2009, 131, 101002. [Google Scholar] [CrossRef]

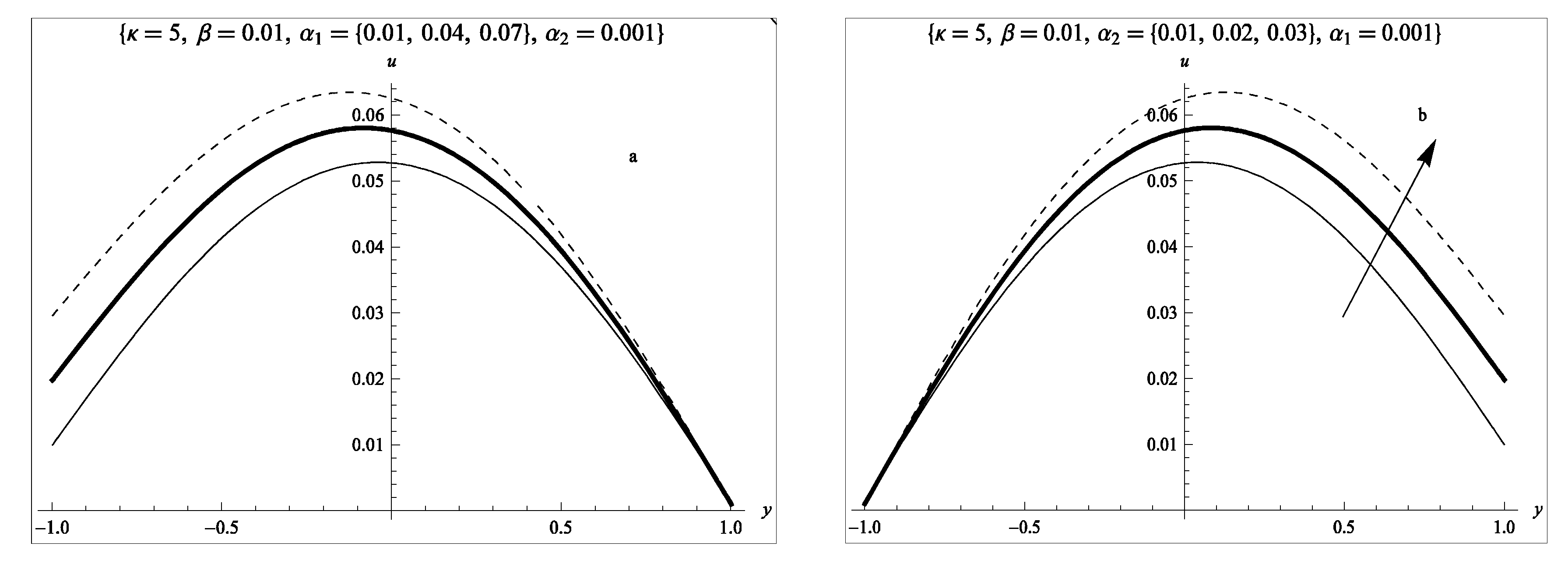

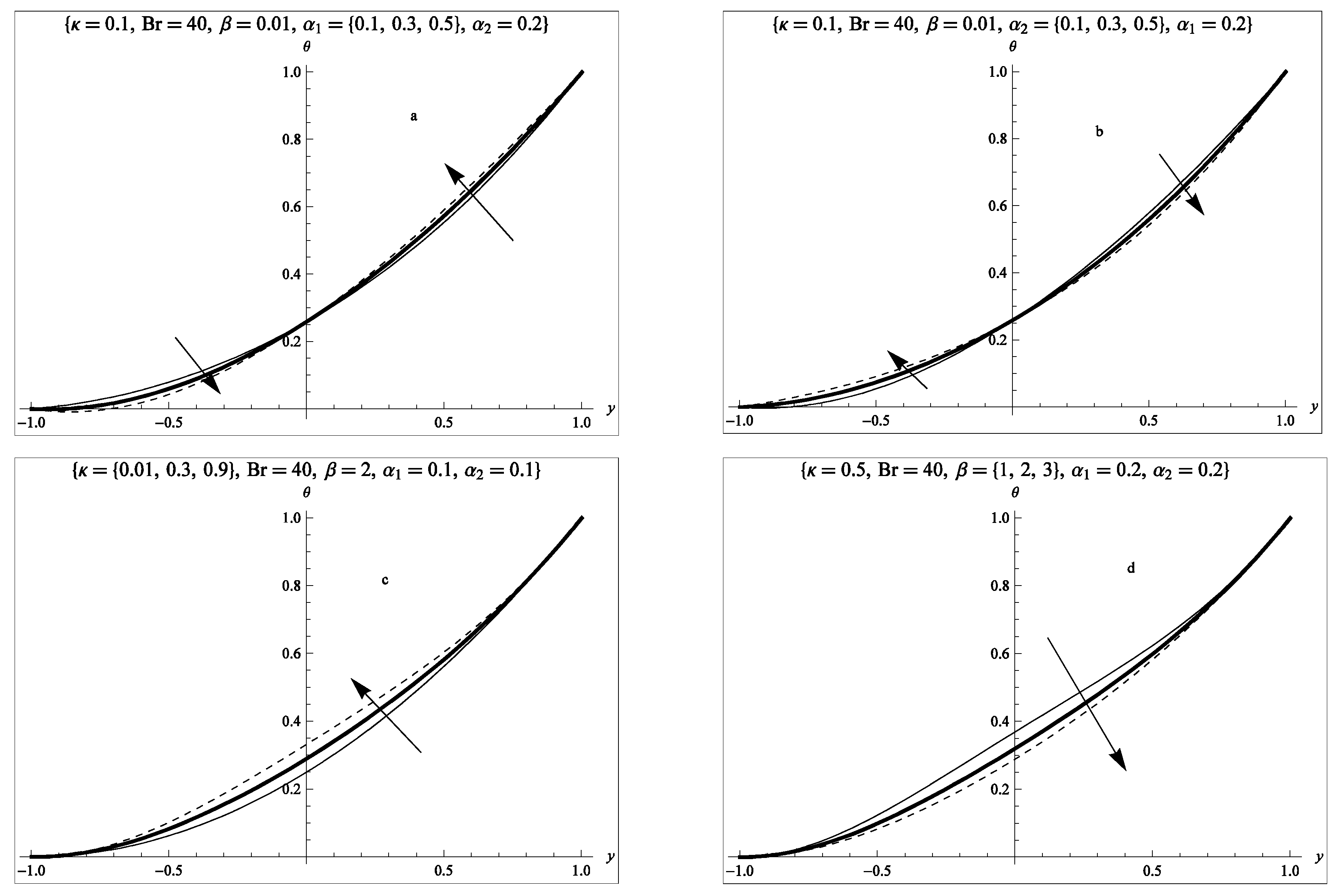
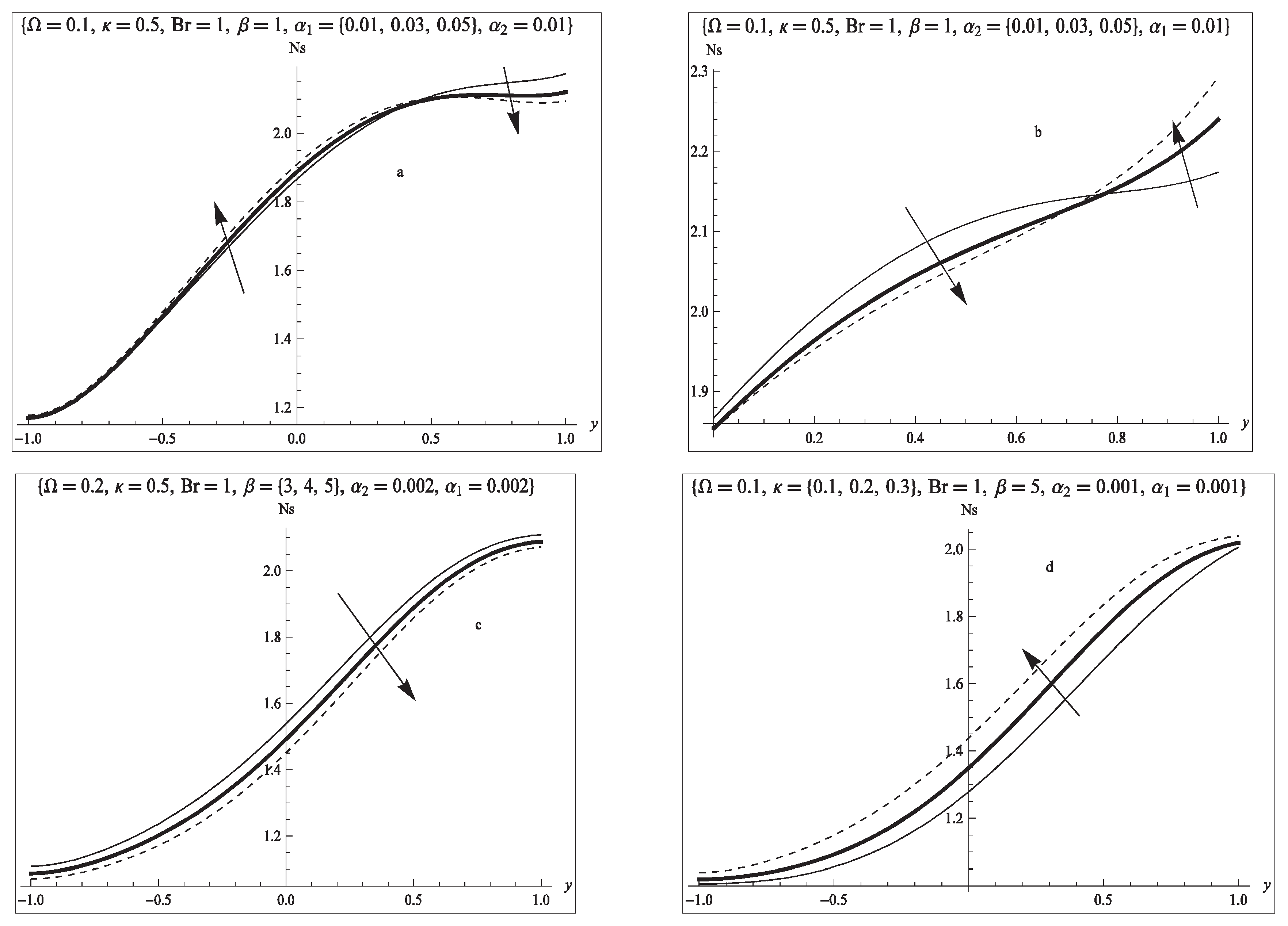

| 1 | 0.0009743 | 0.237460 | −0.736701 | 0.0004275 | −1.00043 | 1.11742 | 0.194213 |
| 2 | 0.0009201 | 0.216357 | −0.703787 | 0.0001636 | −1.00016 | 0.95068 | 0.174346 |
| 3 | 0.0009145 | 0.213902 | −0.700609 | 0.000138 | −1.00001 | 0.91730 | 0.171868 |
| 4 | 0.0009142 | 0.213745 | −0.700042 | 6.33 × 10−7 | −1.00000 | 0.94309 | 0.171704 |
| 5 | 0.0009142 | 0.213738 | −0.700041 | 1.91 × 10−8 | −1.00000 | 0.91416 | 0.171704 |
| 6 | 0.0009142 | 0.213738 | −0.700041 | 6.399 × 10−10 | −1.00000 | 0.91416 | 0.171704 |
| [46] | Present Result | ||
|---|---|---|---|
| −1 | 0.001 | 0.001 | 1.20574 × 10−13 |
| −0.8 | 0.0477304 | 0.0477304 | 1.08022 × 10−11 |
| −0.6 | 0.0892458 | 0.0892458 | 2.09434 × 10−11 |
| −0.4 | 0.121594 | 0.121594 | 2.99819 × 10−11 |
| −0.2 | 0.142059 | 0.142059 | 3.73114 × 10−11 |
| 0.0 | 0.149054 | 0.149054 | 4.22571 × 10−11 |
| 0.2 | 0.142059 | 0.142059 | 4.40482 × 10−11 |
| 0.4 | 0.121594 | 0.121594 | 4.17903 × 10−11 |
| 0.6 | 0.0892458 | 0.0892458 | 3.44567 × 10−11 |
| 0.8 | 0.0477304 | 0.0477304 | 2.10586 × 10−11 |
| 1 | 0.001 | 0.001 | 1.89595 × 10−12 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Adesanya, S.O.; Fakoya, M.B. Second Law Analysis for Couple Stress Fluid Flow through a Porous Medium with Constant Heat Flux. Entropy 2017, 19, 498. https://doi.org/10.3390/e19090498
Adesanya SO, Fakoya MB. Second Law Analysis for Couple Stress Fluid Flow through a Porous Medium with Constant Heat Flux. Entropy. 2017; 19(9):498. https://doi.org/10.3390/e19090498
Chicago/Turabian StyleAdesanya, Samuel Olumide, and Michael Bamidele Fakoya. 2017. "Second Law Analysis for Couple Stress Fluid Flow through a Porous Medium with Constant Heat Flux" Entropy 19, no. 9: 498. https://doi.org/10.3390/e19090498
APA StyleAdesanya, S. O., & Fakoya, M. B. (2017). Second Law Analysis for Couple Stress Fluid Flow through a Porous Medium with Constant Heat Flux. Entropy, 19(9), 498. https://doi.org/10.3390/e19090498





