Understanding Interdependency Through Complex Information Sharing
Abstract
:1. Introduction
2. Preliminaries and the State of the Art
2.1. Negentropy and Total Correlation
2.2. Internal and External Decompositions
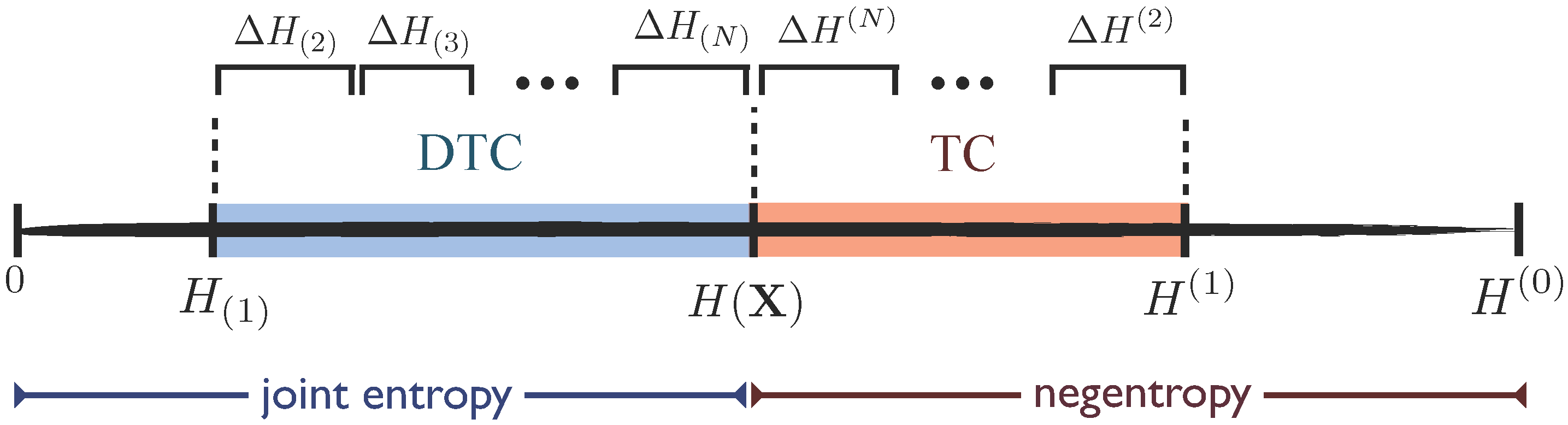
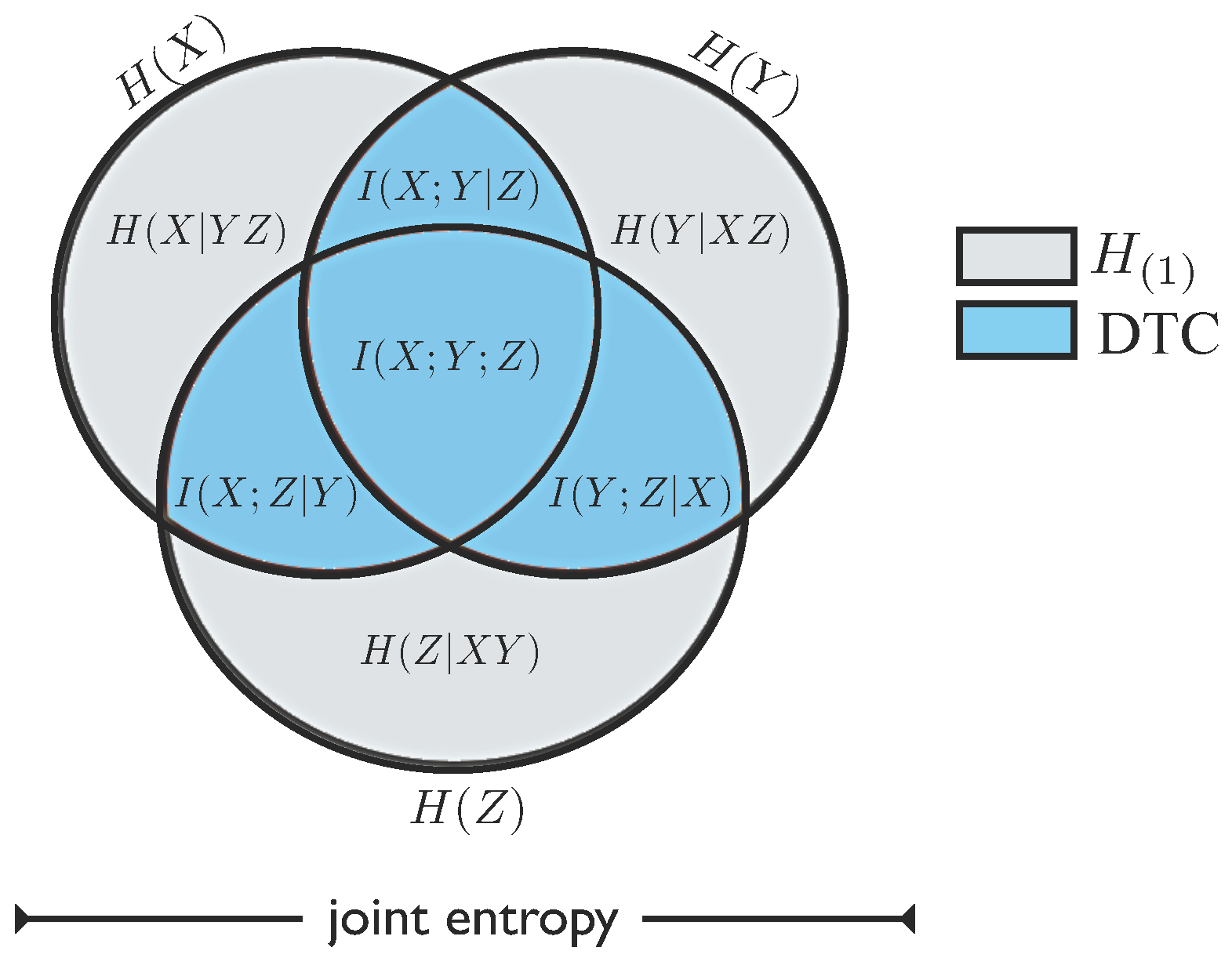
2.3. Inclusion-Exclusion Decompositions
| Name | Formula |
|---|---|
| Total correlation | |
| Dual total correlation | |
| Co-information |
2.4. Synergistic Information
3. A Non-Negative Joint Entropy Decomposition
3.1. Predictability Axioms
- (1)
- Non-negativity: .
- (2)
- .
- (3)
- .
- (4)
- Weak symmetry I: .
3.2. Shared, Private and Synergistic Information
- (5)
- Weak symmetry II: .
- (a)
- Strong symmetry: and are symmetric on their three arguments.
- (b)
- Bounds: these quantities satisfy the following inequalities:
| Directed Measures | Symmetrical Measures |
|---|---|
| Redundant predictability | Shared information |
| Unique predictability | Private information |
| Synergistic predictability | Synergistic information |
3.3. Further Properties of the Symmetrical Decomposition
3.4. Decomposition for the Joint Entropy of Three Variables
4. Pairwise Independent Variables
4.1. Uniqueness of the Entropy Decomposition
4.2. Functions of Independent Arguments
5. Discrete Pairwise Maximum Entropy Distributions and Markov Chains
5.1. Synergy Minimization
5.2. Markov Chains
| Markov Chains | Pairwise Independent Variables |
|---|---|
| Conditional pairwise independency | Pairwise independency |
| No between and | No between and |
| No synergistic information | No shared information |
6. Entropy Decomposition for the Gaussian Case
6.1. Understanding the Synergistic Information Between Gaussians
6.2. Understanding the Shared Information
6.3. Shared, Private and Synergistic Information for Gaussian Variables
7. Applications to Network Information Theory
7.1. Slepian–Wolf Coding
7.2.Multiple Access Channel

7.3. Degraded Wiretap Channel
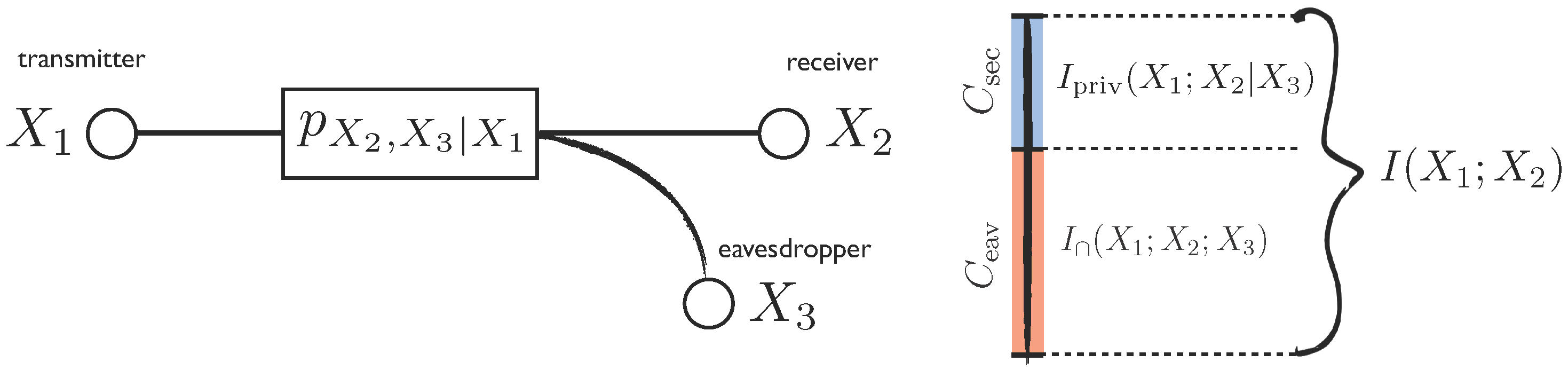
7.4. Gaussian Broadcast Channel
8. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix
A. Proof of Lemma 1
B. Proof of the Consistency of Axiom (3)
C. Proof of Lemma 2
D. Useful Facts about Gaussians
E. Proof of Lemma 5
References
- Kaneko, K. Life: An Introduction to Complex Systems Biology; Springer-Verlag: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Perrings, C. Economy and Environment: A Theoretical Essay on the Interdependence of Economic and Environmental Systems; Cambridge University Press: Cambridge, UK, 2005. [Google Scholar]
- Martignon, L.; Deco, G.; Laskey, K.; Diamond, M.; Freiwald, W.; Vaadia, E. Neural coding: Higher-order temporal patterns in the neurostatistics of cell assemblies. Neural Comput. 2000, 12, 2621–2653. [Google Scholar] [CrossRef] [PubMed]
- Deutscher, D.; Meilijson, I.; Schuster, S.; Ruppin, E. Can single knockouts accurately single out gene functions? BMC Syst. Biol. 2008, 2, 50. [Google Scholar] [CrossRef] [PubMed]
- Anand, K.; Bianconi, G. Entropy measures for networks: Toward an information theory of complex topologies. Phys. Rev. E 2009, 80, 045102. [Google Scholar] [CrossRef] [PubMed]
- Gastpar, M.; Vetterli, M.; Dragotti, P.L. Sensing reality and communicating bits: A dangerous liaison. IEEE Signal Process. Mag. 2006, 23, 70–83. [Google Scholar] [CrossRef]
- Casella, G.; Berger, R.L. Statistical Inference; Duxbury Press: Pacific Grove, CA, USA, 2002. [Google Scholar]
- Cover, T.M.; Thomas, J.A. Elements of Information Theory; John Wiley: Hoboken, NJ, USA, 1991. [Google Scholar]
- Senge, P.M.; Smith, B.; Kruschwitz, N.; Laur, J.; Schley, S. The Necessary Revolution: How Individuals and Organizations Are Working Together to Create a Sustainable World; Crown Business: New York, NY, USA, 2008. [Google Scholar]
- Amari, S.I. Information geometry on hierarchy of probability distributions. IEEE Trans. Inf. Theory 2001, 47, 1701–1711. [Google Scholar] [CrossRef]
- Williams, P.L.; Beer, R.D. Nonnegative decomposition of multivariate information. 2010; arXiv:1004.2515. [Google Scholar]
- Olbrich, E.; Bertschinger, N.; Rauh, J. Information Decomposition and Synergy. Entropy 2015, 17, 3501–3517. [Google Scholar] [CrossRef]
- Li, W. Mutual information functions versus correlation functions. J. Stat. Phys. 1990, 60, 823–837. [Google Scholar] [CrossRef]
- Jaynes, E.T. Probability Theory: The Logic of Science; Cambridge University Press: Cambridge, UK, 2003. [Google Scholar]
- Brillouin, L. The negentropy principle of information. J. Appl. Phys. 1953, 24, 1152–1163. [Google Scholar] [CrossRef]
- Watanabe, S. Information theoretical analysis of multivariate correlation. IBM J. Res. Dev. 1960, 4, 66–82. [Google Scholar] [CrossRef]
- Studenỳ, M.; Vejnarová, J. The multiinformation function as a tool for measuring stochastic dependence. In Learning in Graphical Models; Springer Netherlands: Amsterdam, The Netherlands, 1998; pp. 261–297. [Google Scholar]
- Schneidman, E.; Still, S.; Berry, M.J.; Bialek, W. Network information and connected correlations. Phys. Rev. Lett. 2003, 91, 238701. [Google Scholar] [CrossRef] [PubMed]
- Schneidman, E.; Berry, M.J.; Segev, R.; Bialek, W. Weak pairwise correlations imply strongly correlated network states in a neural population. Nature 2006, 440, 1007–1012. [Google Scholar] [CrossRef] [PubMed]
- Roudi, Y.; Nirenberg, S.; Latham, P.E. Pairwise Maximum Entropy Models for Studying Large Biological Systems: When They Can Work and When They Can’t. PLoS Comput. Biol. 2009, 5, e1000380. [Google Scholar] [CrossRef] [PubMed]
- Bialek, W.; Cavagna, A.; Giardina, I.; Mora, T.; Silvestri, E.; Viale, M.; Walczak, A.M. Statistical mechanics for natural flocks of birds. Proc. Natl. Acad. Sci. USA 2012, 109, 4786–4791. [Google Scholar] [CrossRef] [PubMed]
- Merchan, L.; Nemenman, I. On the sufficiency of pairwise interactions in maximum entropy models of biological networks. 2015; arXiv:1505.02831. [Google Scholar]
- Daniels, B.C.; Krakauer, D.C.; Flack, J.C. Sparse code of conflict in a primate society. Proc. Natl. Acad. Sci. USA 2012, 109, 14259–14264. [Google Scholar] [CrossRef] [PubMed]
- Lee, E.D.; Broedersz, C.P.; Bialek, W. Statistical mechanics of the US Supreme Court. J. Stat. Phys. 2013, 160, 275–301. [Google Scholar] [CrossRef]
- Sun, H.T. Nonnegative entropy measures of multivariate symmetric correlations. Inf. Control 1978, 36, 133–156. [Google Scholar]
- Olbrich, E.; Bertschinger, N.; Ay, N.; Jost, J. How should complexity scale with system size? Eur. Phys. J. B 2008, 63, 407–415. [Google Scholar] [CrossRef]
- Crutchfield, J.P.; Feldman, D.P. Regularities unseen, randomness observed: Levels of entropy convergence. Chaos 2003, 13, 25–54. [Google Scholar] [CrossRef] [PubMed]
- Rosas, F.; Ntranos, V.; Ellison, C.J.; Verhelst, M.; Pollin, S. Understanding high-order correlations using a synergy-based decomposition of the total entropy. In Proceedings of the 5th Joint WIC/IEEE Symposium on Information Theory and Signal Processing in the Benelux, Brussels, Belgium, 6–7 May 2015; pp. 146–153.
- Yeung, R.W. A new outlook on Shannon’s information measures. IEEE Trans. Inf. Theory 1991, 37, 466–474. [Google Scholar] [CrossRef]
- Bar-Yam, Y. Multiscale complexity/entropy. Adv. Complex Syst. 2004, 7, 47–63. [Google Scholar] [CrossRef]
- Bell, A.J. The co-information lattice. In Proceedings of the 4th International Symposium on Independent Component Analysis and Blind Signal Separation (ICA 2003), Nara, Japan, 1–4 April 2003; pp. 921–926.
- McGill, W.J. Multivariate information transmission. Psychometrika 1954, 19, 97–116. [Google Scholar] [CrossRef]
- James, R.G.; Ellison, C.J.; Crutchfield, J.P. Anatomy of a bit: Information in a time series observation. Chaos Interdiscip. J Nonlinear Sci. 2011, 21, 037109. [Google Scholar] [CrossRef] [PubMed]
- Griffith, V.; Koch, C. Quantifying Synergistic Mutual Information. In Guided Self-Organization: Inception; Prokopenko, M., Ed.; Springer-Verlag: Berlin/Heidelberg, Germany, 2014; Volume 9, pp. 159–190. [Google Scholar]
- Griffith, V. Quantifying synergistic information. Ph.D. Thesis, California Institute of Technology, Pasadena, CA, USA, 2014. [Google Scholar]
- Jiao, J.; Courtade, T.; Venkat, K.; Weissman, T. Justification of Logarithmic Loss via the Benefit of Side Information. IEEE Trans. Inf. Theory 2015, 61, 5357–5365. [Google Scholar] [CrossRef]
- Harder, M.; Salge, C.; Polani, D. Bivariate measure of redundant information. Phys. Rev. E 2013, 87, 012130. [Google Scholar] [CrossRef] [PubMed]
- Griffith, V.; Chong, E.K.; James, R.G.; Ellison, C.J.; Crutchfield, J.P. Intersection information based on common randomness. Entropy 2014, 16, 1985–2000. [Google Scholar] [CrossRef]
- Bertschinger, N.; Rauh, J.; Olbrich, E.; Jost, J.; Ay, N. Quantifying unique information. Entropy 2014, 16, 2161–2183. [Google Scholar] [CrossRef]
- Bertschinger, N.; Rauh, J.; Olbrich, E.; Jost, J. Shared information—New insights and problems in decomposing information in complex systems. In Proceedings of the European Conference on Complex Systems 2012, Brussels, Belgium, 3–7 September 2012; pp. 251–269.
- Barrett, A.B. Exploration of synergistic and redundant information sharing in static and dynamical Gaussian systems. Phys. Rev. E 2015, 91, 052802. [Google Scholar] [CrossRef] [PubMed]
- Berkson, J. Limitations of the application of fourfold table analysis to hospital data. Biom. Bull. 1946, 2, 47–53. [Google Scholar] [CrossRef]
- Kim, J.; Pearl, J. A computational model for causal and diagnostic reasoning in inference systems. In Proceedings of the Eighth International Joint Conference on Artificial Intelligence (IJCAI), Karlsruhe, Germany, 8–12 August 1983; pp. 190–193.
- Bloch, M.; Barros, J. Physical-Layer Security: From Information Theory to Security Engineering; Cambridge University Press: Cambrige, UK, 2011. [Google Scholar]
- Shannon, C.E. Communication theory of secrecy systems*. Bell Syst. Tech. J. 1949, 28, 656–715. [Google Scholar] [CrossRef]
- Ahlswede, R.; Cai, N.; Li, S.Y.R.; Yeung, R.W. Network information flow. IEEE Trans. Inf. Theory 2000, 46, 1204–1216. [Google Scholar] [CrossRef]
- Li, S.Y.R.; Yeung, R.W.; Cai, N. Linear network coding. IEEE Trans. Inf. Theory 2003, 49, 371–381. [Google Scholar] [CrossRef]
- Katti, S.; Rahul, H.; Hu, W.; Katabi, D.; Médard, M.; Crowcroft, J. XORs in the air: Practical wireless network coding. IEEE/ACM Trans. Netw. 2008, 16, 497–510. [Google Scholar] [CrossRef]
- Landau, L.; Lifshitz, E. Statistical Physics, 2nd ed.; Pergamon Press: Oxford, UK, 1970; Volume 5. [Google Scholar]
- Wainwright, M.J.; Jordan, M.I. Graphical models, exponential families, and variational inference. Found. Trends Mach. Learn. 2008, 1, 1–305. [Google Scholar] [CrossRef]
- Cipra, B.A. An introduction to the Ising model. Am. Math. Mon. 1987, 94, 937–959. [Google Scholar] [CrossRef]
- Sayed, A.H. Adaptive Filters; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- El Gamal, A.; Kim, Y.H. Network Information Theory; Cambridge University Press: Cambridge, UK, 2011. [Google Scholar]
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons by Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rosas, F.; Ntranos, V.; Ellison, C.J.; Pollin, S.; Verhelst, M. Understanding Interdependency Through Complex Information Sharing. Entropy 2016, 18, 38. https://doi.org/10.3390/e18020038
Rosas F, Ntranos V, Ellison CJ, Pollin S, Verhelst M. Understanding Interdependency Through Complex Information Sharing. Entropy. 2016; 18(2):38. https://doi.org/10.3390/e18020038
Chicago/Turabian StyleRosas, Fernando, Vasilis Ntranos, Christopher J. Ellison, Sofie Pollin, and Marian Verhelst. 2016. "Understanding Interdependency Through Complex Information Sharing" Entropy 18, no. 2: 38. https://doi.org/10.3390/e18020038
APA StyleRosas, F., Ntranos, V., Ellison, C. J., Pollin, S., & Verhelst, M. (2016). Understanding Interdependency Through Complex Information Sharing. Entropy, 18(2), 38. https://doi.org/10.3390/e18020038






