Rectification and Non-Gaussian Diffusion in Heterogeneous Media
Abstract
:1. Introduction
2. Diffusion in Heterogeneous Systems
3. Results
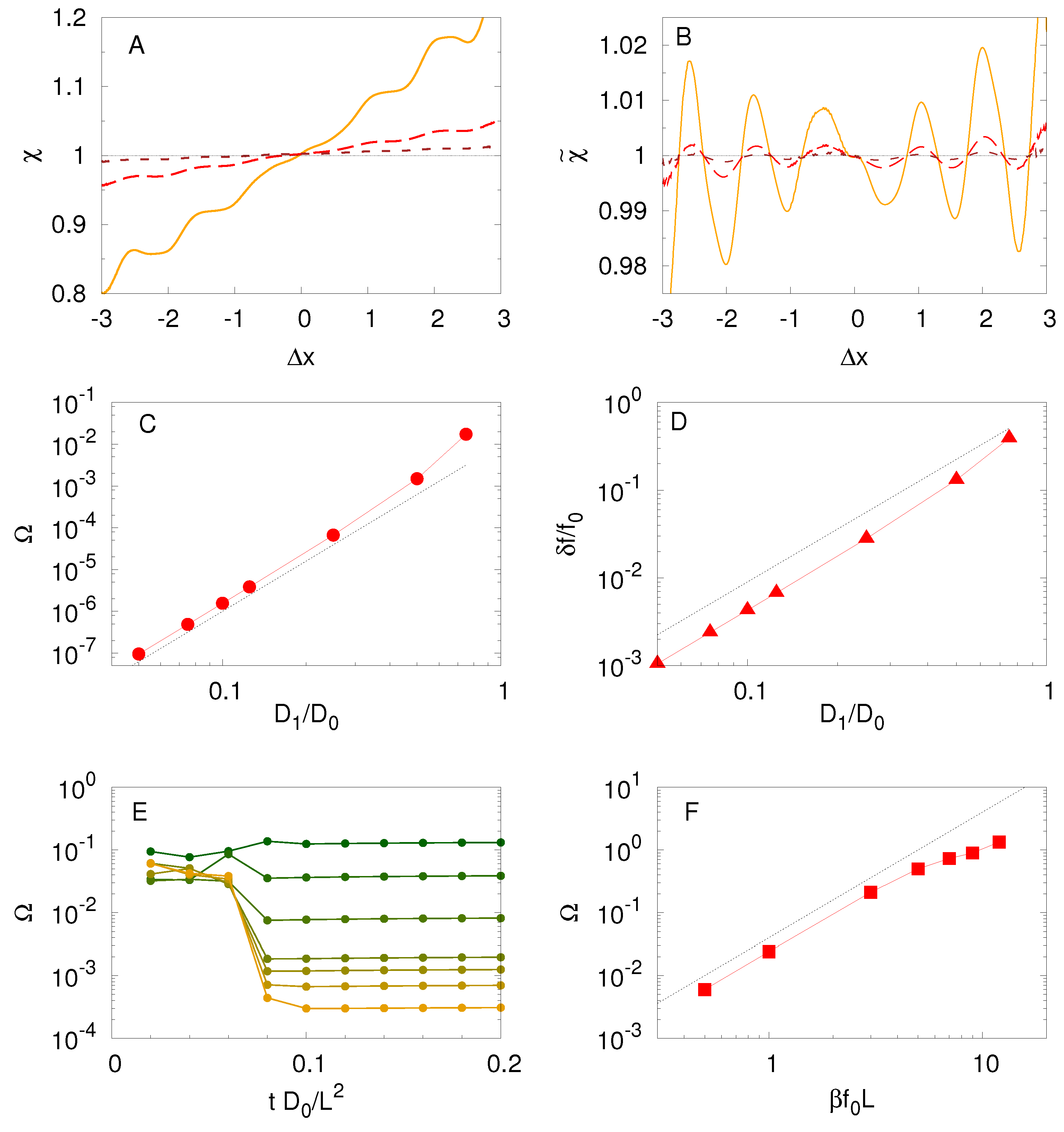
3.1. Diffusion in an Inhomogeneous Unbounded Medium
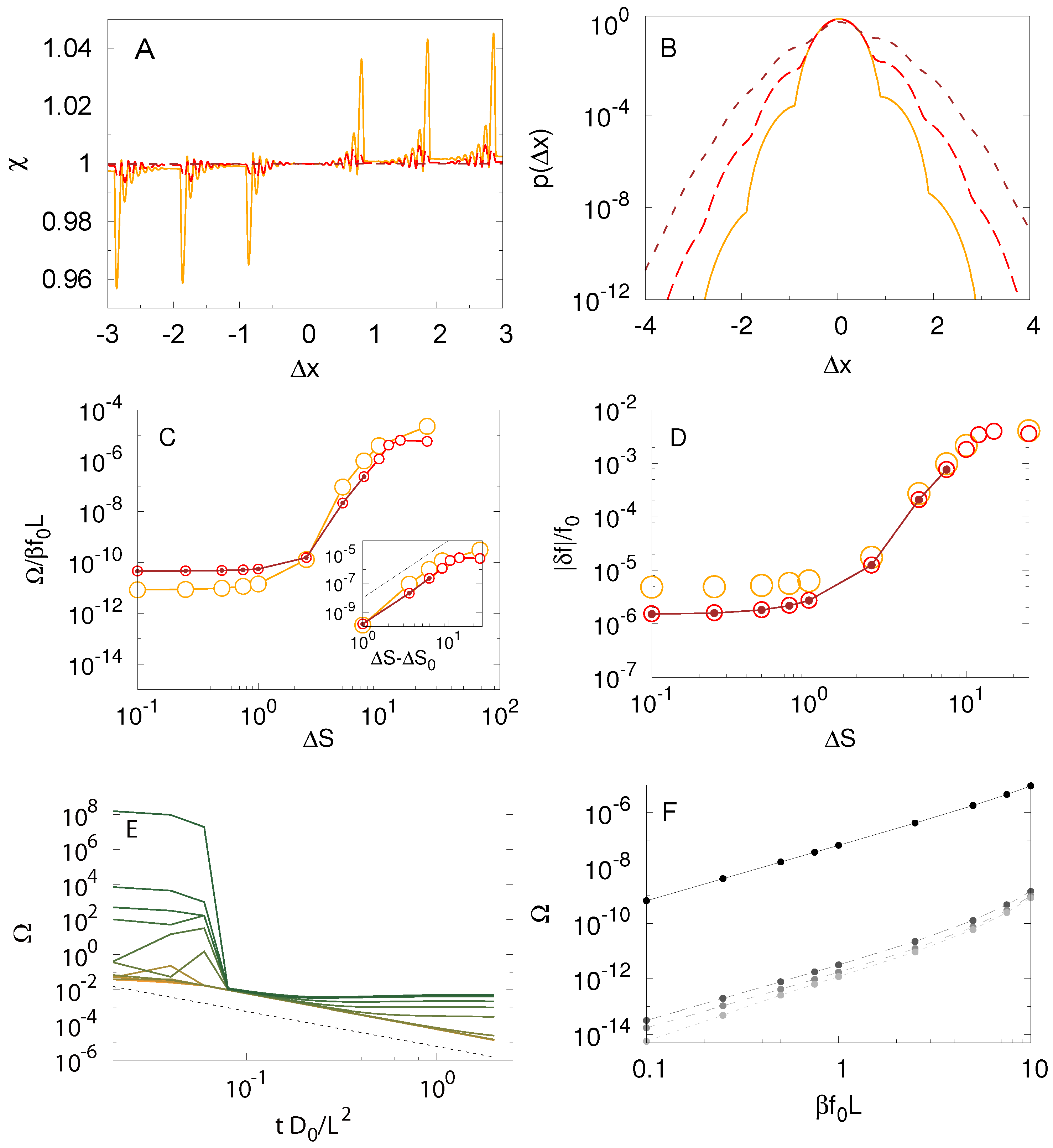
3.2. Diffusion in a Periodic Channel
4. Discussion
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix A
References
- Campisi, M.; Hänggi, P.; Talkner, P. Colloquium: Quantum fluctuation relations: Foundations and applications. Rev. Mod. Phys. 2011, 83, 771. [Google Scholar] [CrossRef]
- Astumian, R.D. The unreasonable effectiveness of equilibrium theory for interpreting nonequilibrium experiments. Am. J. Phys. 2006, 74, 683. [Google Scholar] [CrossRef]
- Astumian, R.D. Equilibrium theory for a particle pulled by a moving optical trap. J. Chem. Phys. 2007, 126, 111102. [Google Scholar] [CrossRef] [PubMed]
- Reguera, D.; Rubi, J.M. Thermodynamics and stochastic dynamics of transport in confined media. Chem. Phys. 2010, 375, 518–522. [Google Scholar]
- Ciliberto, S.; Joubaud, S.; Petrosyan, A. Fluctuations in out-of-equilibrium systems: From theory to experiment. J. Stat. Mech. 2010, 2010, P12003. [Google Scholar] [CrossRef]
- Gallavotti, G.; Cohen, E.G.D. Dynamical Ensembles in Nonequilibrium Statistical Mechanics. Phys. Rev. Lett. 1995, 74, 2694. [Google Scholar] [CrossRef] [PubMed]
- Crooks, G.E. Entropy production fluctuation theorem and the nonequilibrium work relation for free energy differences. Phys. Rev. E 1999, 60, 2721. [Google Scholar] [CrossRef]
- Vainstein, M.H.; Rubi, J.M. Gaussian noise and time-reversal symmetry in nonequilibrium Langevin models. Phys. Rev. E 2007, 75, 031106. [Google Scholar] [CrossRef] [PubMed]
- Risken, H. The Fokker-Planck Equation; Springer: Berlin/Heidelberg, Germany, 1988. [Google Scholar]
- Pagonabarraga, I.; Rubi, J.M. Long-range correlations in diffusive systems away from equilibrium. Phys. Rev. E 1994, 49, 267. [Google Scholar] [CrossRef]
- Höfling, F.; Franosch, T. Anomalous transport in the crowded world of biological cells. Rep. Prog. Phys. 2013, 76, 046602. [Google Scholar] [CrossRef] [PubMed]
- Maes, C.; Steffenoni, S. Friction and noise for a probe in a nonequilibrium fluid. Phys. Rev. E 2015, 91, 022128. [Google Scholar] [CrossRef] [PubMed]
- Bénichou, O.; Illien, P.; Oshanin, G.; Sarracino, A.; Voituriez, R. Diffusion and Subdiffusion of Interacting Particles on Comblike Structures. Phys. Rev. Lett. 2015, 115, 220601. [Google Scholar] [CrossRef] [PubMed]
- Marconi, U.M.B.; Malgaretti, P.; Pagonabarraga, I. Tracer diffusion of hard-sphere binary mixtures under nano-confinement. J. Chem. Phys. 2015, 143, 184501. [Google Scholar] [CrossRef] [PubMed]
- Jacobs, M.H. Diffusion Processes; Springer: Berlin/Heidelberg, Germany, 1967. [Google Scholar]
- Zwanzig, R. Diffusion past an entropy barrier. J. Phys. Chem. 1992, 96, 3926–3930. [Google Scholar] [CrossRef]
- Reguera, D.; Rubi, J.M. Kinetic equations for diffusion in the presence of entropic barriers. Phys. Rev. E 2001, 64, 061106. [Google Scholar] [CrossRef] [PubMed]
- Kalinay, P.; Percus, J.K. Approximations of the generalized Fick-Jacobs equation. Phys. Rev. E 2008, 78, 021103. [Google Scholar] [CrossRef] [PubMed]
- Kalinay, P.; Percus, J.K. Mapping of diffusion in a channel with abrupt change of diameter. Phys. Rev. E 2010, 82, 031143. [Google Scholar] [CrossRef] [PubMed]
- Dagdug, L.; Berezhkovskii, A.M.; Makhnovskii, Y.A.; Zitsereman, V.Y.; Bezrukov, S. Communication: Turnover behavior of effective mobility in a tube with periodic entropy pot. J. Chem. Phys. 2011, 134, 101102. [Google Scholar] [CrossRef] [PubMed]
- Malgaretti, P.; Pagonabarraga, I.; Rubi, J.M. Entropic transport in confined media: A challenge for computational studies in biological and soft-matter systems. Front. Phys. 2013, 1, 21. [Google Scholar] [CrossRef]
- Chacón-Acosta, G.; Pineda, I.; Dagdug, L. Diffusion in narrow channels on curved manifolds. J. Chem. Phys. 2013, 139, 214115. [Google Scholar] [CrossRef] [PubMed]
- Kalinay, P. Moment expansion for mapping of the confined diffusion. Phys. Rev. E 2013, 87, 032143. [Google Scholar] [CrossRef]
- Kalinay, P. Integral formula for the effective diffusion coefficient in two-dimensional channels. Phys. Rev. E 2016, 94, 012102. [Google Scholar] [CrossRef] [PubMed]
- Chinappi, M.; De Angelis, E.; Melchionna, S.; Casciola, C.M.; Succi, S.; Piva, R. Molecular Dynamics Simulation of Ratchet Motion in an Asymmetric Nanochannel. Phys. Rev. Lett. 2006, 97, 144509. [Google Scholar] [CrossRef] [PubMed]
- Marconi, U.M.B.; Melchionna, S.; Pagonabarraga, I. Effective electrodiffusion equation for non-uniform nanochannels. J. Chem. Phys. 2013, 138, 244107. [Google Scholar] [CrossRef] [PubMed]
- Malgaretti, P.; Pagonabarraga, I.; Rubi, J.M. Entropic Electrokinetics: Recirculation, Particle Separation, and Negative Mobility. Phys. Rev. Lett. 2014, 113, 128301. [Google Scholar] [CrossRef] [PubMed]
- Malgaretti, P.; Pagonabarraga, I.; Rubi, J.M. Geometrically Tuned Channel Permeability. Macromol. Symp. 2015, 357, 178–188. [Google Scholar] [CrossRef]
- Malgaretti, P.; Pagonabarraga, I.; Rubi, J.M. Entropically induced asymmetric passage times of charged tracers across corrugated channels. J. Chem. Phys. 2016, 144, 034901. [Google Scholar] [CrossRef] [PubMed]
- Bianco, V.; Malgaretti, P. Non-monotonous polymer translocation time across corrugated channels: Comparison between Fick-Jacobs approximation and numerical simulations. J. Chem. Phys. 2016, 145, 114904. [Google Scholar] [CrossRef]
- Malgaretti, P.; Pagonabarraga, I.; Rubi, J.M. Cooperative rectification in confined Brownian ratchets. Phys. Rev. E 2012, 85, 010105. [Google Scholar] [CrossRef] [PubMed]
- Malgaretti, P.; Pagonabarraga, I.; Rubi, J.M. Confined Brownian ratchets. J. Chem. Phys. 2013, 138, 194906. [Google Scholar] [CrossRef] [PubMed]
- Malgaretti, P.; Pagonabarraga, I.; Rubi, J.M. Working under confinement. Eur. Phys. J. Spec. Top. 2014, 223, 3295–3309. [Google Scholar] [CrossRef]
- Martens, S.; Schmid, G.; Schimansky-Geier, L.; Hänggi, P. Entropic particle transport: Higher-order corrections to the Fick-Jacobs diffusion equation. Phys. Rev. E 2011, 83, 051135. [Google Scholar] [CrossRef] [PubMed]
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Malgaretti, P.; Pagonabarraga, I.; Rubi, J.M. Rectification and Non-Gaussian Diffusion in Heterogeneous Media. Entropy 2016, 18, 394. https://doi.org/10.3390/e18110394
Malgaretti P, Pagonabarraga I, Rubi JM. Rectification and Non-Gaussian Diffusion in Heterogeneous Media. Entropy. 2016; 18(11):394. https://doi.org/10.3390/e18110394
Chicago/Turabian StyleMalgaretti, Paolo, Ignacio Pagonabarraga, and J. Miguel Rubi. 2016. "Rectification and Non-Gaussian Diffusion in Heterogeneous Media" Entropy 18, no. 11: 394. https://doi.org/10.3390/e18110394
APA StyleMalgaretti, P., Pagonabarraga, I., & Rubi, J. M. (2016). Rectification and Non-Gaussian Diffusion in Heterogeneous Media. Entropy, 18(11), 394. https://doi.org/10.3390/e18110394






