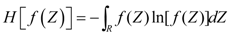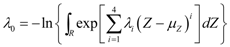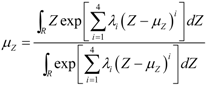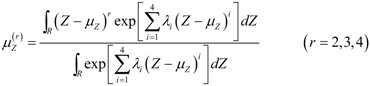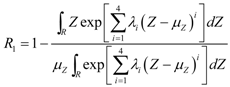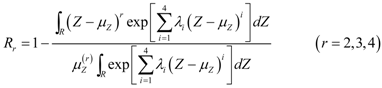1. Introduction
Scour downstream of dams is one of the main concerns in hydraulic engineering. The transfer of water to the downstream river may scour the dam foundation and the downstream riverbed. In the long term, this scour process may create structural safety problems. Hence, accurate predictions of time evolution and ultimate scour depth are required [
1]. A number of equations and models have been developed in the past to predict the potential free jet scour depth below dams and several studies have been carried out to estimate downstream scour below dams [
2,
3,
4,
5,
6].These scour prediction equations are deterministic in nature, and do not account for the uncertainties of the parameters involved in the scour depth equations. Today's most popular evaluation methods are of empirical and semi empirical nature. They do not fully describe the physical background of the phenomenon. A physical based scour prediction model, the Comprehensive Scour Model, has been developed based on a parametric description of the main physical processes responsible for scour [
7,
8].The physically based approach gives a much more reliable scour estimation than simple empirical formulas.
The inherent uncertainties in various model parameters suggest that dam foundation safety would be ensured in a probabilistic sense. Reliability analysis basically provides a quantitative estimation of dam safety against scouring. Several authors have addressed reliability analysis of scouring in recent years. Muzzammil has presented a methodology for the reliability assessment of scour downstream of a ski-jump bucket using the first-order reliability method (FORM) [
9]. Yanmaz has assessed the reliability of the scouring of foundations downstream of outlet facilities [
10]; reliability computations are carried out by simulating the safety margin using the Monte Carlo technique. Ghahfarokhi uses the probabilistic method to estimate plunge pool erosion downstream of a ski jump bucket [
11]. Hydraulic variables involved in plunge pools, such as discharge, flow depth and velocity, are stochastic in nature, and therefore may be represented by relevant probability distributions.
The maximum entropy method (MEM), which is based on Shannon's measure of uncertainty, has been used for estimating distribution functions [
12,
13,
14]. MEM is regarded as the most unbiased estimation for the PDF, which means the most probable PDF from all the PDF under the moment's constraint since “it is maximally noncommittal with regard to missing information”.
The objective of this study is to present a methodology for quantifying the probability of dam failure due to scour. In order to evaluate the stability of dam foundation, the paper combined the maximum entropy principle with Monte Carlo method. The probability density function (PDF) of performance function is calculated based on maximum-entropy method. Monte Carlo simulation (MCS) is used to generate random samples of the variables according to the given probability distributions.
2. Scour Model of Free Over Fall Jet
Free over fall jet scour over the top of arch dams. With free over fall over the top, the jet would fall very near the base of the dam; a deep scour hole (plunge pool) in the riverbed may pose safety problems for the dam foundation. Hydraulic, geotechnical and morphologic factors control the depth of plunge pool. The mechanism of scour is an extremely complex process due to the influence of various hydraulic, hydrological and geological factors. There are many formulae developed over the years to predict the maximum scour depth downstream of a hydraulic structure. No formulas are presently available to accurately determine the maximum depth of the plunge pool. However, based on laboratory studies and field information obtained in several projects, a number of empirical formulas have been developed for predicting the maximum depth of scour of a stable plunge pool. Among these are the ones proposed by Veronese [
15], Mason [
16] and Chen [
17]. Bollaert [
7,
8] presents a physically based model for evaluation of rock scour due to high-velocity jet impact.
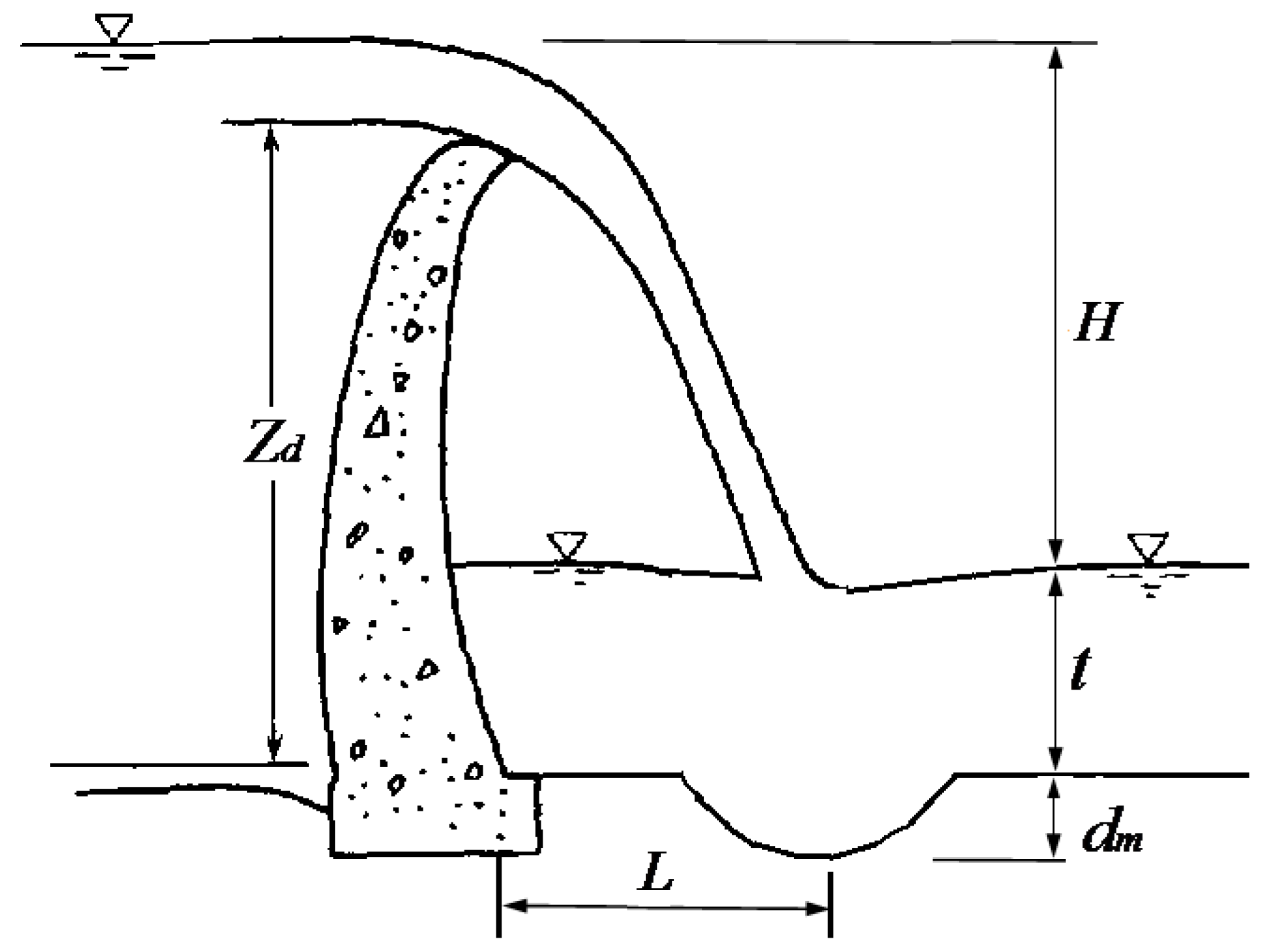
Figure 1 shows a free jet discharging over a dam. The Veronese equation yields an estimate of scour measured from the tail water surface to the bottom of the plunge pool. This equation is given as:
where:
is the maximum scour depth,
is the tail water depth,
is the water discharge per unit width,
is the elevation difference between reservoir and tail water (total water head).
The Veronose equation was published about 60 years ago and has been recognized as a significant basis for scour depth investigations.
Figure 1.
Definition sketch of free over fall jet.
Figure 1.
Definition sketch of free over fall jet.
Based on prototype data from dams in China, Chen [
17] proposed the following formula:
where
is the scouring coefficient, varying with capacity of rocky riverbed to resist scour, for solid rock
for medium rock
, and for soft or broken rock
[
18].
q and
H are the same as in Equation (1). Because of its convenient and concise form, formula (2) is widely used in China to estimate the maximum scour depth at downstream of hydraulic structures or spillways.
The distance for maximum scour depth from the dam (
) can be written as a function of the discharge per unit width
and the elevation difference
. According to the Design Specifications for Concrete Arch Dams (SL282–2003), the distance of the maximum scour point is given as follows [
19]:
where:
L is the distance of maximum scour point from the dam toe,
is the elevation difference between the top of dam and the riverbed.
3. Scouring Reliability of Dam Foundation
3.2. Uncertainty Analysis
Dam foundations may be seriously scoured under jet action. The degree of scour depends on the characteristics of the jet leaving the top of dams, the depth of the tail water, and the properties of the bed material, which are uncertain in nature. The Equations (2) and (3) are deterministic; they don't account for uncertainties in the model parameters, or the hydraulic variables. In fact, hydraulic variables involved in plunge pools, such as discharge, flow depth, and velocity, are stochastic in nature, which may be represented by relevant probability distributions.
In this study, Monte Carlo simulation (MCS), as a significant sampling technique, is used to quantify the uncertainty of desired uncertain random variables. Monte Carlo simulations a powerful analysis tool that involves a random number generation and simulates the behavior of a variable when the data is insufficient to make decisions. In Monte Carlo simulation, probability distributions are assumed for the uncertain variables for the system being studied. Random values of each of the uncertain variables are generated according to their respective probability distributions.
4. Maximum Entropy Distribution
4.1. Determination of Density Function
The maximum entropy method is based on the concept that the distribution that maximizes the information entropy is the statistically most likely to occur. Here the maximum entropy method is used to approximate the PDF of the performance function. Shannon [
20] defined entropy as a measure of uncertainty about a random variable. Based on the entropy principle proposed by Shannon entropy distributions are defined to be those which maximize the information entropy measure:
where
is the probability density function of the performance function,
, and
is the integral domain.
Jaynes [
21] formulated the maximum entropy principle as a rational approach for choosing a consistent probability distribution, amongst all possible distribution, that contains a minimum of spurious information. The principle states that the most unbiased estimate of a probability distribution is that which maximizes the entropy subject to constraints supplied by the available information, e.g., moments of a random variable. The maximum entropy method of estimating
is stated as follows:
where
μz is the mean value of the performance function,
,
denotes the
i-th central moment of
;
m is the number of the given moment constrains. Max means that when the entropy reaches the maximum, we obtain the best probability density function.
denotes the PDF of
g(
X) to be determined by the maximum entropy. The optimal solution of Equation (10) is the maximum entropy estimate of
.
In many studies, it was shown that the first four moments are sufficient to describe a wide range of distribution types [
22,
23]. We use Lagrange's method to solve for the PDF. The solution of Equation (9), subject to Equation (10), can be obtained by using the method of Lagrange multipliers. This yields:
where
,
, are Lagrange multipliers, which can be expressed in terms of constraints given by Equation(10).To compute a MEM density, we have to determine the Lagrange multipliers
by the non-linear of equations.
4.2. Solving the Lagrangian Multipliers
To find the maximum entropy solution, we have to solve for the Lagrangian multipliers. Five Lagrangian multipliers
,
, can be obtained by solving the following equations:
For a more convenient numerical solution, Equation (12) and (13) are changed as follows:
Where the
are the residuals that are reduced to near zero by a numerical technique. A solution can be obtained by using nonlinear programming to obtain the minimum of the sum of the squares of the residuals:
Convergence is achieved when , or , where is the specified acceptable error. Equation (12) is used to obtain .
4.3. Calculation of Failure Probability
Based on the probability density function
, the failure probability of the dam foundation can be calculated as:
where
denotes the probability density function (PDF) of the performance function,
, and the integral is carried out over the failure domain.
5. Example
The application example corresponds to the Ertan Dam. A reliability evaluation of the dam foundation subjected to scouring was carried out. The Ertan Damis an arch dam on the Yalong River, a tributary of the Yangtze River in Sichuan Province, southwest China. The Ertan Dam is a 240 m high and 774.7 long double-curvature arch dam. The dam withholds a normal reservoir of 580 × 10
6 m
3. The dam has four different ways to discharge water downstream. The dam has the two large spillway tunnels (13 m × 13.5 m), seven surface spillways, six middle outlets and four bottom outlets. The seven surface spillways can discharge 6,260 m
3/s. In this example, we study the scour of the surface spillways of Ertan dam. The formula for discharge per unit width is [
24]:
where:
is contraction coefficient,
is design water head,
is discharge coefficient corresponding to
,
,
is water head over the weir crest.
Three random variables are considered: water level (
H), scour coefficient (
k) and the downstream water depth (
).
can be calculated by the downstream water level and the riverbed level. The distributions of three random variables are assumed to obey the normal distribution based on the analysis and study of existing research results [
25,
26,
27]. The means and standard deviations of the random variables are listed in
Table 1.
Table 1.
Parameters of random variables.
Table 1.
Parameters of random variables.
| Variables | Distribution | Mean value | Standard deviation |
|---|
| Scour coefficient, | Normal | 1.35 | 0.22 |
| water head, H (m) | Normal | 202.0 | 3.49 |
| unit discharge, q(m3/m.s) | Normal | 60.08 | 1.03 |
Monte Carlo simulation is used to generate random samples of the variables according to the probability distributions. The values of the variables are randomly generated according to their probability density functions.
The performance function may be written as:
In this equation,
indicates failure. Considering this single performance function, an estimate is required for the probability
. Taking
C as 3, the PDF of the performance function can be expressed as Equation (21) according to the MEM:
The probability of failure is:
To investigate the effect of
k,
H and
q on reliability, different values of
k,
H and
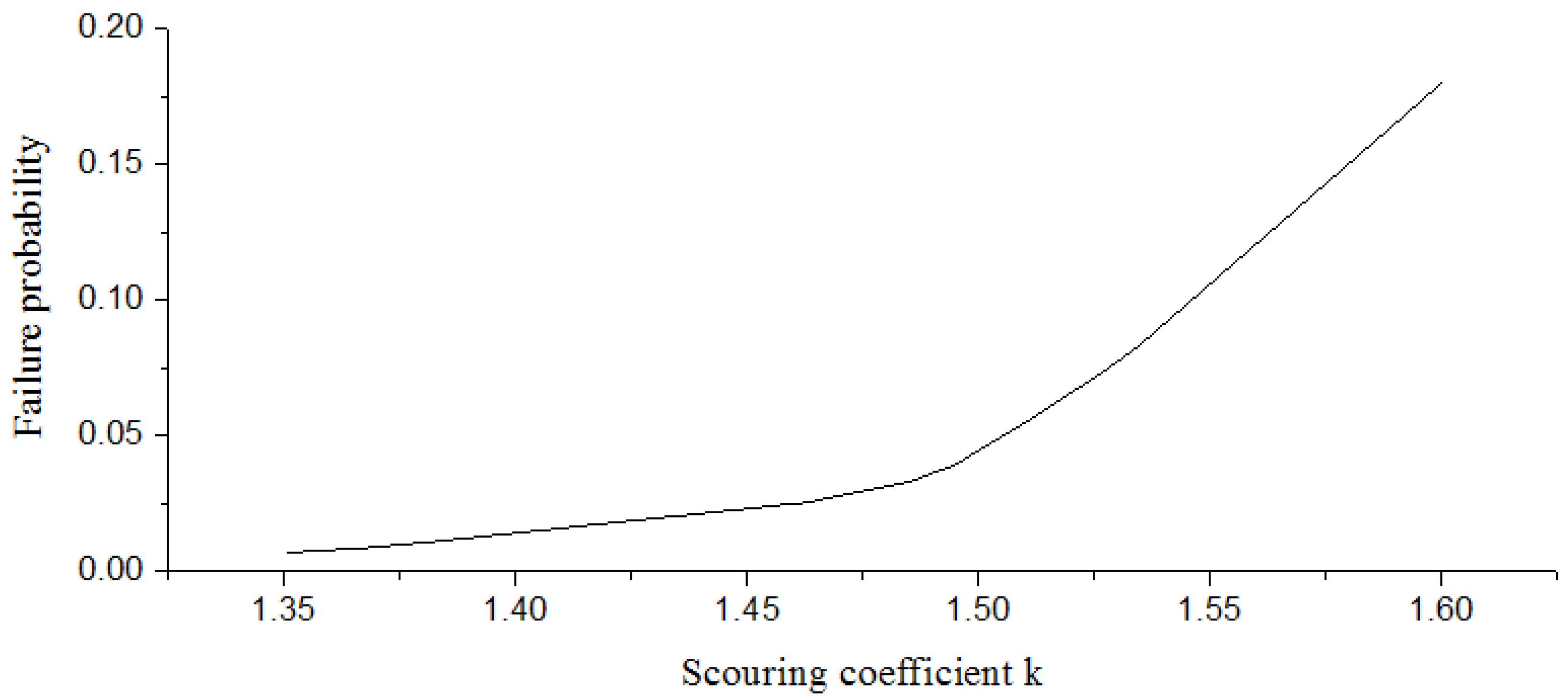
q was taken into account. The variations of the failure probability with respect to the scouring coefficient, discharge per unit width, and water head are shown in
Figure 2,
Figure 3 and
Figure 4.
Figure 2.
Relationship between failure probability and scouring coefficient.
Figure 2.
Relationship between failure probability and scouring coefficient.
Figure 3.
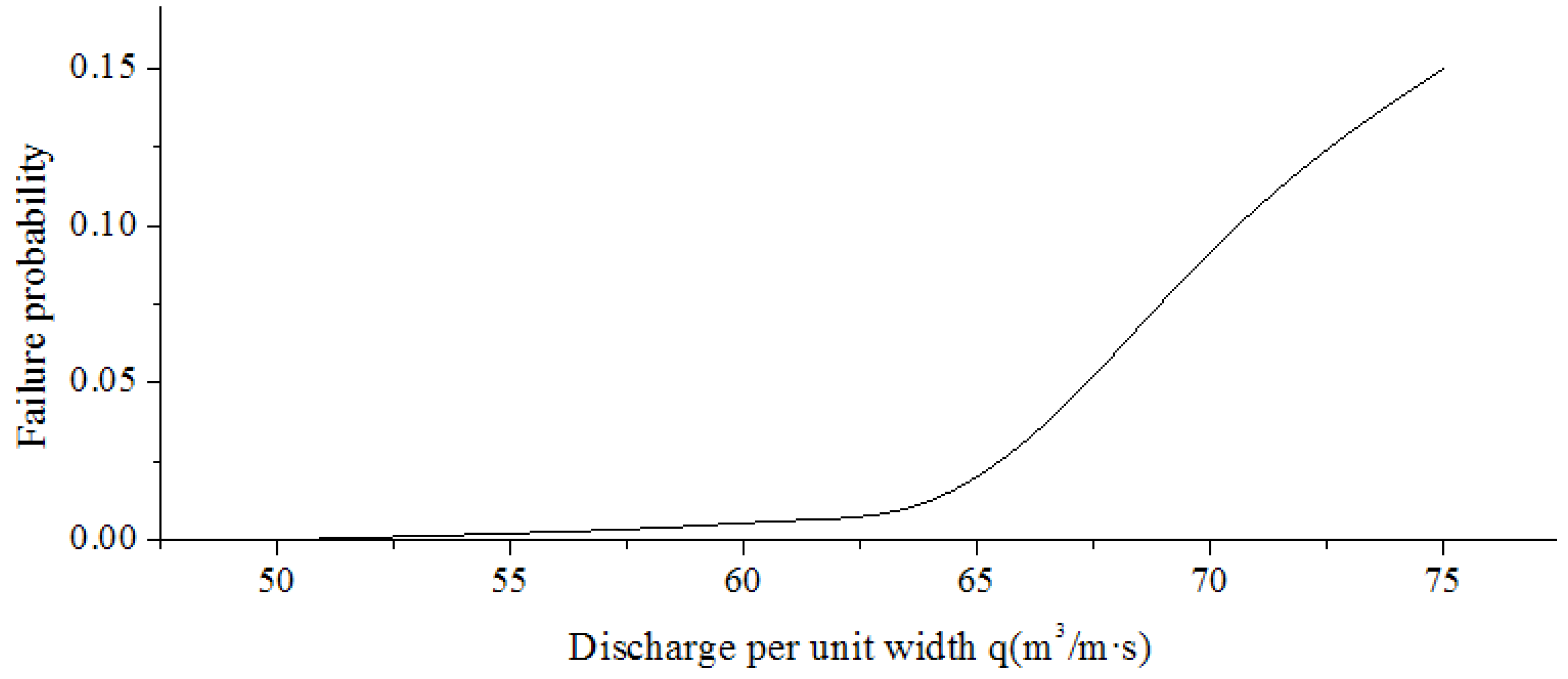
Relationship between failure probability and discharge per unit width.
Figure 3.
Relationship between failure probability and discharge per unit width.
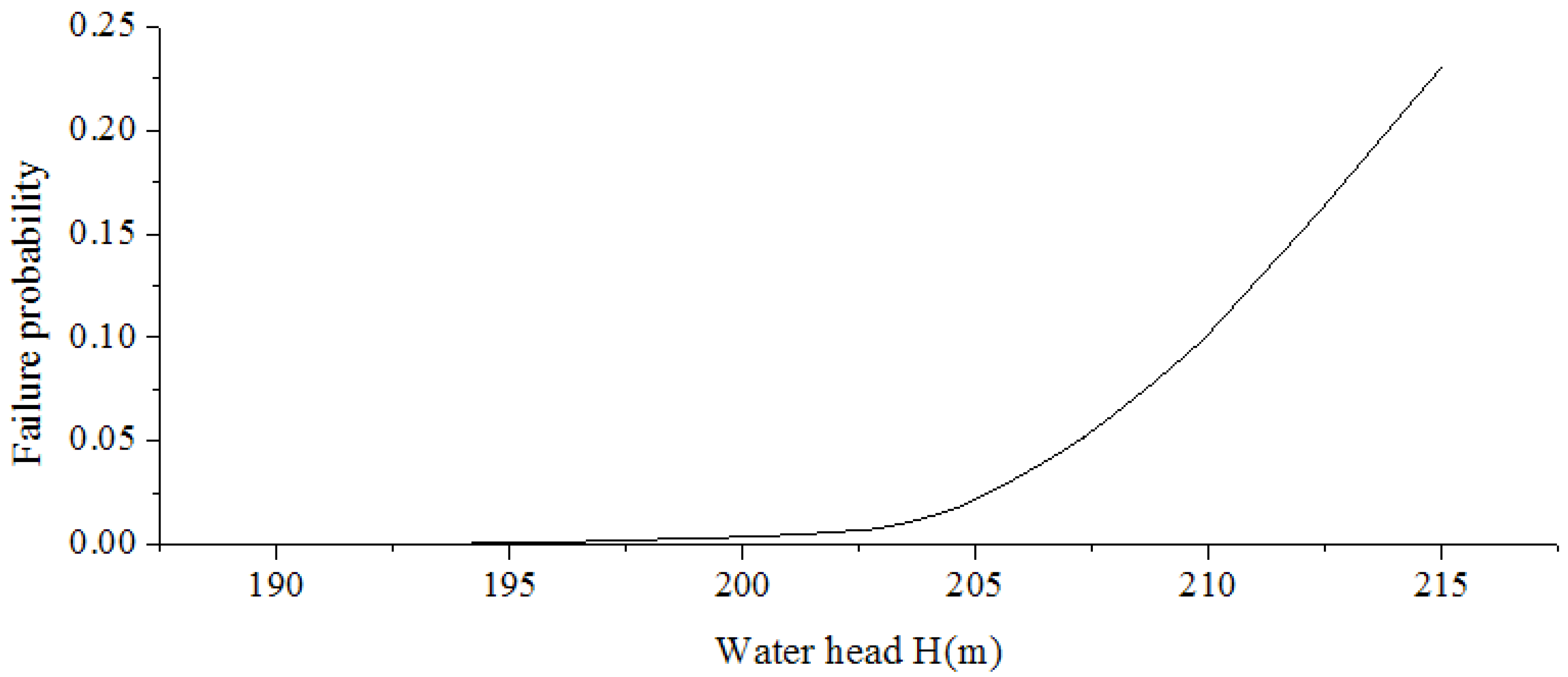
Figure 4.
Relationship between failure probability and water head.
Figure 4.
Relationship between failure probability and water head.
6. Conclusions
In this study, a probabilistic model in order to assess the risk of dam foundation failure due to scour action is presented. Uncertainty in the hydraulic variables involved in plunge pools, such as discharge, flow depth, is explicitly considered. Monte Carlo simulation (MCS) is applied to generate a large number of random sample values according to their respective probabilistic distributions. A limit state function has been formulated for the dam foundation safety under jet action. The probability density function (PDF) of the performance function is calculated based on maximum-entropy method (MEM).As an illustration of the developed methodology, a jet scour case example is analyzed. The example demonstrates how the reliability method can be used to evaluate the risk of dam foundations subjected to scour action.