Impact of Exclusive Choice Policies on Platform Supply Chains: When Both Same-Side and Cross-Side Network Effects Exist
Abstract
1. Introduction
2. Literature Review
3. Model Construction and Analysis
3.1. Enforced “Choose-One-of-Two” Scenario (MS)
- (1)
- , , , , ;
- (2)
- , , , , , ;
- (3)
- , , ; .
- (1)
- , , , ;
- (2)
3.2. Without “Choose-One-of-Two” Enforcement Scenario (MS)
- (1)
- When , We can obtain: , , , , ;
- (2)
- , , , ;
- (3)
- , , , , ;
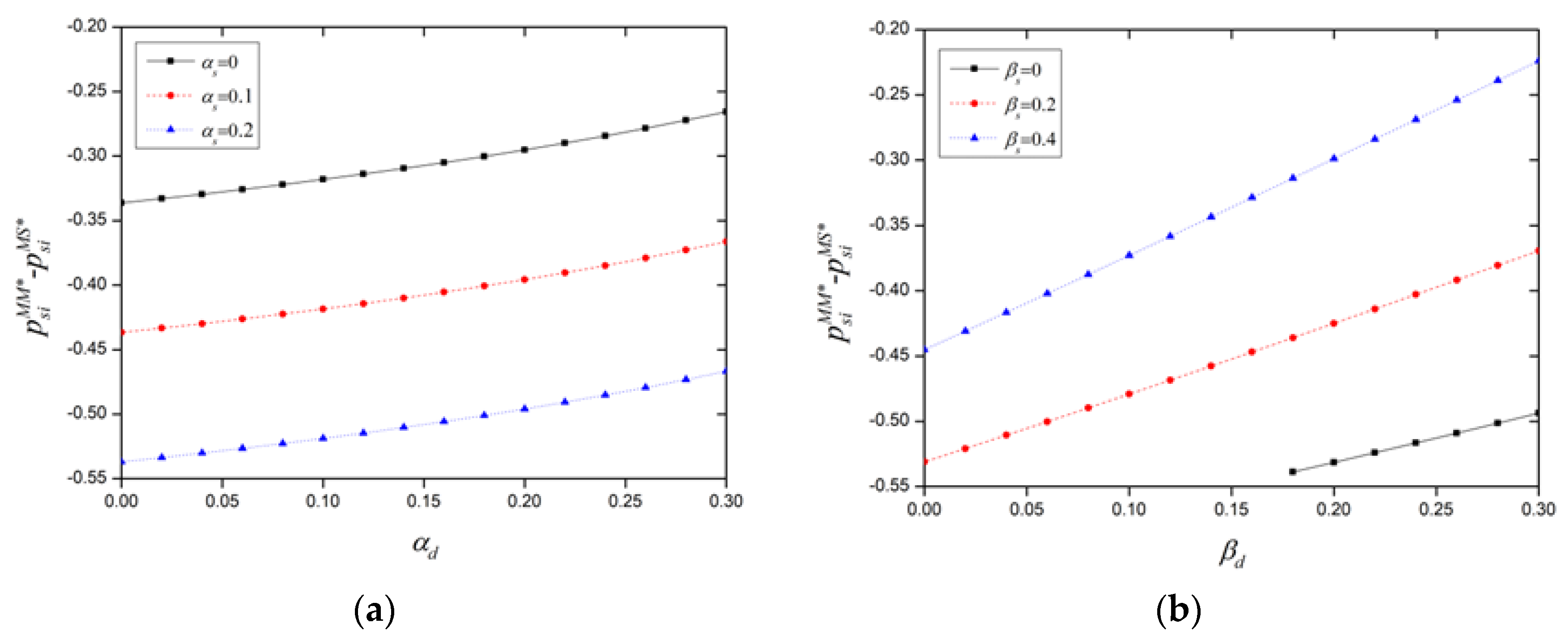
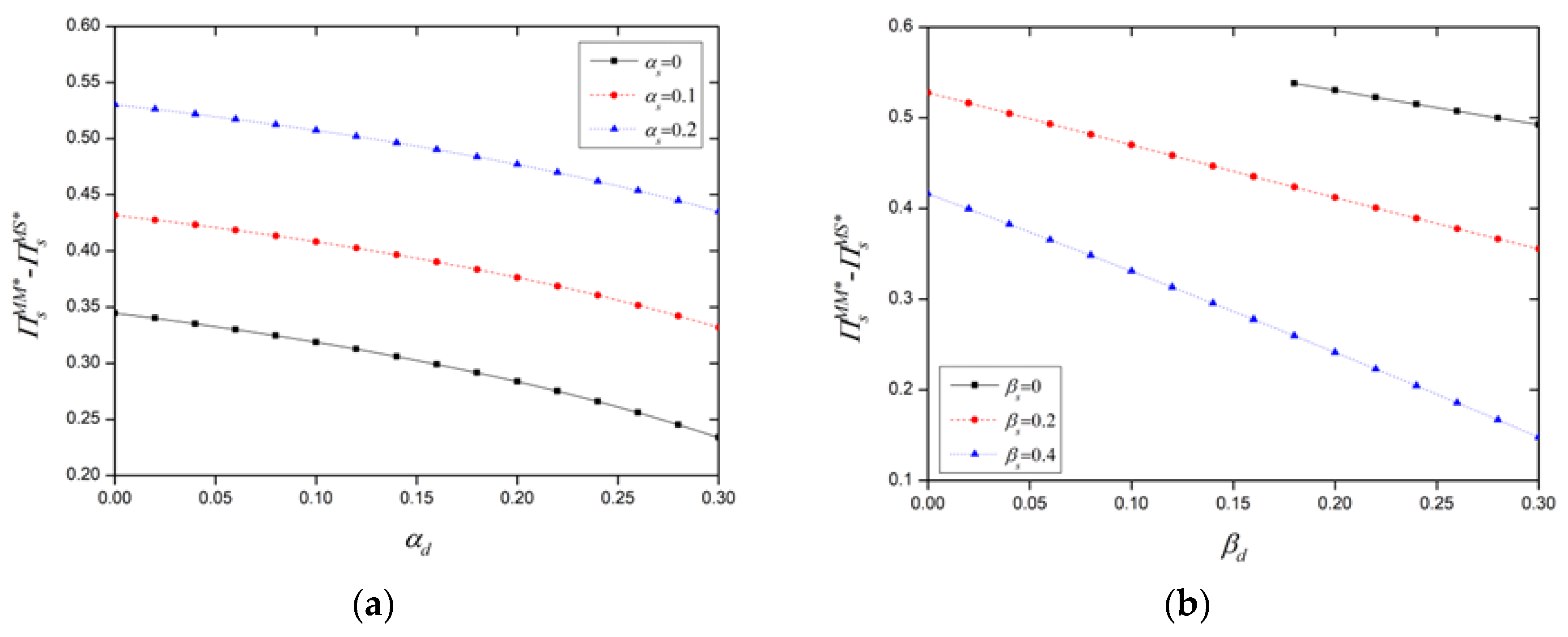
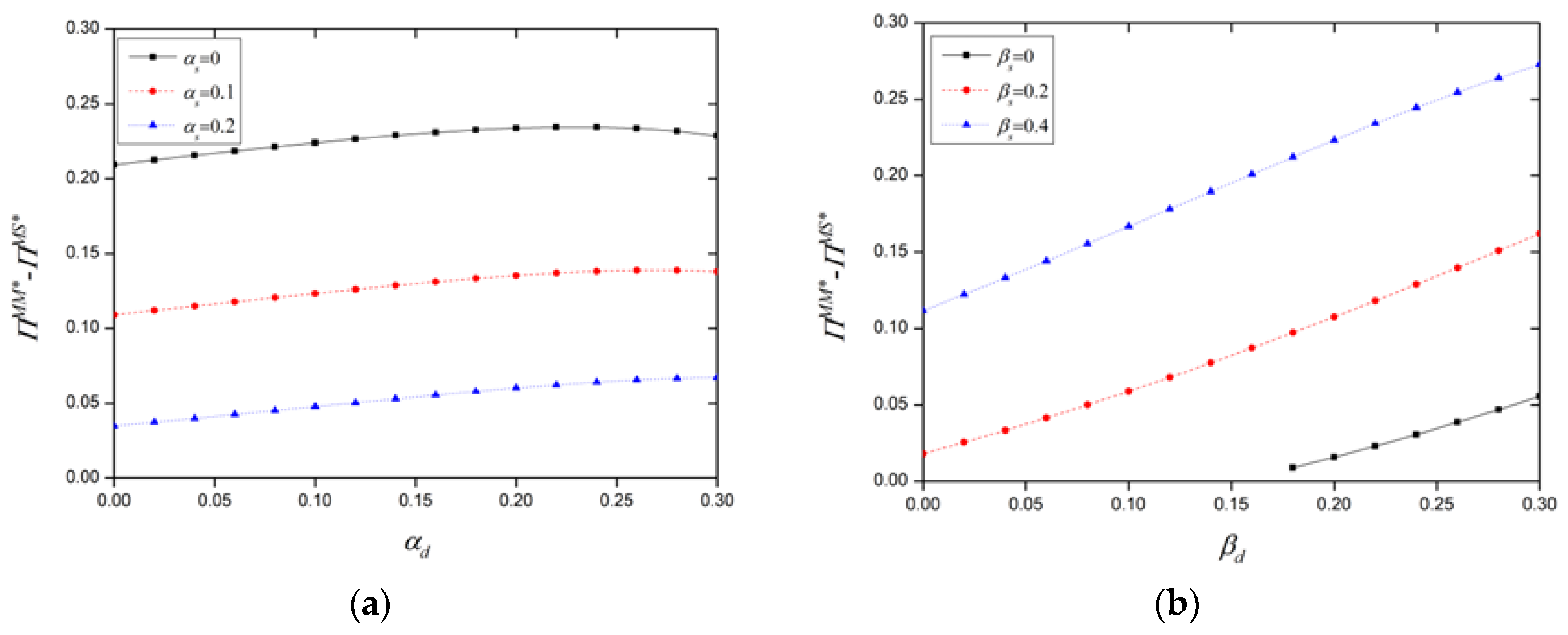
4. Numerical Analysis
5. Conclusions
- Impact of “choose-one-of-two” strategy: When platforms enforce the “choose-one-of-two” strategy, positive network effects between users lead to more multi-homing on the demand side, reducing platform revenue. This aligns with Armstrong’s [17] competitive bottleneck model and the findings of Xie et al. [6]. However, our study emphasizes that intensified negative network effects compel platforms to raise prices for supply-side users to increase revenue, which reduces welfare for demand-side users—an aspect not covered in other literature.
- Post-antitrust scenario: In the absence of the “choose-one-of-two” strategy, similar to the MH-MH scenario in Xie et al. [6], we compared the changes for platform supply chain participants before and after antitrust interventions. We found that when the same-side network strength varies for both user sides, the platform typically increases prices for one side while decreasing prices for the other. More multi-homing supply-side users attract more multi-homing demand-side users. The impact of bilateral network effects on platform revenue differs before and after enforcing the “choose-one-of-two” policy.
- Equilibrium pricing without “choose-one-of-two”: Without the “choose-one-of-two” requirement, the platform’s equilibrium pricing for demand-side users remains largely unaffected, while prices for supply-side users decrease. The smaller the positive network strength between user sides, the more intense the competition among supply-side users, leading to greater price reductions for them. Platform revenue does not necessarily decrease without the “choose-one-of-two” strategy. When positive network effects between users are strong enough, platform revenue may increase. Additionally, without “choose-one-of-two”, both supply-side user and platform supply chain total revenues increase. The weaker the positive network effects or the more intense the competition among supply-side users, the greater the revenue increase for supply-side users and the smaller the increase in total supply chain revenue.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Hagiu, A.; Wright, J. Marketplace or reseller? Manag. Sci. 2015, 61, 184–203. [Google Scholar] [CrossRef]
- Evans, D.S. Some empirical aspects of multi-sided platform industries. Rev. Netw. Econ. 2003, 2, 191–209. [Google Scholar] [CrossRef]
- Eisenmann, T.R.; Parker, G.A.; Van Alstyne, M. Strategies for Two-Sided Markets. Harv. Bus. Rev. 2006, 84, 92–101+149. [Google Scholar]
- Thies, F.; Wessel, M.; Benlian, A. Network effects on crowdfunding platforms: Exploring the implications of relaxing input control. ISJ Inf. Syst. J. 2018, 28, 1239–1262. [Google Scholar] [CrossRef]
- Takigawa, T. What Should We Do about E-Commerce Platform Giants?—The Antitrust and Regulatory Approaches in the US, EU, China, and Japan. Social Science Research Network, 1 January 2022. [Google Scholar] [CrossRef]
- Xie, J.; Zhu, W.; Wei, L.; Liang, L. Platform competition with partial multi-homing: When both same-side and cross-side network effects exist. Int. J. Prod. Econ. 2021, 233, 108016. [Google Scholar] [CrossRef]
- Katz, M.L. Network externalities, competition, and compatibility. Am. Econ. Rev. 1985, 75, 424–440. [Google Scholar]
- Caillaud, B.; Jullien, B. Chicken & Egg: Competition among Intermediation Service Providers. RAND J. Econ. 2003, 34, 309. [Google Scholar] [CrossRef]
- Parker, G.; Van Alstyne, M.W. Two-Sided Network Effects: A Theory of Information Product design. Manag. Sci. 2005, 51, 1494–1504. [Google Scholar] [CrossRef]
- Zhu, F.; Iansiti, M. Entry into platform-based markets. Strateg. Manag. J. 2011, 33, 88–106. [Google Scholar] [CrossRef]
- Anderson, E.G.; Parker, G.; Tan, B. Platform performance investment in the presence of network externalities. Inf. Syst. Res. 2014, 25, 152–172. [Google Scholar] [CrossRef]
- Sui, R.; Zhang, X.; Dan, B.; Zhang, H.; Liu, Y. Bilateral value-added service investment in platform competition with cross-side network effects under multihoming. Eur. J. Oper. Res. 2023, 304, 952–963. [Google Scholar] [CrossRef]
- Reisinger, M.; Ressner, L.; Schmidtke, R. Two-Sided markets with pecuniary and participation externalities. J. Ind. Econ. 2009, 57, 32–57. [Google Scholar] [CrossRef]
- Rochet, J.C.; Tirole, J. Two-sided markets: A progress report. RAND J. Econ. 2006, 37, 645–667. [Google Scholar] [CrossRef]
- Rochet, J.C.; Tirole, J. Platform competition in Two-Sided markets. J. Eur. Econ. Assoc. 2003, 1, 990–1029. [Google Scholar] [CrossRef]
- Rochet, J.C.; Tirole, J. Tying in two-sided markets and the honor all cards rule. Int. J. Ind. Organ. 2008, 26, 1333–1347. [Google Scholar] [CrossRef]
- Armstrong, M. Competition in two-sided markets. RAND J. Econ. 2006, 37, 668–691. [Google Scholar] [CrossRef]
- Belleflamme, P.; Peitz, M. Platform competition: Who benefits from multihoming? Int. J. Ind. Organ. 2019, 64, 1–26. [Google Scholar] [CrossRef]
- Bryan, K.; Gans, J.S. A theory of multihoming in rideshare competition. J. Econ. Manag. Strategy 2019, 28, 89–96. [Google Scholar] [CrossRef]
- Bernstein, F.; DeCroix, G.A.; Keskin, N.B. Competition between Two-Sided platforms under demand and supply congestion effects. Manuf. Serv. Oper. Manag. 2021, 23, 1043–1061. [Google Scholar] [CrossRef]
- Bakos, Y.; Hałaburda, H. Platform Competition with Multihoming on Both Sides: Subsidize or Not? Manag. Sci. 2020, 66, 5599–5607. [Google Scholar] [CrossRef]
- Liu, B.; Zhao, X.; Gu, Q. Pricing strategy and platform competition with partial multi-homing agents: When the aggregation platform exists in ride-sharing market. Transp. Res. Part E Logist. Transp. Rev. 2024, 184, 103483. [Google Scholar] [CrossRef]
- Armstrong, M.; Wright, J. Two-sided markets, competitive bottlenecks and exclusive contracts. Econ. Theory 2006, 32, 353–380. [Google Scholar] [CrossRef]
- Chellappa, R.K.; Mukherjee, R. Platform Preannouncement Strategies: The Strategic Role of Information in Two-Sided Markets Competition. Manag. Sci. 2021, 67, 1527–1545. [Google Scholar] [CrossRef]
- Ji, H.; Wang, X. Competition model of two-sided markets with platform differentiation and users partially multihoming. Syst. Eng.–Theory Pract. 2014, 34, 1398–1406. [Google Scholar]
- Jung, D.; Kim, B.C.; Park, M.; Straub, D.W. Innovation and Policy Support for Two-Sided Market Platforms: Can Government Policy Makers and Executives Optimize Both Societal Value and Profits? Inf. Syst. Res. 2019, 30, 1037–1050. [Google Scholar] [CrossRef]
- Dou, G.; Lin, X.; Xu, X. Value-added service investment strategy of a two-sided platform with the negative intra-group network externality. Kybernetes 2018, 47, 937–956. [Google Scholar] [CrossRef]
- Kurucu, G. Negative intra group network externalities in a monopolistic Two-Sided market. Rev. Netw. Econ. 2018, 17, 51–73. [Google Scholar] [CrossRef]
- Bedre-Defolie, Ö.; Biglaiser, G. Competition for exclusivity of a superior input and quality implications. Social Science Research Network, 1 January 2022. [Google Scholar] [CrossRef]
- Hagiu, A.; Lee, R.S. Exclusivity and control. J. Econ. Manag. Strategy 2011, 20, 679–708. [Google Scholar] [CrossRef]
- Carroni, E.; Madio, L.; Shekhar, S. Superstar Exclusivity in Two-Sided Markets. Manag. Sci. 2024, 70, 991–1011. [Google Scholar] [CrossRef]
- Shah, N.H.; Chaudhari, U.; Jani, M.Y. Optimal policies for time-varying deteriorating item with preservation technology under selling price and trade credit dependent quadratic demand in a supply chain. Int. J. Appl. Comput. Math. 2017, 3, 363–379. [Google Scholar] [CrossRef]
- Shah, N.H.; Chaudhari, U.; Jani, M.Y. Optimal down–stream credit period and replenishment time for deteriorating inventory in a supply chain. J. Basic Appl. Res. Int. 2015, 14, 101–115. [Google Scholar]
- Shah, N.H.; Chaudhari, U.; Jani, M.Y. Inventory control policies for substitutable deteriorating items under quadratic demand. Oper. Supply Chain. Manag. Int. J. 2019, 12, 42–48. [Google Scholar] [CrossRef]





| Negative Same-Side Network Effects (Supply Side) | Positive Same-Side Network Effects (Demand Side) | Positive Cross-Side Network Effects | Both Sides Are Multi-Homing | One Side Is Multi-Homing and Another Side Is Single Homing | |
|---|---|---|---|---|---|
| Armstrong [17]; Armstrong and Wright [23]; Belleflamme and Peitz [18]; Liu et al. [22] | ✓ | ✓ | |||
| Chellappa and Mukherjee [24] | ✓ | ✓ | ✓ | ||
| Bakos and Halaburda [21] | ✓ | ✓ | ✓ | ||
| Ji and Wang [25]; Jung et al. [26] | ✓ | ✓ | |||
| Dou et al. [27]; Kurucu [28] | ✓ | ✓ | |||
| Bernstein et al. [20] | ✓ | ✓ | ✓ | ✓ | |
| Xie et al. [6] | ✓ | ✓ | ✓ | ✓ | |
| Our study | ✓ | ✓ | ✓ | ✓ | ✓ |
| Market Share | Δ (MM − MS) |
|---|---|
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, H.; Xu, Q. Impact of Exclusive Choice Policies on Platform Supply Chains: When Both Same-Side and Cross-Side Network Effects Exist. J. Theor. Appl. Electron. Commer. Res. 2024, 19, 1185-1205. https://doi.org/10.3390/jtaer19020061
Chen H, Xu Q. Impact of Exclusive Choice Policies on Platform Supply Chains: When Both Same-Side and Cross-Side Network Effects Exist. Journal of Theoretical and Applied Electronic Commerce Research. 2024; 19(2):1185-1205. https://doi.org/10.3390/jtaer19020061
Chicago/Turabian StyleChen, Haijun, and Qi Xu. 2024. "Impact of Exclusive Choice Policies on Platform Supply Chains: When Both Same-Side and Cross-Side Network Effects Exist" Journal of Theoretical and Applied Electronic Commerce Research 19, no. 2: 1185-1205. https://doi.org/10.3390/jtaer19020061
APA StyleChen, H., & Xu, Q. (2024). Impact of Exclusive Choice Policies on Platform Supply Chains: When Both Same-Side and Cross-Side Network Effects Exist. Journal of Theoretical and Applied Electronic Commerce Research, 19(2), 1185-1205. https://doi.org/10.3390/jtaer19020061






