Next Article in Journal
Swearing in Sport and Exercise: Development and Validation of a New Questionnaire
Previous Article in Journal
Mental Health and Immigrant Perceptions of Inclusion
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Psychology International
Volume 7
Issue 2
10.3390/psycholint7020036
psycholint-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Relation Between Mathematics Self-Efficacy, Mathematics Anxiety, Behavioural Engagement, and Mathematics Achievement in Japan

by
Yuno Shimizu
Faculty of Letters, Kokushikan University, 4-28-1 Setagaya, Setagaya-ku, Tokyo 154-8515, Japan
Psychol. Int. 2025, 7(2), 36; https://doi.org/10.3390/psycholint7020036
Submission received: 7 March 2025 / Revised: 17 April 2025 / Accepted: 22 April 2025 / Published: 29 April 2025
(This article belongs to the Section Cognitive Psychology)
Browse Figure
Versions Notes

Abstract

:
Enhancing mathematical achievement has been identified as a pivotal issue in school education, extending beyond mathematics education alone. However, research comprehensively examining the relationship between multiple affective variables and learning and mathematics achievement is limited. The present study examines the relationship between self-efficacy, mathematics anxiety, behavioural engagement, and mathematics achievement among students in Japan. Moreover, this study examines whether there are any differences in this relationship according to gender and socio-economic status (SES). A path analysis using the data from students in Japan (n = 5760) in the PISA 2022 dataset revealed that (1) self-efficacy for formal and applied mathematics was significantly negatively related to mathematics anxiety and significantly positively related to behavioural engagement and mathematics achievement, (2) self-efficacy for mathematical reasoning and 21st-century mathematics was found to be significantly negatively associated with mathematics anxiety and positively associated with behavioural engagement, and (3) while a negative correlation was observed between mathematics anxiety and behavioural engagement, a significant relationship was not identified between the two and mathematics achievement. Furthermore, the multiple-group structural equation modelling, with gender and SES as the grouping variable, demonstrated no differences in gender and SES in the relationship between self-efficacy, mathematics anxiety, behavioural engagement, and math achievement.

1. Introduction

Mathematics is considered a core discipline because it is the foundation for understanding and describing a wide range of phenomena in nature and society and involved in a range of sciences as a study of universal structures. Students’ achievement in mathematics not only predicts academic success (Watts et al., 2014) but also future outcomes such as career choice (Wang et al., 2015) and the understanding of risk and decision-making (Reyna et al., 2009). Consequently, enhancing mathematical achievement has been identified as a pivotal issue in school education, extending beyond the domain of mathematics education alone (Ministry of Education, Culture, Sports, Science, and Technology in Japan, 2018; National Council of Teachers of Mathematics, 2000; Victorian Curriculum and Assessment Authority, n.d.).
Unfortunately, a considerable number of students worldwide encounter challenges in achieving success in the field of mathematics. In the Programme for International Student Assessment (PISA) in the 2022 assessment, initiated by the Organisation for Economic Co-operation and Development (OECD), approximately one-quarter of all students failed to attain the lowest level of mathematical literacy (Level 2). Moreover, a mere 2.0% of students achieved mathematical literacy (Level 6), which demands advanced mathematical thinking and reasoning skills (OECD, 2023). This level is difficult to attain across all countries and is intended to reflect the highest levels of cognitive demand in PISA assessments. Even in high-performing regions such as Hong Kong (China), Macau (China), Singapore, and Chinese Taipei, only around 10% of students reach this level (OECD, 2023). In Japan, while 12% of students did not reach Level 2, only 6.8% of students reached Level 6 (OECD, 2023). Given that even in the most proficient educational systems, the proportion of students reaching Level 6 remains low, this level of achievement should be regarded as exceptional rather than normative. Although such advanced competencies may not be essential for routine tasks encountered in everyday life, they are increasingly critical in STEM fields and decision-making contexts. Accordingly, understanding how students develop such skills—and the affective factors that influence this development and improvement—can inform strategies to better support those with the potential to attain high-level mathematical reasoning.
Research on mathematics achievement has historically focused on affective aspects such as attitudes, beliefs, and motivation. This is due to the recognition that demographic variables (i.e., SES, gender) and cognitive processes (i.e., working memory) alone cannot fully account for achievement differences (Batchelor et al., 2019; Hannula, 2015, 2020). Cross-sectional and longitudinal studies have revealed that self-efficacy and self-concept, as well as interest, can be identified as positive predictors of mathematics achievement, while mathematics anxiety is a negative predictor of mathematics achievement (Arens et al., 2022; Du et al., 2021; Lee et al., 2014; Ma & Xu, 2004; Pajares & Graham, 1999; Shimizu, 2025). Consequently, enhancing students’ affect towards mathematics has emerged as a subject of international concern, not only in Japan (Ministry of Education, Culture, Sports, Science, and Technology in Japan, 2018; OECD, 2023).
However, research comprehensively examining the relationship between multiple affective variables and learning and mathematics achievement is limited (Du et al., 2021; Gjicali & Lipnevich, 2021). Examining the relationship between single variables and mathematics achievement is inadequate in itself, as it may lead to an overestimation of the contribution of a single variable, and there is a possibility of spurious correlation. Furthermore, empirical investigations examining the relationship between affect and the learning of mathematics, as well as achievement in mathematics, using representative data within the Japanese educational context remain limited. In order to enhance achievement in mathematics, a matter of concern in Japan and globally, it is imperative to elucidate the intricate mechanisms and pathways to achievement. The present study uses a large survey dataset in Japan to examine the relationship between self-efficacy, mathematics anxiety, behavioural engagement, and mathematics achievement among students in Japan.

2. Materials and Methods

2.1. Literature Review

2.1.1. The Relationships Between Self-Efficacy, Mathematics Anxiety, Behavioural Engagement, and Mathematics Achievement

Self-efficacy is defined as a student’s self-perception of successfully completing a given task in a specific domain. In Bandura’s (1986) social cognitive theory, self-efficacy is a task-specific construct that triggers human behaviour. To clarify this standpoint, a student’s self-efficacy in mathematics does not imply the existence of mathematical ability. Instead, it signifies the student’s confidence in their ability to succeed in a particular mathematics task (Hackett & Betz, 1989). For example, students may feel highly efficacious when solving routine algebraic equations but less confident when engaging in open-ended tasks such as mathematical modelling or real-world data analysis. This specificity of self-efficacy underscores the importance of differentiating between types of mathematical tasks, such as formal/applied mathematics and mathematical reasoning/21st-century mathematics.
Numerous cross-sectional and longitudinal studies have demonstrated a strong positive association between mathematics self-efficacy and mathematics achievement (Arens et al., 2022; Azar et al., 2010; Jiang et al., 2014; OECD, 2014; Özcan & Eren Gümüş, 2019; Pajares & Graham, 1999; Shimizu, 2022, 2025). According to PISA 2012, 15-year-olds with high mathematics self-efficacy for formal and applied mathematics (e.g., solving an equation like 3x + 5 = 17, calculating how much more expensive a computer would be after adding tax) tend to have high mathematics literacy, and self-efficacy explained 29% of the variance in mathematics literacy (OECD, 2014). In PISA 2022, mathematics self-efficacy for mathematical reasoning and 21st-century mathematics, which includes the interpretation and analysis of mathematical data, real-world application, statistical reasoning, mathematical modelling, computational and technological literacy, and geometry and measurement, tend to be related to high mathematics literacy. Furthermore, the association between mathematics self-efficacy and mathematics achievement is significant and positive, even when controlling for self-concept and past academic performance (Arens et al., 2022; Pajares & Graham, 1999). Consequently, mathematics self-efficacy can be regarded as a significant positive predictor of mathematics achievement.
Mathematics anxiety is defined as tension and anxiety that interfere with number operations and mathematical problem-solving in various situations, such as daily life and academic contexts (Ramirez et al., 2018). While there are similarities between general anxiety and test anxiety, the small contribution of genetic and non-shared environmental factors in common with these (Wang et al., 2015), as well as the difference in the correlation pattern with external reference variables (Hembree, 1990), mean that mathematics anxiety is an emotion specific to mathematics. As conceptual definitions would also suggest, it is a robust finding that mathematics anxiety is negatively associated with mathematics achievement (Ahmed et al., 2012; Barroso et al., 2021; Gunderson et al., 2018; Ma, 1999; Ma & Xu, 2004; OECD, 2023; Zhang et al., 2019). In PISA 2022, international differences in the mathematics anxiety index account for approximately 25% of the variance in mathematical literacy (OECD, 2023). Meta-analyses demonstrate a small to moderate negative correlation between mathematics anxiety and mathematics achievement (r = −0.28, Barroso et al., 2021; r = −0.27, Ma, 1999; r = −0.32, Zhang et al., 2019). Consequently, mathematics anxiety can be conceptualised as a significant negative predictor of achievement in mathematics.
Behavioural engagement is the degree to which students participate in, attend to, and exert effort and perseverance in school, classroom, and academic activities (Fredricks, 2011). Engagement also has emotional and cognitive aspects (Fredricks, 2011). Emotional engagement is defined as the degree to which students experience positive emotions, such as interest and enjoyment, in the context of learning. Cognitive engagement refers to the degree to which students participate cognitively by, for example, planning and monitoring their activities and using cognitive learning strategies. It has been proposed that behavioural engagement is a necessary condition for achievement (Christenson et al., 2012). This assertion is predicated on the premise that students who demonstrate high school attendance and active participation in lessons are better positioned to receive instruction, extend and deepen their learning, and receive feedback. Numerous studies have demonstrated a direct and positive association between behavioural engagement and mathematics achievement (Fung et al., 2018; Gjicali & Lipnevich, 2021; Putwain et al., 2018). Indeed, cross-sectional and longitudinal studies have shown that behavioural engagement is directly and positively associated with mathematics achievement. Consequently, the present study has concentrated exclusively on behavioural engagement as a significant positive predictor of mathematics achievement.

2.1.2. The Relationships Between Self-Efficacy, Mathematics Anxiety, and Behavioural Engagement

The control–value theory (Pekrun, 2006; Pekrun & Linnenbrink-Garcia, 2012) comprehensively accounts for the relationship between self-efficacy, mathematics anxiety, and behavioural engagement. According to this theory, achievement emotions such as mathematics anxiety are thought to determine engagement and achievement. The theory further posits that achievement emotions are determined by self-efficacy and cause attribution for the activity and its results, as well as by appraisal, which comprises values such as task value. In summary, the theory posits that self-efficacy influences mathematics anxiety, which, in turn, influences behavioural engagement.
As theorised, cross-sectional and longitudinal studies have also demonstrated that self-efficacy is negatively associated with mathematics anxiety (Du et al., 2021; Pérez-Fuentes et al., 2020; Shimizu, 2025). For instance, Du et al. (2021) conducted a longitudinal survey of Chinese elementary school students. They demonstrated that prior mathematics self-efficacy negatively predicted mathematics anxiety even when controlling for interest and achievement in mathematics. In a cross-sectional survey of Japanese high school students, Shimizu (2025) demonstrated that self-efficacy exhibited a significant negative correlation with mathematics anxiety. A meta-analysis (Q. Li et al., 2021) has shown that self-efficacy is moderately negatively correlated with mathematics anxiety (r = −0.42).
Despite the limited number of previous studies, mathematics anxiety is negatively associated with behavioural engagement. Quintero et al. (2022) conducted a cross-sectional survey of American elementary school students and demonstrated that mathematics anxiety is negatively associated with behavioural engagement. Furthermore, Hembree’s (1990) meta-analysis demonstrates that students with elevated levels of mathematics anxiety are more prone to engage in behaviours that evade math, such as enrolling in fewer high school math courses (r = −0.31) and selecting colleges that are not related to math (r = −0.32).

2.1.3. Considerations of Gender in Mathematics-Related Constructs

Given the existence of stereotypes such as ‘mathematics is a male domain’ and ‘women are not good at mathematics’ (e.g., Fennema & Sherman, 1977; Furnham et al., 2002), it is evident that mathematics is a field characterised by strong gender stereotypes. Consequently, when investigating concepts related to mathematics, it is imperative to consider gender as a variable (Arens et al., 2022). Research indicates that males typically exhibit higher levels of self-efficacy (Borgonovi & Pokropek, 2019; Ferla et al., 2009) and higher achievement in mathematics from high school onwards (Hembree, 1992; Keller et al., 2022). Conversely, they demonstrate reduced levels of mathematics anxiety (Hembree, 1990; Keller et al., 2022) and engagement (Keller et al., 2022). However, many of the gender differences identified in previous studies were at a very small or small level. This is consistent with the gender similarities hypothesis (Hyde, 2005; Zell et al., 2015), which posits that gender differences in psychological constructs are typically negligible or small in magnitude.
No gender differences have been observed in the relationships between the variables. Concerning self-efficacy, Arens et al. (2022) conducted a longitudinal study of German students during their secondary education, which demonstrated that there were no differences in the relationship with mathematics achievement between males and females. Moreover, several meta-analyses have demonstrated no gender disparity in the relationship between mathematics anxiety and mathematics achievement (Barroso et al., 2021; Caviola et al., 2022).
However, the relationship between behavioural engagement and mathematics achievement has not been examined in terms of gender differences. To the best of my knowledge, no studies have examined gender differences in the relationship between variables within a sample of students in Japan as the research subjects.
Understanding gender differences in the structural relationships and mean levels of self-efficacy, mathematics anxiety, behavioural engagement, and mathematics achievement is not intended to reinforce stereotypes or suggest fixed group differences. Rather, such analyses hold practical significance in informing gender-sensitive educational practices and equitable policy design. For example, if the pathways to achievement function differently for subgroups, interventions to improve self-efficacy or reduce anxiety may need to be tailored accordingly. Thus, analysing gender differences contributes not only to theoretical understanding but also to the development of inclusive and context-sensitive educational approaches.

2.1.4. SES Differences

Socio-economic status (SES) has also been demonstrated to exert an influence on the concepts related to achievement in mathematics. SES is defined as the extent to which an individual has access to resources related to economics, society, culture, and human capital (National Center for Education Statistics, 2012). Traditionally, SES includes the parents’ educational level, occupational status, and family income. According to the cultural reproduction theory (Bourdieu & Passeron, 1977), a seminal concept in the sociology of education, students from high-status families, particularly those from families with considerable cultural capital, are more likely to be familiar with the social practices and values that are regarded as legitimate within the school environment. Consequently, these students tend to be more receptive to school life, which can manifest in disparities in academic achievement across different social classes. Empirical studies have demonstrated that students from higher SES backgrounds exhibit higher levels of self-efficacy (Zhou et al., 2020) and engagement (L. Li et al., 2022), along with higher achievement in mathematics (Nakanishi, 2015; Perry & Mcconney, 2010), while concurrently experiencing reduced mathematics anxiety (Zhou et al., 2020).
As can be seen from the above, studies show differences in the average levels of achievement in mathematics and related concepts according to SES. However, surprisingly, no studies have examined whether there are differences in the relationships between variables according to SES. Examining the relationships between self-efficacy, mathematics anxiety, behavioural engagement, and mathematics achievement according to SES is a crucial perspective regarding social justice and educational equity. Identifying a relationship in which students from disadvantaged SES backgrounds consistently demonstrate poorer mathematics achievement would necessitate implementing corrective measures within educational policy and practice.

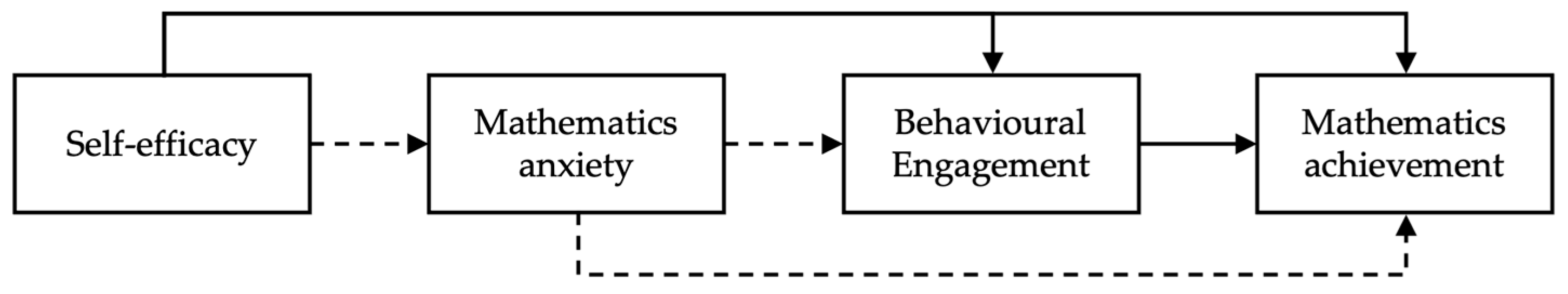
2.2. The Present Study: Aims and Hypotheses

The present study aims to examine the relationship between self-efficacy, mathematics anxiety, behavioural engagement, and mathematics achievement among students in Japan. Moreover, this study examines whether there are any differences in this relationship according to gender and SES. A comprehensive review of the extant literature informed the formulation of the following hypotheses.
Hypothesis 1.
Self-efficacy is negatively associated with mathematics anxiety and positively associated with behavioural engagement and mathematics achievement.
Hypothesis 2.
Mathematics anxiety is negatively related to behavioural engagement and mathematics achievement.
Hypothesis 3.
Behavioural engagement is positively related to mathematics achievement.
Hypothesis 4.
Males have higher self-efficacy and mathematics achievement and lower mathematics anxiety and behavioural engagement, although these gender differences are typically negligible or small in magnitude.
Hypothesis 5.
There is no gender difference in the relationship between self-efficacy, mathematics anxiety, behavioural engagement, and mathematics achievement.
Hypothesis 6.
Students with higher SES have higher self-efficacy, behavioural engagement, and mathematics achievement and lower mathematics anxiety.
Hypothesis 7a (exploratory hypothesis).
There is a difference in the relationship between self-efficacy, mathematics anxiety, behavioural engagement, and mathematics achievement according to SES.
Hypothesis 7b (exploratory hypothesis).
There is no difference in the relationship between self-efficacy and mathematics anxiety, behavioural engagement, and mathematics achievement according to SES.
The aforementioned control-value theory does not assume a direct path from self-efficacy to behavioural engagement and mathematical achievement or from mathematical anxiety to mathematical achievement. However, this study assumed these paths. This assumption enabled the examination of the influence of self-efficacy on achievement in mathematics, the influence of achievement emotions other than mathematics anxiety, and the influence of mathematics anxiety on engagement and achievement in mathematics, disregarding the behavioural aspect. Furthermore, it facilitated the evaluation of the extent of the contribution of self-efficacy and mathematics anxiety to achievement in mathematics.
Figure 1 provides a synopsis of hypotheses 1 to 3.

2.3. Methods

2.3.1. Data

In this study, I used the data from students in Japan (valid responses: 182 schools [departments], 5760 students) from the PISA 2022 dataset published by the OECD. PISA is an international academic achievement survey conducted on a triennial basis for students aged 15 worldwide. In addition to measuring reading, mathematical, and scientific literacy, PISA also conducts surveys of students, parents, schools, and teachers to examine the relationship between these literacies and various characteristics of students and schools (OECD, 2023). PISA 2022 involved approximately 690,000 students representing around 29 million 15-year-olds from schools in 81 countries and economies.

2.3.2. Measures

Mathematics Self-Efficacy

In the present study, the OECD (2023) utilised the self-efficacy score for formal and applied mathematics (MATHEFF) as a self-efficacy indicator, employing a methodology based on item response theory derived from responses to the nine items of ST290 (e.g., ‘Calculating how much more expensive a computer would be after adding tax’) concerning confidence in formal and applied mathematics tasks. Additionally, the self-efficacy score for mathematical reasoning and 21st-century mathematics (MATHEF21) was utilised, which was calculated using item response theory from responses to the 10 items (e.g., ‘Extracting mathematical information from diagrams, graphs, or simulations’) of the ST291 scale for confidence in mathematical reasoning and 21st-century mathematics. Each self-efficacy item was measured on a four-point Likert scale, with ‘Not at all confident’ assigned a value of 1 and ‘Very confident’ assigned a value of 4. The self-efficacy score was standardised so that the average for OECD member countries was zero and the standard deviation was 1. A higher score indicated that the student had more self-efficacy.
Both self-efficacy scales were scaled using the partial credit model (PCM), a polytomous item response theory (IRT) model appropriate for ordered, categorical responses. The MATHEFF scale is a trend scale linked to previous PISA cycles (e.g., 2012), enabling longitudinal comparisons. In contrast, MATHEF21 was newly introduced in PISA 2022 and is not included in the PISA trend series. The scaling procedures were conducted following a within-construct matrix sampling design, and all item responses were calibrated to support cross-national comparability (OECD, 2024a).

Mathematics Anxiety

The mathematics anxiety score (ANXMAT) was utilised as an indicator of mathematics anxiety, which was calculated using item response theory from responses to the six items (e.g., ‘I often worry that it will be difficult for me in mathematics class.’) of the ST292 on mathematics anxiety (OECD, 2023). The items on mathematics anxiety were measured on a four-point Likert scale, with ‘Strongly agree’ as one and ‘Strongly disagree’ as 4. Responses were scaled using the PCM and the scale was linked to prior PISA cycles to ensure trend comparability (OECD, 2024a). The mathematics anxiety score was standardised so that the average value for OECD member countries was zero and the standard deviation was 1. A higher score indicated that the student was more anxious about mathematics.

Behavioural Engagement

As a behavioural engagement indicator, the Proactive Mathematics Study Behaviour score (MATHPERS) was utilised, which was calculated using item response theory from responses to eight items (e.g., ‘I actively participated in group discussions during mathematics class.’) of the OECD (2023) ST293 scale. The ST293 scale measures students’ perceptions of how often they engage in behaviours that indicate effort and persistence in mathematics. The proactive mathematics study behaviour items were measured on a five-point Likert scale, with ‘Never or almost never’ assigned a value of 1 and ‘All or almost all of the time’ assigned a value of 5. It should be noted that although the items were designed to measure different constructs, most of the items in the ST293 scale are consistent with the specific forms of behavioural engagement presented by Skinner (2016). Several items were reverse-coded, and PCM was used to generate scale scores (OECD, 2024a). The MATHPERS scale was standardised so that the mean value for OECD member countries was zero and the standard deviation was 1. A higher score indicated that the student was more likely to show behaviours that indicate effort and persistence in mathematics.

Mathematics Achievement

As an indicator of mathematics achievement, plausible values for mathematical literacy were used. The mathematical literacy scale was developed using IRT models. Specifically, dichotomous items were scaled using the two-parameter logistic model (2PLM), while polytomous items were scaled using the generalised partial credit model (GPCM). These models were applied within a unidimensional, multi-group IRT framework to enable reliable cross-national comparisons. The plausible values were generated based on population modelling, and the final scores were transformed into a reporting metric with a mean of 500 and a standard deviation of 100 across OECD countries (OECD, 2024a). In PISA 2022, mathematical literacy is delineated by the perspectives of ‘Cognitive Processes (formulating, employing, and interpreting/evaluating)’, ‘Content Knowledge (quantity, uncertainty and data, change and relationships, and space and shape)’ and ‘Contexts (personal, occupational, societal, and scientific)’ (OECD, 2024a).

Gender

The item ST004Q01, which enquired about students’ gender, was utilised as an indicator of gender. Participants were instructed to indicate whether they identified as female or male, with the response options delineated as ‘Female (1)’ and ‘Male (2)’ in a two-way format. In this study, it was employed as a ‘male dummy’ variable, with 0 representing female and 1 representing male.

SES

The ESCS (Index of Economic, Social and Cultural Status) score was utilised to indicate SES. The ESCS is an index calculated from data on the number of years of education, occupational status, and household possessions of a student’s parents. The ESCS score was standardised so that the mean for OECD member countries was zero and the standard deviation was 1. A higher score indicated that the student was more economically, socially, and culturally advantaged.

2.4. Analytic Strategy

The following analyses were conducted using the data. Firstly, a preliminary analysis was conducted to understand the population trend of the variables utilised in this study. Descriptive statistics (mean and standard deviation) and correlation coefficients were calculated. Secondly, to examine the hypothetical model in Figure 1, path analysis was conducted with gender and SES as control variables. In the path analysis and subsequent multiple-group analysis, mathematics achievement was designated as a latent variable with 10 plausible values, referring to Oberski (2014). The estimated values were obtained by replicating using W_FSTUWT. Thirdly, to examine gender differences in the hypothetical model in Figure 1, a multiple-group analysis was conducted with the male dummy as the grouping variable. Fourthly, in order to examine the differences in SES between the hypothetical models in Figure 1, multiple-group structural equation modelling was conducted using the ESCS score as a grouping variable based on the upper and lower 25th percentile values, with low (n = 1473; 26%), middle (n = 2832; 50%), and high (n = 1384; 24%) groups. Following the methodological approach of Shimizu (2025), this study conducted multiple-group structural equation modelling for the following four models.
  • Model 1: A model that did not impose equal constraints.
  • Model 2: A model that imposed equal constraints on the intercept.
  • Model 3: A model that imposed equal constraints on the intercept and variance.
  • Model 4: A model that imposed equal constraints on the intercept, variance, and path coefficient.
For the analysis of this study, I used the software R (ver. 4.4.1) and Rstudio (ver. 2024.09.1+394) and the packages intsvy (ver. 2.9), survey (ver. 4.4-2), and lavaan.survey (ver. 1.1.3).

3. Results

3.1. Descriptive Statistics and Correlations

Table 1 presents the descriptive statistics and correlation coefficients for the variables employed in this study. The mean values for self-efficacy and engagement were found to be negative. In contrast, the mean value for mathematics anxiety was found to be positive, so 15-year-old students in Japan tended to have low self-efficacy and high mathematics anxiety internationally. Conversely, the mean value for mathematics achievement was over 500 points, so 15-year-old students in Japan tended to have high mathematics achievement internationally. The findings of this study indicated a negative correlation between mathematics anxiety and self-efficacy, behavioural engagement, and mathematics achievement but a positive correlation between self-efficacy, behavioural engagement, and mathematics achievement.

3.2. Testing the Hypothetical Model Through Path Analysis

After implementing a series of adjustments to account for gender and SES, a path analysis was conducted on the hypothetical model depicted in Figure 1. The goodness-of-fit indices were found to be at an acceptable level (CFI = 1.00, TLI = 1.00, RMSEA = 0.01, SRMR = 0.00). The estimated values obtained from the path analysis are presented in Table 2.
The results show that when controlling for gender and SES, self-efficacy for formal and applied mathematics was significantly negatively related to mathematics anxiety and significantly positively related to behavioural engagement and mathematics achievement. Furthermore, self-efficacy for mathematical reasoning and 21st-century mathematics was found to be significantly negatively associated with mathematics anxiety and positively associated with behavioural engagement. These associations were more potent than those observed for self-efficacy for formal and applied mathematics. Furthermore, the findings indicated a significant negative relationship between mathematics anxiety and behavioural engagement. However, the relationship between self-efficacy for mathematical reasoning and 21st-century mathematics, mathematics anxiety, behavioural engagement, and mathematics achievement was not statistically significant. Furthermore, males were found to have significantly higher self-efficacy but significantly lower mathematics anxiety and behavioural engagement. Furthermore, SES was significantly positively related to self-efficacy, mathematics anxiety, behavioural engagement, and mathematics achievement.
The path model in Table 2 explained 9% of the statistical variance in self-efficacy for formal and applied mathematics, 8% of the variance in self-efficacy for mathematical reasoning and 21st-century mathematics, 19% of the variance in mathematics anxiety, 12% of the variance in behavioural engagement, and 34% of the variance in mathematics achievement.

3.3. Testing Gender Differences in the Hypothetical Model Through Multiple-Group Structural Equation Modelling

Firstly, to examine the gender differences in the variables under investigation, the descriptive statistics for each gender were calculated and a non-correspondence t-test was conducted (see Table 3). The results indicated that male students demonstrated significantly higher levels of self-efficacy and mathematics achievement and significantly lower levels of mathematics anxiety and behavioural engagement. However, based on Cohen’s (1988) guidelines, all observed effect sizes were either very small (d < 0.20) or small (0.20 ≤ d < 0.50) in magnitude.
Subsequently, to further explore the gender disparities in the hypothetical model depicted in Figure 1, multiple-group structural equation modelling was conducted, with the male dummy designated as the grouping variable. As presented in Table 4, the results revealed the information criteria (AIC and BIC) and goodness-of-fit indices (CFI, TLI, RMSEA, SRMR) for Models 1 to 4. Given that the goodness-of-fit indices for all models were satisfactory and the information criteria for Model 1 were the most optimal, the present study adopted Model 1. The results suggested gender differences in the relationships between variables in the hypothetical model, shown in Figure 1.
The results of multiple-group structural equation modelling by gender (Supplementary Table S1) and the test of the difference in path coefficients indicated that the positive association between SES and self-efficacy for mathematical reasoning and 21st-century mathematics was significantly stronger for males (male: B = 0.33, SE B = 0.04, β = 0.21, 95%CI for B = [0.25, 0.40]; female: B = 0.21, SE B = 0.03, β = 0.17, 95%CI for B = [0.16, 0.27]; z = 2.19, p < 0.05). In comparison, the positive association between SES and mathematics anxiety was significantly stronger for females (male: B = 0.11, SE B = 0.03, β = 0.07, 95%CI for B = [0.05, 0.17]; female: B = 0.19, SE B = 0.02, β = 0.13, 95%CI for B = [0.15, 0.23]; z = 2.10, p < 0.05). However, there were no significant gender differences in the associations between other variables (see Table S1).

3.4. Testing SES Differences in the Hypothetical Model Through Multiple-Group Structural Equation Modelling

In order to examine the differences in SES between the groups, descriptive statistics were calculated for each SES group (high, middle, and low) and then a variance analysis and multiple comparisons were conducted using the Bonferroni method (see Table 5). The results indicated that the SES group with the highest SES had the highest self-efficacy, behavioural engagement, and mathematics achievement, followed by the SES group with the middle SES and the SES group with the lowest SES.
Multiple-group structural equation modelling was conducted to explore further the SES disparities in the hypothetical model depicted in Figure 1, with SES groups categorised as high, medium, and low. As presented in Table 6, the results demonstrated the information criteria and goodness-of-fit indices for Models 1 to 4. It was determined that all models’ goodness-of-fit indices were satisfactory, and the information criteria for Model 1 were optimal. Consequently, this study adopted Model 4. This finding suggested no statistically significant difference in SES between the relationships between the variables in the hypothetical model in Figure 1.

4. Discussion

The present study examined the relationship between self-efficacy, mathematics anxiety, behavioural engagement, and mathematics achievement among students in Japan. Moreover, this study examined whether there are any differences in this relationship according to gender and SES. The results of the path analysis (see Table 2) demonstrated that when controlling for gender and SES, self-efficacy for formal and applied mathematics was significantly negatively related to mathematics anxiety and significantly positively related to engagement and mathematical literacy. Additionally, a negative correlation of self-efficacy with mathematics anxiety was observed in the case of mathematical reasoning and 21st-century mathematics and a positive correlation was found with engagement. Overall, this study provides support for Hypothesis 1. The results show that students’ self-efficacy with mathematical reasoning and 21st-century mathematics was significantly negatively related to mathematics anxiety and significantly positively related to engagement. While a negative correlation was observed between mathematics anxiety and behavioural engagement, a significant relationship was not identified between the two and mathematics achievement. This outcome partially corroborates Hypothesis 2 but does not support Hypothesis 3. The results show that males had significantly higher self-efficacy and mathematics achievement and significantly lower mathematics anxiety and behavioural engagement, which fully supports Hypothesis 4. The observed gender differences were either very small or small in magnitude, consistent with the gender similarities hypothesis (Hyde, 2005; Zell et al., 2015). The multiple-group structural equation modelling, with gender as the grouping variable (Table 4), revealed gender differences in the relationships between SES and self-efficacy and between SES and mathematics anxiety. However, no significant gender differences were observed in the relationships and explanatory rates between self-efficacy, mathematics anxiety, behavioural engagement, and math achievement. These findings provide support for Hypothesis 5. The findings indicated that as SES levels increased, self-efficacy, behavioural engagement, and achievement in mathematics also increased significantly, thereby largely supporting Hypothesis 6. Finally, the multiple-group structural equation modelling results with SES as the grouping variable (Table 6) showed no significant differences in the relationships and explanatory rates between self-efficacy and mathematics anxiety, behavioural engagement, and achievement in mathematics, and Hypothesis 7b was supported.
Interestingly, controlling for gender and SES revealed that self-efficacy for formal and applied mathematics was the only variable significantly positively related to mathematics achievement. In contrast, self-efficacy for mathematical reasoning and 21st-century mathematics, mathematics anxiety and behavioural engagement were not related to mathematics achievement. These outcomes appear to contradict Hypotheses 2 and 3, yet they align with Hypothesis 1, Pajares and Graham (1999), and Shimizu (2025). Pajares and Graham (1999) utilised multiple regression analysis, with self-efficacy, self-concept, mathematics anxiety, and engagement as independent variables and mathematics test scores as the dependent variable. The analysis revealed a positive relationship between self-efficacy and mathematics achievement. Furthermore, it demonstrated that the influence of self-efficacy on mathematics achievement was significant, to the point where it countered the impact of mathematics anxiety, self-concept, and engagement on mathematics achievement. Consequently, it was proposed that, even among 15-year-olds in Japan, self-efficacy for formal and applied mathematics is an affective variable that strongly predicts achievement in mathematics, but mathematics anxiety is not an affective variable that strongly predicts achievement in mathematics.
Unexpectedly, self-efficacy for mathematical reasoning and 21st-century mathematics was not significantly associated with mathematics achievement. This finding suggests a potential misalignment between students’ confidence in real-world, reasoning-based mathematical tasks and the actual evaluation standards reflected in academic assessments such as PISA in Japan. From a curriculum design perspective, this result may highlight a gap between student perceptions of competence in modern mathematical applications (e.g., problem-solving with simulations or real-life data) and the skills required to perform well in standardised mathematics assessments. One possible explanation lies in the nature of instructional practices and assessment standards in Japan. PISA 2022 results indicate that students in Japan reported comparatively lower exposure to reasoning-based mathematics tasks than the OECD average (OECD, 2024b), which may explain the disconnect between students’ self-efficacy and their achievement outcomes in this domain. This lack of opportunity to engage with such tasks may hinder students from applying their confidence in these areas to actual performance in standardised assessments, thus disrupting the expected link between self-efficacy and achievement.
The finding that there is no significant relationship between behavioural engagement and mathematics achievement contradicts Hypothesis 3. However, it may be a natural result of Japanese mathematics education. Despite students in Japan demonstrating high performance in the PISA mathematics literacy tests, which indicated mathematics achievement in this study, the test items differ from the mathematics content and problems with which students in Japan are familiar (Senuma, 2008). Consequently, even if students in Japan exhibit high levels of behavioural engagement in their mathematics learning, this may not be strongly linked to their PISA mathematical literacy.
One possible explanation for the non-significant relationship between mathematics anxiety and math achievement observed in this study may lie in cultural differences in the experience and expression of anxiety. Previous cross-cultural psychology research (e.g., Markus & Kitayama, 1991) suggests that in East Asian cultures such as Japan, emotional restraint is culturally valued, as individuals are socialised to regulate their emotions in ways that preserve interpersonal harmony. Although not specific to anxiety, such norms may lead to the internalisation of negative emotions to avoid disrupting social relationships (e.g., Tsai et al., 2006). Students in Japan may feel anxious but still engage actively in class due to a cultural emphasis on perseverance (gaman) and social harmony. As such, behavioural engagement may not be reduced even in the presence of high anxiety, which could attenuate the expected negative correlation between anxiety and performance in Japan.
As expected, the observed gender differences in the mean levels of self-efficacy, mathematics anxiety, behavioural engagement, and mathematics achievement were either negligible or small in magnitude. Furthermore, no significant gender differences were found in the relationships among these variables, nor their respective explanatory power with regard to mathematics achievement. These findings are consistent with Hypotheses 4 and 5 and align with the gender similarities hypothesis (Hyde, 2005; Zell et al., 2015) as well as the relative universality hypothesis of psychological functioning proposed in control–value theory (Pekrun, 2006).
The finding that higher SES is significantly associated with higher self-efficacy, behavioural engagement, and mathematics achievement is consistent with the results of previous empirical studies (e.g., Perry & Mcconney, 2010; Zhou et al., 2020), as well as Bourdieu and Passeron’s (1977) cultural reproduction theory. This presents a significant issue for equity in mathematics education in Japan. This study’s findings indicate that increasing self-efficacy in mathematical formulas and applications effectively improves mathematics achievement among students in Japan However, given the absence of a discrepancy in SES along the pathway from affect to mathematics achievement, a uniform intervention for many students will perpetuate the existing gap in achievement due to SES, making it challenging to reduce. In addressing the issue of equity in Japanese mathematics education, it is proposed that a uniform intervention in the self-efficacy of a large group of students in formal and applied mathematics is insufficient. It is emphasised that it is crucial to implement interventions that specifically enhance the self-efficacy of students from disadvantaged SES backgrounds.
Unlike Hypothesis 6 and prior research (e.g., Zhou et al., 2020), the results showed that higher SES was significantly and positively associated with mathematics anxiety. One possible explanation is that students from higher SES backgrounds, who often attend competitive schools or are under greater academic expectations, may experience increased pressure to perform, leading to elevated anxiety. It is also possible that such students are more aware of performance standards and more self-critical and thus more likely to report anxiety.
It is important to note that this study also demonstrated no differences in SES in the relationship between self-efficacy, mathematics anxiety, behavioural engagement, and math achievement. Previous research (e.g., Perry & Mcconney, 2010; Zhou et al., 2020) showed that SES is associated with differences in mean levels of concepts related to mathematics achievement but ignored SES differences in the pathway from affect to mathematics achievement or, in other words, the moderating effect of SES. Despite the dataset of students in Japan being the sole basis of this study and thus necessitating caution against over-generalisation, it proposes novel issues for research exploring the factors contributing to mathematics achievement. This finding suggests that although students from higher SES backgrounds may enjoy greater access to academic resources and more favourable affective profiles, the psychological mechanisms linking these variables to achievement may operate similarly across SES groups. In this sense, the current findings partially challenge the cultural reproduction theory (Bourdieu & Passeron, 1977), which implies not only differences in access to educational capital but also potential structural differences in how that capital functions. A possible interpretation is that cultural reproduction may explain mean-level disparities (i.e., who has more self-efficacy or less anxiety), while the functional relationships among psychological variables and achievement remain invariant.
Although the path model accounted for 34% of the variance in mathematics achievement, a substantial portion remained unexplained. This highlights the importance of considering other potential predictors beyond self-efficacy, anxiety, and engagement. Prior research has identified cognitive factors such as working memory (e.g., Peng et al., 2016) as well as contextual and environmental factors—including instructional quality—as important predictors of mathematics achievement (e.g., De Corte et al., 2004; Kunter et al., 2013). While PISA includes some items related to these factors, the present study focused exclusively on affective and learning variables to investigate the relationship between multiple affective variables and learning and mathematics achievement. Future research could extend the model by integrating these contextual and cognitive variables to provide a more comprehensive explanation of mathematics achievement.

4.1. Implications for Education and Practices

These findings have several implications. First, they underscore the critical role of self-efficacy in mathematics learning, suggesting that interventions aimed at enhancing students’ efficacy for formal and applied mathematics may be more effective in improving achievement than those focusing solely on reducing anxiety or increasing behavioural engagement. Self-efficacy is conceptualised as being formed from four sources: mastery experiences, verbal and social persuasion, physiological and affective states, and vicarious experiences (Bandura, 1986). In an intervention study based on these principles, Siegle and McCoach (2007) demonstrated that teacher training involving goal setting, high-quality feedback, and peer modelling can enhance students’ mathematical self-efficacy. Goal setting helps students reflect on mastery experiences, teacher feedback serves as social persuasion, and peer modelling offers various experiences.
Second, this study highlights concerns regarding educational equity. While SES did not moderate the relationships among variables, students from lower SES backgrounds exhibited significantly lower self-efficacy, behavioural engagement, and mathematics achievement. This suggests that universal interventions may inadvertently reinforce existing disparities. Therefore, to address equity in mathematics education, educators and policymakers may need to prioritise targeted support for students from disadvantaged SES backgrounds, particularly in developing self-efficacy in formal and applied mathematics.

4.2. Limitations

It is important to acknowledge the four limitations of this study. Firstly, the mathematical literacy questions used in PISA, which served as the measure of mathematical achievement in this study, are unfamiliar to students in Japan, so it is not certain to what extent the results of this study can be applied to problems that students are familiar with and have studied up to this point. Future research should investigate whether similar findings can be obtained by investigating problems that students are familiar with and have studied up to this point.
Secondly, as the subjects of this study were only 15-year-old students in Japan, it is not clear to what extent the findings of this study can be applied to elementary and junior high school students. It is recommended that subsequent research consider whether the transition from motivation to achievement in mathematics varies according to developmental stage, focusing on Japanese elementary and junior high school students.
Thirdly, as PISA 2022 is a cross-sectional survey conducted at a single point, it does not satisfy the necessary condition for a causal relationship in which the independent variable precedes the dependent variable in time. Therefore, it is impossible to refer to a strictly causal relationship. To address this limitation, future research should adopt a longitudinal panel design that follows students across multiple grade levels (e.g., from grade 7 to grade 10), collecting repeated measures of self-efficacy, mathematics anxiety, behavioural engagement, and achievement. Cross-Lagged Panel Models can be used to test reciprocal effects and temporal precedence between variables. Furthermore, Random Intercept Cross-Lagged Panel Models would allow for the separation of within-person and between-person variance, providing a more nuanced estimation of causal direction. It would also be beneficial to use developmentally sensitive measures to examine how these relationships evolve as students mature.
Fourthly, the structural equation models estimated in this study yielded near-perfect fit indices (e.g., CFI = 1.000, RMSEA = 0.01), which may appear overly ideal. However, similar fit indices have been reported in prior research using PISA datasets. For example, Ferla et al. (2009) and Sözer Boz (2025) observed comparably high CFI and low RMSEA values in SEMs examining motivational and cognitive constructs among large student samples. These high fit values are likely attributable to a combination of factors, including large sample sizes, theory-driven model structures with relatively low degrees of freedom, and the standardised nature of the PISA instruments. In this study, such fit indices were consistently observed across estimation platforms (lavaan.survey and Mplus), both of which incorporated complex survey design features using Fay’s BRR method. Nonetheless, these values should be interpreted with caution. Future research may benefit from examining alternative model specifications (e.g., nested or reduced models), incorporating multigroup comparisons, or conducting cross-validation to ensure model robustness and generalisability.

5. Conclusions

The present study examined the relationships between mathematics self-efficacy, mathematics anxiety, behavioural engagement, and mathematics achievement among students in Japan using PISA 2022 data. Moreover, this study examined whether there are any differences in this relationship according to gender and socio-economic status. The results highlight the central role of self-efficacy—particularly in formal and applied mathematics—in promoting achievement, while the anticipated direct associations of mathematics anxiety and behavioural engagement with achievement were not observed.
The study contributes to the growing body of research emphasising the importance of affective factors in academic performance and suggests that self-efficacy is a robust predictor of mathematics achievement regardless of demographic background. Future longitudinal studies are needed to further explore these pathways and inform targeted educational interventions.

Supplementary Materials

The following supporting information can be downloaded at: https://www.mdpi.com/article/10.3390/psycholint7020036/s1, Table S1: Results of the multiple group structural equation modelling by gender.

Funding

This research received no external funding.

Institutional Review Board Statement

The present study utilised data from the PISA 2022 cycle. As such, it was presumed that the OECD had obtained the necessary ethical approvals from all participants. Since this was a secondary analysis of publicly available data, further ethical approval was not applicable. This study adhered to the ethical principles of the Committee on Publication Ethics (COPE) and this study was conducted in accordance with the Declaration of Helsinki.

Informed Consent Statement

Not applicable.

Data Availability Statement

The PISA 2022 data used in this study were obtained from the OECD website: https://www.oecd.org/en/data/datasets/pisa-2022-database.html. (accessed on 7 March 2025).

Conflicts of Interest

The author declares no conflict of interest.

References

  1. Ahmed, W., Minnaert, A., Kuyper, H., & Van Der Werf, G. (2012). Reciprocal relationships between math self-concept and math anxiety. Learning and Individual Differences, 22(3), 385–389. [Google Scholar] [CrossRef]
  2. Arens, A. K., Frenzel, A. C., & Goetz, T. (2022). Self-concept and self-efficacy in math: Longitudinal interrelations and reciprocal linkages with achievement. The Journal of Experimental Education, 90(3), 615–633. [Google Scholar] [CrossRef]
  3. Azar, H. K., Lavasani, M. G., Malahmadi, E., & Amani, J. (2010). The role of self-efficacy, task value, and achievement goals in predicting learning approaches and mathematics achievement. Procedia—Social and Behavioral Sciences, 5, 942–947. [Google Scholar] [CrossRef]
  4. Bandura, A. (1986). Social foundations of thought and action: A social cognitive theory. Prentice-Hall, Inc. [Google Scholar]
  5. Barroso, C., Ganley, C. M., McGraw, A. L., Geer, E. A., Hart, S. A., & Daucourt, M. C. (2021). A meta-analysis of the relation between math anxiety and math achievement. Psychological Bulletin, 147(2), 134–168. [Google Scholar] [CrossRef] [PubMed]
  6. Batchelor, S., Torbeyns, J., & Verschaffel, L. (2019). Affect and mathematics in young children: An introduction. Educational Studies in Mathematics, 100(3), 201–209. [Google Scholar] [CrossRef]
  7. Borgonovi, F., & Pokropek, A. (2019). Seeing is believing: Task-exposure specificity and the development of mathematics self-efficacy evaluations. Journal of Educational Psychology, 111(2), 268–283. [Google Scholar] [CrossRef]
  8. Bourdieu, P., & Passeron, J. C. (1977). Reproduction in education, society and culture. Sage Publications. [Google Scholar]
  9. Caviola, S., Toffalini, E., Giofrè, D., Ruiz, J. M., Szűcs, D., & Mammarella, I. C. (2022). Math performance and academic anxiety forms, from sociodemographic to cognitive aspects: A meta-analysis on 906,311 participants. Educational Psychology Review, 34(1), 363–399. [Google Scholar] [CrossRef]
  10. Christenson, S. L., Reschly, A. L., & Wylie, C. (Eds.). (2012). Handbook of research on student engagement. Springer. [Google Scholar]
  11. Cohen, J. (1988). Statistical power analysis for the behavioral sciences (2nd ed.). Lawrence Erlbaum Associates, Publishers. [Google Scholar]
  12. De Corte, E., Verschaffel, L., & Masui, C. (2004). The CLIA-model: A framework for designing powerful learning environments for thinking and problem solving. European Journal of Psychology of Education, 19(4), 365–384. [Google Scholar] [CrossRef]
  13. Du, C., Qin, K., Wang, Y., & Xin, T. (2021). Mathematics interest, anxiety, self-efficacy and achievement: Examining reciprocal relations. Learning and Individual Differences, 91, 102060. [Google Scholar] [CrossRef]
  14. Fennema, E., & Sherman, J. (1977). Sex-related differences in mathematics achievement, spatial visualization and affective factors. American Educational Research Journal, 14(1), 51–71. [Google Scholar] [CrossRef]
  15. Ferla, J., Valcke, M., & Cai, Y. (2009). Academic self-efficacy and academic self-concept: Reconsidering structural relationships. Learning and Individual Differences, 19(4), 499–505. [Google Scholar] [CrossRef]
  16. Fredricks, J. A. (2011). Engagement in school and out-of-school contexts: A multidimensional view of engagement. Theory Into Practice, 50(4), 327–335. [Google Scholar] [CrossRef]
  17. Fung, F., Tan, C. Y., & Chen, G. (2018). Student engagement and mathematics achievement: Unraveling main and interactive effects. Psychology in the Schools, 55(7), 815–831. [Google Scholar] [CrossRef]
  18. Furnham, A., Reeves, E., & Budhani, S. (2002). Parents Think their sons are brighter than their daughters: Sex differences in parental self-estimations and estimations of their children’s multiple intelligences. The Journal of Genetic Psychology, 163(1), 24–39. [Google Scholar] [CrossRef] [PubMed]
  19. Gjicali, K., & Lipnevich, A. A. (2021). Got math attitude? (In)direct effects of student mathematics attitudes on intentions, behavioral engagement, and mathematics performance in the U.S. PISA. Contemporary Educational Psychology, 67, 102019. [Google Scholar] [CrossRef]
  20. Gunderson, E. A., Park, D., Maloney, E. A., Beilock, S. L., & Levine, S. C. (2018). Reciprocal relations among motivational frameworks, math anxiety, and math achievement in early elementary school. Journal of Cognition and Development, 19(1), 21–46. [Google Scholar] [CrossRef]
  21. Hackett, G., & Betz, N. E. (1989). An exploration of the mathematics self-efficacy/mathematics performance correspondence. Journal for Research in Mathematics Education, 20(3), 261–273. [Google Scholar] [CrossRef]
  22. Hannula, M. S. (2015). Emotions in problem solving. In S. J. Cho (Ed.), Selected regular lectures from the 12th international congress on mathematical education (pp. 269–288). Springer International Publishing. [Google Scholar]
  23. Hannula, M. S. (2020). Affect in mathematics education. In S. Lerman (Ed.), Encyclopedia of mathematics education (pp. 32–36). Springer International Publishing. [Google Scholar]
  24. Hembree, R. (1990). The nature, effects, and relief of mathematics anxiety. Journal for Research in Mathematics Education, 21(1), 33–46. [Google Scholar] [CrossRef]
  25. Hembree, R. (1992). Experiments and relational studies in problem solving: A meta-analysis. Journal for Research in Mathematics Education, 23(3), 242–273. [Google Scholar] [CrossRef]
  26. Hyde, J. S. (2005). The gender similarities hypothesis. The American Psychologist, 60(6), 581–592. [Google Scholar] [CrossRef]
  27. Jiang, Y., Song, J., Lee, M., & Bong, M. (2014). Self-efficacy and achievement goals as motivational links between perceived contexts and achievement. Educational Psychology, 34(1), 92–117. [Google Scholar] [CrossRef]
  28. Keller, L., Preckel, F., Eccles, J. S., & Brunner, M. (2022). Top-performing math students in 82 countries: An integrative data analysis of gender differences in achievement, achievement profiles, and achievement motivation. Journal of Educational Psychology, 114(5), 966–991. [Google Scholar] [CrossRef]
  29. Kunter, M., Klusmann, U., Baumert, J., Richter, D., Voss, T., & Hachfeld, A. (2013). Professional competence of teachers: Effects on instructional quality and student development. Journal of Educational Psychology, 105(3), 805–820. [Google Scholar] [CrossRef]
  30. Lee, W., Lee, M.-J., & Bong, M. (2014). Testing interest and self-efficacy as predictors of academic self-regulation and achievement. Contemporary Educational Psychology, 39(2), 86–99. [Google Scholar] [CrossRef]
  31. Li, L., Valiente, C., Eisenberg, N., Spinrad, T. L., Johns, S. K., Berger, R. H., Thompson, M. S., Southworth, J., Pina, A. A., Hernández, M. M., & Gal-Szabo, D. E. (2022). Longitudinal relations between behavioral engagement and academic achievement: The moderating roles of socio-economic status and early achievement. Journal of School Psychology, 94, 15–27. [Google Scholar] [CrossRef]
  32. Li, Q., Cho, H., Cosso, J., & Maeda, Y. (2021). Relations between students’ mathematics anxiety and motivation to learn mathematics: A meta-analysis. Educational Psychology Review, 33(3), 1017–1049. [Google Scholar] [CrossRef]
  33. Markus, H. R., & Kitayama, S. (1991). Culture and the self: Implications for cognition, emotion, and motivation. Psychological Review, 98(2), 224–253. [Google Scholar] [CrossRef]
  34. Ma, X. (1999). A meta-analysis of the relationship between anxiety toward mathematics and achievement in mathematics. Journal for Research in Mathematics Education, 30(5), 520–540. [Google Scholar] [CrossRef]
  35. Ma, X., & Xu, J. (2004). The causal ordering of mathematics anxiety and mathematics achievement: A longitudinal panel analysis. Journal of Adolescence, 27(2), 165–179. [Google Scholar] [CrossRef]
  36. Ministry of Education, Culture, Sports, Science, and Technology in Japan. (2018). The course of study for mathematics in high school; MEXT. Available online: https://www.mext.go.jp/content/20230217-mxt_kyoiku02-100002620_05.pdf (accessed on 21 April 2025).
  37. Nakanishi, H. (2015). A Study of the changing academic achievement gap through panel data. The Japanese Journal of Educational Research, 82(4), 583–593. [Google Scholar]
  38. National Center for Education Statistics. (2012). Improving the measurement of socioeconomic status for the national assessment of educational progress: A theoretical foundation; National Center for Education Statistics. Available online: https://nces.ed.gov/nationsreportcard/pdf/researchcenter/socioeconomic_factors.pdf (accessed on 7 March 2025).
  39. National Council of Teachers of Mathematics. (2000). Principles and standards for school mathematics. National Council of Teachers of Mathematics. [Google Scholar]
  40. Oberski, D. (2014). lavaan.survey: An R package for complex survey analysis of structural equation models. Journal of Statistical Software, 57(1), 1–27. [Google Scholar] [CrossRef]
  41. OECD. (2014). Ready to learn: Students’ engagement, drive and self-beliefs; programme for International Student Assessment. OECD. [Google Scholar]
  42. OECD. (2023). PISA 2022 results (volume I): The state of learning and equity in education. OECD. [Google Scholar]
  43. OECD. (2024a). PISA 2022 technical report. OECD. [Google Scholar]
  44. OECD. (2024b). PISA 2022 results (volume V): Learning strategies and attitudes for life. OECD. [Google Scholar]
  45. Özcan, Z. Ç., & Eren Gümüş, A. (2019). A modeling study to explain mathematical problem-solving performance through metacognition, self-efficacy, motivation, and anxiety. Australian Journal of Education, 63(1), 116–134. [Google Scholar] [CrossRef]
  46. Pajares, F., & Graham, L. (1999). Self-efficacy, motivation constructs, and mathematics performance of entering middle school students. Contemporary Educational Psychology, 24(2), 124–139. [Google Scholar] [CrossRef]
  47. Pekrun, R. (2006). The control-value theory of achievement emotions: Assumptions, corollaries, and implications for educational research and practice. Educational Psychology Review, 18(4), 315–341. [Google Scholar] [CrossRef]
  48. Pekrun, R., & Linnenbrink-Garcia, L. (2012). Academic emotions and student engagement. In S. L. Christenson, A. L. Reschly, & C. Wylie (Eds.), Handbook of research on student engagement (pp. 259–282). Springer. [Google Scholar]
  49. Peng, P., Namkung, J., Barnes, M., & Sun, C. (2016). A meta-analysis of mathematics and working memory: Moderating effects of working memory domain, type of mathematics skill, and sample characteristics. Journal of Educational Psychology, 108(4), 455–473. [Google Scholar] [CrossRef]
  50. Perry, L. B., & Mcconney, A. (2010). Does the SES of the school matter? An examination of socioeconomic status and student achievement using PISA 2003. Teachers College Record: The Voice of Scholarship in Education, 112(4), 1137–1162. [Google Scholar] [CrossRef]
  51. Pérez-Fuentes, M. D. C., Núñez, A., Molero, M. D. M., Gázquez, J. J., Rosário, P., & Núñez, J. C. (2020). The role of anxiety in the relationship between self-efficacy and math achievement. Psicología Educativa, 26(2), 137–143. [Google Scholar] [CrossRef]
  52. Putwain, D. W., Symes, W., Nicholson, L. J., & Becker, S. (2018). Achievement goals, behavioural engagement, and mathematics achievement: A mediational analysis. Learning and Individual Differences, 68, 12–19. [Google Scholar] [CrossRef]
  53. Quintero, M., Hasty, L., Li, T., Song, S., & Wang, Z. (2022). A multidimensional examination of math anxiety and engagement on math achievement. British Journal of Educational Psychology, 92(3), 955–973. [Google Scholar] [CrossRef]
  54. Ramirez, G., Shaw, S. T., & Maloney, E. A. (2018). Math anxiety: Past research, promising interventions, and a new interpretation framework. Educational Psychologist, 53(3), 145–164. [Google Scholar] [CrossRef]
  55. Reyna, V. F., Nelson, W. L., Han, P. K., & Dieckmann, N. F. (2009). How numeracy influences risk comprehension and medical decision making. Psychological Bulletin, 135(6), 943–973. [Google Scholar] [CrossRef] [PubMed]
  56. Senuma, H. (2008). What mathematical literacy PISA measures, and how. Journal of Science Education in Japan, 32(4), 358–365. [Google Scholar]
  57. Shimizu, Y. (2022). Learning engagement as a moderator between self-efficacy, math anxiety, problem-solving strategy, and vector problem-solving performance. Psych, 4(4), 816–832. [Google Scholar] [CrossRef]
  58. Shimizu, Y. (2025). Learning engagement as moderator between self-efficacy, math anxiety, use of diagrams, and complex plane problem-solving. Eurasia Journal of Mathematics, Science and Technology Education, 21(2), em2586. [Google Scholar] [CrossRef]
  59. Siegle, D., & McCoach, D. B. (2007). Increasing student mathematics self-efficacy through teacher training. Journal of Advanced Academics, 18(2), 278–312. [Google Scholar]
  60. Skinner, E. A. (2016). Engagement and disaffection as central to processes of motivational resilience and development. In K. R. Wentzel, & D. B. Miele (Eds.), Handbook of motivation at school (pp. 145–168). Routledge. [Google Scholar]
  61. Sözer Boz, E. (2025). Evaluating measurement invariance of students’ practices regarding online information questionnaire in PISA 2022: A comparative study using MGCFA and alignment method. Education and Information Technologies, 30(1), 1219–1237. [Google Scholar] [CrossRef]
  62. Tsai, J. L., Knutson, B., & Fung, H. H. (2006). Cultural variation in affect valuation. Journal of Personality and Social Psychology, 90(2), 288–307. [Google Scholar] [CrossRef]
  63. Victorian Curriculum and Assessment Authority. n.d. (). Victorian curriculum: Mathematics. Available online: https://victoriancurriculum.vcaa.vic.edu.au/mathematics/introduction/rationale-and-aims (accessed on 7 March 2025).
  64. Wang, M.-T., Degol, J., & Ye, F. (2015). Math achievement is important, but task values are critical, too: Examining the intellectual and motivational factors leading to gender disparities in STEM careers. Frontiers in Psychology, 6, 36. [Google Scholar] [CrossRef]
  65. Watts, T. W., Duncan, G. J., Siegler, R. S., & Davis-Kean, P. E. (2014). What’s past is prologue: Relations between early mathematics knowledge and high school achievement. Educational Researcher, 43(7), 352–360. [Google Scholar] [CrossRef]
  66. Zell, E., Krizan, Z., & Teeter, S. R. (2015). Evaluating gender similarities and differences using metasynthesis. The American Psychologist, 70(1), 10–20. [Google Scholar] [CrossRef]
  67. Zhang, J., Zhao, N., & Kong, Q. P. (2019). The relationship between math anxiety and math performance: A meta-analytic investigation. Frontiers in Psychology, 10, 1613. [Google Scholar] [CrossRef] [PubMed]
  68. Zhou, D., Du, X., Hau, K.-T., Luo, H., Feng, P., & Liu, J. (2020). Teacher-student relationship and mathematical problem-solving ability: Mediating roles of self-efficacy and mathematical anxiety. Educational Psychology, 40(4), 473–489. [Google Scholar] [CrossRef]
Psycholint 07 00036 g001
Figure 1. Hypothesised model of the current study. Solid lines assume a positive association and dashed lines assume a negative association.
Figure 1. Hypothesised model of the current study. Solid lines assume a positive association and dashed lines assume a negative association.
Psycholint 07 00036 g001
Table 1. Means, standard deviations, and Pearson correlations with confidence intervals for study variables.
Table 1. Means, standard deviations, and Pearson correlations with confidence intervals for study variables.
MSDα123456
1. MALE0.500.50――
2. SES−0.010.71―0.00
[−0.03, 0.03]		―
3. MATHEFF−0.491.230.870.14 ***
[0.11, 0.16]		0.26 ***
[0.23, 0.28]		―
4. MATHEF21−0.401.000.890.20 ***
[0.17, 0.22]		0.19 ***
[0.16, 0.21]		0.67 ***
[0.65, 0.69]		―
5. ANXMAT0.331.120.87−0.15 ***
[−0.18, −0.13]		0.00
[−0.03, 0.02]		−0.34 ***
[−0.37, −0.32]		−0.41 ***
[−0.43, −0.38]		―
6. BEHENG−0.050.940.64−0.03 *
[−0.06, −0.01]		0.11 ***
[0.08, 0.14]		0.28 ***
[0.25, 0.30]		0.29 ***
[0.26, 0.31]		−0.24 ***
[−0.26, −0.21]		―
7. MATHACHI536.2793.01―0.05 ***
[0.02, 0.08]		0.35 ***
[0.32, 0.37]		0.51 ***
[0.49, 0.54]		0.33 ***
[0.31, 0.36]		−0.15 ***
[−0.17, −0.12]		0.13 ***
[0.11, 0.16]
*** p < 0.001, ** p < 0.01, * p < 0.05. Values in square brackets indicate the 95% confidence interval for each correlation. MALE, male dummy; MATHEFF, self-efficacy for formal and applied mathematics; MATHEF21, self-efficacy for mathematical reasoning and 21st-century mathematics; ANXMAT, mathematics anxiety; BEHENG, behavioural engagement; and MATHACHI, mathematics achievement.
Table 2. Results of path analysis.
Table 2. Results of path analysis.
MATHEFFMATHEF21
PredictorBSE Bβ95%CI for BBSE Bβ95%CI for B
MALE0.34 ***0.040.14[0.26, 0.42]0.40 ***0.030.20[0.35, 0.46]
ESCS0.45 ***0.040.26[0.36, 0.53]0.27 ***0.030.19[0.22, 0.32]
MATHEFF
MATHEF21
ANXMAT
BEHENG
R20.09 0.08
ANXMATBEHENG
PredictorBSE Bβ95%CI for BBSE Bβ95%CI for B
MALE−0.15 ***0.03−0.07[−0.21, −0.10]−0.20 ***0.03−0.10[−0.25, −0.14]
ESCS0.15 ***0.020.10[0.11, 0.19]0.06 **0.020.05[0.02, 0.10]
MATHEFF−0.14 ***0.02−0.15[−0.17, −0.10]0.10 ***0.010.13[0.07, 0.12]
MATHEF21−0.35 ***0.02−0.31[−0.39, −0.30]0.15 ***0.020.16[0.11, 0.18]
ANXMAT −0.12 ***0.02−0.15[−0.16, −0.09]
BEHENG
R20.19 0.12
MATHACHI
PredictorBSE Bβ95%CI for B
MALE−0.030.04−0.01[−0.11, 0.05]
ESCS0.41 ***0.030.24[0.35, 0.48]
MATHEFF0.49 ***0.020.49[0.45, 0.54]
MATHEF21−0.020.02−0.02[−0.06, 0.02]
ANXMAT0.010.020.01[−0.02, 0.04]
BEHENG−0.020.02−0.02[−0.06, 0.01]
R20.34
*** p < 0.001, ** p < 0.01, * p < 0.05. CI, confidence interval; MALE, male dummy; MATHEFF, self-efficacy for formal and applied mathematics; MATHEF21, self-efficacy for mathematical reasoning and 21st-century mathematics; ANXMAT, mathematics anxiety; BEHENG, behavioural engagement; and MATHACHI, mathematics achievement.
Table 3. Means, standard deviations, and t-test results by gender.
Table 3. Means, standard deviations, and t-test results by gender.
MaleFemaletCohen’s d
MSDMSD
SES−0.010.70−0.010.720.110.00
MATHEFF−0.331.30−0.661.138.34 ***0.27
MATHEF21−0.201.07−0.600.9013.47 ***0.41
ANXMAT0.161.150.491.0711.59 ***0.30
BEHENG−0.080.98−0.010.892.20 *0.07
MATHACHI540.0698.35531.1586.642.17 *0.10
*** p < 0.001, ** p < 0.01, * p < 0.05. MALE, male dummy; MATHEFF, self-efficacy for formal and applied mathematics; MATHEF21, self-efficacy for mathematical reasoning and 21st-century mathematics; ANXMAT, mathematics anxiety; BEHENG, behavioural engagement; and MATHACHI, mathematics achievement.
Table 4. Comparison of the hypothesised model for gender.
Table 4. Comparison of the hypothesised model for gender.
ModelAICBICCFITLIRMSEASRMR
Model 1607,350.00608,052.401.001.000.010.00
Model 2607,643.20608,259.501.001.000.030.03
Model 3607,759.90608,283.401.001.000.030.05
Model 4607,774.40608,205.101.001.000.030.05
Table 5. Means, standard deviations, ANOVA results, and multiple comparisons by SES group.
Table 5. Means, standard deviations, ANOVA results, and multiple comparisons by SES group.
High SESMiddle SESLow SESF (2, 77)η2Multiple Comparison
MSDMSDMSD
SES0.860.240.040.29−0.960.378139.41 ***0.82H > M > L
MALE0.490.500.500.500.490.500.260.00
MATHEFF−0.101.26−0.491.16−0.901.2163.57 ***0.05H > M > L
MATHEF21−0.151.01−0.410.98−0.630.9952.25 ***0.03H > M > L
ANXMAT0.311.120.341.120.321.120.440.00
BEHENG0.080.91−0.040.94−0.170.9620.61 ***0.01H > M > L
MATHACHI575.3088.79537.4187.93494.3687.3384.18 ***0.10H > M > L
*** p < 0.001, ** p < 0.01, * p < 0.05. H, high SES; M, middle SES; and L, low SES. MALE, male dummy; MATHEFF, self-efficacy for formal and applied mathematics; MATHEF21, self-efficacy for mathematical reasoning and 21st-century mathematics; ANXMAT, mathematics anxiety; BEHENG, behavioural engagement; and MATHACHI, mathematics achievement.
Table 6. Comparison of the hypothesised model for SES.
Table 6. Comparison of the hypothesised model for SES.
ModelAICBICCFITLIRMSEASRMR
Model 1607,534.70608,687.801.001.000.010.00
Model 2607,518.20608,498.901.001.000.010.01
Model 3607,515.00608,310.201.001.000.010.02
Model 4607,489.30608,032.701.001.000.010.02
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

Share and Cite

MDPI and ACS Style

Shimizu, Y. Relation Between Mathematics Self-Efficacy, Mathematics Anxiety, Behavioural Engagement, and Mathematics Achievement in Japan. Psychol. Int. 2025, 7, 36. https://doi.org/10.3390/psycholint7020036

AMA Style

Shimizu Y. Relation Between Mathematics Self-Efficacy, Mathematics Anxiety, Behavioural Engagement, and Mathematics Achievement in Japan. Psychology International. 2025; 7(2):36. https://doi.org/10.3390/psycholint7020036

Chicago/Turabian Style

Shimizu, Yuno. 2025. "Relation Between Mathematics Self-Efficacy, Mathematics Anxiety, Behavioural Engagement, and Mathematics Achievement in Japan" Psychology International 7, no. 2: 36. https://doi.org/10.3390/psycholint7020036

APA Style

Shimizu, Y. (2025). Relation Between Mathematics Self-Efficacy, Mathematics Anxiety, Behavioural Engagement, and Mathematics Achievement in Japan. Psychology International, 7(2), 36. https://doi.org/10.3390/psycholint7020036

Article Metrics

Back to TopTop