Univariate and Bivariate Log-Topp-Leone Distribution Using Censored and Uncensored Datasets ^†

Abstract

The univariate Topp–Leone distribution introduced by with closed forms of the cumulative distribution function, i.e., [0, 1], was extended to an unbounded limit called the log-Topp–Leone distribution, where the shapes of the hazard function can increase, decrease or remain constant; therefore, this distribution can serve as an alternative distribution to the gamma, Weibull and exponential distributions. The bivariate form of this proposed distribution was introduced by joining the probability density function using three distinct copulas. The MLE, IFM and Bayesian estimation methods were employed to estimate the parameters. The Plackett copula was the best when using the MLE and IFM estimation methods, while the Clayton copula was the best when using the Bayesian method.

1. Introduction

The development and use of statistical distributions are not new matters in statistics. Generating statistical distributions began with the use of systems of differential equations, as in [1], the method of generating systems of frequency curves and the quantile method introduced by [2]. Since then, the trend has changed to the addition of parameters to an existing distribution, as in [3], or the combination of existing standard distributions, as in [4]. Other methods include the beta-generated method and transformed-transformer method, proposed by [5,6], respectively.

Many real-life phenomena, for example, engineering, science and economics are presented in bivariate datasets, in which one component may influence the lifetime of the other component, i.e., in science, one may study the age and resting heart rate of an individual. To model these datasets, several bivariate distributions have been introduced by [7,8,9,10,11] and many others.

2. Methods

This section provides the structural form of the proposed unbounded univariate and bivariate log-Topp–Leone distribution using three different copula functions.

The probability distribution and the density function of the Topp–Leone distribution introduced by [12] are, respectively, given by

$$F(x/\alpha )=x^{\alpha }{(2-x)}^{\alpha } 0<x<1; \alpha >0$$

(1)

$$f(x/\alpha )=2\alpha x^{\alpha -1}(1-x){(2-x)}^{\alpha -1} 0<x<1; \alpha >0$$

(2)

where $\alpha >0$ is the shape parameter.

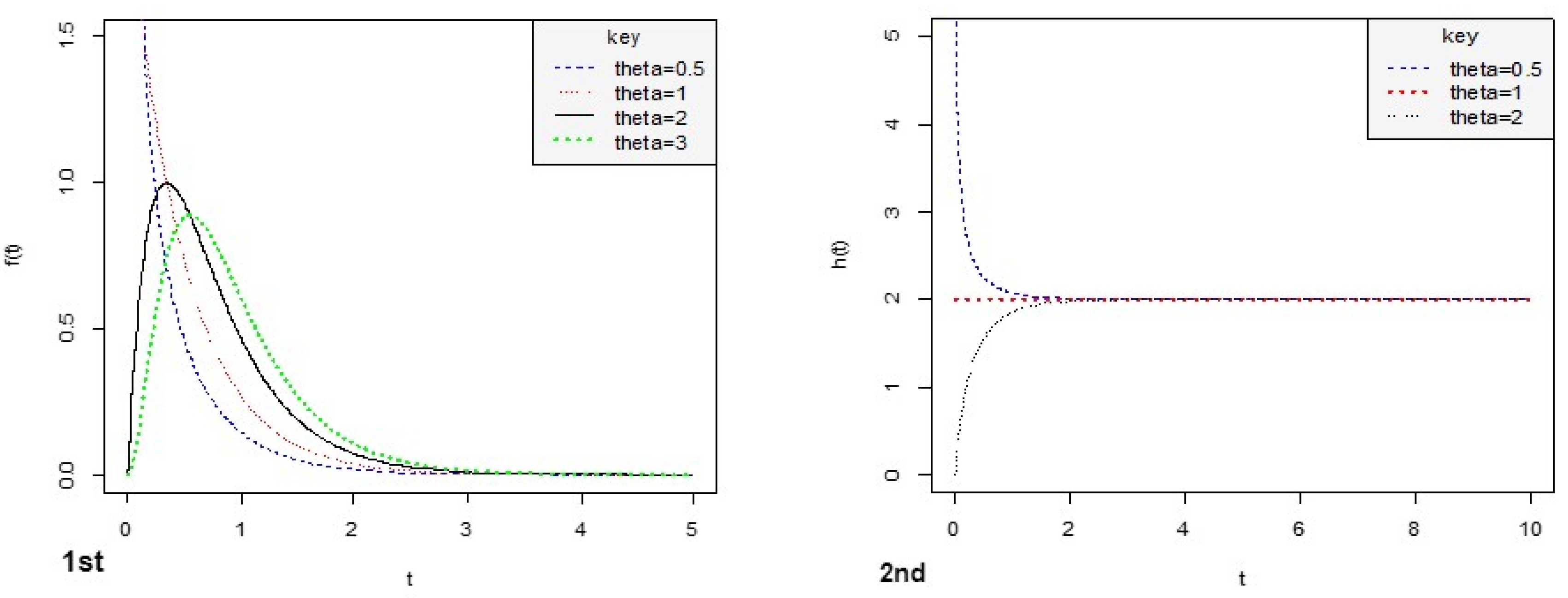
2.1. Log-Topp–Leone Distribution

The newly proposed log-Topp–Leone distribution is introduced by transforming $X=-\log (1-t)$ in Equation (1). When T serves as a random variable denoting the time to the occurrence of an event of interest:

$$F(t/\alpha )={(1-e^{-t})}^{\alpha }{(2-(1-e^{-t}))}^{\alpha } = {(1-e^{-2t})}^{\alpha } , t>0; \alpha >0$$

(3)

the parameter $\alpha$ will maintain its status as a shape parameter. The corresponding pdf is obtained by differentiating Equation (3) as

$$f(t/\alpha )=2\alpha e^{-2t}{(1-e^{-2t})}^{\alpha -1} , t>0; \alpha >0$$

(4)

The survival and hazard functions of the log-Topp–Leone distribution are, respectively, given by

$$S(t/\alpha )=1-{(1-e^{-2t})}^{\alpha }$$

(5)

Figure 1 (1st and 2nd) depicts the pdf and hazard function of the proposed distribu-tion, respectively. The shapes of the hazard function can increase, decrease or remain constant; therefore, this distribution can serve as an alternative distribution to the gamma, Weibull and exponential distributions.

Figure 1. Plots of the pdf (1st) and hazard function (2nd) of the log-Topp–Leone distribution for some parameter values.

2.2. Copula

Sklar [13] first introduced the copula function to connect the multivariate distribution function with individual marginals.

2.2.1. Model Based on Farlie–Gumbel–Morgenstern Copula

The joint survival function based on the FGM copula for $T_1$ and $T_2$ is given by

$$S\left(t_1, t_2\right)=S\left(t_1\right)S\left(t_2\right)\left\{1+\varphi F\left(t_1\right)F\left(t_2\right)\right\}$$

(6)

where $\varphi \in \left[-1,1\right]$.

2.2.2. Model Based on Clayton Copula

The joint survival function based on the Clayton copula for $T_1$ and $T_2$ is given by

$$S(t_1,t_2)={\left(S{(t_1)}^{-\varphi }+S{(t_2)}^{-\varphi }-1\right)}^{-\frac{1}{\varphi }}$$

(7)

where $\varphi$ is the dependence parameter, taking values in the interval $(0,\infty )$.

2.2.3. Model Based on Plackett Copula

The joint survival function based on the Plackett copula for $T_1$ and $T_2$ is given by

$$S(t_1,t_2)=\frac{1+\left(\theta -1\right)\left(S(t_1)+S(t_2)\right)-\sqrt{{\left[1+\left(\theta -1\right)S(t_1)+S(t_2)\right]}^2-4S(t_1)S(t_2)\theta \left(\theta -1\right)}}{2(\theta -1)}$$

(8)

where $\theta \in \left(0,\infty \right).$

2.3. Inference Methods

This section provides the parameter estimates of the bivariate log-Topp–Leone distribution using the MLE, IFM and Bayesian estimation methods.

Bayesian Method of Estimation

This section considers cases where both $t_{1i}$ and $t_{2i}$ are censored and uncensored observations.

(a)

When both $t_{1i}$ and $t_{2i}$ are censored observations.

Assuming that ${\omega }_{ji}=1$ when $t_{ji}$ and $t_{2i}$ are both censored observations, where $j=1,2$ and $i=1,2,\dots n$, the likelihood function is given by

$${\displaystyle \prod _{i=1}^n{\left[\frac{{\partial }^{2}S(t_{1i},t_{2i})}{\partial t_{1i}\partial t_{2i}}\right]}^{{\omega }_{1i}{\omega }_{2i}}{\left[\frac{-\partial S(t_{1i},t_{2i})}{\partial t_{1i}}\right]}^{{\omega }_{1i}(1-{\omega }_{2i})}{\left[\frac{-\partial S(t_{1i},t_{2i})}{\partial t_{2i}}\right]}^{{\omega }_{2i}(1-{\omega }_{1i})} {\left[S(t_{1i},t_{2i})\right]}^{(1-{\omega }_{1i})(1-{\omega }_{2i})} }$$

(9)

where ${\omega }_{1i}$ and ${\omega }_{2i}$ are two indicator variables, and $i=1,2,\dots n$.

(b)

When both $t_{1i}$ and $t_{2i}$ are uncensored or complete observations.

When both $t_{1i}$ and $t_{2i}$ are uncensored or complete observations, i.e., ${\omega }_{ji}=1$, the likelihood function in Equation (8) will reduce to

$${\displaystyle \prod _{i=1}^n\left[\frac{{\partial }^{2}S(t_{1i},t_{2i})}{\partial t_{1i}\partial t_{2i}}\right] }$$

(10)

2.4. Deviance Information Criterion

The deviance information criterion (DIC) proposed by [14] is defined as

$$D(\mathrm{Z})=D(\widehat{\mathrm{Z}})-2n_p$$

where $D(\widehat{\mathrm{Z}})$ is the deviance, $n_p=\overline{D}-D(\widehat{\mathrm{Z}})$ and $\overline{D}$ is the posterior deviance.

3. Results and Discussion

This section provides the goodness-of-fit results for all the copulas.

In Table 1, all the results of the p-values for the copulas are statistically significant. This proves the suitability of all the copulas for the dataset. Goodness-of-fit measures, i.e., AIC and BIC, were employed to select the best model, where the model with the lowest AIC and BIC values was regarded as the best model. The results from the Plackett copula were the lowest for all criteria; therefore, the Plackett copula was better than the FGM copula. Regarding the estimation methods, the MLE estimates were better than the IFM estimates for the two models, based on the standard error values.

Table 1. Standard error, p-value and goodness-of-fit measure results for the copulas.

Copula	Methods	SE	p-Value	Dependence Parameter	AIC	BIC
FGM Copula		0.0000	0.0000	37.0010	6177.690	6179.106
Plackett Copula	MLE	2.0970	0.0000	41.1350	6168.544	6169.960
FGM Copula		2.0970	0.0000	28.8580	6200.704	6202.120
Plackett Copula	IFM	2.9660	0.0000	29.9265	6196.408	6197.824

As shown in Table 2 and Table 3, the joint posterior distribution was obtained by combining the likelihood function with the joint prior distribution to obtain some information of interest. This information was obtained by generating different Gibbs samples for each parameter. The different samples generated helped in observing the DIC values as the sample size increased, and it is clearly shown that this process requires a large sample size for small DIC values and a better model selection. Here, the Clayton copula with the lowest DIC value for different sample sizes was regarded as a better model than the FGM copula for the censored and uncensored cases.

Table 2. Posterior summary statistics for censored dataset using FGM and Clayton copula functions.

Gibbs Samples for Parameters		FGM Copula			CLAYTON Copula
	Par.	Mean	MC Error	95% CI	Mean	MC Error	95% CI
1000	${\alpha }_1$	0.9738	0.0040	(0.8889, 0.9998)	0.8720	0.0204	(0.6556, 0.9986)
	${\alpha }_2$	61.000	5.9670	(5.1140, 94.960)	13.450	2.4130	(1.5550, 36.860)
	$\varphi$	8.7960	0.8389	(0.9910, 14.340)	17.850	3.3990	(1.4890, 49.380)
			DIC = 4409		DIC = 2981
10,000	${\alpha }_1$	0.9734	0.0021	(0.8894, 0.9994)	0.7746	0.0052	(0.6479, 0.9887)
	${\alpha }_2$	45.180	1.1470	(9.7790, 84.0200)	25.700	0.5973	(1.8310, 33.680)
	$\varphi$	69.660	2.1100	(1.5960, 92.9900)	44.270	1.0790	(1.7960, 57.560)
		DIC = 3868			DIC = 2502
100,000	${\alpha }_1$	0.9747	0.0004	(0.9063, 0.9994)	0.7624	0.0010	(0.6425, 0.8983)
	${\alpha }_2$	43.650	0.1995	(34.740, 53.680)	26.850	0.1072	(20.940, 33.500)
	$\varphi$	76.340	0.3931	(60.640, 93.550)	46.940	0.1988	(36.750, 58.350)
Gibbs samples for parameters	DIC = 3786				DIC = 2448

Table 3. Posterior summary statistics for uncensored dataset using FGM and Clayton copula functions.

Gibbs Samples for Parameters		FGM Copula			CLAYTON Copula
	Par.	Mean	MC Error	95% CI	Mean	MC Error	95% CI
1000	${\alpha }_1$	0.9224	0.0154	(0.6035, 0.9993)	0.7164	0.0113	(0.5797, 0.8807)
	${\alpha }_2$	15.750	1.2350	(5.1590, 29.210)	8.3210	0.4135	(0.9622, 11.610)
	$\varphi$	22.100	2.9410	(0.9919, 44.240)	22.370	0.9948	(4.8590, 31.990)
			DIC = 4489		DIC = 2679
10,000	${\alpha }_1$	0.9665	0.0030	(0.8747, 0.9992)	0.7255	0.0022	(0.6162, 0.8484)
	${\alpha }_2$	14.540	0.2097	(11.080, 21.480)	9.8050	0.0897	(7.0100, 12.130)
	$\varphi$	36.100	0.7086	(5.9070, 45.080)	21.360	0.1585	(16.650, 27.690)
		DIC = 4188			DIC = 2631
100,000	${\alpha }_1$	0.9681	0.0006	(0.8864, 0.9991)	0.7267	0.0006	(0.6159, 0.8497)
	${\alpha }_2$	14.400	0.0223	(11.640, 17.500)	9.9510	0.0173	(7.8550, 12.230)
	$\varphi$	37.610	0.1258	(30.400, 45.410)	21.240	0.0238	(16.730, 26.390)
		DIC = 4156			DIC = 2631

4. Conclusions

The univariate Topp–Leone distribution introduced by [12] with closed forms of the cumulative distribution function, i.e., [0, 1], was extended to an unbounded limit called the log-Topp–Leone distribution, where the shapes of the hazard function can increase, decrease or remain constant; therefore, this distribution can serve as an alternative distribution to the gamma, Weibull and exponential distributions. The bivariate form of this proposed distribution was introduced by joining the probability density function using three distinct copulas. First, two models were studied based on the FGM and Plackett copulas, and the parameters were estimated using the MLE and IFM estimation methods. The Plackett copula with the lowest AIC and BIC values for both of the estimation methods fit the dataset very well compared to the FGM copula. Two copulas, namely the Clayton and FGM copulas, were implemented using the Bayesian estimation method. This method is based on the Markov chain Monte Carlo simulation technique, and the criterion used is the deviance information criterion (DIC). The Clayton copula with the lowest DIC value for different sample sizes was regarded as a better model than the FGM copula for the censored and uncensored cases.