The Impact of a Non-Uniform Magnetic Field on Mixed Convection in a Lid-Driven Cavity Filled with Fe3O4/Water Nanofluid †
Abstract
:1. Introduction
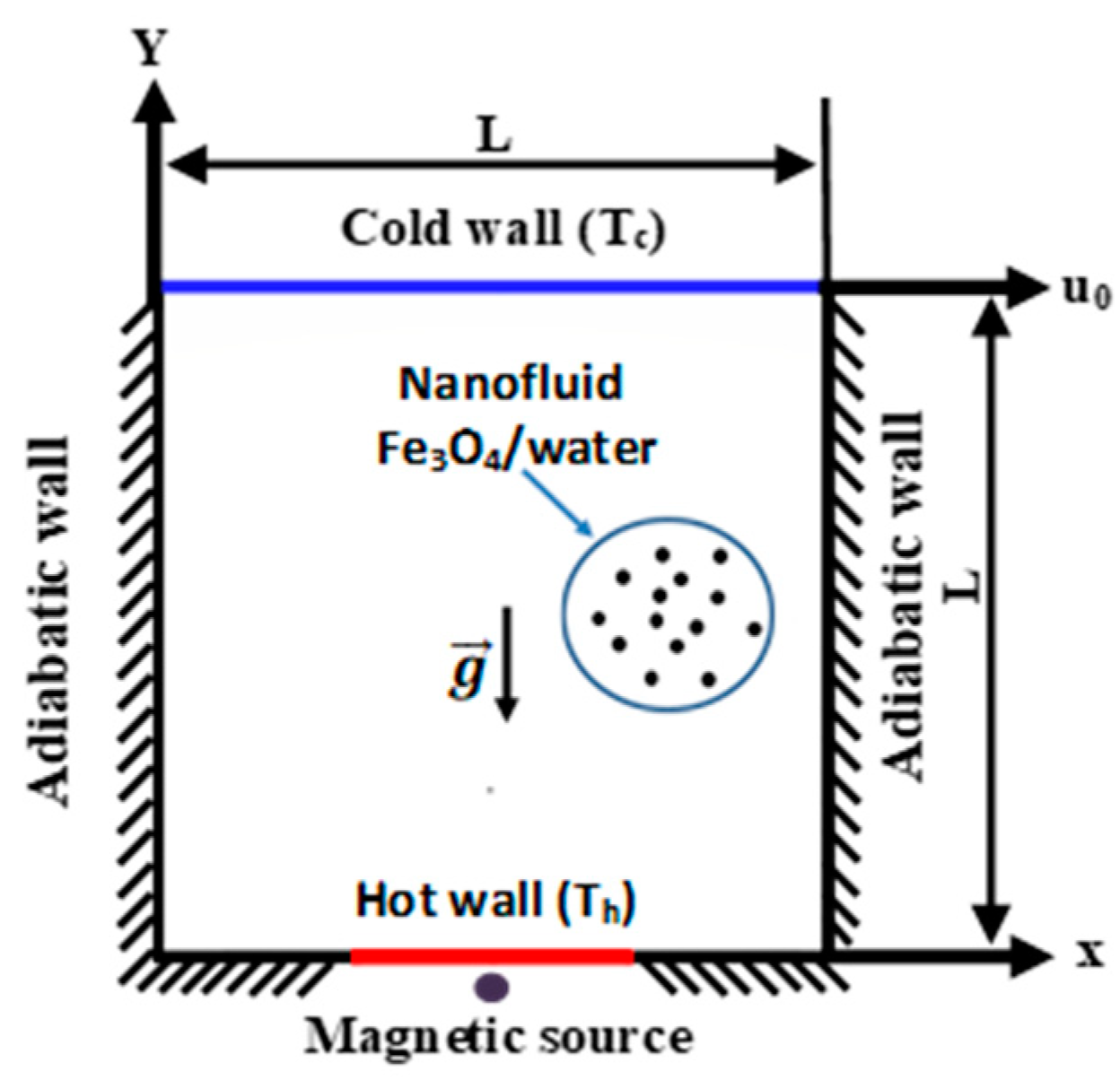
2. Physical Model
3. Problem Geometry
4. Results
5. Conclusions
- ✓
- The magnetic field is more beneficial at lower Richardson values.
- ✓
- The buoyancy force is dominant, which reduces the effectiveness of the MF.
- ✓
- The average Nusselt number is higher at a lower Richardson number.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Siddiqui, A.A.; Turkyilmazoglu, M. Natural convection in the ferrofluid enclosed in a porous and permeable cavity. Int. Commun. Heat Mass Transf. 2020, 113, 104499. [Google Scholar] [CrossRef]
- Ghorbani, B.; Ebrahimi, S.; Vijayaraghavan, K. CFD modeling and Sensitivity Analysis of heat transfer enhancement of a ferrofluid flow in the presence of a magnetic field. Int. J. Heat Mass Transf. 2018, 127, 544–552. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Mehryan, S.A.M.; Shafee, A.; Sheremet, M.A. Variable magnetic forces impact on magnetizable hybrid nanofluid heat transfer through a circular cavity. J. Mol. Liq. 2019, 277, 388–396. [Google Scholar] [CrossRef]
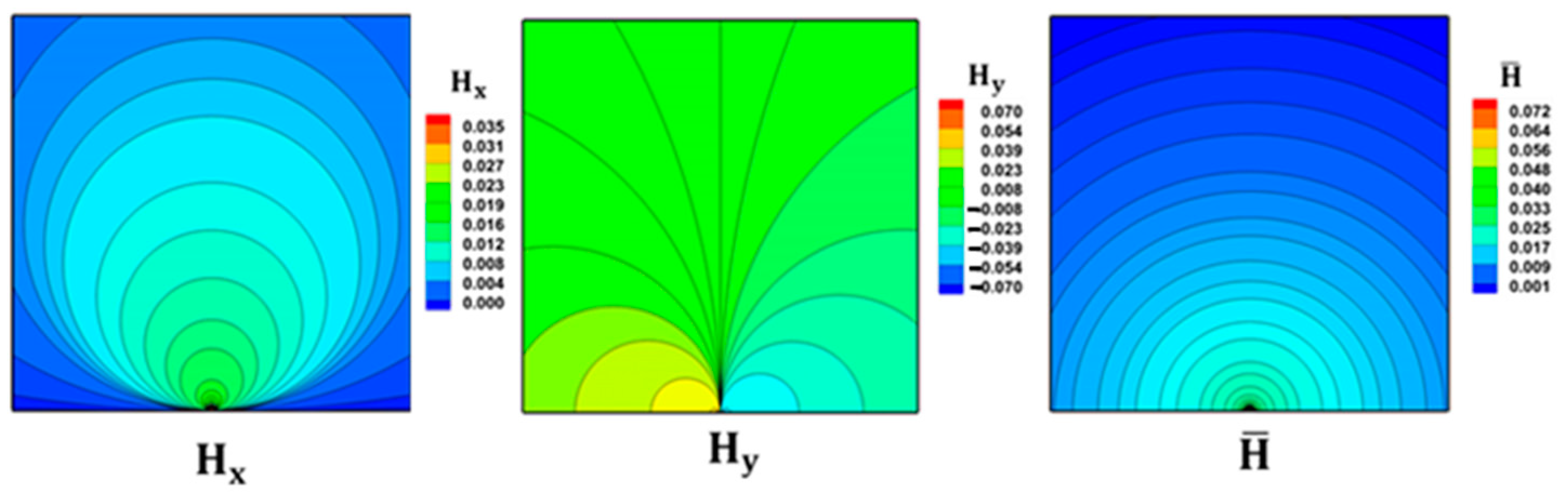

| Ri = 0.4 | Ri = 0.04 | |||
|---|---|---|---|---|
| Mn = 25 | Mn = 50 | Mn = 25 | Mn = 50 | |
| Average Nusselt number | 15.3111 | 18.403 | 52.7946 | 67.643 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Korei, Z.; Bentoumi, L. The Impact of a Non-Uniform Magnetic Field on Mixed Convection in a Lid-Driven Cavity Filled with Fe3O4/Water Nanofluid. Comput. Sci. Math. Forum 2023, 7, 23. https://doi.org/10.3390/IOCMA2023-14410
Korei Z, Bentoumi L. The Impact of a Non-Uniform Magnetic Field on Mixed Convection in a Lid-Driven Cavity Filled with Fe3O4/Water Nanofluid. Computer Sciences & Mathematics Forum. 2023; 7(1):23. https://doi.org/10.3390/IOCMA2023-14410
Chicago/Turabian StyleKorei, Zakaria, and Lemya Bentoumi. 2023. "The Impact of a Non-Uniform Magnetic Field on Mixed Convection in a Lid-Driven Cavity Filled with Fe3O4/Water Nanofluid" Computer Sciences & Mathematics Forum 7, no. 1: 23. https://doi.org/10.3390/IOCMA2023-14410
APA StyleKorei, Z., & Bentoumi, L. (2023). The Impact of a Non-Uniform Magnetic Field on Mixed Convection in a Lid-Driven Cavity Filled with Fe3O4/Water Nanofluid. Computer Sciences & Mathematics Forum, 7(1), 23. https://doi.org/10.3390/IOCMA2023-14410






