Abstract
Half-Heusler alloys are promising materials for thermoelectric applications, yet the impact of the compositional disorder on their lattice dynamics remains incompletely understood. This study investigates the effect of systematic Zr substitution on the lattice dynamics and thermal properties of TixZr1-xNiSn half-Heusler alloys using first-principles calculations. Through careful analysis of phonon dispersions, density of states, and thermodynamic properties, it is revealed that Zr substitution (25%, 50%, and 75%) introduces minimal structural distortion while enhancing system stability. It is shown that increasing Zr content systematically modifies the phonons, particularly affecting the high-frequency optical modes above 5 THz. Notably, with Zr content, these findings provide valuable insights for tailoring the thermal properties of half-Heusler alloys for high-temperature applications in thermoelectric devices and components.
1. Introduction
Half-Heusler alloys crystallize in the MgAgAs-type structure with a C1b structure and 1:1:1 stoichiometry, representing an interesting class of materials that have gained research interest due to their remarkable versatility in applications such as microelectronics, photovoltaics, and thermoelectric energy conversion [,]. The structural framework of these ternary semi-metallic compounds consists of two interpenetrating face-centered cubic sublattices, exhibiting a combination of covalent and ionic bonding. The physical properties of half-Heusler alloys are tunable through compositional modifications, for instance, doping with a substitutional element. Recent theoretical investigations have shown how strategic atomic substitution can fundamentally alter the lattice dynamics and electronic structure, underscoring the profound influence of compositional modifications on material stability and functional properties [,,,,,,,]. Studies suggest that alloying the A-site sublattice (e.g., Ti, Zr, Hf) in TiNiSn alloys can significantly reduce lattice thermal conductivity without considerably affecting electrical conductivity [,,,]. Isoelectronic (Congeners) substitution in these systems can introduce mass disorder and anharmonic scattering, which are beneficial for lowering thermal conductivity [,,]. Hence, it becomes crucial to understand the lattice dynamics of these materials. TiNiSn and ZrNiSn half-Heusler compounds have emerged as promising materials for thermoelectric and structural applications due to their remarkable combination of properties.
TiNiSn exhibits a Seebeck coefficient of −142 μV/K and a thermal conductivity of 9.3 W/mK, making it particularly attractive for thermoelectric power generation in the mid-temperature range (400–700 K) [,,]. Its relatively high melting point (~1450 K) and chemical stability enable sustained operation in demanding environments. ZrNiSn, with a Seebeck coefficient of −176 μV/K, higher thermal stability (melting point ~1700 K), and lower thermal conductivity of 6.7 W/mK, demonstrates enhanced high-temperature performance [,,]. This investigation focuses on the lattice dynamics of TixZr1-xNiSn system, specifically examining the impact of A-site substitutional doping on vibrational properties. This work addresses a critical knowledge gap in understanding how isovalent–homolog elemental substitution influences phonon behavior in TixZr1-xNiSn. The systematic replacement of Ti with its homolog element “Zr” provides control over tuning structural and vibrational characteristics. This approach enables us to understand key factors that influence the behavior of TixZr1-xNiSn, which is essential for assessing the effects of Zr and Ti properties.
2. Methods
For all first-principles computations, we employed the projector augmented wave (PAW) method within VASP code [,]. To optimize the unit cell and atomic positions, we used the Perdew–Burke–Ernzerhof (PBE) functional []. Additionally, we set a high energy cutoff of 520 eV for planewave expansion. A smearing width of 0.01 eV with the Monkhorst–Pack method was utilized to create a K-point mesh for Brillouin zone integration, aiming for a precision level of 0.02 []. An electronic convergence criterion 10−8 eV was set between two self-consistent loops, and an ionic convergence criterion of 10−6 eV/Å was set to obtain a structure without residual forces for phonon calculations. Phonopy code was employed to create displacement based on the frozen phonon model to calculate thermal properties [,,,,]. A unit cell with 12 atoms was used for TiNiSn half-Heusler alloy, and a disorder was created on the Ti site with Zr atoms in percentages of 25%, 50%, and 75%. A disordered unit cell of TixZr1-xNiSn half-Heusler alloy was generated using sqsgen code []. Table 1 shows the Wyckoff position for Ti/Zr, Ni, and Sn in the C1b structure. The VESTA [] program was used to visualize the unit cells of all alloys, as shown in Figure 1.

Table 1.
Atomic positions of elements in TiNiSn or ZrNiSn half-Heusler alloys.
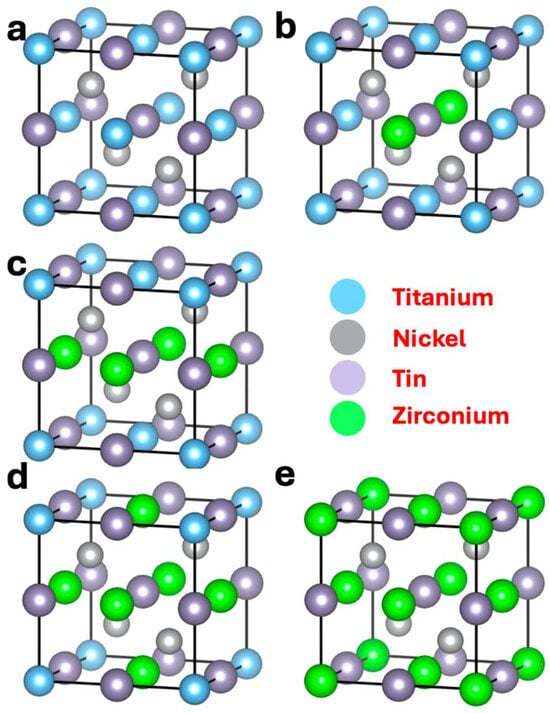
Figure 1.
Relaxed crystal structures of (a) TiNiSn; (b) Ti0.75Zr0.25NiSn; (c) Ti0.5Zr0.5NiSn; (d) Ti0.25Zr0.75NiSn; and (e) ZrNiSn are illustrated with sky blue, gray, purple, and green atoms representation titanium, nickel, tin, and zirconium. The relaxed structures show minimal distortion due to the incorporation of Zr atoms into the TiNiSn half-Heusler alloy.
Heat capacity at equilibrium volume was determined by mapping the total energy to the Vinet equation of state, while the variation in thermal properties with temperature was computed using the equation of states and quasi-harmonic approximation based on the Helmholtz free energy and volume [,]. The energy of a system of phonons is expressed as , where is the Planck’s constant, kB is the Boltzmann constant, T is the temperature, and ωqi is the phonon frequency for wave vector q and band index i. The Helmholtz free energy is given by , and the heat capacity at constant volume is given by . The phonon dispersion relation is given by , where C is a constant, M is the mass, and a is the distance traversed by the wave [,].
3. Results and Discussion
Figure 1 shows relaxed crystal structures of TixZr1-xNiSn half-Heusler alloys. Minimal distortion in the unit cells of TixZr1-xNiSn with increasing substitution is reported from the relaxed unit cells. Furthermore, the increment in Zr in TiNiSn stabilizes the structure, as evidenced by the values of ΔE/atom shown in Figure 2a; similar values (550 to 750 meV) and variation in energies from TiNiSn to ZrNiSn are reported by Gürth et al. []. The increases in lattice constant and density, as shown in Figure 2b,c, are attributed to the fact that Zr atoms are larger and heavier than Ti atoms. It is also interesting to note that Ti and Zr form a nearly isomorphous system, as reported in their binary alloy phase diagram []. This is attributed to the fact that titanium and zirconium exhibit remarkable crystallographic similarities, featuring both hcp (α) and bcc (β) phases. Their comparable atomic radii (147 pm vs. 160 pm), electronegativities (~1.54 vs. ~1.33), and identical valency states (+4) promote isomorphous behavior. The advantage of their atomic and crystallographic similarities is seen through minimal lattice distortion, enhanced structural stability, and variation in lattice constant with increasing Zr content.
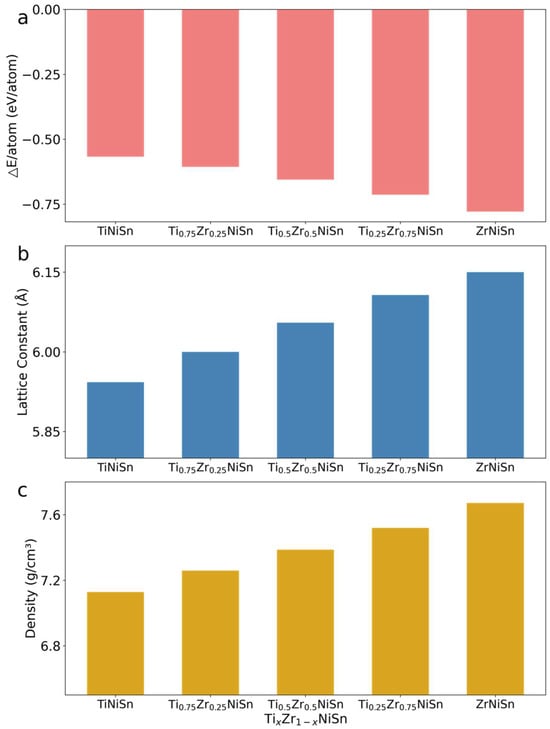
Figure 2.
Energetics and structural characteristics of TixZr1-xNiSn half-Heusler alloys shown as bar charts for (a) ΔE/atom in eV/atom; (b) lattice constant in Å; and (c) density in gm/cm3. The trend suggests a unanimous increase in stability with Zr concentration, while in the cases of lattice constant and density, we report an opposite trend of unanimous decrease with Zr concentration.
In Figure 3, phonon density of states (Ph-DOS) for TixZr1-xNiSn half-Heusler alloys is shown; it not only highlights the vibrational states of elements but also provides an understanding of the force constants and bond strength between nearest neighbors. In the pristine TiNiSn compound, the high-frequency modes above 8 THz indicate strong (short) Ti-X (X = Ni, Sn) bonding characterized by a large value of force constants. Upon systematic Zr substitution, several notable modifications emerge in the vibrational spectrum. The Ti-specific Ph-DOS exhibits progressive intensity reduction coupled with systematic peak shifts toward lower frequencies, reflecting enlarged bond lengths and reduced force constants. This behavior stems from the interplay between lattice misfit/expansion and electronic structure perturbations induced by the larger Zr atoms. The distinctive peak characterized by the highest intensity, observed in the Ph-DOS for Zr in ZrNiSn and Ti in TiNiSn at frequencies around 5–7 THz, represents localized vibrational modes, originating from an ordered structure and regular bonds. The remarkably sharp peak for Zr demonstrates a highly constrained vibrational state, primarily arising from uniform bond lengths and a similar value of force constant due to an ordered structure. This effect is influenced by Zr’s larger atomic radius and higher atomic mass (91.2 amu compared to Ti’s 47.9 amu), which result in more uniform force constants by minimizing coupling with other vibrational modes. The higher peak intensity for Zr/Ti reflects the uniformity of bonding with neighboring atoms, while the narrow peak width indicates a high degree of mode localization resulting in a small frequency range for vibrations. Concurrently, the Ni vibrational response, predominantly manifesting in the 5–7 THz range, demonstrates enhanced Ph-DOS intensity and spectral broadening with increasing Zr content, indicating an elongation in the Zr-Ni bond as compared to the Ti-Ni bond as well as the influence of mass disparities between Ni and Ti/Zr. Since Zr is heavier than Ni, Ni exhibits higher Ph-DOS, whereas in TiNiSn, the lower mass of Ti compared to Ni results in a more prominent Ti peak. Sn, being the heaviest element in the group, has vibrational characteristics centered in the low-frequency 2–4 THz range; these maintain relatively stable spectral profiles with subtle peak broadening and several small peaks arising due to disorder, suggesting minimal perturbations in local electronic environments coupled with lattice and bond distortions. The underlying factors governing these observations consist of the electronic configuration differences between Ti 3d and Zr 4d orbitals affecting hybridization strengths, while atomic size disparities introduce local lattice as well as bond distortions leading to variations in force constants. These electronic and structural perturbations collectively manifest in the systematic evolution of Ph-DOS, also influencing the alloy’s thermodynamic properties.
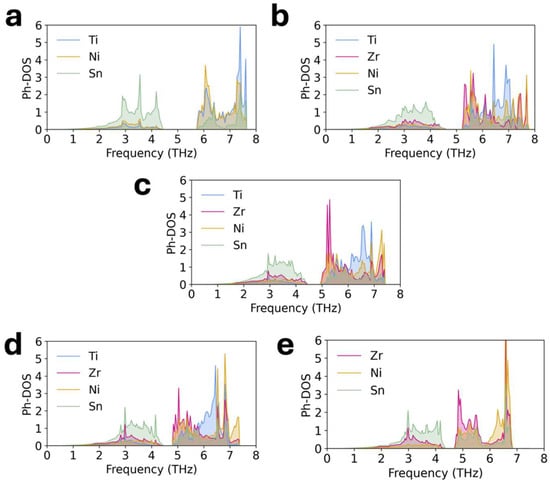
Figure 3.
Elemental phonon density of states (Ph-DOS) for (a) TiNiSn; (b) Ti0.75Zr0.25NiSn; (c) Ti0.5Zr0.5NiSn; (d) Ti0.25Zr0.75NiSn; and (e) ZrNiSn. With the incorporation of Zr, the maximum frequency of vibration for the system reduced.
The phonon band structures shown in Figure 4 reveal the vibrational properties of TiNiSn and its Zr-substituted derivatives, Ti0.75Zr0.25NiSn, Ti0.50Zr0.50NiSn, Ti0.25Zr0.75NiSn, and ZrNiSn. The phonon band structure is plotted on a high-symmetry path corresponding to an FCC lattice in order to compare its variation across all compositions with different symmetries while maintaining the same underlying FCC structure. Across all compositions, the absence of imaginary frequencies in the phonon dispersion curves confirms the dynamic stability of these half-Heusler alloys. Substitution of Ti with Zr introduces noticeable softening in certain optical phonon branches, particularly near the M and A high-symmetry points. While Zr has a larger atomic mass and radius than Ti, the observed softening of optical phonon modes near M and A high-symmetry points is primarily driven by bonding characteristics rather than lattice expansion. Ti (1.54) forms stronger covalent bonds with Ni (1.91) and Sn (1.96) due to its higher electronegativity. In contrast, Zr’s (1.33) lower electronegativity results in more ionic bonding, leading to weaker bond stiffness and lower force constants. This increased bond ionicity in ZrNiSn, combined with Zr’s higher mass, explains the observed reduction in optical phonon frequencies. The acoustic branches near the Γ-point remain robust, indicating mechanical stability across the series. As the Zr content increases, the gradual shift in phonon modes suggests the tunability of vibrational dynamics, which influences lattice thermal conductivity as well as thermoelectric response. Such modifications to the phonon spectrum are a pathway for tailoring thermoelectric properties.
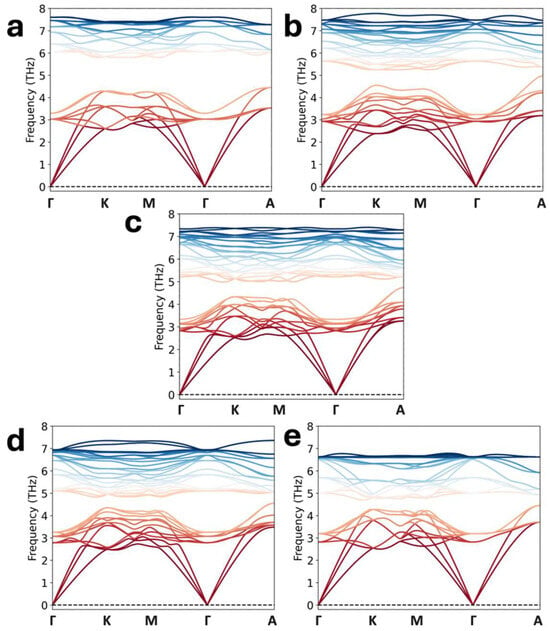
Figure 4.
Phonon band structure for (a) TiNiSn; (b) Ti0.75Zr0.25NiSn; (c) Ti0.5Zr0.5NiSn; (d) Ti0.25Zr0.75NiSn; and (e) ZrNiSn. The substitution of Ti with Zr introduces gradual changes in the phonon dispersion, reflecting mass and bonding variations. No imaginary frequencies are observed in any structure, confirming dynamic stability.
The vibrational entropy (Svib) and heat capacity (Cv) of TiNiSn and its Zr-substituted derivatives provide insights into the thermodynamic behavior of these half-Heusler alloys. The Svib curves in Figure 5a show a consistent increase with temperature, reflecting enhanced vibrational activity as thermal energy increases. Substitution of Ti with Zr leads to subtle differences in Svib, particularly at high temperatures (as highlighted in the inset), which can be attributed to the larger atomic mass of Zr. This indicates a minor influence of Zr substitution on the vibrational entropy, suggesting that the alloys retain similar lattice dynamic behavior across compositions. The heat capacity Cv, shown in Figure 5b, exhibits a rapid increase at lower temperatures and plateaus near the Dulong–Petit limit (~300 J/K·mol) at high temperatures. These results highlight that the Ti-to-Zr substitution allows for compositional flexibility without compromising the high-temperature thermal properties, which are advantageous for thermoelectric and structural applications.
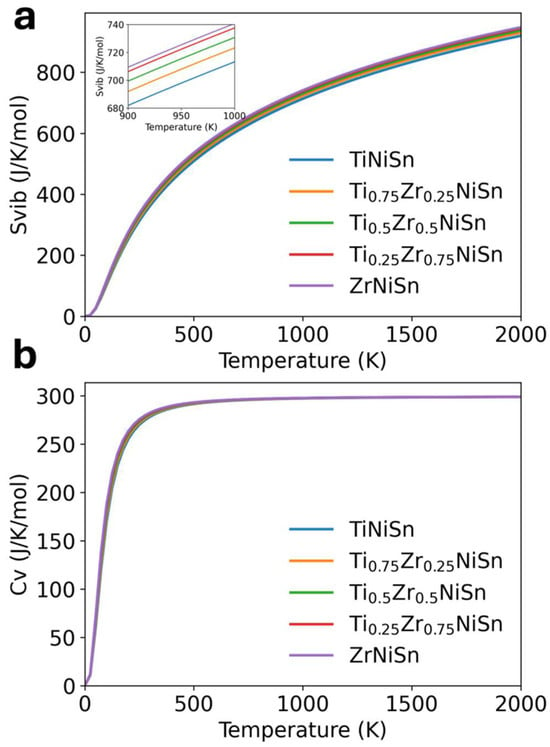
Figure 5.
Vibrational entropy (Svib) and heat capacity (Cv) of TiNiSn and Zr-substituted derivatives as a function of temperature. (a) Svib increases monotonically with temperature, showing minimal differences across compositions. The inset highlights subtle variations in Svib at high temperatures, attributed to the mass and bonding differences introduced by Zr substitution. (b) Cv approaches the Dulong–Petit limit (~300 J/K·mol) at high temperatures, with negligible variation between compositions.
4. Conclusions
First-principles calculations of TixZr1-xNiSn half-Heusler alloys reveal several key findings regarding the impact of Zr substitution on lattice dynamics and thermal properties. The systematic substitution of Zr in TixZr1-xNiSn maintains structural integrity while enhancing the system’s stability, which is evidenced by the absence of imaginary modes in phonon dispersions for all compositions. The observed modifications in Ph-DOS, particularly the systematic evolution of Ti and Ni vibrational modes, is a demonstration of the tunability of lattice dynamics through compositional modifications. The preservation of similar vibrational entropy and heat capacity profiles for all compositions, suggests that Zr substitution offers a viable strategy for enhancing stability with optimized thermal properties. These results provide valuable design principles for developing next-generation half-Heusler alloys with enhanced thermal properties for thermoelectric and structural applications in high-temperature environments. Finally, the established structure-property relationships offer a foundation for future investigations into similar multicomponent systems.
Funding
This research received no external funding.
Data Availability Statement
Data will be made available on reasonable request.
Acknowledgments
The author would like to acknowledge the Lehigh University LTS Computing Cluster, Hawk.
Conflicts of Interest
The author declares no conflicts of interest.
References
- Bende, D.; Grin, Y.; Wagner, F.R. Chemical Bonding in MgAgAs-Type Compounds. In Heusler Alloys: Properties, Growth, Applications; Springer: Cham, Switzerland, 2016; pp. 133–156. [Google Scholar]
- Galanakis, I. Theory of Heusler and Full-Heusler Compounds. In Heusler Alloys: Properties, Growth, Applications; Springer: Cham, Switzerland, 2016; pp. 3–36. [Google Scholar]
- Sharma, P.; Singh, P.; Balasubramanian, G. Engineering Phonon Transport Through Cation Disorder in Dimensionally Constricted High Entropy MXene. Carbon 2024, 223, 119015. [Google Scholar] [CrossRef]
- Sharma, P.; Balasubramanian, G. Electronic and Lattice Distortions Induce Elastic Softening in Refractory Multicomponent Borides. Chem. Mater. 2023, 35, 7511–7520. [Google Scholar] [CrossRef] [PubMed]
- Sharma, P.; Balasubramanian, G. High Configurational Entropy Driven Increased Heat Capacity of Refractory Multicomponent Di-Silicides. Materialia 2023, 30, 101861. [Google Scholar] [CrossRef]
- Zarkevich, N.A.; Singh, P.; Smirnov, A.V.; Johnson, D.D. Effect of Substitutional Doping and Disorder on the Phase Stability, Magnetism, and Half-Metallicity of Heusler Alloys. Acta Mater. 2022, 225, 117477. [Google Scholar] [CrossRef]
- Patel, P.D.; Pillai, S.B.; Shinde, S.M.; Gupta, S.D.; Jha, P.K. Electronic, Magnetic, Thermoelectric and Lattice Dynamical Properties of Full Heusler Alloy Mn2RhSi: DFT Study. Phys. B Condens. Matter 2018, 550, 376–382. [Google Scholar] [CrossRef]
- Sharma, P.; Johnson, D.D.; Balasubramanian, G.; Singh, P. Unraveling the Connection of Electronic and Phononic Structure with Mechanical Properties of Commercial AZ80 Alloy. Mater. Lett. 2024, 366, 136501. [Google Scholar] [CrossRef]
- Sharma, P.; Gandhi, P.M.; Chintersingh, K.-L.; Schoenitz, M.; Dreizin, E.L.; Liou, S.-C.; Balasubramanian, G. Accelerated Intermetallic Phase Amorphization in a Mg-Based High-Entropy Alloy Powder. J. Magnes. Alloys 2024, 12, 1792–1798. [Google Scholar] [CrossRef]
- Sharma, P.; Naushin, N.; Rohila, S.; Tiwari, A. Magnesium Containing High Entropy Alloys. In Magnesium Alloys Structure and Properties; IntechOpen: London, UK, 2022. [Google Scholar]
- Eliassen, S.N.H.; Katre, A.; Madsen, G.K.H.; Persson, C.; Løvvik, O.M.; Berland, K. Lattice Thermal Conductivity of TixZryHf1−x−yNiSn Half-Heusler Alloys Calculated from First Principles: Key Role of Nature of Phonon Modes. Phys. Rev. B 2017, 95, 045202. [Google Scholar] [CrossRef]
- Hohl, H.; Ramirez, A.P.; Goldmann, C.; Ernst, G.; Wölfing, B.; Bucher, E. Efficient Dopants for ZrNiSn-Based Thermoelectric Materials. J. Phys. Condens. Matter 1999, 11, 1697–1709. [Google Scholar] [CrossRef]
- Shutoh, N.; Sakurada, S. Thermoelectric Properties of the TiX(Zr0.5Hf0.5)1−XNiSn Half-Heusler Compounds. J. Alloys Compd. 2005, 389, 204–208. [Google Scholar] [CrossRef]
- Sakurada, S.; Shutoh, N. Effect of Ti Substitution on the Thermoelectric Properties of (Zr,Hf)NiSn Half-Heusler Compounds. Appl. Phys. Lett. 2005, 86, 082105. [Google Scholar] [CrossRef]
- Singh, P.; Acemi, C.; Kuchibhotla, A.; Vela, B.; Sharma, P.; Zhang, W.; Mason, P.; Balasubramanian, G.; Karaman, I.; Arroyave, R.; et al. Alloying Effects on the Transport Properties of Refractory High-Entropy Alloys. Acta Mater. 2024, 276, 120032. [Google Scholar] [CrossRef]
- Chai, Y.W.; Oniki, T.; Kenjo, T.; Kimura, Y. The Effect of an Isoelectronic Ti–Zr Substitution on Heusler Nanoprecipitation and the Thermoelectric Properties of a (Ti0.2,Zr0.8)Ni1.1Sn Half-Heusler Alloy. J. Alloys Compd. 2016, 662, 566–577. [Google Scholar] [CrossRef]
- Choudhary, M.K.; Ravindran, P. First Principle Design of New Thermoelectrics from TiNiSn Based Pentanary Alloys Based on 18 Valence Electron Rule. Comput. Mater. Sci. 2022, 209, 111396. [Google Scholar] [CrossRef]
- Echevarria-Bonet, C.; Garrido-Alvarez, J.L.; Martinez-Blanco, D.; Gorria, P.; Sørby, M.H.; Riktor, M.D.; Blanco, J.A.; Hauback, B.C. Anharmonicity Effects and Thermal Expansion of Thermoelectric (M,M’,M”)NiSn (M,M’,M”=Ti, Zr, Hf) Half-Heusler Alloys. J. Alloys Compd. 2023, 959, 170583. [Google Scholar] [CrossRef]
- Graf, T.; Felser, C.; Parkin, S.S.P. Simple rules for the understanding of Heusler compounds. Prog. Solid State Chem. 2011, 39, 1–50. [Google Scholar] [CrossRef]
- Jung, D.; Kurosaki, K.; Kim, C.; Muta, H.; Yamanaka, S. Thermal Expansion and Melting Temperature of the Half-Heusler Compounds: MNiSn (M = Ti, Zr, Hf). J. Alloys Compd. 2010, 489, 328–331. [Google Scholar] [CrossRef]
- Hafner, J. Ab-Initio Simulations of Materials Using VASP: Density-Functional Theory and Beyond. J. Comput. Chem. 2008, 29, 2044–2078. [Google Scholar] [CrossRef]
- Kresse, G.; Joubert, D. From Ultrasoft Pseudopotentials to the Projector Augmented-Wave Method. Phys. Rev. B 1999, 59, 1758. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized Gradient Approximation Made Simple. Phys. Rev. Lett. 1996, 77, 3865. [Google Scholar] [CrossRef]
- Monkhorst, H.J.; Pack, J.D. Special Points for Brillouin-Zone Integrations. Phys. Rev. B 1976, 13, 5188. [Google Scholar] [CrossRef]
- Chaput, L. Direct Solution to the Linearized Phonon Boltzmann Equation. Phys. Rev. Lett. 2013, 110, 265506. [Google Scholar] [CrossRef] [PubMed]
- Togo, A.; Chaput, L.; Tadano, T.; Tanaka, I. Implementation Strategies in Phonopy and Phono3py. J. Phys. Condens. Matter 2023, 35, 353001. [Google Scholar] [CrossRef] [PubMed]
- Togo, A.; Tanaka, I. First Principles Phonon Calculations in Materials Science. Scr. Mater. 2015, 108, 1–5. [Google Scholar] [CrossRef]
- Parlinski, K.; Li, Z.Q.; Kawazoe, Y. First-Principles Determination of the Soft Mode in Cubic ZrO2. Phys. Rev. Lett. 1997, 78, 4063–4066. [Google Scholar] [CrossRef]
- Giannozzi, P.; de Gironcoli, S.; Pavone, P.; Baroni, S. Ab Initio Calculation of Phonon Dispersions in Semiconductors. Phys. Rev. B 1991, 43, 7231–7242. [Google Scholar] [CrossRef]
- Gehringer, D.; Friák, M.; Holec, D. Models of Configurationally-Complex Alloys Made Simple. Comput. Phys. Commun. 2023, 286, 108664. [Google Scholar] [CrossRef]
- Momma, K.; Izumi, F. VESTA: A Three-Dimensional Visualization System for Electronic and Structural Analysis. J. Appl. Crystallogr. 2008, 41, 653–658. [Google Scholar] [CrossRef]
- Böer, K.W.; Pohl, U.W. Phonon-Induced Thermal Properties. In Semiconductor Physics; Springer: Cham, Switzerland, 2018; pp. 151–179. [Google Scholar]
- Ashcroft, N.W.; Mermin, N.D. Solid State Physics, 1st ed.; Academic Press: Cambridge, MA, USA, 1976. [Google Scholar]
- Gürth, M.; Rogl, G.; Romaka, V.V.; Grytsiv, A.; Bauer, E.; Rogl, P. Thermoelectric High ZT Half-Heusler Alloys Ti1−x−yZrxHfyNiSn (0 ≤ x ≤ 1; 0 ≤ y ≤ 1). Acta Mater. 2016, 104, 210–222. [Google Scholar] [CrossRef]
- Murray, J.L. The Ti−Zr (Titanium-Zirconium) System. Bull. Alloy Phase Diagr. 1981, 2, 197–201. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).