Magnetic, Thermal, and Transport Properties of Co2Ti1.5Sn0.5 Heusler Alloy
Abstract
1. Introduction
2. Materials and Methods
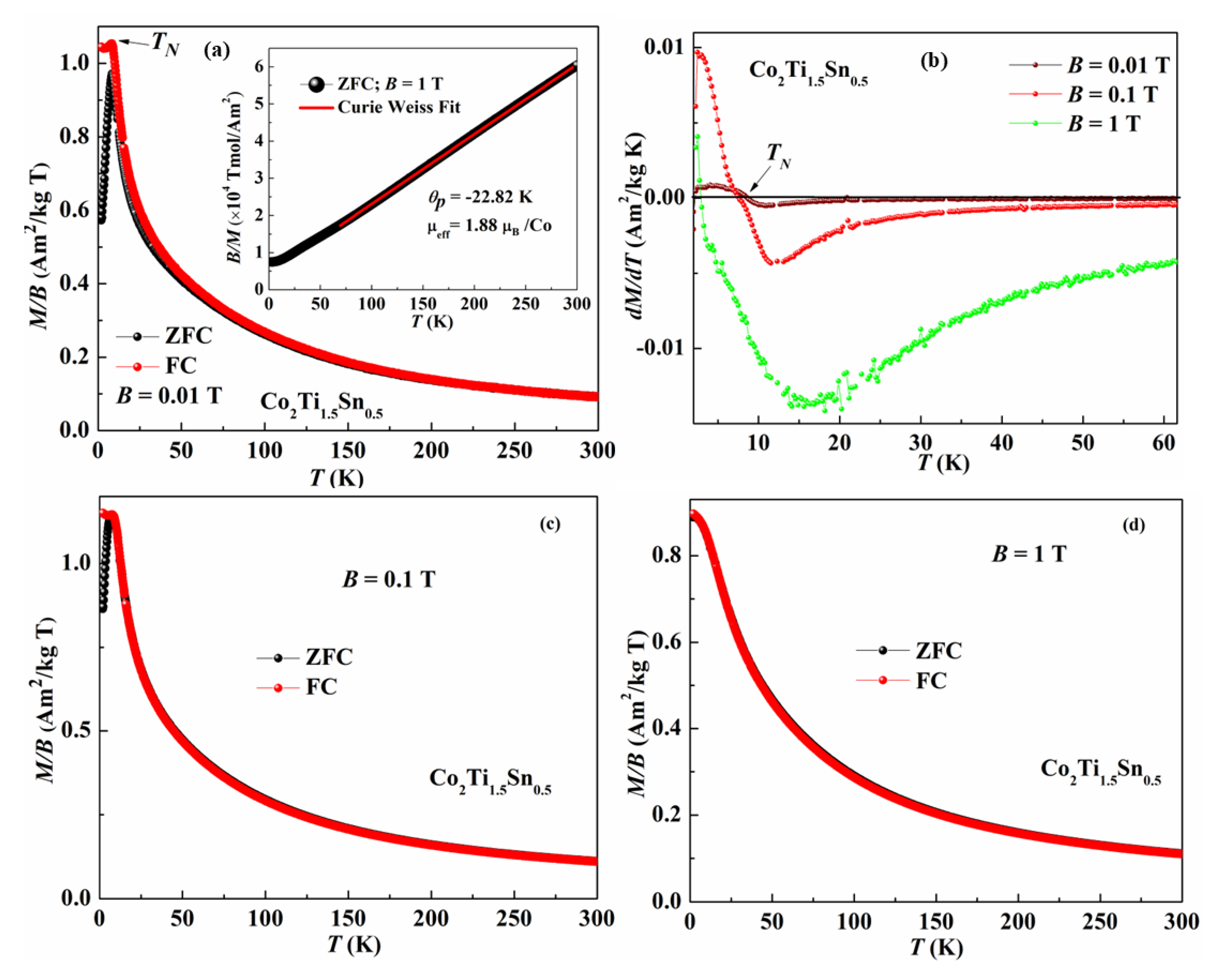
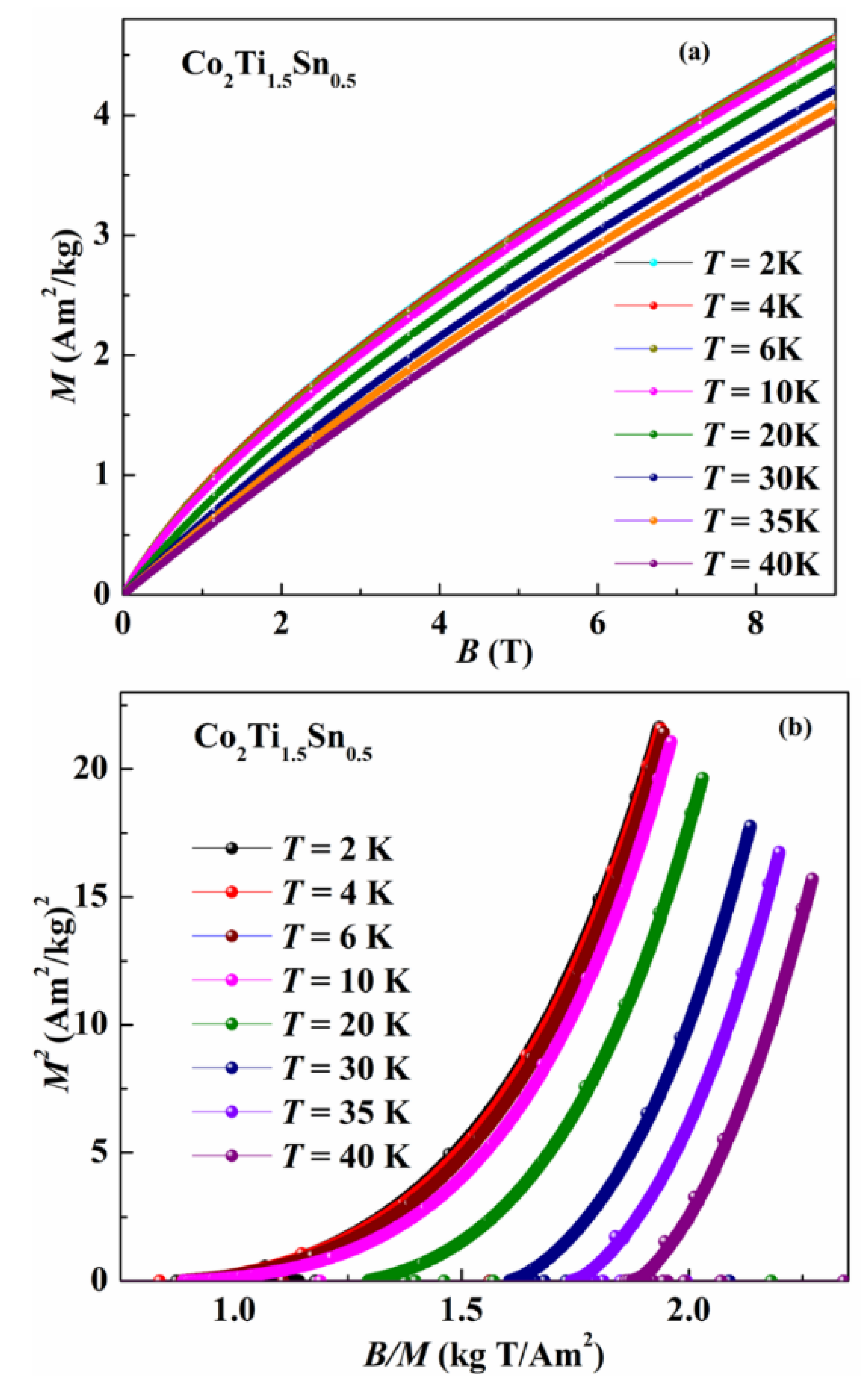
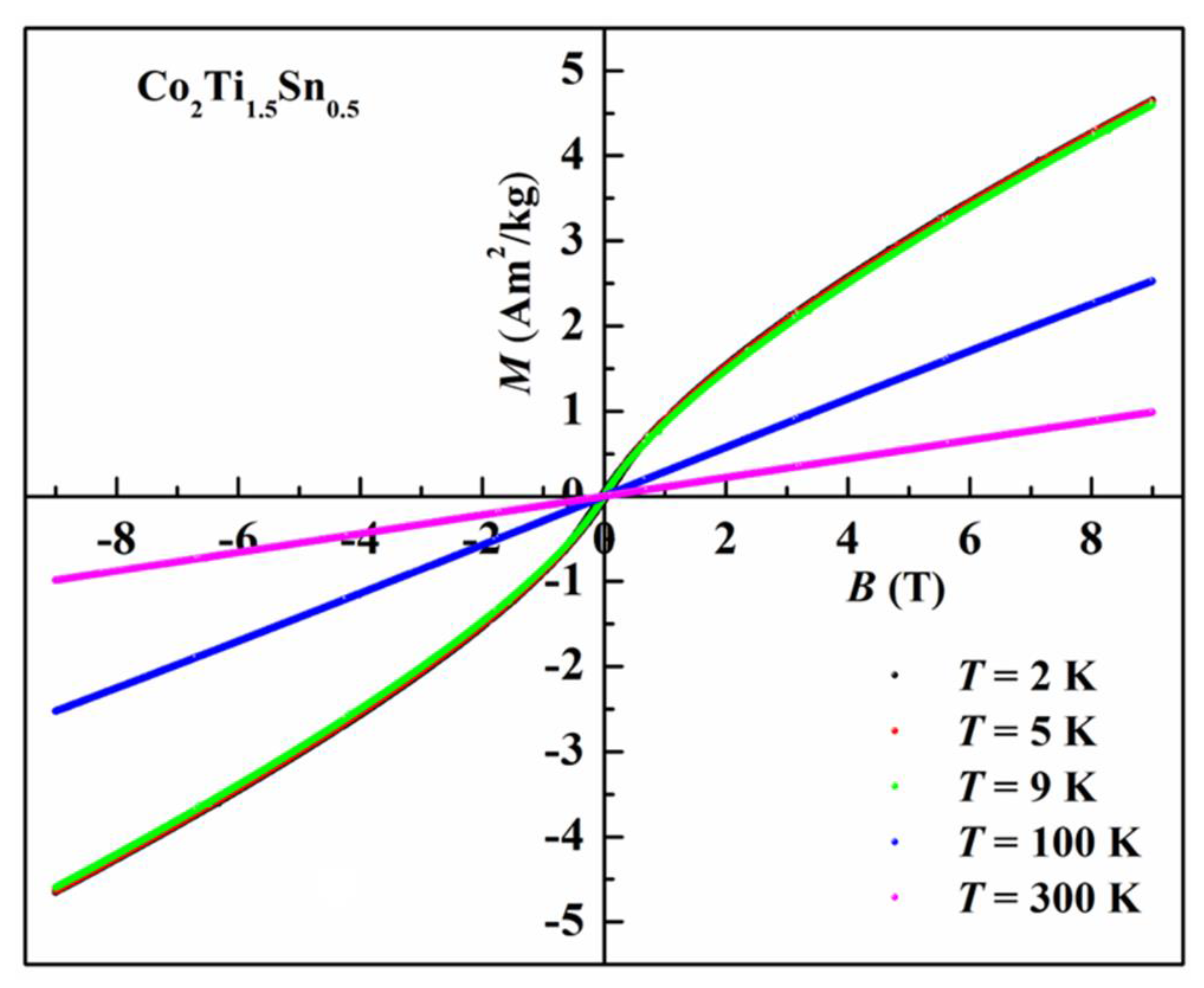
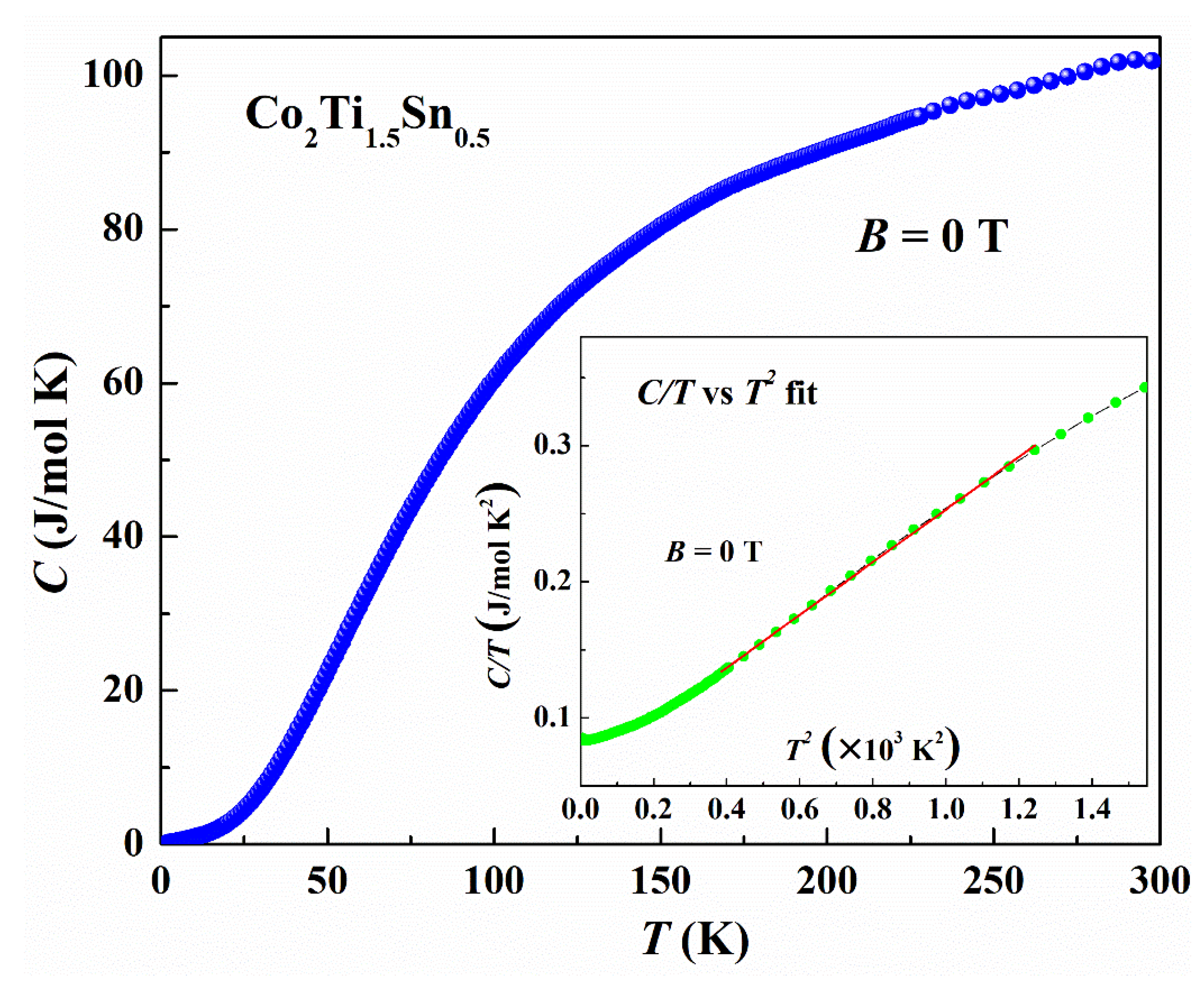
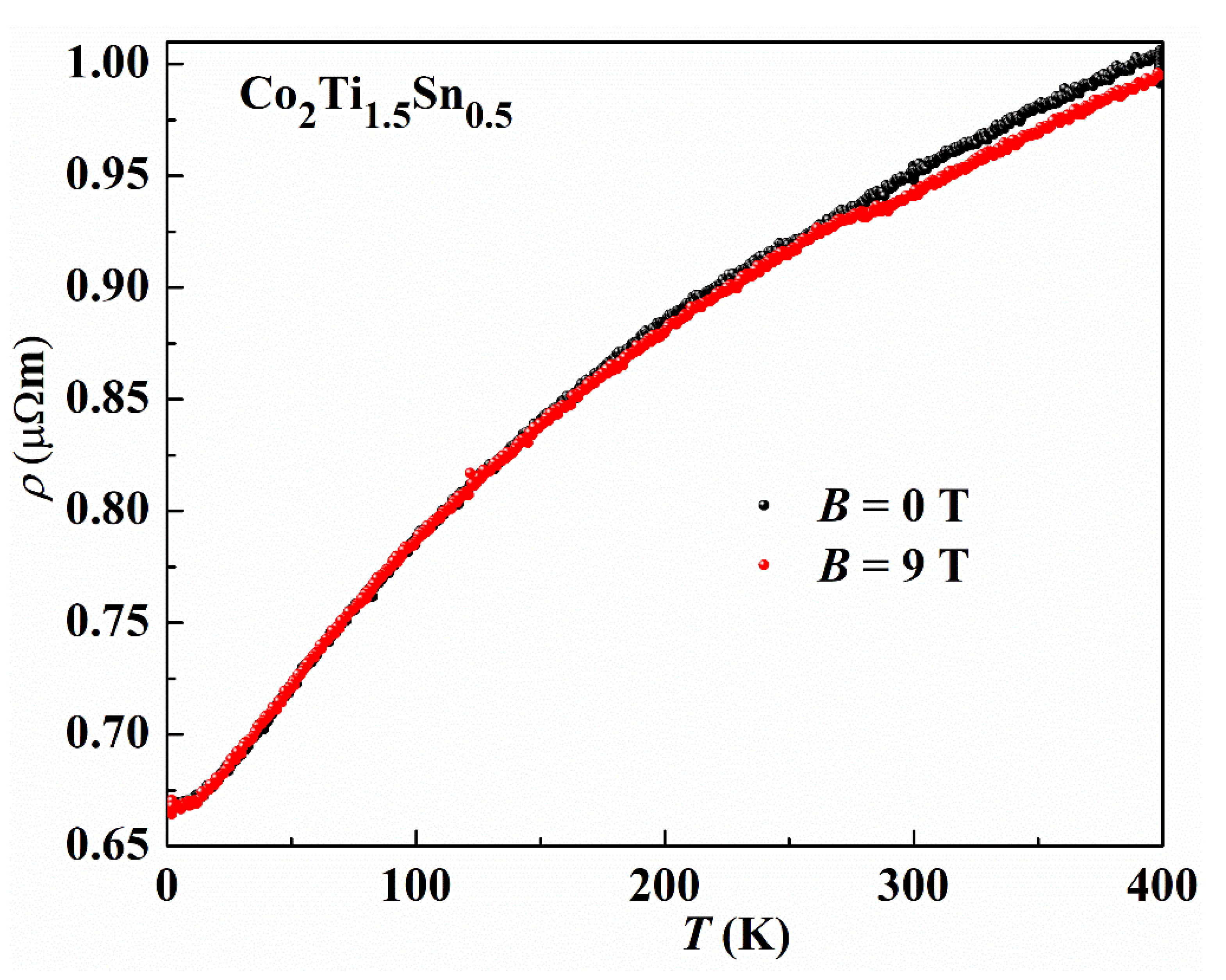
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Varaprasad, B.; Srinivasan, A.; Takahashi, Y.; Hayashi, M.; Rajanikanth, A.; Hono, K. Spin polarization and Gilbert damping of Co2Fe(GaxGe1−x) Heusler alloys. Acta Mater. 2012, 60, 6257–6265. [Google Scholar] [CrossRef]
- Bainsla, L.; Mallick, A.I.; Raja, M.M.; Nigam, A.K.; Varaprasad, B.S.D.C.S.; Takahashi, Y.K.; Alam, A.; Suresh, K.G.; Hono, K. Spin gapless semiconducting behavior in equiatomic quaternary CoFeMnSi Heusler alloy. Phys. Rev. B-Condens. Matter Mater. Phys. 2015, 91, 104408. [Google Scholar] [CrossRef]
- Kubota, H.; Nakata, J.; Oogane, M.; Ando, Y.; Sakuma, A.; Miyazaki, T. Large Magnetoresistance in Magnetic Tunnel Junctions Using Co-Mn-Al Full Heusler Alloy. Jpn. J. Appl. Phys. 2004, 43, L984–L986. [Google Scholar] [CrossRef]
- Feng, Y.; Ding, H.; Li, X.; Wu, B.; Chen, H. Spin Transport Properties of Highly Lattice- Matched All-Heusler-Alloy Magnetic Tunnel Junction Spin Transport Properties of Highly Lattice-Matched All-Heusler-Alloy Magnetic Tunnel Junction. J. Applied Phys. 2022, 131, 133901. [Google Scholar] [CrossRef]
- Sakuraba, Y.; Iwase, T.; Saito, K.; Mitani, S.; Takanashi, K. Enhancement of spin-asymmetry by L21-ordering in Co2MnSi/Cr/Co2MnSi current-perpendicular-to-plane magnetoresistance devices. Appl. Phys. Lett. 2009, 94, 012511. [Google Scholar] [CrossRef]
- Liu, J.; Xu, Z.; Xu, J.; Zuo, S.; Zhang, Y.; Liu, D.; Zheng, X.; Wang, L.; Zhao, T.; Hu, F.; et al. Multiple Transitions and Wide Refrigeration Temperature Range in R3NiSi2 (R = Tb, Dy) Compounds. J. Magn. Magn. Mater. 2020, 502, 166551. [Google Scholar] [CrossRef]
- Wang, W.; Liu, E.; Kodzuka, M.; Sukegawa, H.; Wojcik, M.; Jedryka, E.; Wu, G.H.; Inomata, K.; Mitani, S.; Hono, K. Coherent Tunneling and Giant Tunneling Magnetoresistance in Co2FeAl/MgO/CoFe Magnetic Tunneling Junctions. Phys. Rev. B 2010, 81, 1–4. [Google Scholar] [CrossRef]
- Nakatani, T.M.; Furubayashi, T.; Kasai, S.; Sukegawa, H.; Takahashi, Y.K.; Mitani, S.; Hono, K. Bulk and interfacial scatterings in current-perpendicular-to-plane giant magnetoresistance with Co2Fe(Al0.5Si0.5) Heusler alloy layers and Ag spacer. Appl. Phys. Lett. 2010, 96, 212501. [Google Scholar] [CrossRef]
- Cui, Z.; Ding, H.; Xu, K.; Feng, Y. Electronic Structures of Various (001) and (111) Surfaces, Interfaces and Spin Transport Properties of Half-Metallic Fully Compensated Ferrimagnet Cr2Se. J. Alloys Compd. 2021, 884, 5–13. [Google Scholar] [CrossRef]
- Cui, Z.; Ding, H.; Feng, Y. Investigation of the half-metallicity, magnetism and spin transport properties of double half-Heusler alloys Mn2CoCrZ2 (Z = P, As). Phys. Chem. Chem. Phys. 2021, 23, 17984–17991. [Google Scholar] [CrossRef] [PubMed]
- Barth, J.; Fecher, G.H.; Balke, B.; Ouardi, S.; Graf, T.; Felser, C.; Shkabko, A.; Weidenkaff, A.; Klaer, P.; Elmers, H.J.; et al. Itinerant Half-Metallic Ferromagnets Co2TiZ (Z=Si, Ge, Sn ): Ab Initio Calculations and Measurement of the Electronic Structure and Transport Properties. Phys. Rev. B Condens. Matter Mater. Phys. 2010, 81, 1–20. [Google Scholar] [CrossRef]
- Graf, T.; Barth, J.; Balke, B.; Populoh, S.; Weidenkaff, A.; Felser, C. Tuning the Carrier Concentration for Thermoelectrical Application in the Quaternary Heusler Compound Co2TiAl1-xSiX. Scr. Mater. 2010, 63, 925–928. [Google Scholar] [CrossRef]
- Okubo, A.; Umetsu, R.Y.; Kainuma, R.; Ishida, K. Magnetic Properties and Phase Stability of Co2(Ti1-xMnx)Ga Heusler Alloys. J. Phys. Conf. Ser. 2010, 200, 14–18. [Google Scholar] [CrossRef]
- Nakatsu, R.; Hiroi, M.; Tareuchi, N.; Kawakami, M. Transport and Magnetic Properties of the Half-Heusler Compounds CoTi1-xVxSb. AIP Conf. Proc. 2006, 850, 1247–1248. [Google Scholar] [CrossRef]
- Waterman, E.H.; Franse, J.J.M. Magnetic and Electronic Properties of CoTi1-xAlx Compounds. J. Phys. F Met. Phys. 1980, 10, 947–958. [Google Scholar] [CrossRef]
- Ooiwa, K. The Magnetic Moment of Co in a Heusler Alloy Co2TiGa and a Pseudobinary Alloy System CoTi1-yGaY. J. Phys. Soc. Japan 1985, 54, 1581–1591. [Google Scholar] [CrossRef]
- Mostefa, Z.; Cherifi, F.; Boukra, A.; Meghoufel, Z.F.; Boukortt, A. Martensitic Phase Transformations in Quaternary Heusler Alloys Co2Ti1-xFexAs. Acta Phys. Pol. A 2021, 140, 175–180. [Google Scholar] [CrossRef]
- Rani, D.; Suresh, K.G.; Alam, A. Effect of Fe Substitution for Ti on the Structural and Magnetic Properties of Half-Metallic Ferromagnet Co2TiSn. AIP Conf. Proc. 2018, 1953, 1–5. [Google Scholar] [CrossRef]
- Majumdar, S.; Chattopadhyay, M.K.; Sharma, V.K.; Sokhey, K.J.S.; Roy, S.B.; Chaddah, P. Transport Properties of the Ferromagnetic Heusler Alloy Co2TiSn. Phys. Rev. B-Condens. Matter Mater. Phys. 2005, 72, 1–4. [Google Scholar] [CrossRef]
- van Engen, P.G.; Buschow, K.H.J.; Erman, M. Magnetic Properties and Magneto-Optical Spectroscopy of Heusler Alloys Based on Transition Metals and Sn. J. Magn. Magn. Mater. 1983, 30, 374–382. [Google Scholar] [CrossRef]
- Remya, U.D.; Athul, S.R.; Arun, K.; Swathi, S.; Dzubinska, A.; Reiffers, M.; Ramamoorthi, N. Investigations on Magnetic, Magnetocaloric and Transport Properties of Co2Ti1-xSn1+x(x = 0.25, 0.5) Heusler Alloys. AIP Conf. Proc. 2021, 2369, 1–6. [Google Scholar] [CrossRef]
- Rodríguez-Carvajal, J. Recent Advances in Magnetic Structure Determination by Neutron Powder Diffraction. Phys. B Condens. Matter 1993, 192, 55–69. [Google Scholar] [CrossRef]
- Aryal, A.; Bakkar, S.; Samassekou, H.; Pandey, S.; Dubenko, I.; Stadler, S.; Ali, N.; Mazumdar, D. Mn2FeSi: An antiferromagnetic inverse-Heusler alloy. J. Alloy. Compd. 2020, 823, 153770. [Google Scholar] [CrossRef]
- Banerjee, B.K. On a Generalised Approach to First and Second Order Magnetic Transitions. Phys. Lett. 1964, 12, 16–17. [Google Scholar] [CrossRef]
- Duc, N.H.; Kim Anh, D.T.; Brommer, P.E. Metamagnetism, Giant Magnetoresistance and Magnetocaloric Effects in RCo2-Based Compounds in the Vicinity of the Curie Temperature. Phys. B Condens. Matter 2002, 319, 1–8. [Google Scholar] [CrossRef]
- Nehla, P.; Kareri, Y.; Gupt, G.D.; Hester, J.; Babu, P.D.; Ulrich, C.; Dhaka, R.S. Neutron Diffraction and Magnetic Properties of Co2Cr1-xTixAl Heusler Alloys. Phys. Rev. B 2019, 100, 1–11. [Google Scholar] [CrossRef]
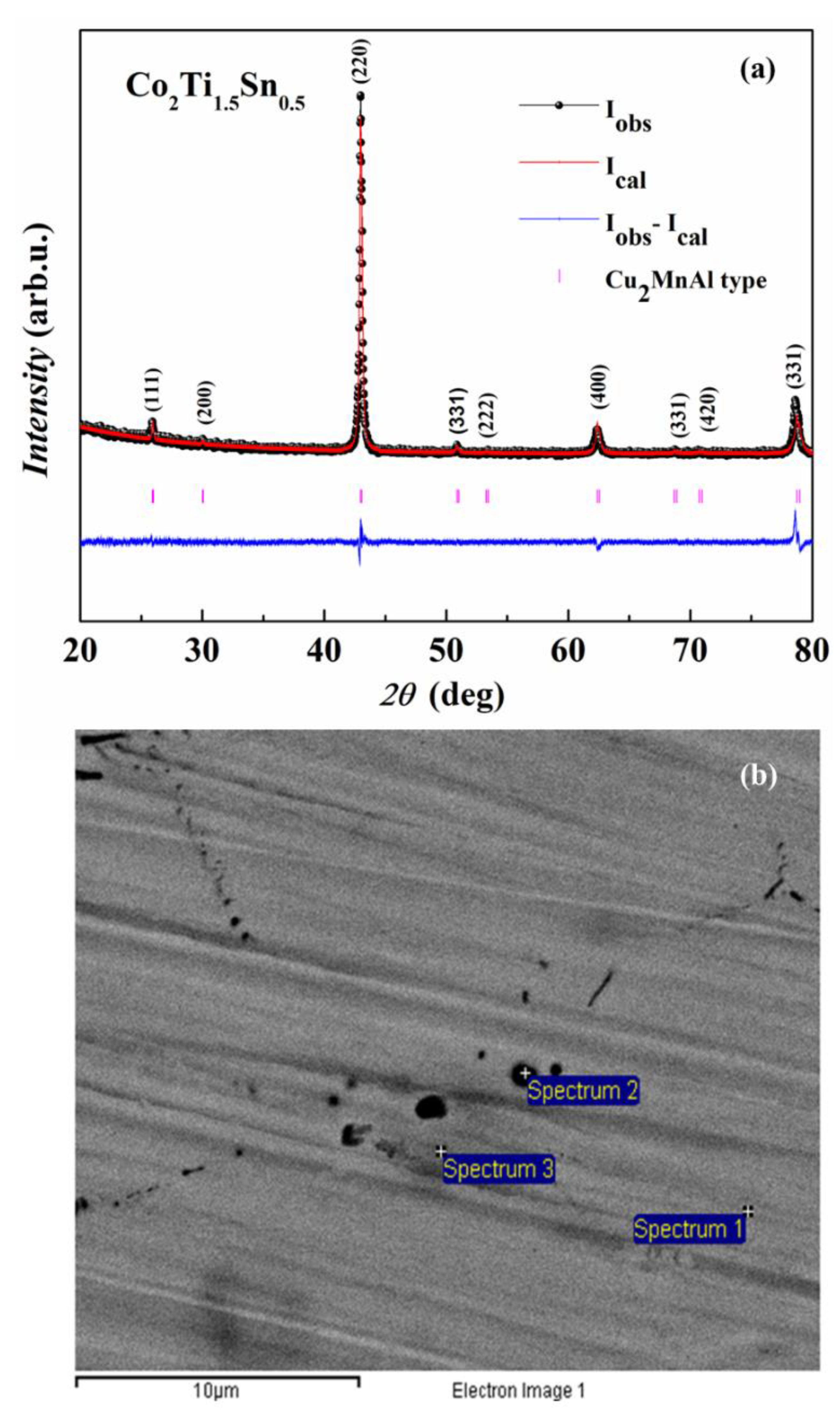
| Atomic % (Error %) | |||
|---|---|---|---|
| Spectrum 1 | Co | Ti | Sn |
| 49.3 (2.9) | 38.7 (3.2) | 12 (4.3) | |
| Spectrum 2 | 50 (2.8) | 38.4 (3.2) | 11.7 (4.2) |
| Spectrum 3 | 46.7 (3.2) | 39.3 (3.6) | 14 (4.6) |
| Spectrum Mean | 48.4 | 38.8 | 12.6 |
| Composition | Co2Ti1.55Sn0.48 | ≈Co2Ti1.5Sn0.5 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Athul, S.R.; Arun, K.; Swathi, S.; Remya, U.D.; Dzubinska, A.; Reiffers, M.; Nagalakshmi, R. Magnetic, Thermal, and Transport Properties of Co2Ti1.5Sn0.5 Heusler Alloy. Alloys 2022, 1, 254-262. https://doi.org/10.3390/alloys1030016
Athul SR, Arun K, Swathi S, Remya UD, Dzubinska A, Reiffers M, Nagalakshmi R. Magnetic, Thermal, and Transport Properties of Co2Ti1.5Sn0.5 Heusler Alloy. Alloys. 2022; 1(3):254-262. https://doi.org/10.3390/alloys1030016
Chicago/Turabian StyleAthul, Sunitha Raveendran, Kumar Arun, Saktivel Swathi, Uralath Dhanavardhanan Remya, Andrea Dzubinska, Marian Reiffers, and Ramamoorti Nagalakshmi. 2022. "Magnetic, Thermal, and Transport Properties of Co2Ti1.5Sn0.5 Heusler Alloy" Alloys 1, no. 3: 254-262. https://doi.org/10.3390/alloys1030016
APA StyleAthul, S. R., Arun, K., Swathi, S., Remya, U. D., Dzubinska, A., Reiffers, M., & Nagalakshmi, R. (2022). Magnetic, Thermal, and Transport Properties of Co2Ti1.5Sn0.5 Heusler Alloy. Alloys, 1(3), 254-262. https://doi.org/10.3390/alloys1030016