Multidimensional In Situ Characterization of Surface and Free Nanobubbles in Oxidic Nanofluids
Abstract
1. Introduction
2. Materials and Methods
2.1. Overall Experimental Setup
2.2. Experimental System to Produce Nanobubbles
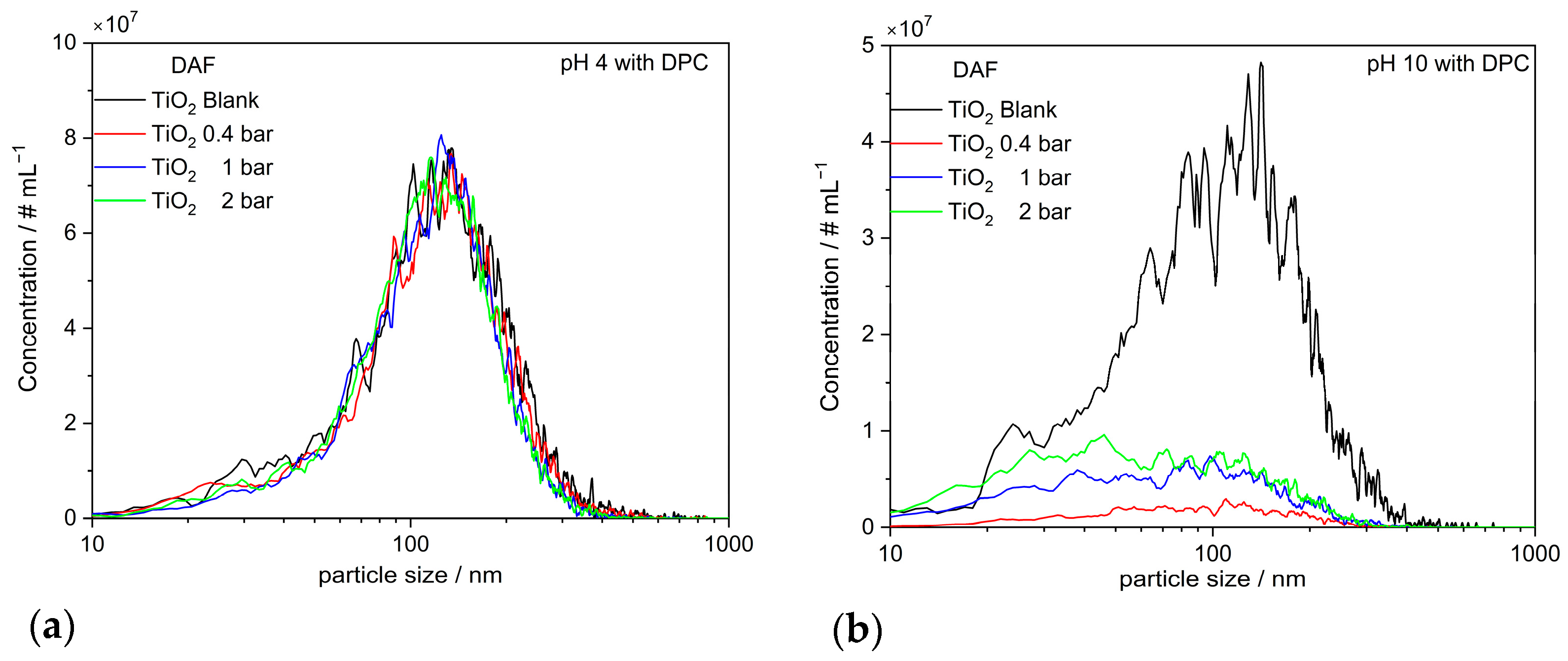
2.3. Flotation Experiments
2.4. Ultrasonic (US) Treatment
2.5. Characterization of Nanoparticle and Nanobubble Suspensions
3. Results
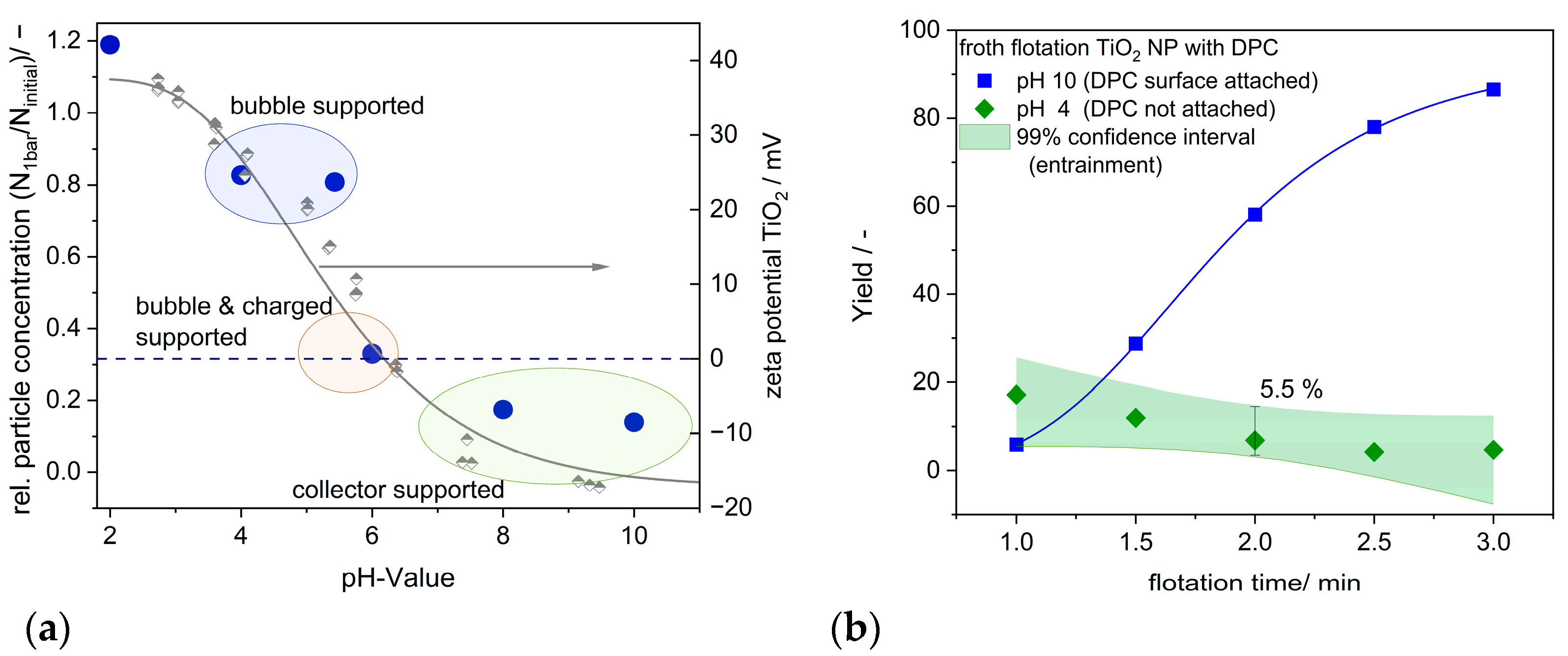
3.1. Background and Homogeneous NB Nucleation
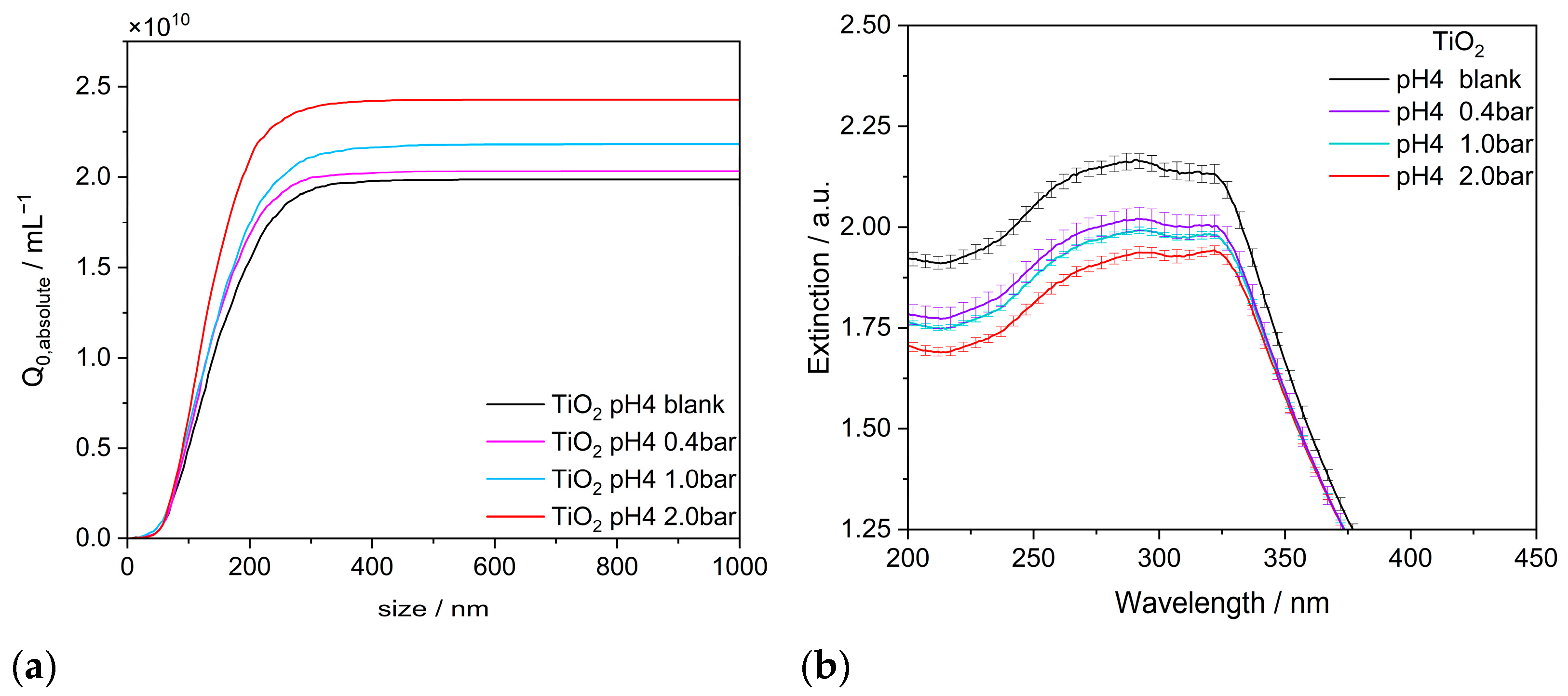
3.2. Formation of Surface Nanobubbles
3.3. Mechanical Stability of NP-SNB Clusters—The Impact of Ultrasonic Treatment
3.4. Flotation Efficiency of Nanoparticles
4. Discussion and Possible Fields of Application
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Symbols and Abbreviations
| projection area of an aggregate (averaged over all orientations) | m2 | |
| cross-section of a primary particle with attached air | m2 | |
| projection area of primary particles | m2 | |
| , a | radius of primary particles | m |
| particle number concentration | m−3 | |
| cv | volume concentration | m3 m−3 |
| cv_BNB | volume concentration of bulk nanobubbles | m3 m−3 |
| cv_SNB | volume concentration of surface nanobubbles | m3 m−3 |
| fractal dimension | - | |
| particle diameter | m | |
| primary particle diameter | m | |
| hydrodynamic equivalent diameter through the attached air | m | |
| extinction | - | |
| factor | - | |
| extinction coefficient | - | |
| buoyancy force of an aggregate | N | |
| drag force of an aggregate | N | |
| centrifugal force of an aggregate | N | |
| buoyancy force of a primary particle | N | |
| drag force of a primary particle | N | |
| centrifugal force of a primary particle | N | |
| factor | - | |
| structure factor | - | |
| ,h | thickness of the air-shell | m |
| intensity of the transmitted light | Wm−2 | |
| intensity of the incident light | Wm−2 | |
| wave number | m−1 | |
| complex refractive index of solids relative to that of the surrounding medium | - | |
| number of (primary) particles | - | |
| Q0 | cumulative number distribution (here: not normalized) | m−3 |
| Qabs | efficiency factor for absorption | - |
| Qext | efficiency factor for extinction | - |
| Qsca | efficiency factor for scattering | - |
| radius of gyration | m | |
| radius of a cylindrical gas volume | m | |
| optical path length through the cuvette | m | |
| attached air volume on an aggregate | m3 | |
| volume of the primary particle | m3 | |
| wsettl,eff | settling velocity of an aggregate with attached air | m s−1 |
| wsettl,0 | settling velocity of an aggregate without attached air | m s−1 |
| settling velocity of primary particle | m s−1 | |
| ,z | half-length of a cylindrical gas volume | m |
| α | absorption coefficient | - |
| dynamic viscosity of the medium | Pas | |
| particle density | kg m−3 | |
| medium density | kg m−3 | |
| absorption cross-section of aggregates | m2 | |
| absorption cross-section of primary particle | m2 | |
| scattering cross-section of aggregates | m2 | |
| scattering cross-section of primary particle | m2 | |
| angular velocity | rad s−1 |
| BNB | bulk nanobubbles (free nanobubbles in the surrounding liquid) |
| BET | specific surface area (Brunauer–Emmett–Teller) |
| CCD | charged coupled device |
| cmc | critical micelle concentration [molL−1] |
| CPC | condensation particle counter (manufacturer: Grimm Aerosol Technik) |
| DAF | dissolved air flotation |
| DPC | dodecyl-pyridinium-chloride |
| HCl | hydrochloric acid |
| ICP-OES | inductively coupled plasma optical emission spectrometry |
| LumiFuge® | analytical centrifuge (manufacturer: LUM GmbH) |
| NaOH | sodium hydroxide |
| NB | nanobubble |
| NP | nanoparticle |
| NTA | nanoparticle tracking analysis (manufacturer: Particle Metrix) |
| P 25 | titania nanopowder (manufacturer: Evonik) |
| PS | polystyrene |
| SNB | surface nanobubbles attached to a particle’s surface |
| STXM | synchrotron-based scanning transmission X-ray microscopy |
| TEM | transmission electron microscopy |
| US | ultrasonic |
| UV–vis | ultraviolet–visible spectrophotometry |
| ZP | zeta potential |
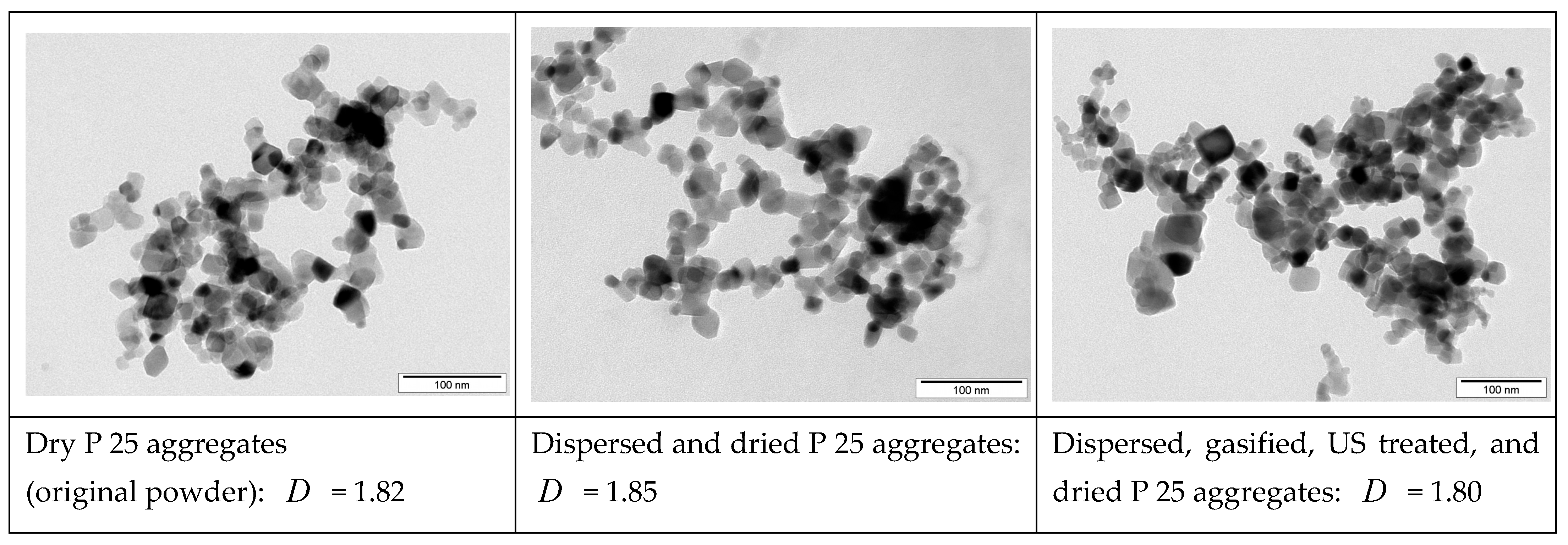
Appendix A. Morphology of TiO2 Nanoparticle Aggregates
Appendix B. Settling Velocity of Gas-Treated Agglomerates

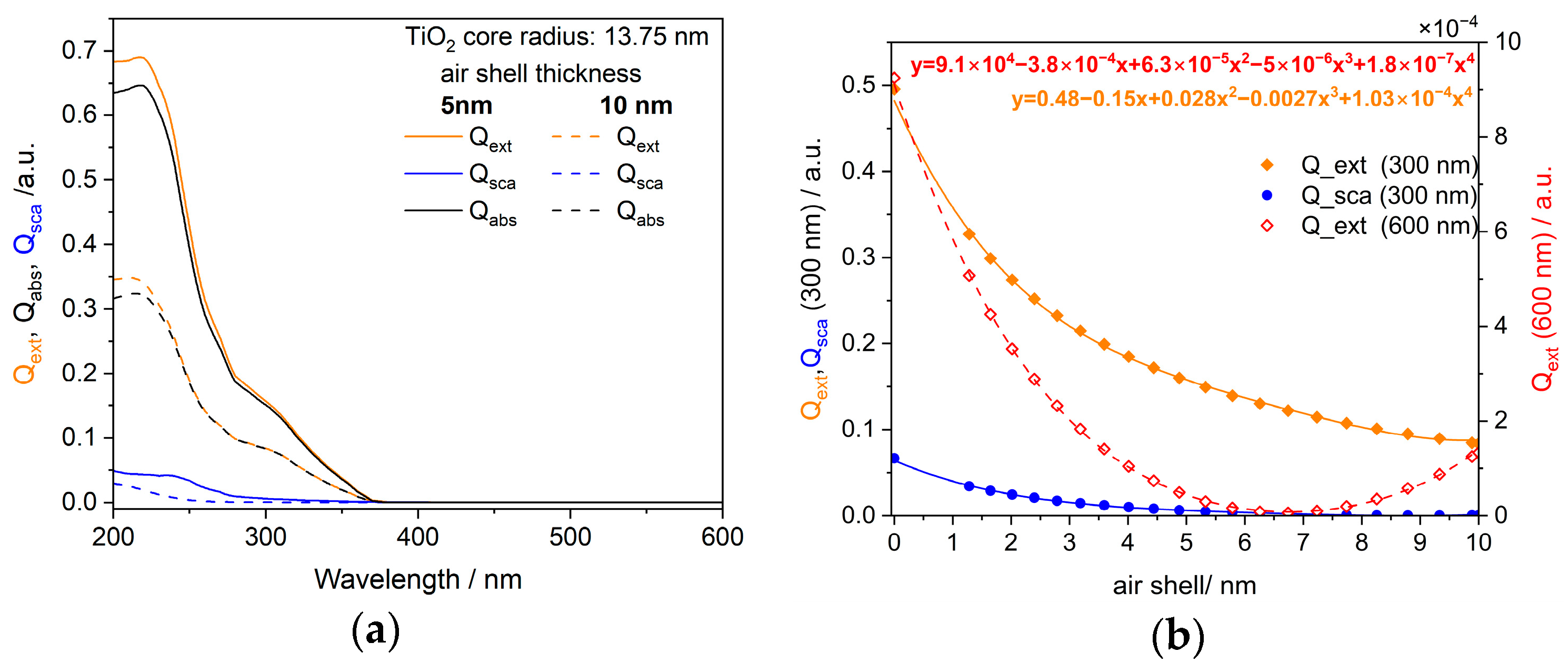
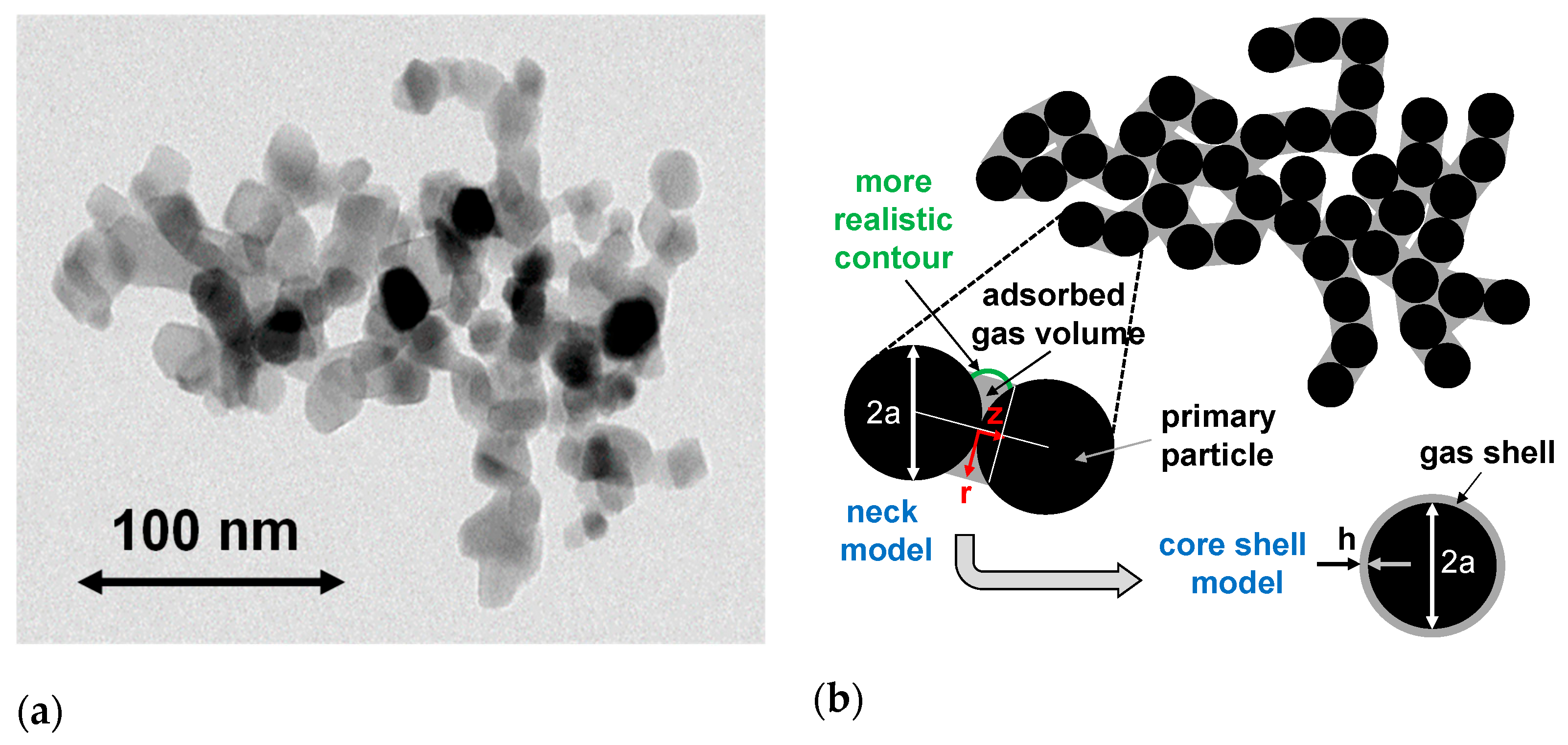
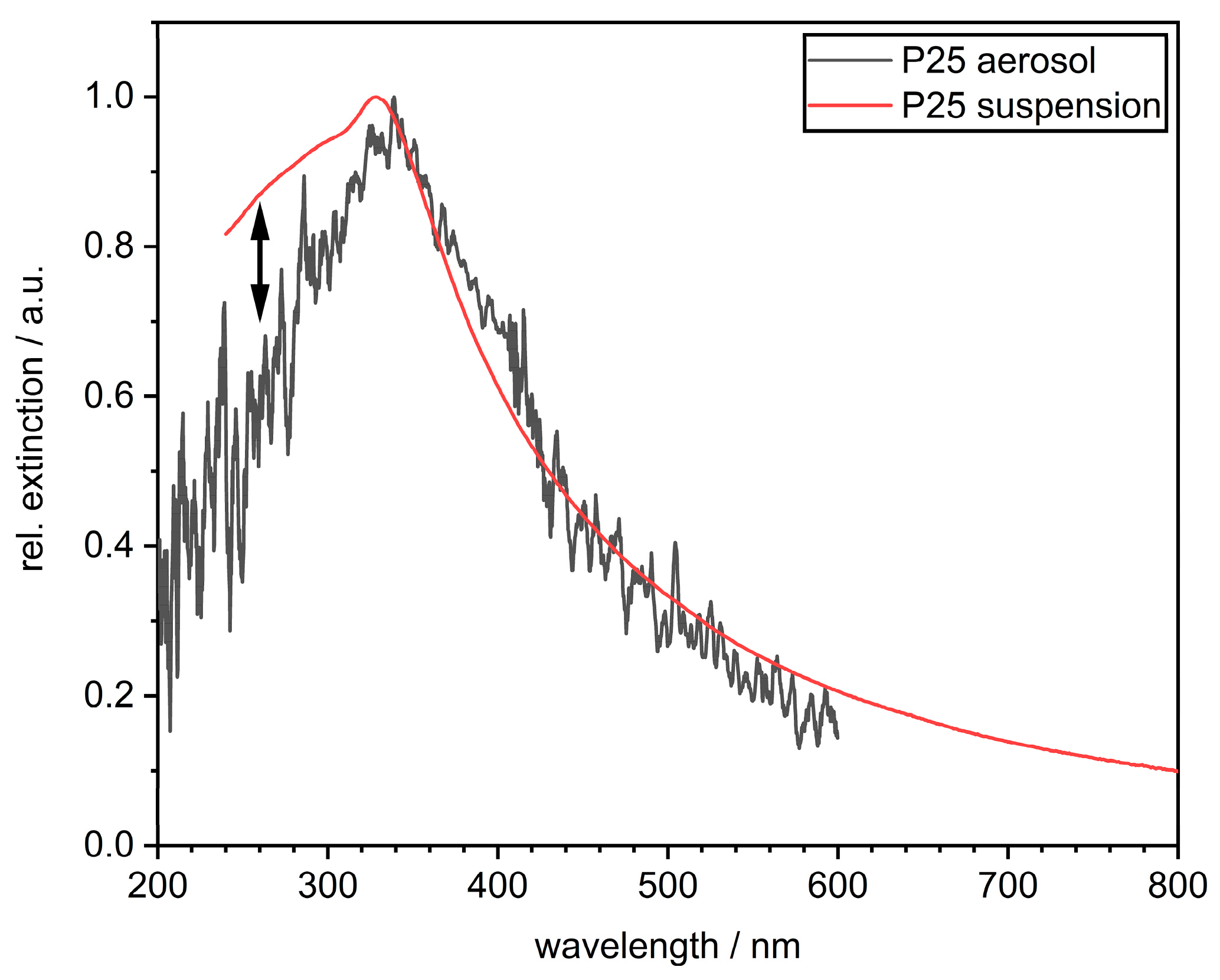
Appendix C. Extinction Behavior of Nanoparticle Aggregates and Comparison Neck to the Core–Shell Model

Appendix D. Zeta Potential of TiO2 with DPC at Different pH Values

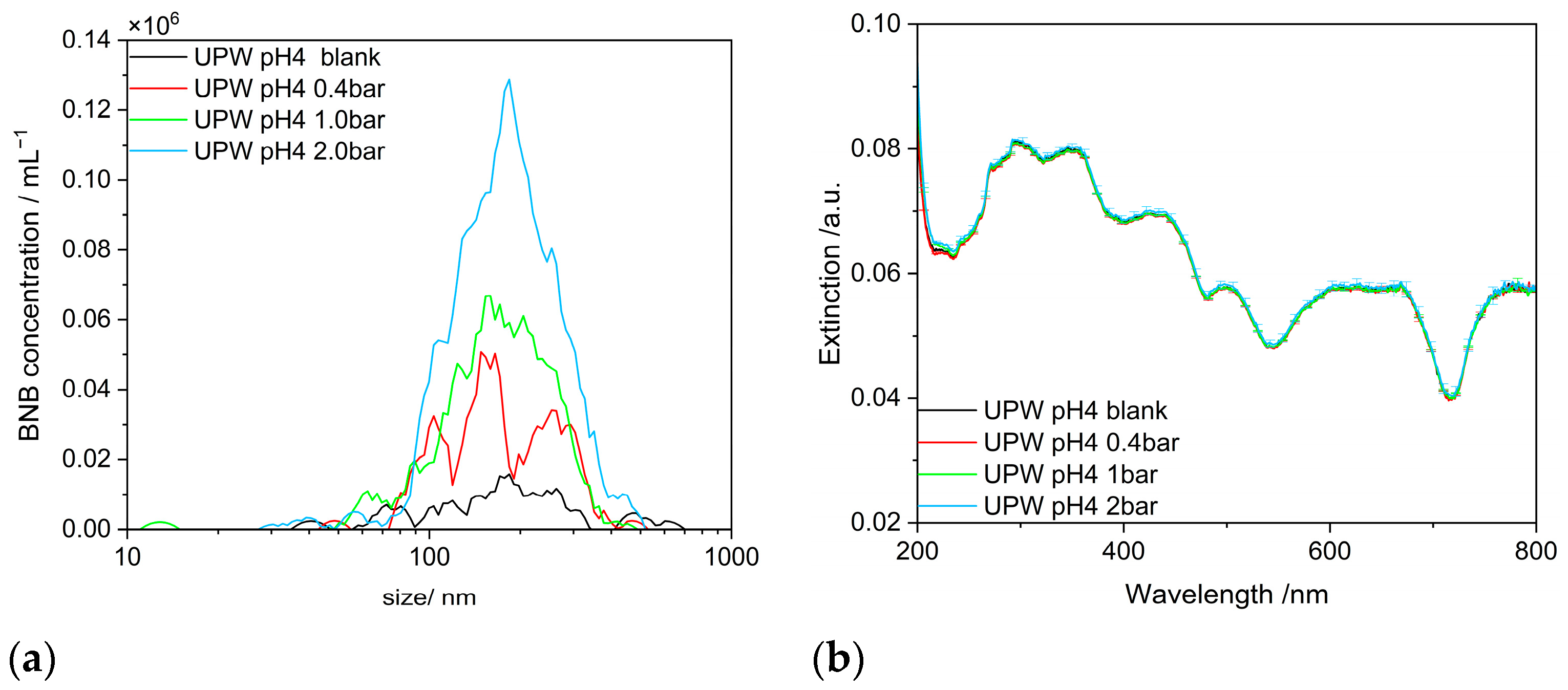
Appendix E. TiO2 Samples After Gas Treatment at Different Pressures

Appendix F. Zeta Potential of Free Nanobubbles (BNBs)

References
- Gu, Y.; Xia, K.; Wu, D.; Mou, J.; Zheng, S. Technical Characteristics and Wear-Resistant Mechanism of Nano Coatings: A Review. Coatings 2020, 10, 233. [Google Scholar] [CrossRef]
- Parthasarathy, M. Challenges and Emerging Trends in Toner Waste Recycling: A Review. Recycling 2021, 6, 57. [Google Scholar] [CrossRef]
- Masuhr, M.; Kruis, F.E. Fractionation of Aerosols by Particle Size and Material Composition Using a Classifying Aerodynamic Lens. Powders 2024, 3, 392–415. [Google Scholar] [CrossRef]
- Giesler, J.; Weirauch, L.; Thöming, J.; Baune, M.; Pesch, G.R. Separating Microparticles by Material and Size Using Dielectrophoretic Chromatography with Frequency Modulation. Sci. Rep. 2021, 11, 16861. [Google Scholar] [CrossRef]
- Farrokhpay, S.; Filippov, L.; Fornasiero, D. Flotation of Fine Particles: A Review. Miner. Process. Extr. Metall. Rev. 2021, 42, 473–483. [Google Scholar] [CrossRef]
- Karakashev, S.; Grozev, N.; Ozdemir, O.; Guven, O.; Ata, S.; Bournival, G.; Batjargal, K.; Boylu, F.; Hristova, S.; Çelik, M. Physical Restrictions of the Flotation of Fine Particles and Ways to Overcome Them. Physicochem. Probl. Miner. Process. 2022, 58, 153944. [Google Scholar] [CrossRef]
- Nguyen, A.V.; George, P.; Jameson, G.J. Demonstration of a Minimum in the Recovery of Nanoparticles by Flotation: Theory and Experiment. Chem. Eng. Sci. 2006, 61, 2494–2509. [Google Scholar] [CrossRef]
- Zhang, F.; Sun, L.; Yang, H.; Gui, X.; Schönherr, H.; Kappl, M.; Cao, Y.; Xing, Y. Recent Advances for Understanding the Role of Nanobubbles in Particles Flotation. Adv. Colloid Interface Sci. 2021, 291, 102403. [Google Scholar] [CrossRef]
- Kyzas, G.Z.; Mitropoulos, A.C.; Matis, K.A. From Microbubbles to Nanobubbles: Effect on Flotation. Processes 2021, 9, 1287. [Google Scholar] [CrossRef]
- Tao, D. Recent Advances in Fundamentals and Applications of Nanobubble Enhanced Froth Flotation: A Review. Miner. Eng. 2022, 183, 107554. [Google Scholar] [CrossRef]
- Schubert, H. Nanobubbles, Hydrophobic Effect, Heterocoagulation and Hydrodynamics in Flotation. Int. J. Miner. Process. 2005, 78, 11–21. [Google Scholar] [CrossRef]
- Attard, P. Nanobubbles and the Hydrophobic Attraction. Adv. Colloid Interface Sci. 2003, 104, 75–91. [Google Scholar] [CrossRef] [PubMed]
- Tyrrell, J.W.G.; Attard, P. Images of Nanobubbles on Hydrophobic Surfaces and Their Interactions. Phys. Rev. Lett. 2001, 87, 176104. [Google Scholar] [CrossRef]
- Ferraro, G.; Jadhav, A.J.; Barigou, M. A Henry’s Law Method for Generating Bulk Nanobubbles. Nanoscale 2020, 12, 15869–15879. [Google Scholar] [CrossRef]
- Nirmalkar, N.; Pacek, A.W.; Barigou, M. Interpreting the Interfacial and Colloidal Stability of Bulk Nanobubbles. Soft Matter 2018, 14, 9643–9656. [Google Scholar] [CrossRef]
- Jin, J.; Feng, Z.; Yang, F.; Gu, N. Bulk Nanobubbles Fabricated by Repeated Compression of Microbubbles. Langmuir 2019, 35, 4238–4245. [Google Scholar] [CrossRef]
- Meegoda, J.N.; Aluthgun Hewage, S.; Batagoda, J.H. Stability of Nanobubbles. Environ. Eng. Sci. 2018, 35, 1216–1227. [Google Scholar] [CrossRef]
- Ditscherlein, L.; Fritzsche, J.; Peuker, U.A. Study of Nanobubbles on Hydrophilic and Hydrophobic Alumina Surfaces. Colloids Surf. Physicochem. Eng. Asp. 2016, 497, 242–250. [Google Scholar] [CrossRef]
- Maheshwari, S.; van der Hoef, M.; Zhang, X.; Lohse, D. Stability of Surface Nanobubbles: A Molecular Dynamics Study. Langmuir 2016, 32, 11116–11122. [Google Scholar] [CrossRef]
- Oh, S.H.; Kim, J.M. Generation and Stability of Bulk Nanobubbles. Langmuir 2017, 33, 3818–3823. [Google Scholar] [CrossRef]
- Tan, B.H.; An, H.; Ohl, C.-D. Stability of Surface and Bulk Nanobubbles. Curr. Opin. Colloid Interface Sci. 2021, 53, 101428. [Google Scholar] [CrossRef]
- Knüpfer, P.; Ditscherlein, L.; Peuker, U.A. Nanobubble Enhanced Agglomeration of Hydrophobic Powders. Colloids Surf. Physicochem. Eng. Asp. 2017, 530, 117–123. [Google Scholar] [CrossRef]
- Olszok, V.; Rivas-Botero, J.; Wollmann, A.; Benker, B.; Weber, A.P. Particle-Induced Nanobubble Generation for Material-Selective Nanoparticle Flotation. Colloids Surf. Physicochem. Eng. Asp. 2020, 592, 124576. [Google Scholar] [CrossRef]
- Li, C.; Zhang, H. Surface Nanobubbles and Their Roles in Flotation of Fine Particles—A Review. J. Ind. Eng. Chem. 2022, 106, 37–51. [Google Scholar] [CrossRef]
- Pan, G.; He, G.; Zhang, M.; Zhou, Q.; Tyliszczak, T.; Tai, R.; Guo, J.; Bi, L.; Wang, L.; Zhang, H. Nanobubbles at Hydrophilic Particle–Water Interfaces. Langmuir 2016, 32, 11133–11137. [Google Scholar] [CrossRef]
- Wang, K.; Wei, Z.; Colbeau-Justin, C.; Nitta, A.; Kowalska, E. P 25 and Its Components—Electronic Properties and Photocatalytic Activities. Surf. Interfaces 2022, 31, 102057. [Google Scholar] [CrossRef]
- Asakura, Y.; Yasuda, K. Frequency and Power Dependence of Ultrasonic Degassing. Ultrason. Sonochem. 2022, 82, 105890. [Google Scholar] [CrossRef]
- Azevedo, A.; Etchepare, R.; Calgaroto, S.; Rubio, J. Aqueous Dispersions of Nanobubbles: Generation, Properties and Features. Miner. Eng. 2016, 94, 29–37. [Google Scholar] [CrossRef]
- Smith, P.G.; Warren, L.J. Entrainment of Particles into Flotation Froths. Miner. Process. Extr. Metall. Rev. 1989, 5, 123–145. [Google Scholar] [CrossRef]
- Kanniah, V.; Wu, P.; Mandzy, N.; Grulke, E.A. Fractal Analysis as a Complimentary Technique for Characterizing Nanoparticle Size Distributions. Powder Technol. 2012, 226, 189–198. [Google Scholar] [CrossRef]
- Binder, C.; Feichtinger, C.; Schmid, H.-J.; Thürey, N.; Peukert, W.; Rüde, U. Simulation of the Hydrodynamic Drag of Aggregated Particles. J. Colloid Interface Sci. 2006, 301, 155–167. [Google Scholar] [CrossRef] [PubMed]
- Mäntele, W.; Deniz, E. UV–VIS Absorption Spectroscopy: Lambert-Beer Reloaded. Spectrochim. Acta. A. Mol. Biomol. Spectrosc. 2017, 173, 965–968. [Google Scholar] [CrossRef] [PubMed]
- Sorensen, C.M. Light Scattering by Fractal Aggregates: A Review. Aerosol Sci. Technol. 2001, 35, 648–687. [Google Scholar] [CrossRef]
- Laven, P. MiePlot v4. 2014. Available online: http://www.philiplaven.com/mieplot (accessed on 10 February 2025).
- Haarhoff, J.; Edzwald, J.K. Dissolved Air Flotation Modelling: Insights and Shortcomings. J. Water Supply Res. Technol.-Aqua 2004, 53, 127–150. [Google Scholar] [CrossRef]
- Edzwald, J.K. Principles and Applications of Dissolved Air Flotation. Water Sci. Technol. 1995, 31, 1–23. [Google Scholar] [CrossRef]
- Zhu, P.; Dastan, D.; Liu, L.; Wu, L.; Shi, Z.; Chu, Q.-Q.; Altaf, F.; Mohammed, M.K.A. Surface Wettability of Various Phases of Titania Thin Films: Atomic-Scale Simulation Studies. J. Mol. Graph. Model. 2023, 118, 108335. [Google Scholar] [CrossRef]
- Brotchie, A.; Zhang, X.H. Response of Interfacial Nanobubbles to Ultrasound Irradiation. Soft Matter 2011, 7, 265–269. [Google Scholar] [CrossRef]
- Yang, S.; Pelton, R.; Raegen, A.; Montgomery, M.; Dalnoki-Veress, K. Nanoparticle Flotation Collectors: Mechanisms Behind a New Technology. Langmuir 2011, 27, 10438–10446. [Google Scholar] [CrossRef]
- Yang, S.; Pelton, R.; Montgomery, M.; Cui, Y. Nanoparticle Flotation Collectors III: The Role of Nanoparticle Diameter. ACS Appl. Mater. Interfaces 2012, 4, 4882–4890. [Google Scholar] [CrossRef]
- Somasundaran, P.; Fuerstenau, D.W. Mechanisms of Alkyl Sulfonate Adsorption at the Alumina-Water Interface1. J. Phys. Chem. 1966, 70, 90–96. [Google Scholar] [CrossRef]
- Scamehorn, J.F.; Schechter, R.S.; Wade, W.H. Adsorption of Surfactants on Mineral Oxide Surfaces from Aqueous Solutions. J. Colloid Interface Sci. 1982, 85, 463–478. [Google Scholar] [CrossRef]
- Takahashi, M. ζ Potential of Microbubbles in Aqueous Solutions: Electrical Properties of the Gas−Water Interface. J. Phys. Chem. B 2005, 109, 21858–21864. [Google Scholar] [CrossRef] [PubMed]
- Harvey, E.N.; Barnes, D.K.; McElroy, W.D.; Whiteley, A.H.; Pease, D.C.; Cooper, K.W. Bubble Formation in Animals. I. Physical Factors. J. Cell. Comp. Physiol. 1944, 24, 1–22. [Google Scholar] [CrossRef]
- Groß, T.F.; Pelz, P.F. Diffusion-Driven Nucleation from Surface Nuclei in Hydrodynamic Cavitation. J. Fluid Mech. 2017, 830, 138–164. [Google Scholar] [CrossRef]
- Calgaroto, S.; Azevedo, A.; Rubio, J. Flotation of Quartz Particles Assisted by Nanobubbles. Int. J. Miner. Process. 2015, 137, 64–70. [Google Scholar] [CrossRef]
- Chang, G.; Xing, Y.; Zhang, F.; Yang, Z.; Liu, X.; Gui, X. Effect of Nanobubbles on the Flotation Performance of Oxidized Coal. ACS Omega 2020, 5, 20283–20290. [Google Scholar] [CrossRef]
- Rox, H.; Ränke, F.; Mädler, J.; Marzec, M.M.; Sokołowski, K.; Baumann, R.; Hamedi, H.; Yang, X.; Mutschke, G.; Urbas, L.; et al. Boosting Electrode Performance and Bubble Management via Direct Laser Interference Patterning. ACS Appl. Mater. Interfaces 2025, 17, 9364–9377. [Google Scholar] [CrossRef]






| ICP-OES Measurement Rel. TiO2-Mass Concentration % | NTA Measurement Rel. Particle Number Concentration % | |
|---|---|---|
| blank | 100 | 100 |
| 0.4 bar | 7 | 6 |
| 1 bar | 14 | 19 |
| 2 bar | 16 | 25 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wollmann, A.; Benker, B.; Olszok, V.; Weber, A.P. Multidimensional In Situ Characterization of Surface and Free Nanobubbles in Oxidic Nanofluids. Powders 2025, 4, 7. https://doi.org/10.3390/powders4010007
Wollmann A, Benker B, Olszok V, Weber AP. Multidimensional In Situ Characterization of Surface and Free Nanobubbles in Oxidic Nanofluids. Powders. 2025; 4(1):7. https://doi.org/10.3390/powders4010007
Chicago/Turabian StyleWollmann, Annett, Bernd Benker, Vinzent Olszok, and Alfred P. Weber. 2025. "Multidimensional In Situ Characterization of Surface and Free Nanobubbles in Oxidic Nanofluids" Powders 4, no. 1: 7. https://doi.org/10.3390/powders4010007
APA StyleWollmann, A., Benker, B., Olszok, V., & Weber, A. P. (2025). Multidimensional In Situ Characterization of Surface and Free Nanobubbles in Oxidic Nanofluids. Powders, 4(1), 7. https://doi.org/10.3390/powders4010007







