Selective Multidimensional Particle Fractionation Applying Acoustic Fields
Abstract
1. Introduction
1.1. State of Science
1.2. Physical Background
1.2.1. Acoustic Waves
1.2.2. Acoustic Standing Wave Field
1.2.3. Particle Behavior in an Acoustic Field
1.3. Motivation and Preparatory Work
2. Materials and Methods
2.1. Process Scheme and General Set-Up
2.1.1. Acoustic Sources and Acoustic Field Parameter
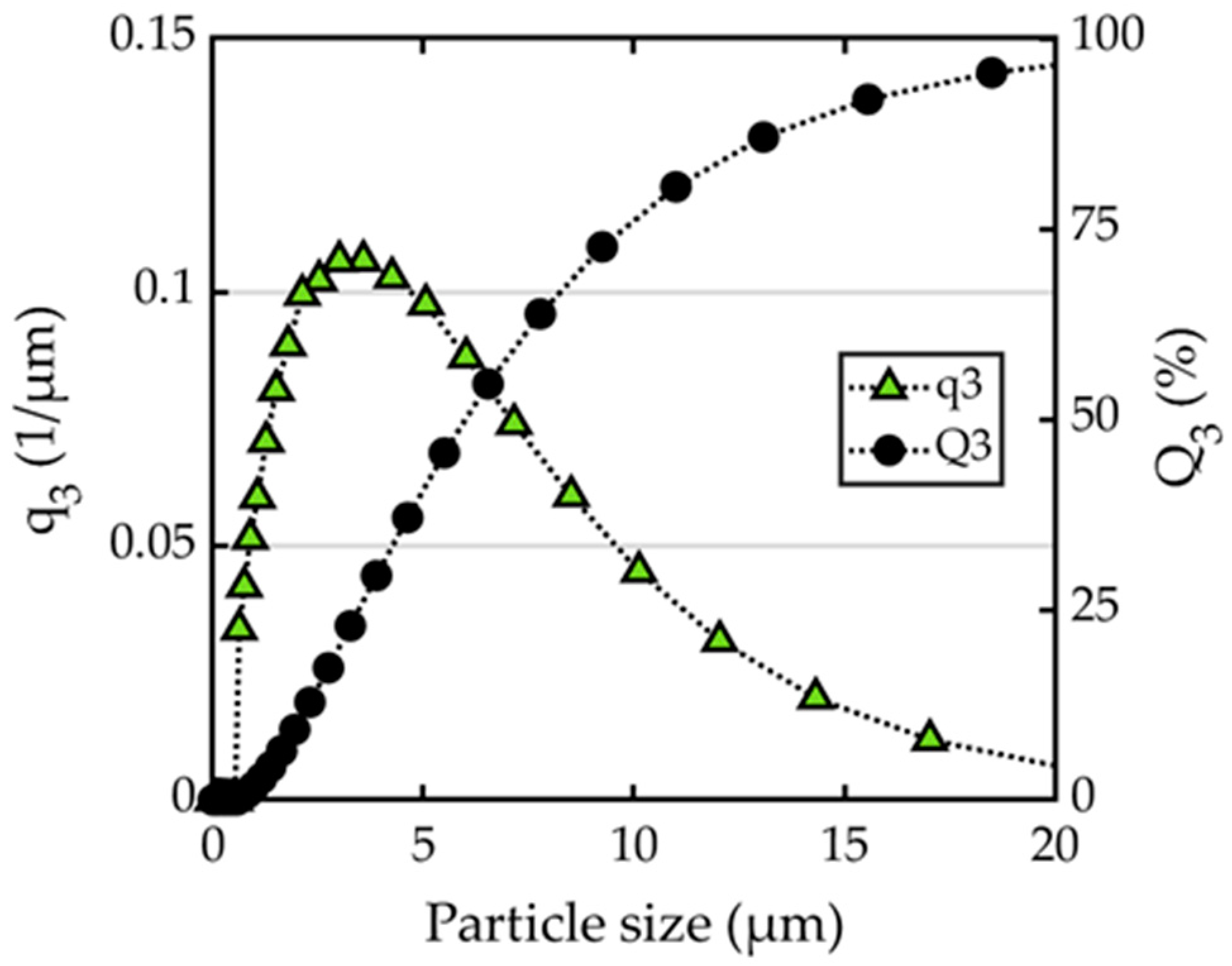
2.1.2. Particle System
2.1.3. Particle Dispersion System
2.1.4. Cross-Flow Through Experimental Setup and Aerosol Capture
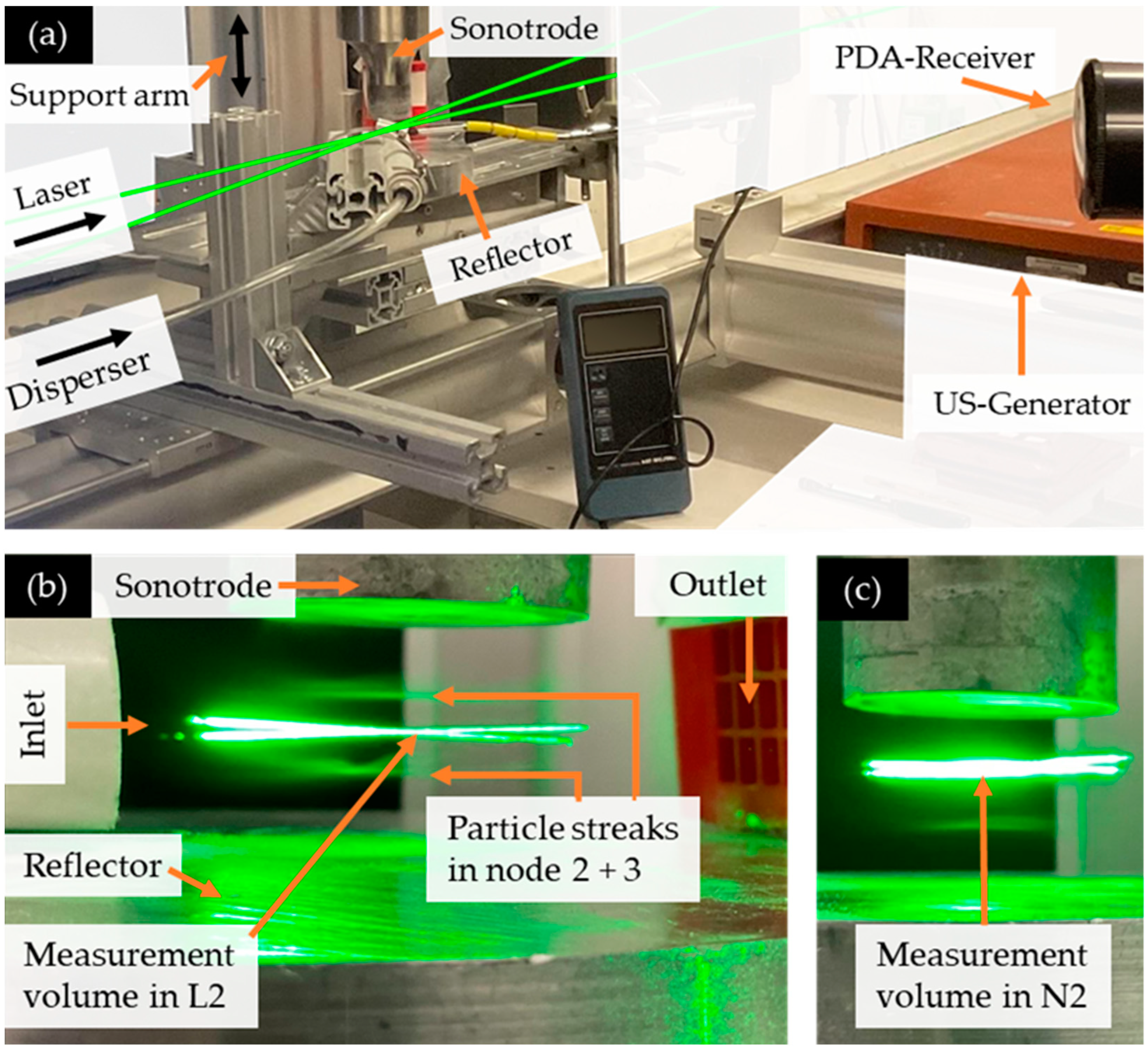
2.2. Methods and Measurement Set-Up
2.2.1. Phase Doppler Anemometry
2.2.2. PDA Measurement Procedure
2.2.3. Filter Deposition Set-Up
3. Results
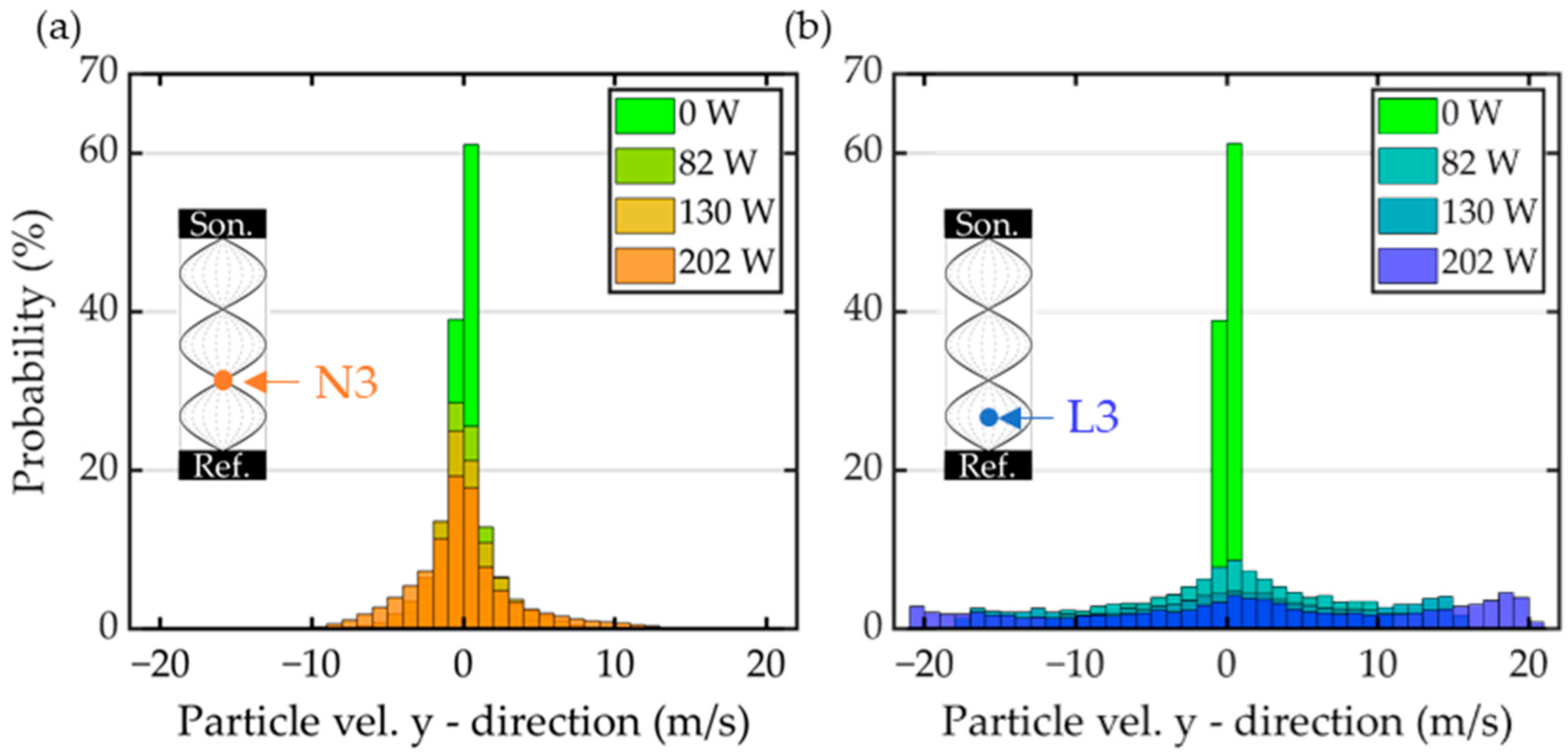
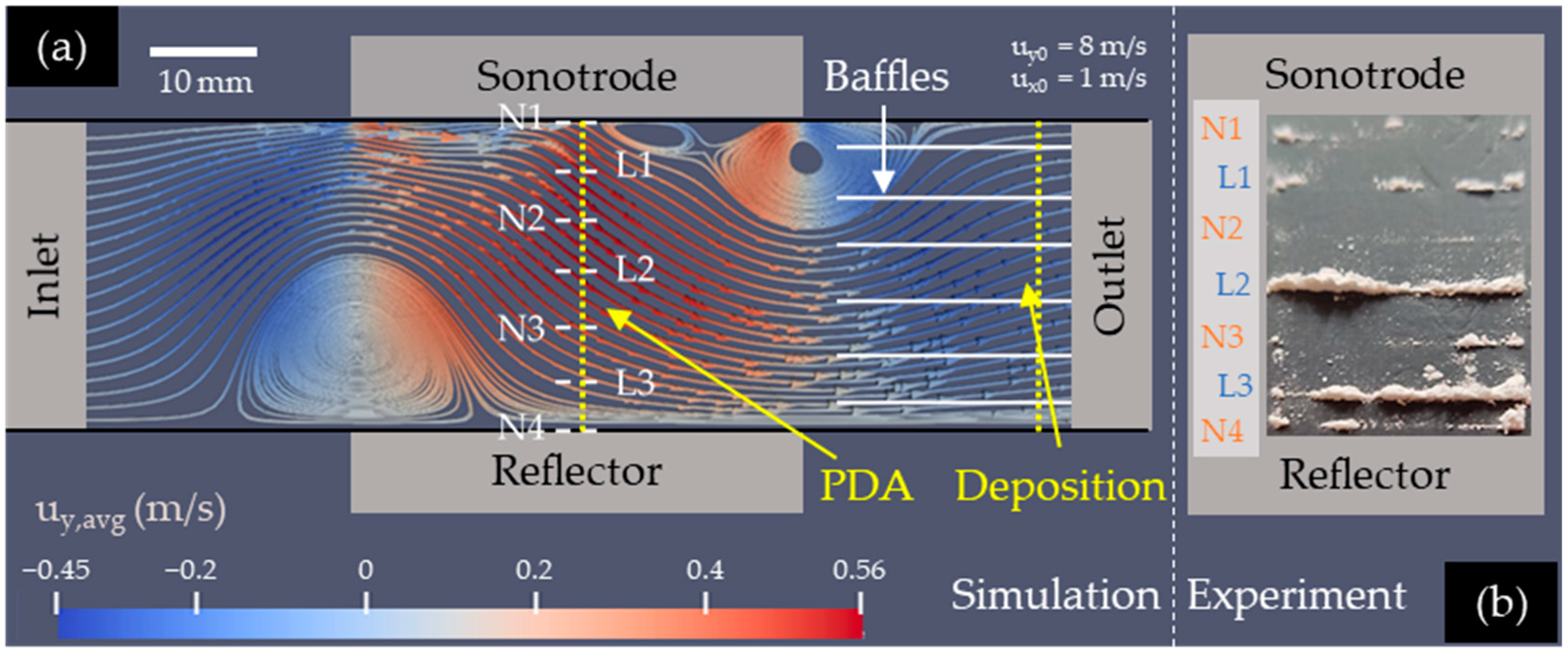
3.1. Particle Velocity Changes Due to Acoustic Radiation
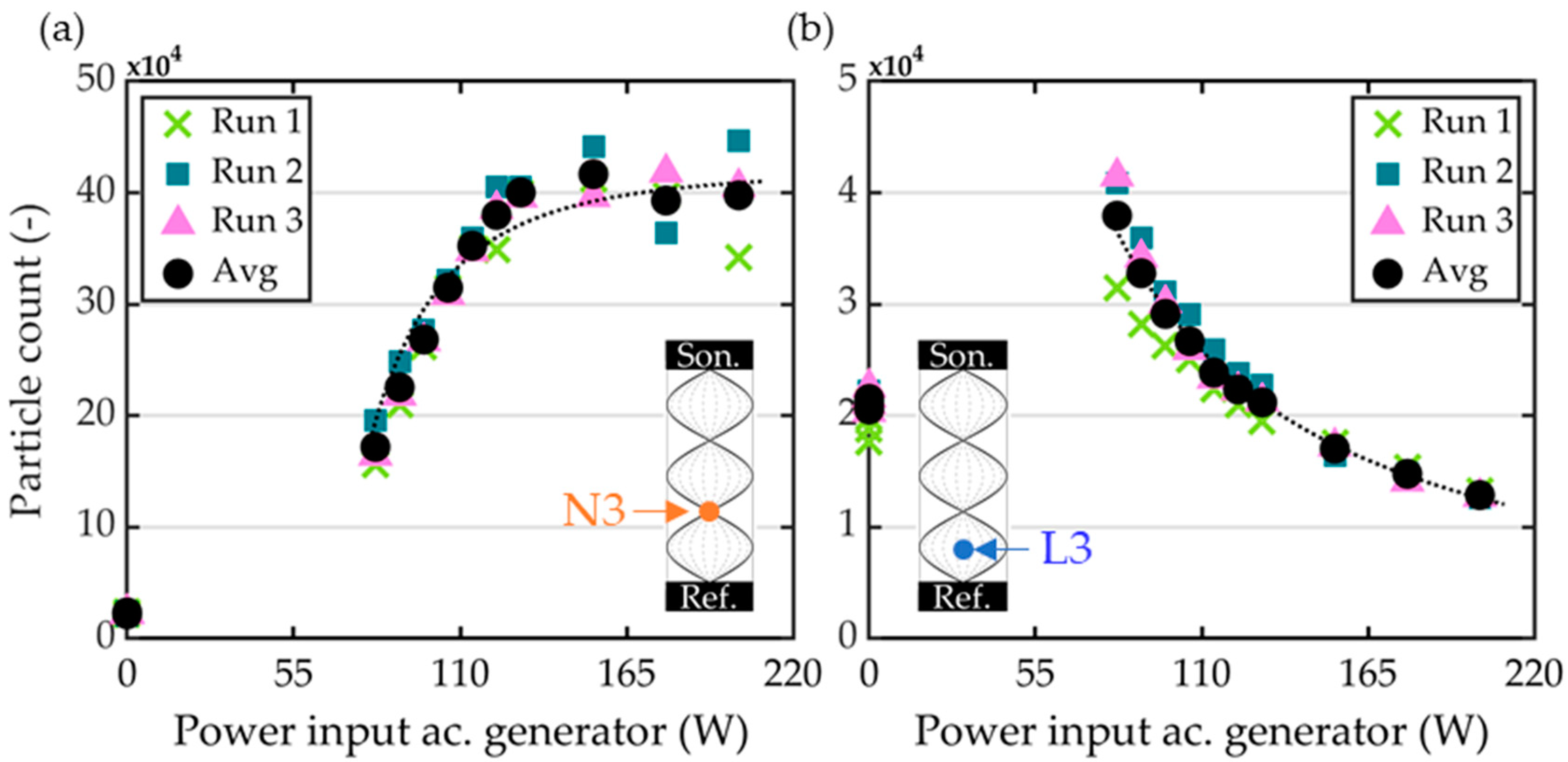
3.2. Acoustic Influence on Local Particle Number Density
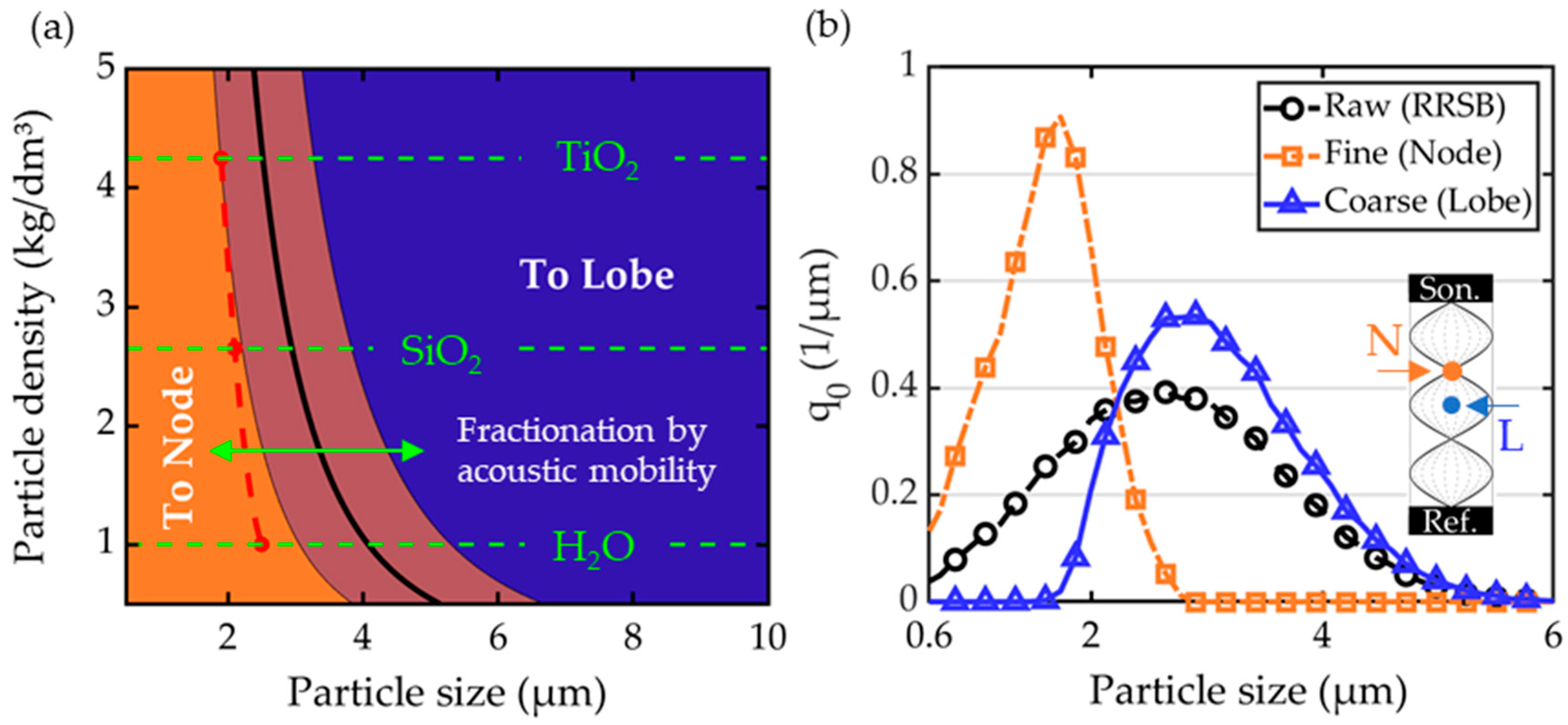
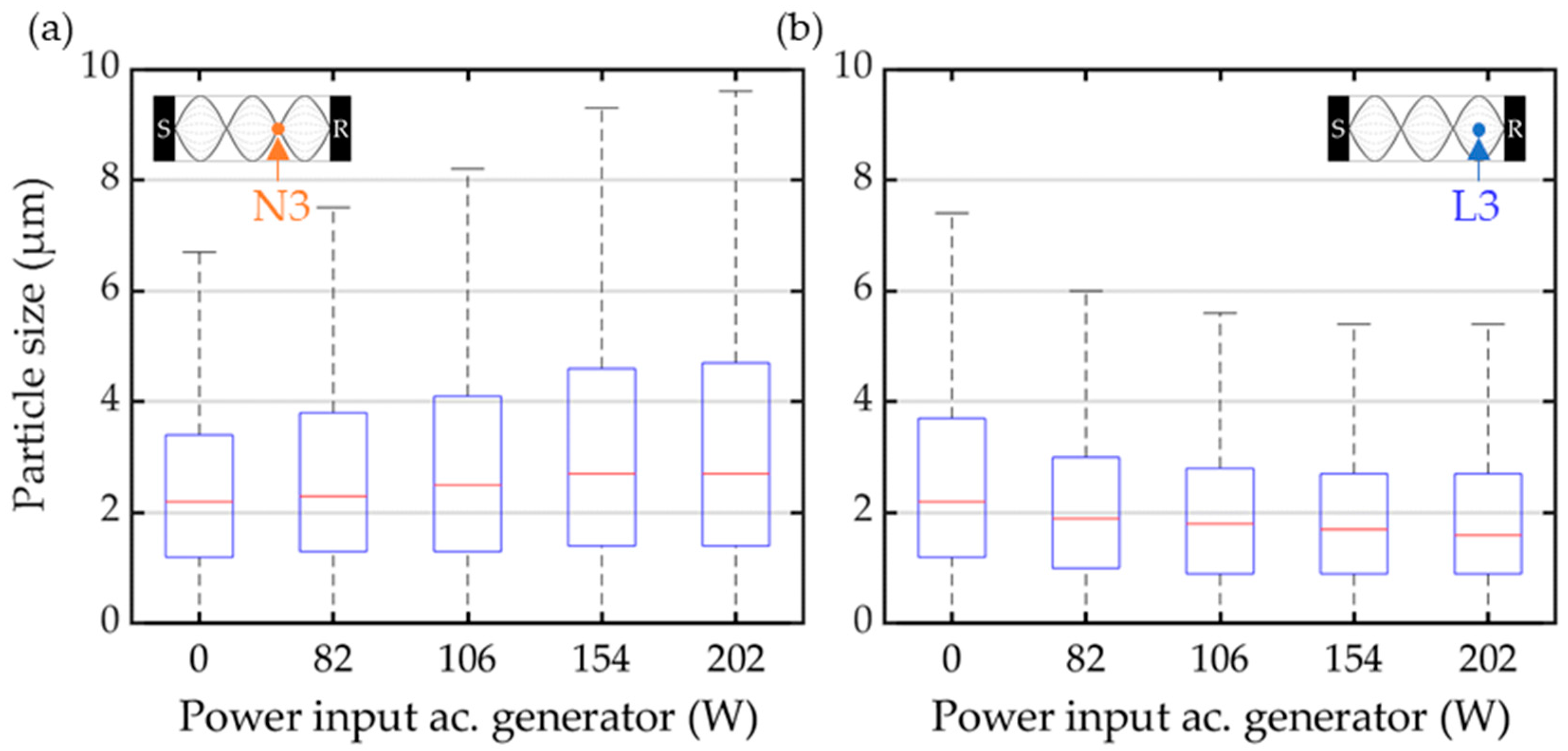
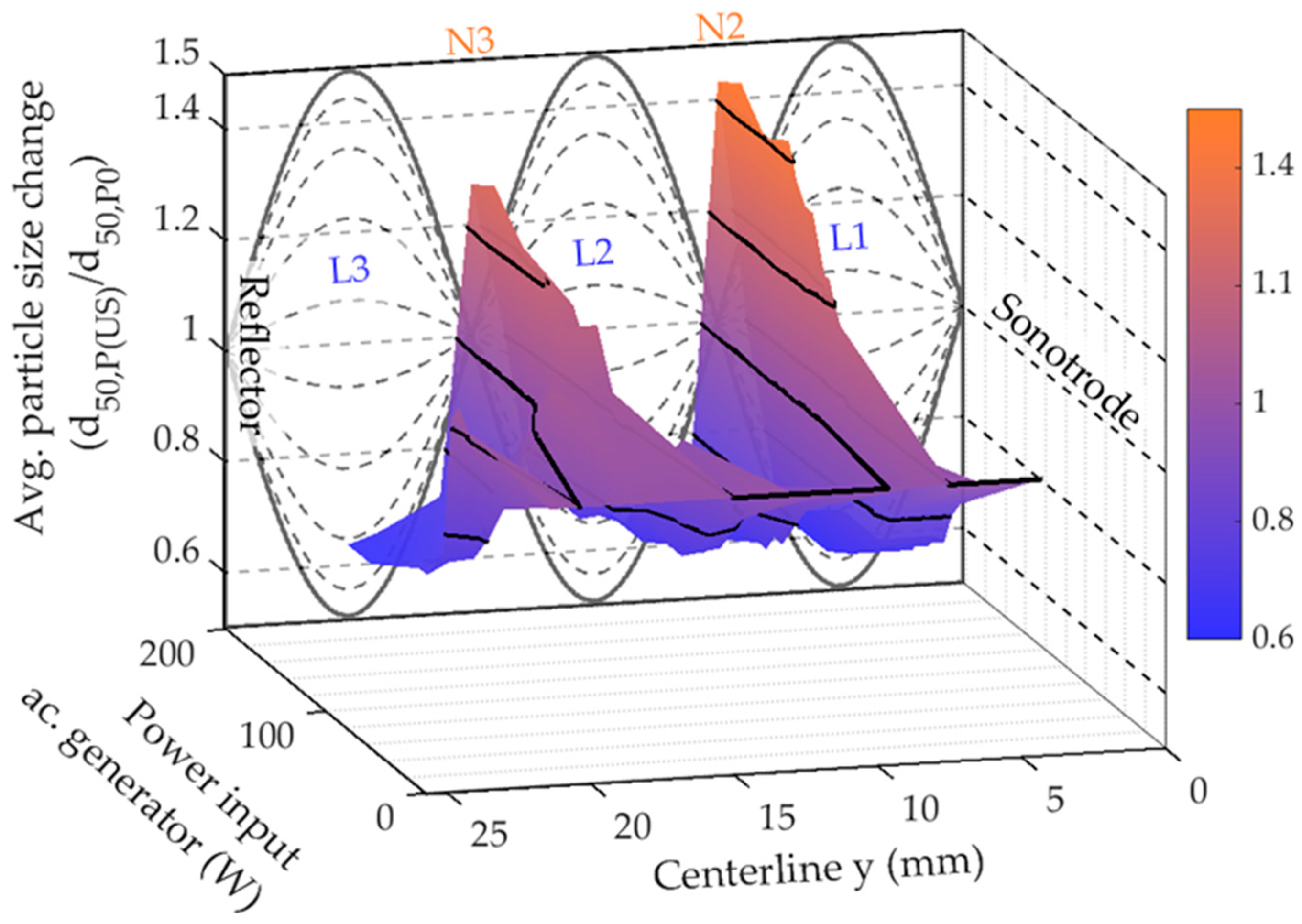
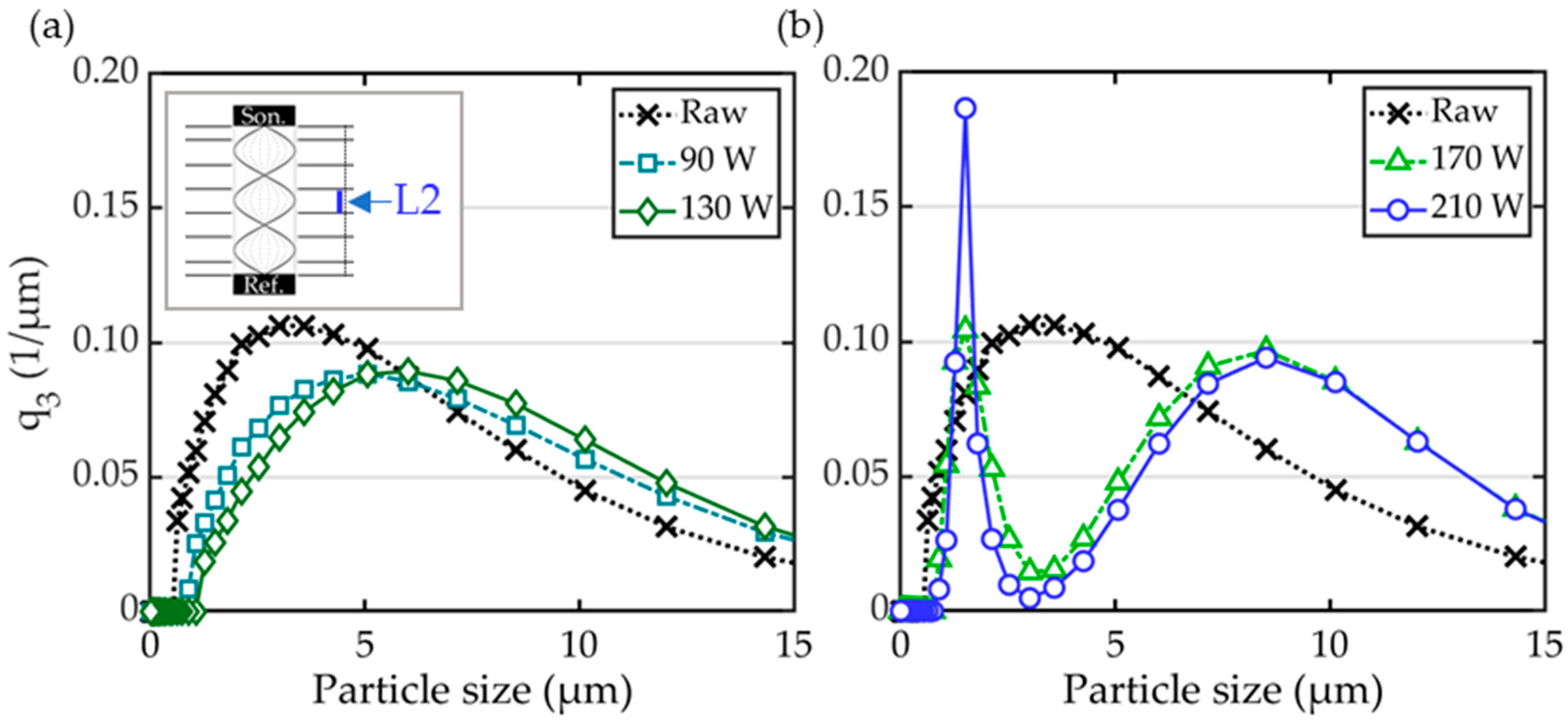
3.3. Particle Size Dependence on Acoustic Irradiation
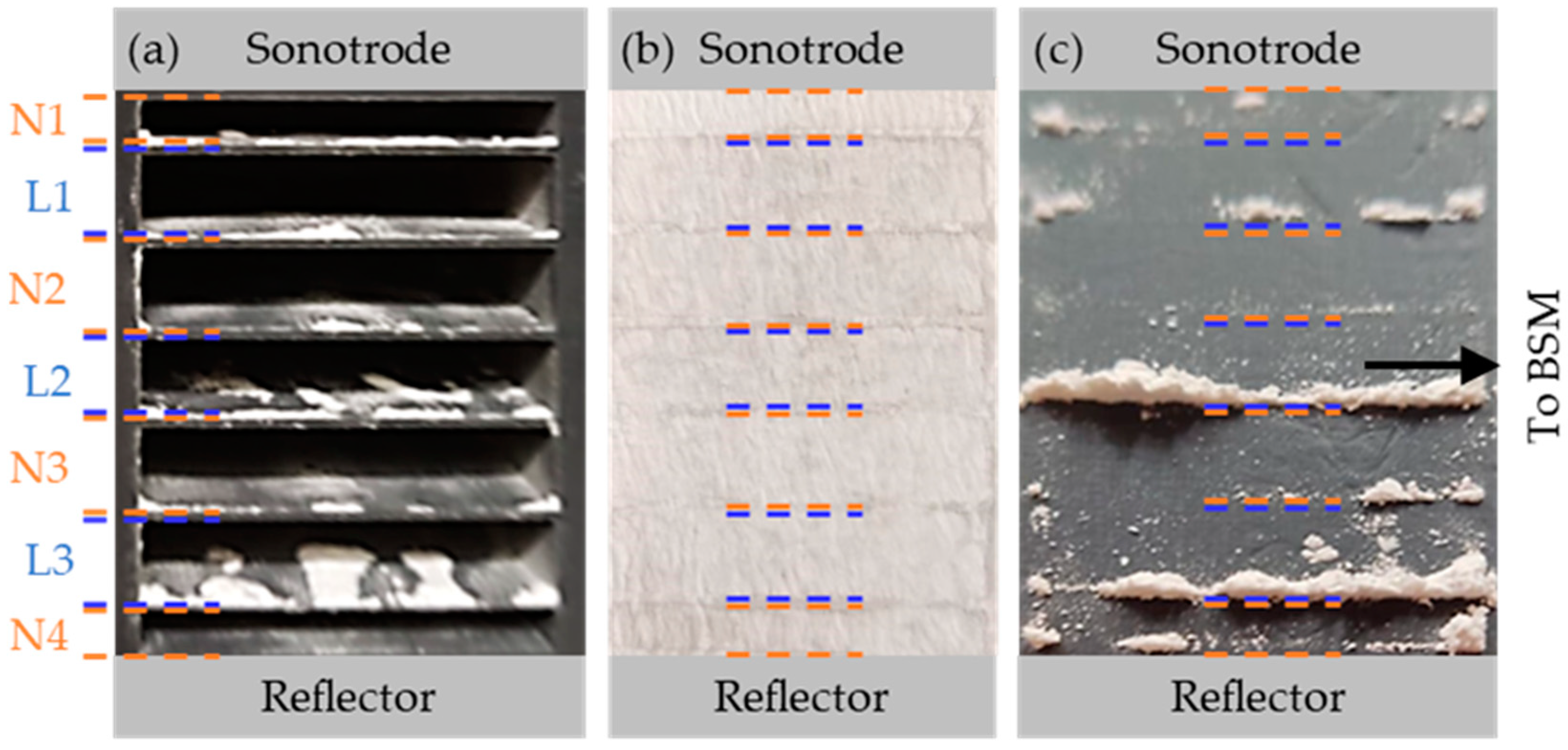
3.4. Filter Deposition Experiments
4. Discussion
5. Summary and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Buchwald, T.; Ditscherlein, R.; Peuker, U.A. Beschreibung von Trennoperationen mit mehrdimensionalen Partikeleigenschaftsverteilungen. Chem. Ing. Tech. 2022, 95, 199–209. [Google Scholar] [CrossRef]
- Bell, T.A. Challenges in the scale-up of particulate processes—An industrial perspective. Powder Technol. 2005, 150, 60–71. [Google Scholar] [CrossRef]
- Damm, C.; Long, D.; Walter, J.; Peukert, W. Size and Shape Selective Classification of Nanoparticles. Powders 2024, 3, 255–279. [Google Scholar] [CrossRef]
- Löffler, F. Staubabscheiden; Georg Thieme Verlag: Stuttgart, Germany; New York, NY, USA, 1988. [Google Scholar]
- Spötter, C.; Legenhausen, K.; Weber, A.P. Separation Characteristics of a Deflector Wheel Classifier in Stationary Conditions and at High Loadings: New Insights by Flow Visualization. KONA Powder Part. J. 2018, 35, 172–185. [Google Scholar] [CrossRef]
- Weers, M.; Hansen, L.; Schulz, D.; Benker, B.; Wollmann, A.; Kykal, C.; Kruggel-Emden, H.; Weber, A.P. Development of a Model for the Separation Characteristics of a Deflector Wheel Classifier Including Particle Collision and Rebound Behavior. Minerals 2022, 12, 480. [Google Scholar] [CrossRef]
- Stieß, M. Mechanische Verfahrenstechnik—Partikeltechnologie 1; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar] [CrossRef]
- Kuger, L.; Franzreb, M. Design of a Magnetic Field-Controlled Chromatography Process for Efficient and Selective Fractionation of Rare Earth Phosphors from End-of-Life Fluorescent Lamps. ACS Sustain. Chem. Eng. 2024, 12, 2988–2999. [Google Scholar] [CrossRef]
- Arlt, C.-R. Mehrdimensionale Fraktionierung von Feinstpartikeln Mittels Magnetischer, Kontinuierlicher Gegenstromchromatographie. Ph.D. Thesis, Karlsruher Institut für Technologie (KIT), Karlsruhe, Germany, 2022. [Google Scholar]
- Winkler, M.; Rhein, F.; Nirschl, H.; Gleiss, M. Real-Time Modeling of Volume and Form Dependent Nanoparticle Fractionation in Tubular Centrifuges. Nanomaterials 2022, 12, 3161. [Google Scholar] [CrossRef]
- Eckelt, J.; Maskos, M.; Wolf, B.A. Fractionation. In Polymer Science: A Comprehensive Reference; Elsevier: Amsterdam, The Netherlands, 2012; pp. 65–91. [Google Scholar] [CrossRef]
- Sandmann, K.; Fritsching, U. Selektive Partikelklassierung in ultraschallangeregten Aerosolen. Chem. Ing. Tech. 2020, 92, 635–642. [Google Scholar] [CrossRef]
- Sachs, S.; Baloochi, M.; Cierpka, C.; Konig, J. On the acoustically induced fluid flow in particle separation systems employing standing surface acoustic waves—Part I. Lab. Chip 2022, 22, 2011–2027. [Google Scholar] [CrossRef] [PubMed]
- Sandmann, K.; Fritsching, U. Acoustic separation and fractionation of property-distributed particles from the gas phase. Adv. Powder Technol. 2023, 34, 104270. [Google Scholar] [CrossRef]
- González, I.a.; Hoffmann, T.L.; Gallego, J.A. Precise measurements of particle entrainment in a standing-wave acoustic field between 20 and 3500 Hz. J. Aerosol Sci. 2000, 31, 1461–1468. [Google Scholar] [CrossRef]
- Fan, F.; Xu, X.; Zhang, S.; Su, M. Modeling of particle interaction dynamics in standing wave acoustic field. Aerosol Sci. Technol. 2019, 53, 1204–1216. [Google Scholar] [CrossRef]
- Arai, T.; Sato, T.; Matsubara, T. Effective Cell Transfection in An Ultrasonically Levitated Droplet for Sustainable Technology. Adv. Sci. 2022, 9, e2203576. [Google Scholar] [CrossRef]
- Lieber, C.; Autenrieth, S.; Schönewolf, K.-Y.; Lebanoff, A.; Koch, R.; Smith, S.; Schlinger, P.; Bauer, H.-J. Application of acoustic levitation for studying convective heat and mass transfer during droplet evaporation. Int. J. Multiph. Flow. 2024, 170, 104648. [Google Scholar] [CrossRef]
- Ran, W.; Saylor, J.R. The directional sensitivity of the acoustic radiation force to particle diameter. J. Acoust. Soc. Am. 2015, 137, 3288–3298. [Google Scholar] [CrossRef] [PubMed]
- Fritsching, U.; Bauckhage, K. The interaction of drops and particles with ultrasonic standing wave fields. Comput. Acoust. Its Environ. Appl. Ii 1997, 25, 151–160. [Google Scholar] [CrossRef]
- Wu, M.; Ozcelik, A.; Rufo, J.; Wang, Z.; Fang, R.; Jun Huang, T. Acoustofluidic separation of cells and particles. Microsyst. Nanoeng. 2019, 5, 32. [Google Scholar] [CrossRef]
- Luo, X.; Cao, J.; Gong, H.; Yan, H.; He, L. Phase separation technology based on ultrasonic standing waves: A review. Ultrason. Sonochem 2018, 48, 287–298. [Google Scholar] [CrossRef]
- Doinikov, A.A. Acoustic radiation force on a spherical particle in a viscous heat-conducting fluid. III. Force on a liquid drop. J. Acoust. Soc. Am. 1997, 101, 731–740. [Google Scholar] [CrossRef]
- Danilov, S.D.; Mironov, M.A. Mean force on a small sphere in a sound field in a viscous fluid. J. Acoust. Soc. Am. 2000, 107, 143–153. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Chen, X. Particle separation in microfluidics using different modal ultrasonic standing waves. Ultrason. Sonochem. 2021, 75, 105603. [Google Scholar] [CrossRef] [PubMed]
- Holwill, I.L.J. The use of ultrasonic standing waves to enhance optical particle sizing equipment. Ultrasonics 2000, 38, 650–653. [Google Scholar] [CrossRef]
- Sajeesh, P.; Sen, A.K. Particle separation and sorting in microfluidic devices: A review. Microfluid. Nanofluid. 2013, 17, 1–52. [Google Scholar] [CrossRef]
- Townsend, R.J.; Hill, M.; Harris, N.R.; White, N.M. Modelling of particle paths passing through an ultrasonic standing wave. Ultrasonics 2004, 42, 319–324. [Google Scholar] [CrossRef] [PubMed]
- Imani, R.J.; Robert, E. Estimation of acoustic forces on submicron aerosol particles in a standing wave field. Aerosol Sci. Technol. 2017, 52, 57–68. [Google Scholar] [CrossRef]
- Manneberg, O. Multidimensional Ultrasonic Standing Wave Manipulation in Microfluidic Chips. Ph.D. Thesis, Royal Institute of Technology, Stockholm, Sweden, 2009. [Google Scholar]
- Settnes, M.; Bruus, H. Forces acting on a small particle in an acoustical field in a viscous fluid. Phys. Rev. E Stat. Nonlin Soft Matter Phys. 2012, 85, 016327. [Google Scholar] [CrossRef]
- Karlsen, J.T.; Bruus, H. Forces acting on a small particle in an acoustical field in a thermoviscous fluid. Phys. Rev. E Stat. Nonlin Soft Matter Phys. 2015, 92, 043010. [Google Scholar] [CrossRef]
- Online Representation of AcouSort AB. Available online: https://acousort.com/ (accessed on 7 March 2024).
- Funcke, G.; Frohn, A. 29 O 02 Numerical investigation of the influence of different forces on the motion of aerosol particles in a standing sonic field. J. Aerosol Sci. 1993, 24, S339–S340. [Google Scholar] [CrossRef]
- Lerch, R.; Sessler, G.; Wolf, D. Technische Akustik; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar] [CrossRef]
- Weller, H.G.; Tabor, G.; Jasak, H.; Fureby, C. A tensorial approach to computational continuum mechanics using object-oriented techniques. Comput. Phys. 1998, 12, 620–631. [Google Scholar] [CrossRef]
- Greenshields, C. OpenFOAM v10 User Guide; The OpenFOAM Foundation: London, UK, 2022. [Google Scholar]
- Ofner, B. Phase Doppler Anemometry (PDA). In Optical Measurements; Franz Mayinger, O.F., Ed.; Springer: Berlin/Heidelberg, Germany, 2001; pp. 139–152. [Google Scholar] [CrossRef]




| US-Setting (%) | 20 | 30 | 40 | 50 | 60 | 70 | 80 | 90 | 100 |
| Power input (W) | 80 | 95 | 108 | 123 | 145 | 160 | 176 | 196 | 207 |
| Elong.pp (µm) | 65 | 79 | 90 | 101 | 108 | 116 | 126 | 134 | 137 |
| Position | mm | 2.2 | 3.2 | 4.3 | 5.4 | 6.4 | 7.5 | 8.6 | 9.7 | 10.7 | 11.8 | 12.9 | 13.9 | 15.0 | 16.1 | 17.2 | 18.2 | 19.3 | 20.4 | 21.4 |
| λ | 1/8 | 3/16 | 1/4 | 5/16 | 3/8 | 7/16 | 1/2 | 9/16 | 5/8 | 11/16 | 3/4 | 13/16 | 7/8 | 15/16 | 1 | 17/16 | 9/8 | 19/16 | 5/4 | |
| Id. | L1 | N2 | L2 | N3 | L3 | |||||||||||||||
| # | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 | |
| # | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | |
| US Power | % | 0 | 20 | 25 | 30 | 0 | 35 | 40 | 45 | 0 | 50 | 65 | 0 | 80 | 95 | 0 |
| W | 0 | 82 | 90 | 98 | 0 | 106 | 114 | 122 | 0 | 130 | 154 | 0 | 178 | 202 | 0 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sandmann, K.; Fritsching, U. Selective Multidimensional Particle Fractionation Applying Acoustic Fields. Powders 2025, 4, 5. https://doi.org/10.3390/powders4010005
Sandmann K, Fritsching U. Selective Multidimensional Particle Fractionation Applying Acoustic Fields. Powders. 2025; 4(1):5. https://doi.org/10.3390/powders4010005
Chicago/Turabian StyleSandmann, Krischan, and Udo Fritsching. 2025. "Selective Multidimensional Particle Fractionation Applying Acoustic Fields" Powders 4, no. 1: 5. https://doi.org/10.3390/powders4010005
APA StyleSandmann, K., & Fritsching, U. (2025). Selective Multidimensional Particle Fractionation Applying Acoustic Fields. Powders, 4(1), 5. https://doi.org/10.3390/powders4010005







