Axion Mass and the Ground State of Deconfining SU(2) Yang–Mills Thermodynamics

Abstract

For the deconfinement phase of an SU(2) Yang–Mills theory, we compute the axion mass $m_A$ by appealing to the Veneziano–Witten formula. The topological susceptibility $\chi$ arises (i) from a precisely computable thermal ground-state contribution due to a center of a relevant (anti)caloron, and (ii) from contributions due to free thermal quasi-particles in the effective theory. Both (i) and (ii) are derived by using standard Euclidean thermal field theory techniques. While contribution (i) is positive and $\propto T^4$, contribution (ii) is negative, as demanded by reflection positivity, but negligible compared to contribution (i). As a consequence, practically from the critical temperature $T_c$ onward, a real-valued axion mass $m_A\left(T\right)=\sqrt{{\displaystyle \frac{2}{3}}}\pi {\displaystyle \frac{T^2}{M_P}}$ emerges when the Peccei–Quinn scale is assumed to be the Planck mass $M_P$, independently of the Yang–Mills scale that the axion associates with. We discuss why our results deviate from those found in the dilute instanton gas and interacting instanton liquid approximations, and from results obtained in lattice simulations. Assuming the universe is dark sector to be based on such ultralight axion species, which are nonrelativistic for $T\ll M_P$, we investigate the cosmological conditions for their global Bose condensation as the very early universe cooled to temperatures of the order of ${10}^9$eV.

1. Introduction

The temperature (T)-dependence of the topological susceptibility $\chi$ in the deconfinement phase of an SU(2) or SU(3) Yang–Mills theory was estimated by various methods: dilute instanton gas [1], instanton liquid [2], lattice simulations [3,4,5,6,7,8,9], and models of the stochastic vacuum [10]. Knowing the T-dependence of $\chi$ precisely is important for understanding cosmological models whose (fuzzy) dark-matter sector is based on the quantum dynamics of ultralight axions [11,12,13,14,15,16,17].

Based on $\chi \left(T\right)$, the Veneziano–Witten formula can be employed to calculate the axion mass $m_A\left(T\right)$. Originally, this formula was proposed as an estimate for the mass of the pseudoscalar ${\eta }^{\prime }$-meson in QCD. For cosmologically stable axions, however, the required smallness of axion masses and decay constants compared to particle physics scales could originate from the extreme hierarchy between the Planck mass $M_P$ (the Peccei–Quinn scale), at which the regime gravitational attraction [18] may condense massless fermion flavors [19,20] and generate a massless, pseudoscalar, flavor-singlet field $\varphi$, and the Yang–Mills scales of pure SU(2) or SU(3) theories, which associate with visible matter and radiation described by the Standard Model of Particle Physics in an evolving universe [21,22,23]. These hierarchically smaller-than-$M_P$ Yang–Mills scales may also, by some as-yet-unknown mechanism, be determined by physics of the Planckian regime.

As in [20], we thus consider a dynamical breaking of the global symmetry U(1)_A [24,25] at the Planck scale.

Gauge field configurations that are assumed to saturate the topological susceptibility $\chi$ (see Equation (1)) are usually taken to be instantons in the literature, subject to a finite-temperature electric Debye screening to modify the $T=0$ instanton density. This only allows for instanton radii $\rho <{\left(\pi T\right)}^{-1}$ to contribute to the model partition function [26]. The results for $\chi$, when computed in a dilute gas of instantons (DIGA) [1], or in a liquid of instantons (IILA) [2] have, in turn, inspired fit models in lattice simulations of $\chi$ [3]. Note that these instanton-based approaches do not resemble the situation of a thermal ground state [23], which (i) is composed of spatially densely packed Harrington–Shepard (HS) calorons or anticalorons [27], exhibiting an intrinsic T-dependence of the topological charge density Q per isolated field configuration, and which (ii) is dominated by caloron radii $\rho \propto T^{1/2}$ [23]. Point (ii) implies that properly capturing the topological charge of a relevant gauge-field configuration requires enormous spatial volumes at high T, which are not available in lattice simulations [23,28]. Indeed, Figure 1 depicts the ratio of the lattice size $L=2/T_c$, employed in an SU(3) simulation [4], and the size of the relevant caloron radius $\rho ={\displaystyle \frac{{\lambda }_c^{3/2}}{2\pi T_c}}{\left({\displaystyle \frac{T}{T_c}}\right)}^{1/2}$ [23] as a function of $T/T_c$ for both SU(2) and SU(3).

Therefore, throughout the deconfinement phase ($T\ge T_c$), the lattice size L never accommodates the center of a relevant caloron; the spatial lattice simply falls through the topological charge distribution. That is, the integral $\int d^{4}xQ\left(x\right)$ in Equation (1) below1 is not saturated over the lattice volume.

In the rapid power-law decay of $\chi \sim a{T}^{-b}$ suggested for the gauge group SU(3) by DIGA [1], or by IILA [2] (for the SU(3) case, b ranges as $4.9\le b\le 9$, depending on the number of fermion flavors in the simulation, and there is disagreement in the normalization a by a factor of ten between DIGA, and fits to lattice results [4]) could, therefore, well be an artifact of the assumed diluteness of the topological charge carriers, a neglect of their intrinsic T dependence (instantons are not periodic in the Euclidean time coordinate $x_4$), and the above-mentioned, perturbatively motivated, T-dependent constraint on the instanton scale on the modeling side. As mentioned above, lattice simulations [3,4] suffer from severe finite-spatial-volume constraints; see also [9] for an insightful discussion.

The purpose of the present paper is to compute the T-dependence of $\chi$ for deconfining SU(2) Yang–Mills thermodynamics, and to discuss some implications for a modeling of the cosmological dark sector, based on the T-dependence of the axion mass derived from the right-hand side of the Veneziano–Witten formula (VWF). Our work is organized as follows: In Section 2 we briefly review the VWF for the axion mass, and comment on its interpretation in the realm of cosmology. The computation of the topological susceptibility, $\chi$, by far dominated by the spatial center of a HS caloron or anticaloron, relevant for the emergence of the thermal ground state of deconfining SU(2) Yang–Mills thermodynamics, is carried out in Section 3. Here, we will show that, in contrast to lattice and DIGA/IILA modeling, and as a result of the dense spatial packing of (anti)caloron centers in the deconfining thermal ground state, as well as the rapid, power-law rise of the caloron scale parameter with T, the topological susceptibility $\chi$ evolves as $\chi =a{T}^4$ for $T\gg T_c$. This can be expected from dimensional analysis and, motivated by perturbative asymptotic freedom at $T=0$ [29,30], the irrelevance of the Yang–Mills scale $\mathsf{\Lambda }$ at large T [9]. Confirming this expectation, we compute the factor of proportionality to be $a=2{\pi }^2/3$ for an SU(2) Yang–Mills theory in terms of an effective contact term in the topological charge correlation. We also show that the (negative) contribution of lower spatial resolutions to the right-hand side of VWF, which can be computed in the effective theory [23] in terms of a non-contact term, are negligible. In Section 4 we indicate cosmological implications for very high redshifts in a model of the dark sector where the present dark matter and dark energy are related to selfgravitating depercolated and global condensates of ultralight axions, respectively. More specifically, cosmological dark matter requires such condensates to be spatially confined to subgalactic scales [11,16,31] (fuzzy dark matter), while dark energy requires a superhorizon-sized axion condensate [31]. Finally, we summarize our results, and present an outlook on future research in Section 5.

Throughout the paper, we work in supernatural units: $\hslash =k_B=c=1$, where ℏ denotes Planck’s (reduced) quantum of action, $k_B$ is Boltzmann’s constant, and c refers to the speed of light in vacuum.

2. Axion Mass Squared via the Veneziano–Witten Formula at a Finite Temperature

The VWF for the mass of the U(1)_A Goldstone field A in QCD, defined on ${\mathbf{R}}^4$ and subject to gauge group SU($N_c$), as well as $N_f$ massless fundamentally charged fermion flavors, reads [32,33]

$$\underset{N_c\to \infty }{lim}{\displaystyle \frac{m_A^2{f}^2}{2N_f}}=\underset{N_c\to \infty }{lim}\int d^{4}x{\langle Q\left(x\right)Q\left(0\right)\rangle }_T\equiv \chi ,$$

(1)

where the topological charge density $Q\left(x\right)$ is given as

$$Q\left(x\right)\equiv {\displaystyle \frac{1}{64{\pi }^2}}{ϵ}_{\mu \nu \rho \sigma }{F}_{\mu \nu }^a{F}_{\rho \sigma }^a\equiv {\displaystyle \frac{1}{32{\pi }^2}}{F}_{\mu \nu }^a{\tilde{F}}_{\mu \nu }^a.$$

(2)

Here, ${\langle \cdots \rangle }_T$ denotes the canonical ensemble average in pure SU($N_c$) Yang–Mills thermodynamics, and Greek indices take on values 4, 1, 2, 3. In the present work, we assume, per [1,2,3,4,5,6,7,8,9,26], the validity of Equation (1) in the deconfinement phase of Yang–Mills thermodynamics at finite temperature ($T>0$).

In computing the T-dependence of the axion mass $m_A$, the large $N_c$ limit is relevant to those SU(2)_i or SU(3)_j subgroups of SU($N_c$) only (associated with a Yang–Mills scale ${\mathsf{\Lambda }}_i$ or ${\mathsf{\Lambda }}_j$) which are in their deconfinement phases. We will show in Section 3.2 that for such Yang–Mills theories, practically only the thermal ground state [23] contributes to the right-hand side of Equation (1). In applying SU(2) Yang–Mills thermodynamics to cosmology, the simulated evolution of the Cosmic Microwave Background (CMB) [34], initialized at a redshift of $z\sim {10}^9$ and terminating today ($z=0$), requires a single such SU(2) theory only [21,23]: SU(2)_CMB with ${\mathsf{\Lambda }}_{\mathrm{CMB}}\sim {10}^{-4}$eV [35]. According to [20] we may therefore fix in Equation (1) ${lim}_{N_c\to \infty }{f}^2/\left(2N_f\right)\sim M_P^2$ for $N_f\gg 1$, where $M_P$ denotes the Planck mass $M_P=1.22\times {10}^{19}$GeV.

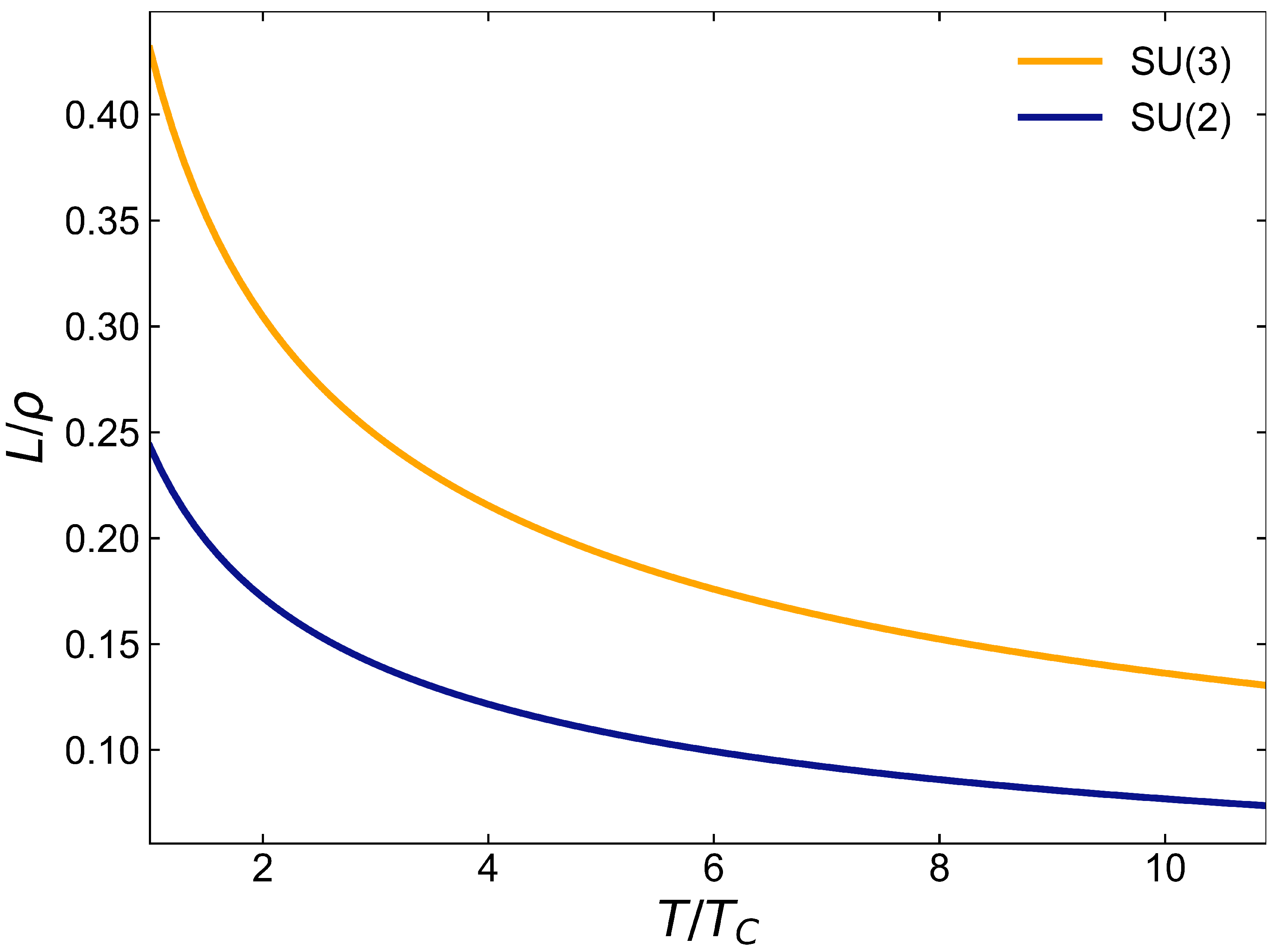
3. The Veneziano–Witten Integral for Deconfining SU(2) Yang–Mills Thermodynamics

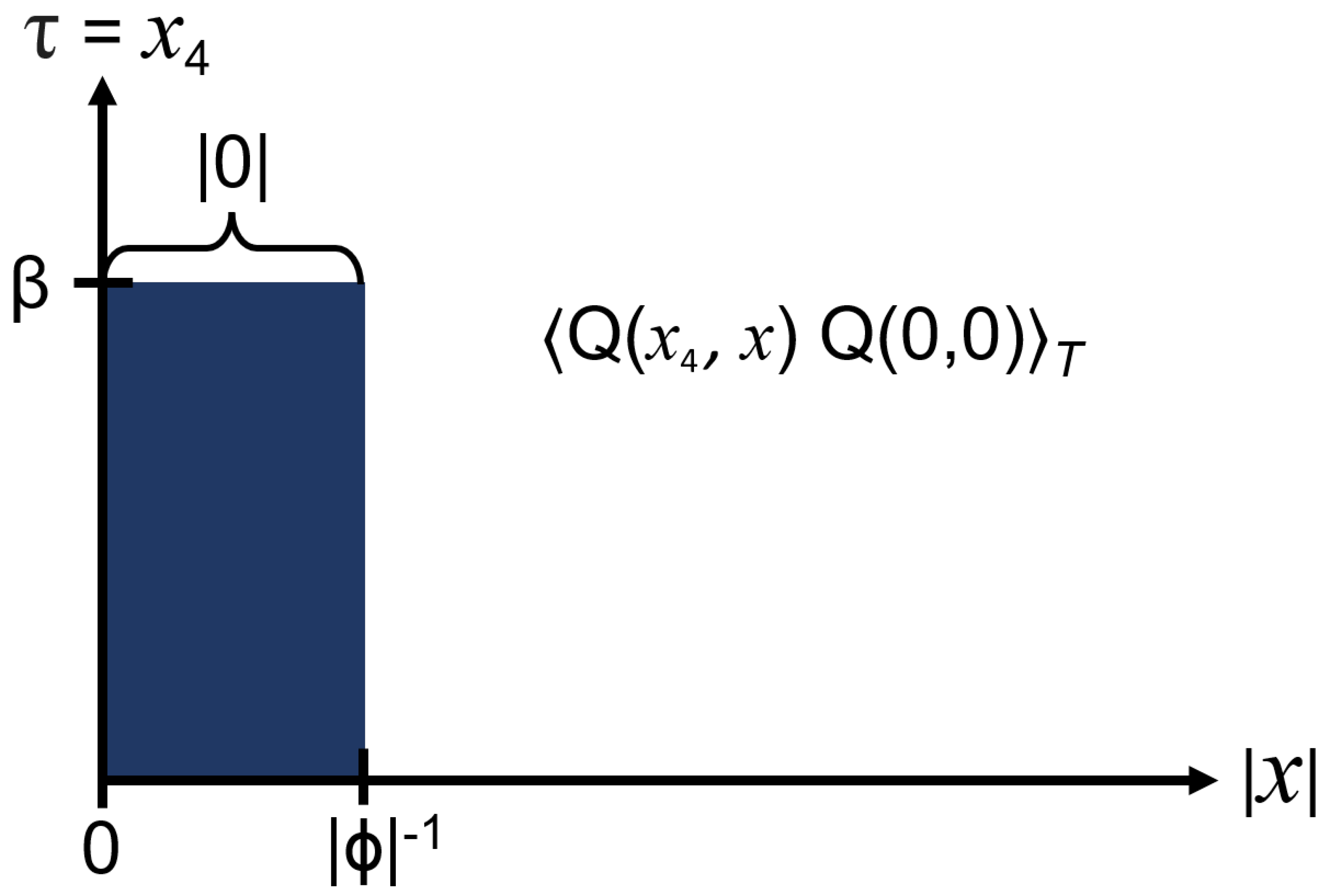
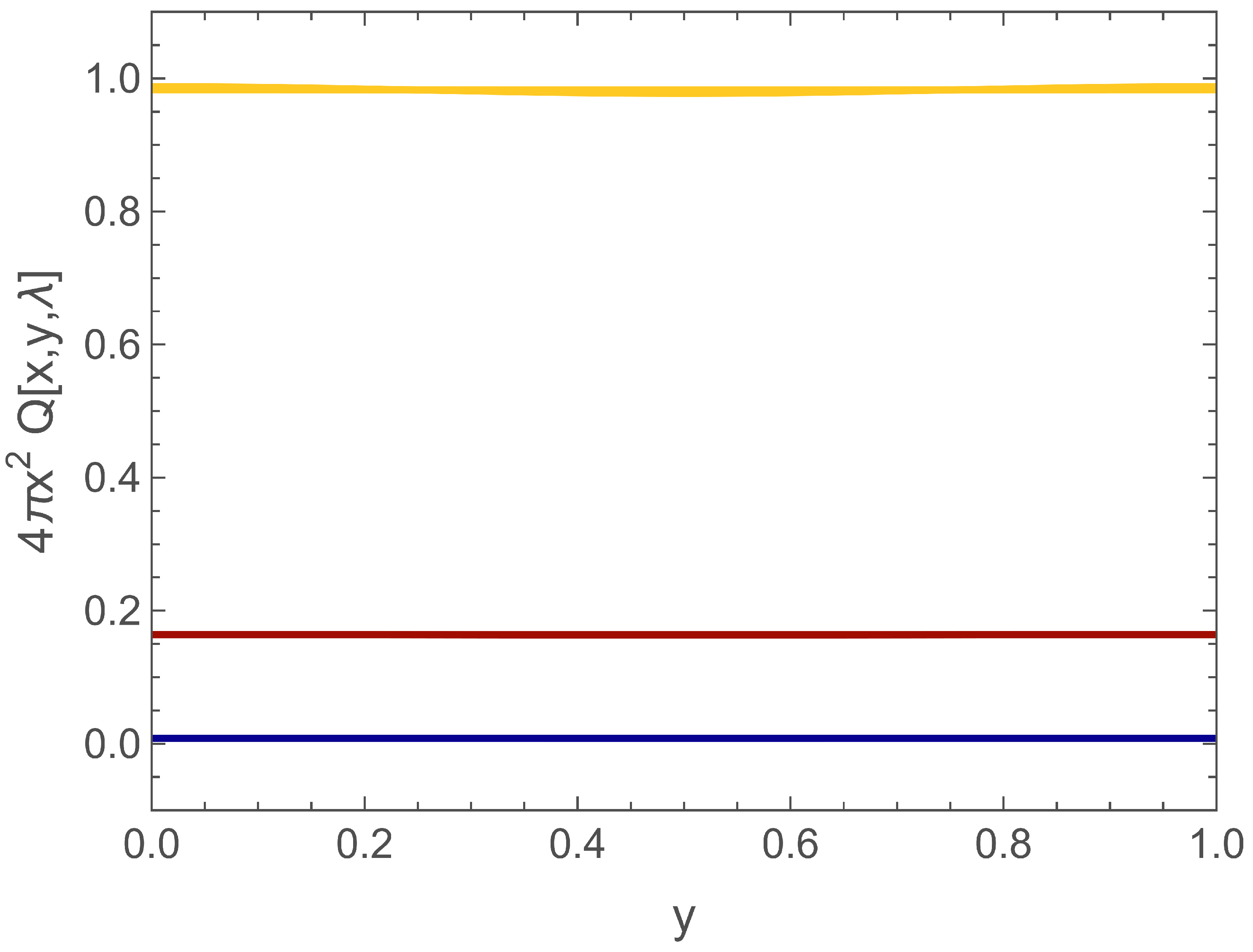
3.1. The Topological Susceptibility $\chi$ on a Harrington–Shepard (HS) (Anti)Caloron

In this section, we compute the short-distance contribution to the axion mass squared, relying on Equation (1), which represents a contact term in the effective theory [23]. In the deconfinement phase, the topological susceptibility $\chi$ of Equation (1) originates from an HS (anti)caloron center [27] in the thermal ground state (a thermal quantum vacuum [23]. Thereby, HS (anti)caloron centers are densely packed spatially, subject to overlapping peripheries. There is no winding in these peripheries. Thus, the ground-state portion of ${\langle Q\left(x\right)Q\left(0\right)\rangle }_T$ is computed on a single caloron center.

At a finite temperature, the spacetime average of $Q\left(x\right)$ is over the Euclidean cylinder $S_1\times {\mathbf{R}}^3$, $x_4\equiv \tau ,x_i=r{\widehat{x}}_i$ ($i=1,2,3$), where $\widehat{x}$ is a unit vector in ${\mathbf{R}}^3$ and $r=\mid x\mid \le \mid \varphi {\mid }^{-1}$; see Figure 2.

Since the right-hand side of Equation (1) is quadratic in Q, and since we average over the contribution of an HS caloron and an HS anticaloron [23], the thermal ground-state part of ${\langle Q\left(x\right)Q\left(0\right)\rangle }_T$ is represented by an HS caloron which, due to spatial isotropy, spatially is centered at $r=0$ (peak position of the HS caloron’s action density).

When expressing the field strength $F_{\mu \nu }^a$, expanded into a basis $t^a$ ($a=1,2,3$) of the Lie algebra SU(2) such that tr $t^a{t}^b={\displaystyle \frac{1}{2}}{\delta }^{ab}$, in terms of $O\left(4\right)$ scalar and tensor valued functionals $P\left(x\right)$ and $P_{\mu \nu }\left(x\right)$ of the caloron prepotential $\Pi \left(x\right)$, we follow the convention of [36]. Namely, in a singular gauge one has for the gauge field of an HS caloron with topological charge $k=1$,

$$A_{\mu }^a=-{\overline{\eta }}_{\mu \kappa }^a{\displaystyle \frac{{\partial }_{\kappa }\Pi }{\Pi }},$$

(3)

where ${\overline{\eta }}_{\mu \kappa }^a$ denotes the (anti-selfdual) ’t Hooft symbol, and $\Pi$ is the prepotential given as [27]

$$\Pi (\tau ,r)=1+{\displaystyle \frac{{\rho }^2}{\beta r}}{\displaystyle \frac{sinh\frac{2\pi r}{\beta }}{cosh\frac{2\pi r}{\beta }-cos\frac{2\pi \tau }{\beta }}}.$$

(4)

Here, $\beta$ denotes the inverse of temperature T, $\beta \equiv {\displaystyle \frac{1}{T}}$, and $\rho$ is the associated instanton scale parameter [37,38,39]. Note that the second term on the right-hand side of Equation (4) originates from summing the instanton prepotential in singular gauge over equidistantly shifted values of its time coordinate $\tau$. This renders $\Pi (\tau ,r)$ periodic in $\tau$, which can be seen by the dependence on $cos{\displaystyle \frac{2\pi \tau }{\beta }}$. For the field strength, this implies staticity for sufficiently large values of r. The field strength $F_{\mu \nu }^a\equiv {\partial }_{\mu }{A}_{\nu }^a-{\partial }_{\nu }{A}_{\mu }^a+{ϵ}^{abc}{A}_{\mu }^b{A}_{\nu }^c$ is expressed as

$$F_{\mu \nu }^a={\overline{\eta }}_{\mu \nu }^{a}P-{\overline{\eta }}_{\nu \kappa }^a{P}_{\mu \kappa }+{\overline{\eta }}_{\mu \kappa }^a{P}_{\nu \kappa }$$

(5)

if we define

$$P\equiv {\displaystyle \frac{({\partial }_{\kappa }\Pi )({\partial }_{\kappa }\Pi )}{{\Pi }^2}},P_{\mu \nu }\equiv {\displaystyle \frac{\Pi {\partial }_{\mu }{\partial }_{\nu }\Pi -2({\partial }_{\mu }\Pi )({\partial }_{\nu }\Pi )}{{\Pi }^2}}.$$

(6)

Here, ${ϵ}^{abc}$ denotes the 3D totally antisymmetric tensor with ${ϵ}^{123}=1$. Note that

$$P_{\mu \nu }=P_{\nu \mu },P_{\mu \mu }=-2P.$$

(7)

Therefore, one has, for a selfdual caloron field2,

$$Q\equiv {\displaystyle \frac{1}{32{\pi }^2}}{F}_{\mu \nu }^a{F}_{\mu \nu }^a={\displaystyle \frac{1}{8{\pi }^2}}\left(P_{\mu \nu }^2-P^2\right).$$

(8)

Considering that $\Pi =\Pi (\tau ,r)$, we have

$$\begin{array}{ccc}\hfill F_{\mu \nu }^a{F}_{\mu \nu }^a& =& 4\left(3{\left({\displaystyle \frac{{\partial }_r\Pi }{\Pi }}\right)}^4+{\left({\displaystyle \frac{{\partial }_r^2\Pi }{\Pi }}\right)}^2+2{\left({\displaystyle \frac{{\partial }_r\Pi }{r\Pi }}\right)}^2+3{\left({\displaystyle \frac{{\partial }_{\tau }\Pi }{\Pi }}\right)}^4+\right.\hfill \\ & & {\left({\displaystyle \frac{{\partial }_r\Pi }{\Pi }}\right)}^2\left(-4{\displaystyle \frac{{\partial }_r^2\Pi }{\Pi }}+6{\left({\displaystyle \frac{{\partial }_{\tau }\Pi }{\Pi }}\right)}^2\right)-4{\left({\displaystyle \frac{{\partial }_{\tau }\Pi }{\Pi }}\right)}^2{\displaystyle \frac{{\partial }_{\tau }^2\Pi }{\Pi }}+{\left({\displaystyle \frac{{\partial }_{\tau }^2\Pi }{\Pi }}\right)}^2-\hfill \\ & & \left.8{\displaystyle \frac{{\partial }_r\Pi }{\Pi }}{\displaystyle \frac{{\partial }_{\tau }\Pi }{\Pi }}{\displaystyle \frac{{\partial }_r{\partial }_{\tau }\Pi }{\Pi }}+2{\left({\displaystyle \frac{{\partial }_r{\partial }_{\tau }\Pi }{\Pi }}\right)}^2\right).\hfill \end{array}$$

(9)

Introducing rescaled variables, $y\equiv {\displaystyle \frac{\tau }{\beta }}$, $x\equiv {\displaystyle \frac{r}{\beta }}$, the dimensionless temperature $\lambda ={\displaystyle \frac{2\pi T}{\mathsf{\Lambda }}}$, $\mathsf{\Lambda }$ denoting the SU(2) Yang–Mills scale, and setting $\rho =\mid \varphi {\mid }^{-1}=\sqrt{{\displaystyle \frac{2\pi T}{{\mathsf{\Lambda }}^3}}}$ to be the dominating value of the scale parameter in the thermal ground state [23], Equation (4) is cast into

$$\Pi (\tau ,r,T)=\overline{\Pi }(y,x,\lambda )=1+{\displaystyle \frac{{\lambda }^3}{4\pi x}}{\displaystyle \frac{sinh2\pi x}{cosh2\pi x-cos2\pi y}}.$$

(10)

Here, we have introduced the general dimensionless function $\overline{f}(y,x,\lambda )=T^{-n}f(\tau ,r,T)$, where n is the mass dimension of function f. Substituting Equation (10) into Equation (9) and using Equation (8) yields

$$\underset{r\to 0}{lim}Q(\tau ,r,T)=T^4\underset{x\to 0}{lim}\overline{Q}(y,x,\lambda )=2{\pi }^2{T}^4{\lambda }^6{\displaystyle \frac{{\left(8+{\lambda }^3+4cos2\pi y\right)}^2}{3{\left(2+{\lambda }^3-2cos2\pi y\right)}^4}}.$$

(11)

According to Equation (11), the y and the $\lambda$ dependencies of $\overline{Q}(y,x=0,\lambda )$ are very weak for $\lambda \ge {\lambda }_c=13.87$ [23]. At finite x, this behavior of $\overline{Q}(y,x,\lambda )$ with respect to variations in y and $\lambda$ persists, which is demonstrated in Figure 3 for $x=0,0.5,1.0$ and $\lambda ={\lambda }_c,3{\lambda }_c,10{\lambda }_c$.

For $\lambda \ge {\lambda }_c=13.87$, the topological charge $k\equiv 4\pi {\int }_0^{1}dy{\int }_0^{\infty }dx{x}^2\overline{Q}(y,x,\lambda )=1$, thus, to a very good accuracy, can be computed as

$$k=4\pi {\int }_0^{\infty }dx{x}^2\overline{Q}({\displaystyle \frac{1}{2}},x,\lambda ).$$

(12)

If we truncate the integration over x in Equation (12) at the scale $x_{\max }={\displaystyle \frac{\rho }{\beta }}={\displaystyle \frac{\mid \varphi {\mid }^{-1}}{\beta }}={\displaystyle \frac{{\lambda }^{3/2}}{2\pi }}$,

$$k=4\pi {\int }_0^{{\displaystyle \frac{{\lambda }^{3/2}}{2\pi }}}dx{x}^2\overline{Q}({\displaystyle \frac{1}{2}},x,\lambda ),$$

(13)

then we numerically obtain $k=0.9768,0.9927,0.9938$ for $\lambda ={\lambda }_c,2{\lambda }_c,2.5{\lambda }_c$, respectively. This shows that, for practically all $\lambda$, the topological charge of an HS (anti)caloron resides within its center. The (dimensionless) radius $x_{\max }$ of this center, however, grows $\propto {\lambda }^{3/2}$ with dimensionless temperature $\lambda$. This explains why lattice simulations, essentially operating at a fixed spatial volume at varying T, fail to capture large portions of the topological charge of a relevant (anti)caloron in $\chi \left(T\right)$; compare this with Figure 1.

The short-distance correlation of the thermal ground state of an SU(2) Yang–Mills theory are mediated by the centers of an HS caloron or anticaloron [23]. As confirmed above, the (anti)caloron action, representing a nontrivial topological charge of this gauge-field configuration, is localized within the (anti)caloron center. As a consequence, the ground-state contribution to the ensemble average on the right-hand side of Equation (1) represents the contribution of an (anti)caloron center—a contact term within the effective, deconfining Minkowskian quantum thermodynamics [23]; see also Figure 2. In contrast to calorons, which are action minimizers of the 4D defining, classical, and Euclidean Yang–Mills theory, and are infinitely resolved, the effective theory describes the quantum dynamics of gauge fields in (3+1)D Minkowski space at spatial distances larger than $\mid \varphi {\mid }^{-1}$. By matching the effective theory with the fundamental one at this resolution limit, it was argued in [40] that the caloron action coincides with Planck’s quantum of action ℏ. Light-cone localized, propagating solutions to the source-free Minkowskian Yang–Mills equations—photons—, which could be initiated by sufficiently resolving, time-periodic probes of (anti)caloron centers, were constructed recently by A. Rabinowitch [41]. Given these localized solutions, a probe’s circular frequency $\omega$ could thus provoke propagating quanta of energy ℏω.

We are now in a position to compute the thermal ground state contribution of the right-hand side of Equation (1) as

$$\begin{array}{ccc}\hfill {\chi }_{\mathrm{cal}.\mathrm{cen}.}& =& {\int }_{\mathrm{cal}.\mathrm{cen}.}d\tau d\Omega dr{r}^{2}Q(\tau ,r,T)Q(0,0,T)\hfill \\ & =& 4\pi T^4\overline{Q}(0,0,\lambda ){\int }_0^{1}dy{\int }_0^{{\displaystyle \frac{{\lambda }^{3/2}}{2\pi }}}dx{x}^2\overline{Q}(y,x,\lambda )\hfill \\ & \simeq & 4\pi T^4\overline{Q}(0,0,\lambda ){\int }_0^{{\displaystyle \frac{{\lambda }^{3/2}}{2\pi }}}dx{x}^2\overline{Q}({\displaystyle \frac{1}{2}},x,\lambda )\hfill \\ & \simeq & {\displaystyle \frac{2}{3}}{\displaystyle \frac{{(12+{\lambda }^3)}^2{\pi }^2}{{\lambda }^6}}{T}^4\hfill \\ & {\displaystyle \genfrac{}{}{0pt}{}{\lambda \gg {\lambda }_c}{⟶}}& {\displaystyle \frac{2}{3}}{\pi }^2{T}^4,\hfill \end{array}$$

(14)

where $d\Omega$ denotes the angular part of the measure in the spatial integration. Therefore,

$$m_A=\sqrt{{\displaystyle \frac{2}{3}}}\pi {\displaystyle \frac{T^2}{M_P}},(T\gg T_c).$$

(15)

Note that, due to the integral of Equation (13) being close to unity and due to the function on the right-hand side in Equation (11) being near to $2{\pi }^2{T}^4/3$ already at $\lambda ={\lambda }_c=13.87$, the T-dependence of $m_A$, as in Equation (15), is already an excellent approximation for $T\sim T_c$.

3.2. Do Thermal Quasiparticle Fluctuations Contribute to $\chi$?

Equation (14) associates with a contact term of the correlator ${\langle Q(x_4,x)Q(0,0)\rangle }_T$ in the effective theory of the deconfining phase of SU(2) Yang–Mills thermodynamics [23]. In [42], it is suggested that precisely such a contact term saturates the right-hand side of Equation (1) at $T=0$; see also an associated argument for the Schwinger model in [43]. In Appendix A, we show that, in the effective theory for the deconfining phase of SU(2) Yang–Mills thermodynamics [23], a negative contribution to $\chi$ arises from massless and massive, effectively free thermal quasi-particles. Judging by the smallness of two- and three-loop corrections to the free quasi-particle approximation of thermodynamic quantities, this should be precise to the sub-percent level [23]. As computed in Appendix A, free quasi-particles in $\chi \left(T\right)$ generate a positive coefficient in front of $-T^4$, which is much smaller than unity. Therefore, we can consider the result of Equation (14), a reliable estimate of the entire topological susceptibility $\chi$: the expression for the axion mass $m_A$ in Equation (15), which emerges from the axial anomaly invoked by SU(2) (anti)calorons, should be accurate to the sub-percent level. In [10], $\chi \left(T\right)$ was computed in the model of the stochastic vacuum. For the nonperturbative part of the correlator ${\langle Q(x_4,x)Q(0,0)\rangle }_T$, a factorized negative contribution was shown to be overcompensated by a positive nonfactorized contribution. Both are expressed in terms of nonperturbative vacuum parameters measured at zero temperature, which were extracted from lattice simulations. As a result, a $T^4$-dependence with a positive coefficient also was found in [10].

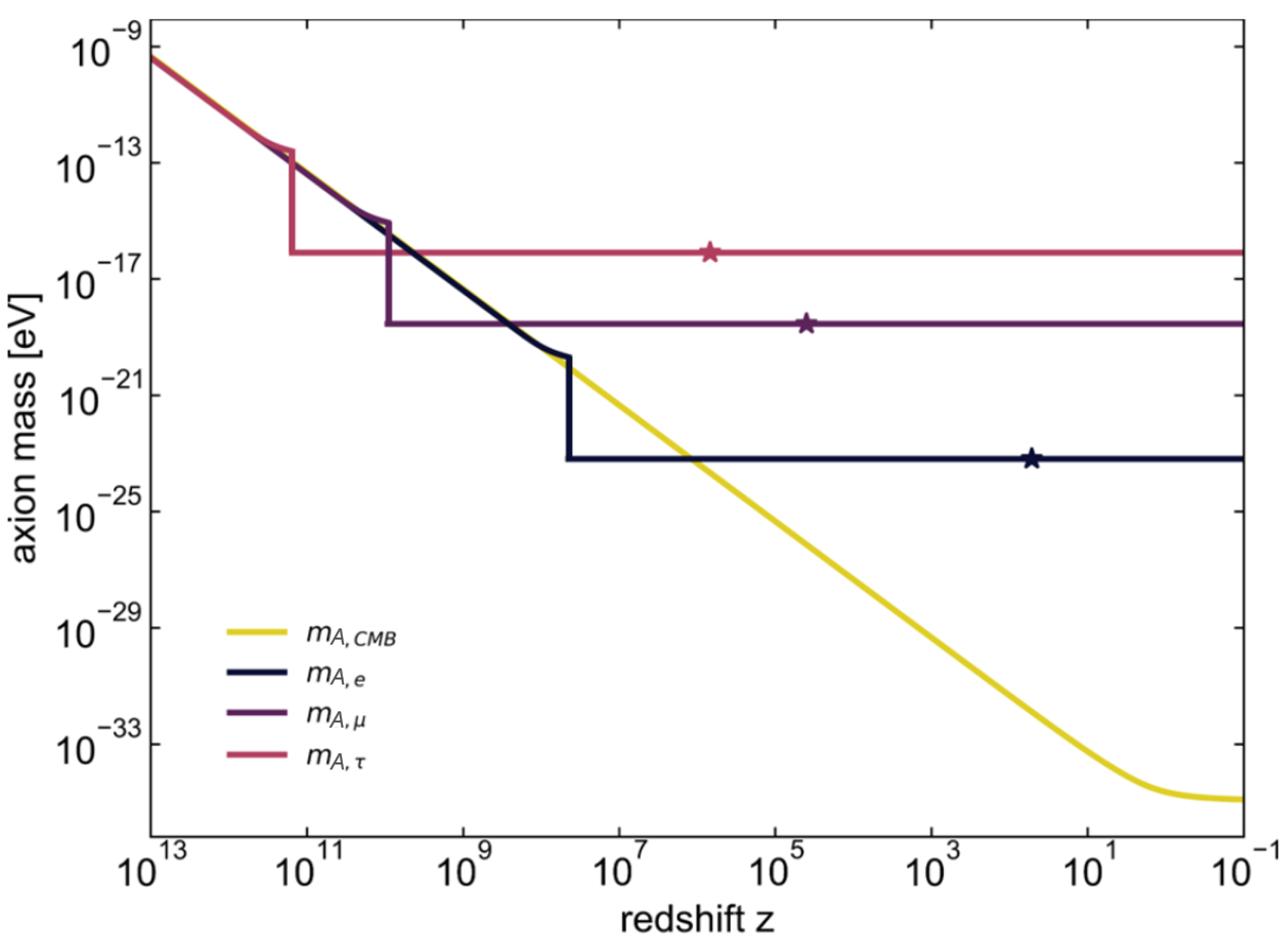
4. Cosmological Implications: Bose Condensation of Axion Particles

As a function of increasing cosmological redshift z axion masses for four different species are shown in Figure 4. In the confining phase of the associated SU(2) Yang–Mills theory, the axion mass is constant in z. For the three species concerned at the present temperature of the CMB, $T_0=2.725$K, and up to $z\sim {10}^8$, the lowest axion mass can be extracted phenomenologically from rotation curves of low-surface-brightness galaxies [31]. The other two masses follow from Yang–Mills scaling relations (subject to a common Peccei–Quinn scale, $M_P$) in the fuzzy dark matter model. Such scaling relies on a link to lepton families introduced in [31], and explicated for the first lepton family in [21]. As soon as a SU(2) Yang–Mills theory transitions to its deconfinement phase, the associated axion mass starts to depend on z via Equation (15), and is subject to the T–z relation in the deconfinement phase [44]. At high redshifts ($z>{10}^{12}$) all axion species exhibit the same dependence of their mass on redshift z. Note that the validity of our axion–mass computation in an expanding Friedmann–Lemaître–Robertson–Walker universe hinges on temperature T being larger than the Hubble expansion rate H. In a radiation dominated universe we estimate $H/T=O\left(10\right)T/M_P$. Therefore, we demand $T/M_P\ll O({10}^{-1})$, which allows us to address most of the universe is expansion history.

Let us now investigate the implications of Equation (15) for the critical temperature $T_{c,B}$ of Bose condensation involving non-relativistic axion particles of mass $m_A$ and number density3$n_A$. One has [50]

$$T_{c,B}={\displaystyle \frac{2\pi }{m_A}}{\left({\displaystyle \frac{n_A}{\zeta \left(\frac{3}{2}\right)}}\right)}^{{\displaystyle \frac{2}{3}}},$$

(16)

where $\zeta \left(x\right)$ denotes the Riemann zeta function ($\zeta \left({\displaystyle \frac{3}{2}}\right)=2.6124$). If we assume that, due to the long-range quantum correlations facilitated by a super-horizon (reduced) Compton wave length4 $m_{A,i}^{-1}$, the energy density ${\rho }_{{\mathsf{\Lambda }}_i}=m_{A,i}{n}_{A,i}$ of a global axion condensate exhibits negligible redshift (or T)-dependence then the dependence of the axion mass on temperature of Equation (15) predicts

$$T_{c,B,i}={\left({\displaystyle \frac{486}{{\pi }^4}}\right)}^{1/26}{\left({\displaystyle \frac{{\rho }_{{\mathsf{\Lambda }}_i}}{\zeta \left(\frac{3}{2}\right)}}\right)}^{2/13}{M}_P^{5/13}.$$

(17)

Setting ${\rho }_{{\mathsf{\Lambda }}_{\mathrm{CMB}}}={\left({10}^{-3}\mathrm{eV}\right)}^4$ and $M_P=1.22\times {10}^{28}\mathrm{eV}$ yields $T_{c,B,\mathrm{CMB}}=8.3\times {10}^8\mathrm{eV}$, which associates with a redshift $z>{10}^{12}$. This is beyond the initial redshift $z\sim {10}^9$ assumed in CMB simulations [34]. Higher vacuum energy densities, ${\rho }_{{\mathsf{\Lambda }}_i}>{\rho }_{{\mathsf{\Lambda }}_{\mathrm{CMB}}}$, yield higher values of $T_{c,B,i}$, even though their spread is small due to the small power of $2/13$ in Equation (17).

Due to the large hierarchy between $T_{c,B,i}$ and $M_P$, and by virtue of Equation (15), thermal axions constitute radiation for all temperatures T within the regime $M_P\gg T>T_{c,B,i}$. However, in a radiation dominated universe, they only represent a small fraction of radiation energy density. This is because radiation domination arises due the Stefan–Boltzmann limit of Yang–Mills thermodynamics. For $T\sim M_P$, where we expect Einstein gravity to profoundly develop quantum behavior, thermal axions would become nonrelativistic again.

5. Summary and Outlook

In this paper, we have revisited the computation of axion mass according to the Veneziano–Witten formula applied to the deconfining phase of an SU(2) Yang–Mills theory. Our result for the T-dependence of the topological susceptibility $\chi$ deviates from what is found in the literature: a $T^4$-dependence, which is expected from dimensional analysis [9], and indeed shown to emerge in our computation involving the center of a Harrington–Shepard caloron to represent the thermal ground state locally [23], contrasts with power laws of large negative exponents [1,2,3,4,5,6,7,8]. In Section 1, we have pointed out various possible reasons for why such a discrepancy arises. We also have shown that the contributions to $\chi$ stemming from massless and massive effective excitations of the thermal ground state of a given SU(2) Yang–Mills theory yield an extremely suppressed negative correction (reflection positivity) to the positive caloron contribution. Finally, we have investigated the consequences of this result for cosmological model building. Such models consider the dark sector as axial-anomaly induced by virtue of SU(2) Yang–Mills theories subject to a common Peccei–Quinn scale $M_P$ [20]. Such gauge theories possibly relate to the three lepton families of the Standard Model of Particle Physics [21,31]. A fourth SU(2) theory of scale ${\mathsf{\Lambda }}_{\mathrm{CMB}}\sim {10}^{-4}$eV (and Peccei–Quinn scale $M_P$)—SU(2)_CMB—may generate a present axion mass of $\sim {10}^{-35}$eV; therefore, a super-horizon Compton wavelength could, thus, relate to dark energy. It is worth asking at what temperatures these theories Bose condense their axion particles in a cooling universe. We find that the associated critical temperatures depend on the respective energy densities of the condensate through a power law of fractional power $2/13$. This suggests, in heating the very early universe through these transitions, that for all axion species, the epoch of dissolving dark energy into radiation is occurring at nearly the same temperature.

The results obtained here could be useful for cosmological model building in a framework where the dark sector of the universe is due to the selfgravitating quantum dynamics of ultralight axions [11,14,15,16,17,51].