Impact of Particle Creation in Rastall Gravity †
Abstract
1. Introduction
2. Field Equations
3. Solution of the Modified Field Equations
3.1. Model I
3.2. Model II
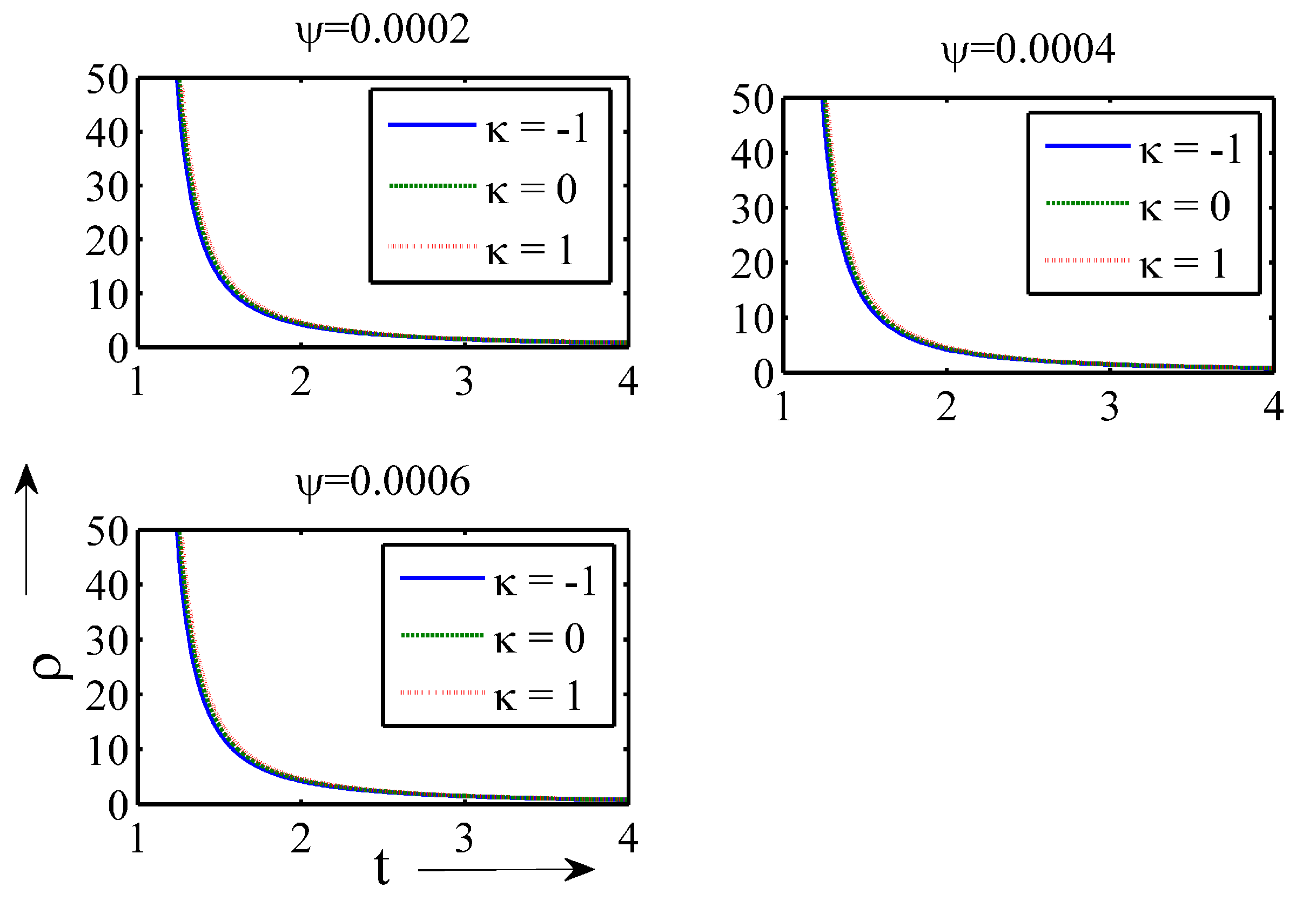
4. Discussions
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Capozziello, S.; Cardone, V.F.; Farajollahi, H.; Ravanpak, A. Cosmography in f(T) gravity. Phys. Rev. D 2011, 84, 043527. [Google Scholar] [CrossRef]
- Jimenez, J.B.; Heisenberg, L.; Koivisto, T.; Pekar, S. Cosmology in f(Q) geometry. Phys. Rev. D 2020, 101, 103507. [Google Scholar] [CrossRef]
- Najera, A.; Fajardo, A. Fitting f(Q, T) gravity models with a CDM limit using H(z) and Pantheon data. Phys. Dark Universe 2021, 34, 100889. [Google Scholar] [CrossRef]
- Cembranos, J.A. Dark matter from R2 gravity. Phys. Rev. Lett. 2009, 102, 141301. [Google Scholar] [CrossRef] [PubMed]
- De Felice, A.; Tsujikawa, S. Construction of Cosmologically viable f(G) gravity models. Phys. Lett. B 2009, 675, 1–8. [Google Scholar] [CrossRef]
- De Laurentis, M.; Paolella, M.; Capozziello, S. Cosmological inflation in f(R, G) gravity. Phys. Rev. D 2015, 91, 083531. [Google Scholar] [CrossRef]
- Akbar, M.; Cai, R.G. Friedmann equations of FRW universe in scalar–tensor gravity, f(R) gravity and first law of thermodynamics. Phys. Lett. B 2006, 635, 7–10. [Google Scholar] [CrossRef]
- Harko, T.; Lobo, F.S.; Nojiri, S.I.; Odintsov, S.D. f(R, T) gravity. Phys. Rev. D 2011, 84, 024020. [Google Scholar] [CrossRef]
- Rastall, P. Generalization of the Einstein theory. Phys. Rev. D 1972, 6, 3357. [Google Scholar] [CrossRef]
- De Moraes, W.A.G.; Santos, A.F. Lagrangian formalism for Rastall theory of gravity and Godel-type universe. Gen. Relativ. Gravit. 2019, 51, 1–17. [Google Scholar] [CrossRef]
- Shabani, H.; Ziaie, A.H. A connection between Rastall-type and f(R, T) gravities. EPL 2020, 129, 20004. [Google Scholar] [CrossRef]
- Prigogine, I.; Géhéniau, J.; Gunzig, E.; Nardone, P. Thermodynamics of cosmological matter creation. Proc. Natl. Acad. Sci. USA 1988, 85, 7428–7432. [Google Scholar] [CrossRef]
- Lima, J.A.S. Thermodynamics of decaying vacuum cosmologies. Phys. Rev. D 1996, 54, 2571. [Google Scholar] [CrossRef]
- Gunzig, E.; Maartens, R.; Nesteruk, A.V. Inflationary cosmology and thermodynamics. Class Quantum Gravity 1998, 15, 923. [Google Scholar] [CrossRef]
- Hamil, B.; Merad, M.; Birkandan, T. Particle creation in the context of the emergent universe. Rev. Mex. Física 2021, 67, 219–225. [Google Scholar] [CrossRef]
- Lyth, D.H.; Roberts, D.; Smith, M. Cosmological consequences of particle creation during inflation. Phys. Rev. D 1998, 57, 7120. [Google Scholar] [CrossRef]
- Ford, L.H. Gravitational particle creation and inflation. Phys. Rev. D 1987, 35, 2955. [Google Scholar] [CrossRef] [PubMed]
- Calzetta, E.; Hu, B.L. Dissipation of quantum fields from particle creation. Phys. Rev. D 1989, 40, 656. [Google Scholar] [CrossRef] [PubMed]
- Good, M.R.; Anderson, P.R.; Evans, C.R. Time dependence of particle creation from accelerating mirrors. Phys. Rev. D 2013, 88, 025023. [Google Scholar] [CrossRef]
- Bishi, B.K.; Lepse, P.V. Particle creation and quadratic deceleration parameter in Lyra geometry. New Astron. 2021, 85, 101563. [Google Scholar] [CrossRef]
- Kumar, R.; Singh, B.P.; Ali, M.S.; Ghosh, S.G. Shadows of black hole surrounded by anisotropic fluid in Rastall theory. Phys. Dark Universe 2021, 34, 10088. [Google Scholar] [CrossRef]
- Li, R.; Wang, J.; Xu, Z.; Guo, X. Constraining the Rastall parameters in static space–times with galaxy-scale strong gravitational lensing. Mon. Not. R. Astron. Soc. 2019, 486, 2407–2411. [Google Scholar] [CrossRef]
- Akarsu, O.; Katırcı, N.; Kumar, S.; Nunes, R.C.; Öztürk, B.; Sharma, S. Rastall gravity extension of the standard ΛCDM model: Theoretical features and observational constraints. Eur. Phys. J. C 2020, 80, 1050. [Google Scholar] [CrossRef]
- Calvao, M.O.; Lima, J.A.S.; Waga, I. On the thermodynamics of matter creation in cosmology. Phys. Lett. A 1992, 162, 223–226. [Google Scholar] [CrossRef]
- Lima, J.A.S.; Germano, A.S.M. On the equivalence of bulk viscosity and matter creation. Phys. Lett. A 1992, 170, 373–378. [Google Scholar] [CrossRef]
- Lima, J.A.S.; Calvao, M.O.; Waga, I. Cosmology, Thermodynamics and Matter Creation, Frontier Physics, Essays in Honor of Jayme Timno; World Scientific: Singapore, 1990. [Google Scholar]
- Hulke, N.; Singh, G.P.; Bishi, B.K.; Singh, A. Variable Chaplygin gas cosmologies in f(R, T) gravity with particle creation. New Astron. 2020, 77, 101357. [Google Scholar] [CrossRef]
- Barrow, J.D.; Saich, P. The behaviour of intermediate inflationary universes. Phys. Lett. B 1990, 249, 406–410. [Google Scholar] [CrossRef]
- Rendall, A.D. Intermediate inflation and the slow-roll approximation. Class Quantum Gravity 2005, 22, 1655. [Google Scholar] [CrossRef]
- Khatua, P.B.; Debnath, U. Dynamics of logamediate and intermediate scenarios in the dark energy filled universe. Int. J. Theor. Phys. 2011, 50, 799–832. [Google Scholar] [CrossRef][Green Version]
- Farajollahi, H.; Ravanpak, A. Tachyon field in intermediate inflation on the brane. Phys. Rev. D 2011, 84, 084017. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bishi, B.K.; Lepse, P.V.; Beesham, A. Impact of Particle Creation in Rastall Gravity. Phys. Sci. Forum 2023, 7, 38. https://doi.org/10.3390/ECU2023-14057
Bishi BK, Lepse PV, Beesham A. Impact of Particle Creation in Rastall Gravity. Physical Sciences Forum. 2023; 7(1):38. https://doi.org/10.3390/ECU2023-14057
Chicago/Turabian StyleBishi, Binaya Kumar, Pratik Vijay Lepse, and Aroonkumar Beesham. 2023. "Impact of Particle Creation in Rastall Gravity" Physical Sciences Forum 7, no. 1: 38. https://doi.org/10.3390/ECU2023-14057
APA StyleBishi, B. K., Lepse, P. V., & Beesham, A. (2023). Impact of Particle Creation in Rastall Gravity. Physical Sciences Forum, 7(1), 38. https://doi.org/10.3390/ECU2023-14057





