Information Geometry Control under the Laplace Assumption †
Abstract
1. Introduction
2. Model
2.1. The Laplace Assumption
Limits of the Laplace Assumption
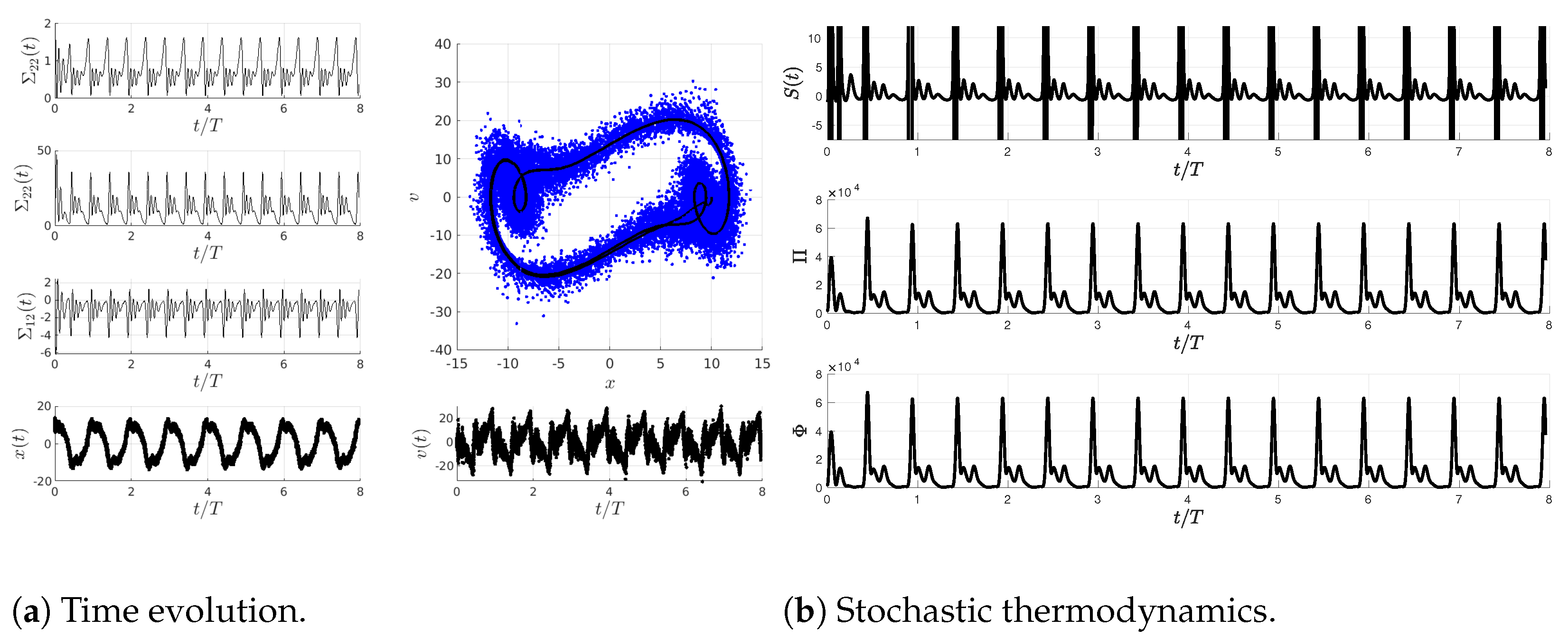
3. Stochastic Thermodynamics
3.1. Entropy Rate
3.2. Example
4. Information Length and Information Rate
Relation with Stochastic Thermodynamics
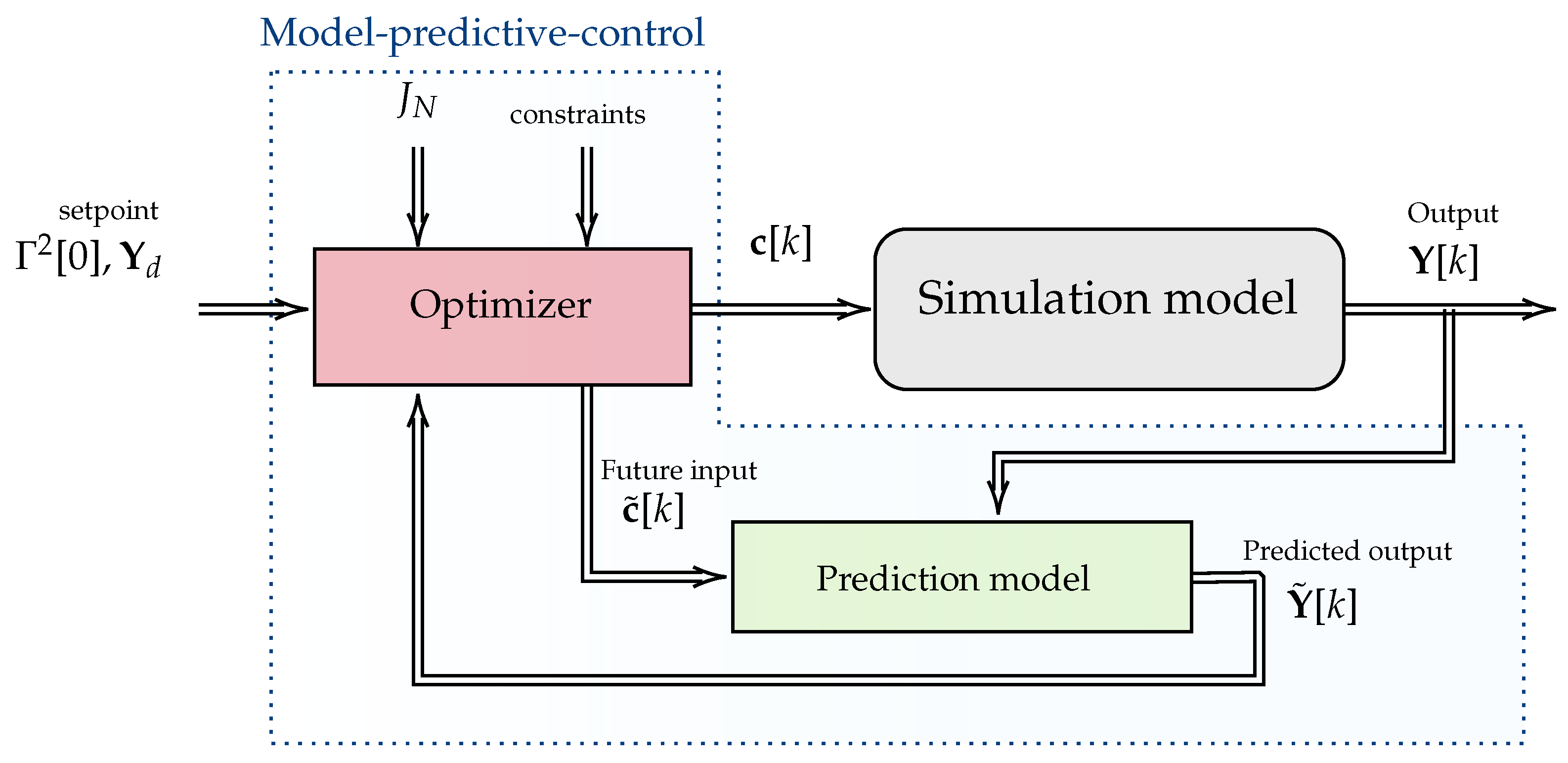
5. Minimum Variability Control
5.1. Model Predictive Control
5.2. Example
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A. Proof of Proposition 1
Appendix B. Proof of Proposition 2
References
- Seifert, U. Stochastic thermodynamics, fluctuation theorems and molecular machines. Rep. Prog. Phys. 2012, 75, 126001. [Google Scholar] [CrossRef] [PubMed]
- Gontis, V.; Kononovicius, A. Consentaneous agent-based and stochastic model of the financial markets. PLoS ONE 2014, 9, e102201. [Google Scholar] [CrossRef] [PubMed]
- de Freitas, R.A.; Vogel, E.P.; Korzenowski, A.L.; Rocha, L.A.O. Stochastic model to aid decision making on investments in renewable energy generation: Portfolio diffusion and investor risk aversion. Renew. Energy 2020, 162, 1161–1176. [Google Scholar] [CrossRef]
- Marreiros, A.C.; Kiebel, S.J.; Daunizeau, J.; Harrison, L.M.; Friston, K.J. Population dynamics under the Laplace assumption. Neuroimage 2009, 44, 701–714. [Google Scholar] [CrossRef] [PubMed]
- Maybeck, P.S. Stochastic Models, Estimation, and Control; Academic Press: Cambridge, MA, USA, 1982. [Google Scholar]
- Peliti, L.; Pigolotti, S. Stochastic Thermodynamics: An Introduction; Princeton University Press: Princeton, NJ, USA, 2021. [Google Scholar]
- Baltieri, M.; Buckley, C.L. PID control as a process of active inference with linear generative models. Entropy 2019, 21, 257. [Google Scholar] [CrossRef] [PubMed]
- Kim, E.; Hollerbach, R. Geometric structure and information change in phase transitions. Phys. Rev. E 2017, 95, 062107. [Google Scholar] [CrossRef]
- Nielsen, F. An elementary introduction to information geometry. Entropy 2020, 22, 1100. [Google Scholar] [CrossRef]
- Amari, S.I. Information Geometry and Its Applications; Springer: Berlin/Heidelberg, Germany, 2016; Volume 194. [Google Scholar]
- Kim, E. Information geometry and nonequilibrium thermodynamic relations in the over-damped stochastic processes. J. Stat. Mech. Theory Exp. 2021, 2021, 093406. [Google Scholar] [CrossRef]
- Kim, E. Information Geometry, Fluctuations, Non-Equilibrium Thermodynamics, and Geodesics in Complex Systems. Entropy 2021, 23, 1393. [Google Scholar] [CrossRef]
- Hollerbach, R.; Kim, E.; Schmitz, L. Time-dependent probability density functions and information diagnostics in forward and backward processes in a stochastic prey–predator model of fusion plasmas. Phys. Plasmas 2020, 27, 102301. [Google Scholar] [CrossRef]
- Kim, E.; Heseltine, J.; Liu, H. Information length as a useful index to understand variability in the global circulation. Mathematics 2020, 8, 299. [Google Scholar] [CrossRef]
- Guel-Cortez, A.J.; Kim, E. Information Geometric Theory in the Prediction of Abrupt Changes in System Dynamics. Entropy 2021, 23, 694. [Google Scholar] [CrossRef] [PubMed]
- Guel-Cortez, A.J.; Kim, E. Information length analysis of linear autonomous stochastic processes. Entropy 2020, 22, 1265. [Google Scholar] [CrossRef] [PubMed]
- Saridis, G.N. Entropy in Control Engineering; World Scientific: Singapore, 2001; Volume 12. [Google Scholar]
- Fan, J.; Marron, J.S. Fast implementations of nonparametric curve estimators. J. Comput. Graph. Stat. 1994, 3, 35–56. [Google Scholar]
- Stochastic Simulation Versus Laplace Assumption in a Cubic System. Available online: https://github.com/AdrianGuel/StochasticProcesses/blob/main/CubicvsLA.ipynb (accessed on 6 June 2022).
- Tomé, T. Entropy production in nonequilibrium systems described by a Fokker-Planck equation. Braz. J. Phys. 2006, 36, 1285–1289. [Google Scholar] [CrossRef]
- Soto, F.; Wang, J.; Ahmed, R.; Demirci, U. Medical micro/nanorobots in precision medicine. Adv. Sci. 2020, 7, 2002203. [Google Scholar] [CrossRef] [PubMed]
- Lee, J.H. Model predictive control: Review of the three decades of development. Int. J. Control. Autom. Syst. 2011, 9, 415–424. [Google Scholar] [CrossRef]
- Mehrez, M.W.; Worthmann, K.; Cenerini, J.P.; Osman, M.; Melek, W.W.; Jeon, S. Model predictive control without terminal constraints or costs for holonomic mobile robots. Robot. Auton. Syst. 2020, 127, 103468. [Google Scholar] [CrossRef]
- Kristiansen, B.A.; Gravdahl, J.T.; Johansen, T.A. Energy optimal attitude control for a solar-powered spacecraft. Eur. J. Control 2021, 62, 192–197. [Google Scholar] [CrossRef]
- Salesch, T.; Gesenhues, J.; Habigt, M.; Mechelinck, M.; Hein, M.; Abel, D. Model based optimization of a novel ventricular assist device. at-Automatisierungstechnik 2021, 69, 619–631. [Google Scholar] [CrossRef]
- Andersson, J.A.E.; Gillis, J.; Horn, G.; Rawlings, J.B.; Diehl, M. CasADi—A software framework for nonlinear optimization and optimal control. Math. Program. Comput. 2019, 11, 1–36. [Google Scholar] [CrossRef]
- Bemporad, A. Hybrid Toolbox—User’s Guide. 2004. Available online: http://cse.lab.imtlucca.it/~bemporad/hybrid/toolbox (accessed on 1 June 2022).
- Utkin, V.; Lee, H. Chattering problem in sliding mode control systems. In Proceedings of the International Workshop on Variable Structure Systems, Alghero, Sardinia, 5–7 June 2006; pp. 346–350. [Google Scholar]
- Petersen, K.B.; Pedersen, M.S. The matrix cookbook. Tech. Univ. Den. 2008, 7, 510. [Google Scholar]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guel-Cortez, A.-J.; Kim, E.-j. Information Geometry Control under the Laplace Assumption. Phys. Sci. Forum 2022, 5, 25. https://doi.org/10.3390/psf2022005025
Guel-Cortez A-J, Kim E-j. Information Geometry Control under the Laplace Assumption. Physical Sciences Forum. 2022; 5(1):25. https://doi.org/10.3390/psf2022005025
Chicago/Turabian StyleGuel-Cortez, Adrian-Josue, and Eun-jin Kim. 2022. "Information Geometry Control under the Laplace Assumption" Physical Sciences Forum 5, no. 1: 25. https://doi.org/10.3390/psf2022005025
APA StyleGuel-Cortez, A.-J., & Kim, E.-j. (2022). Information Geometry Control under the Laplace Assumption. Physical Sciences Forum, 5(1), 25. https://doi.org/10.3390/psf2022005025






