Resonance Leading to Unexpected and Sudden Aneurysmal Rupture ^†

Abstract

The Solitonic Windkessel model, which is successful for explaining the intracranial aneurysmal rupture based on the Windkessel-type hemodynamic circulation modelling, is employed for explaining unexpected and sudden intracranial aneurysmal rupture. In this paper, by focusing on the appearance of resonance, some aneurysmal ruptures are shown to possibly arise unexpectedly and suddenly as a resonance phenomenon.

1. Introduction

The Windkessel model is known as a nonlinear model for explaining hemodynamic circulation. While turbulence and vortex formation in the blood vessel are not considered in Windkessel models, steady fluid mechanics are taken into account to a sufficient extent. In the preceding work [1], a five-element type solitonic Windkessel model was introduced for modelling the two-story aneurysm, and the rupture condition of the intracranial aneurysm was identified. Ujiie-Iwata [1] was the first attempt to utilize the Windkessel model to evaluate aneurysmal flow and local pressure. In particular, that attempt was successful in identifying some resonating beats and the soliton wave propagation as key factors for aneurysmal rupture. In fact, significant increase of local blood pressure is naturally understood by the existence of resonating beats and soliton propagation.

Although there have been a lot of studies related to risk factors to cause aneurysmal rupture [2,3,4] it is still impossible to accurately predict when an intracranial aneurysm will rupture. Our study is showing one of the answers to this question. Flow-mediated resonance within a daughter aneurysm gives sudden rise to the forced vibration and then destroys the aneurysm. Therefore, we have to clarify the specific condition to realize the flow-mediated resonance.

In this paper, following the preceding work [1], we employ a solitonic Windkessel model for understanding unexpected and sudden rupture of intracranial aneurysm. It will clarify a substantial role of so-called “resonance” in the aneurysmal rupture. Here, we focus on the resonance between the heartbeat and the oscillatory frequency inherent to the mother and daughter aneurysms.

2. Solitonic Windkessel Model

2.1. Five-Element Formalism

The two-story aneurysm consisting of the mother and daughter aneurysm on the parent artery is shown in Figure 1. The five-element solitonic Windkessel model is exactly the same as the model studied in Ref. [1]. In the solitonic Windkessel model, blood flow inside the two-story aneurysm is analogously modelled by the electric flow inside electric circuit shown in Figure 2. Let C₁ and C₂ represent the compliance of the mother and daughter aneurysm, L₁ and L₂ stand for the inductance of the mother and daughter aneurysm, and F_1, and F₂ be the blood flow in the mother aneurysm and that in the daughter aneurysm, respectively. According to analogy between electric circuits and blood system, the following three equations are logically introduced (Figure 2). Let t be the time variable. The solitonic Windkessel model, which corresponds to the five-element Windkessel model, reads:

$$R\left(F\left(t\right)-F_1\left(t\right)\right)=P\left(t\right)$$

$$\frac{Q_1\left(t\right)}{C_1}+L_1\frac{d{F}_1\left(t\right)}{dt}=P\left(t\right)$$

(1)

$$\frac{Q_2\left(t\right)}{C_2}+L_2\frac{d{F}_2\left(t\right)}{dt}=\frac{Q_1\left(t\right)}{C_1}$$

where F(t) and P(t) mean the blood flow and the blood pressure of the parent artery, respectively. The quantity Q₁(t) and Q₂(t) denote the amount of blood inside the mother and daughter aneurysms, respectively. The quantity R is the resistance associated with the neck size, which is taken to be low for most of the blood flows going through the parent vessel. As the oscillation of flows possibly appear inside aneurysms, the flows F₁(t) and F₂(t) can be either positive, zero, or negative (for the default direction of the flows inside the aneurysms, see Figure 2).

2.2. Reduced Single Equation

Following Ref. [1], the solitonic Windkessel model (1) consisting of three equations is reduced to the single equation. Let local pressures P₁ and P₂ correspond to the pressures on the capacitor C₁ and C₂, respectively. Much attention is paid to the effect due to the existence of the daughter aneurysm. Here, blood pressure P(t) and the blood flow F(t) in the parent artery are assumed to be given. In this situation, the blood flows F₁(t) and F₂(t) inside the aneurysms are the unknowns, as well as the local pressures P₁(t) and P₂(t) inside the aneurysms. By substituting first and second equality of (1) to the third equality, the model equation is reduced to a single equation

$$\frac{d^2}{d{t}^2}{F}_2\left(t\right)+\frac{1}{C_2{L}_2}{F}_2\left(t\right)=\frac{d}{dt}\left[\frac{P\left(t\right)}{L_2}-\frac{L_1}{L_2}\frac{d}{dt}\left(F\left(t\right)-\frac{P\left(t\right)}{R}\right)\right]$$

(2)

It is the second-order ordinary differential equation. For this equation, external conditions arising from the heartbeat are given as:

F(t) = 10 sin(2πft) + F(0),

P(t) = 20 sin(2πft) + P(0),

where f = 70/60 [1/sec], F(0) = 50 [mL/min], and P(0) = 100 [mmHg] are the frequency of the heartbeat, initial blood flow, and initial blood pressure, respectively. The values follow from the typical blood flow and heart rate (the same setting as Ref. [1]). Indeed, for the human being, the typical blood flow of the middle cerebral artery is around 50 mL/min, the typical blood velocity is 60 cm/sec, the blood pressure is between 80 to 120 mmHg, and the heart rate is from 40 to 120 beat per minutes with mean value 70. In the natural situation, the blood flow in the parent artery (F + F₁) is not so different before and after aneurysmal formation. To realize this feature, the amount of artery blood flow is adjusted by choosing the value of R, and the resistance parameter R is fixed to R = 2.2 [mmHg min/mL] for all of the systematic calculations. Consequently, the initial condition is given by

F₂(0) = 0

$$\frac{d}{dt}{F}_2\left(t\right)=0$$

which means that no blood flow and no blood velocity are assumed to exist inside the daughter aneurysm at the beginning. Note that the constants L₁, L₂, C_1, and C₂ are assumed to be positive.

Since F(t) and P(t) are given by the heartbeat in this specific modelling, the right-hand side of Equation (2) is a given function, and unknown function is only F₂(t). If F₂(t) is obtained, Q₂(t) is automatically obtained by dQ₂(t)/dt = F₂(t), and then Q₁(t) follows from the third equality of Equation (1). P₂(t) is obtained by Q₂(t) = C₂(t) P₂(t). Next, F₁(t) is obtained by the second equality of Equation (1). Q₁(t) and P₁(t) are obtained similarly to Q₂(t) and P₂(t). Consequently, all the unknowns are obtained by solving single Equation (2). For the details of solitonic Windkessel model, see Ref. [1].

3. Result

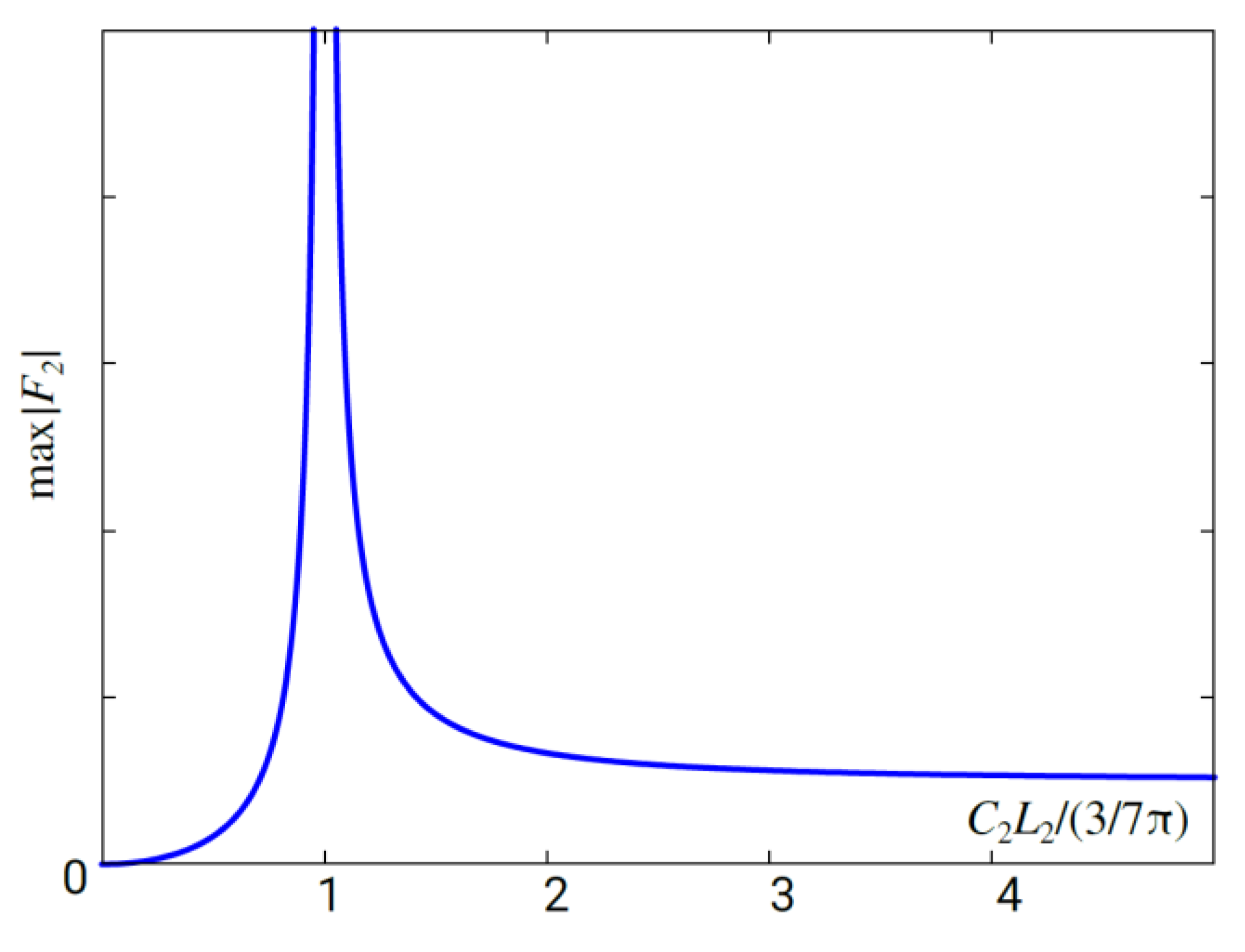
3.1. Analytic Solution

Although a systematic numerical research has been carried out in Ref. [1], here we present an analytical solution of solitonic Windkessel model in the given setting. The inhomogeneous term (the right-hand side of Equation (2)) is calculated as

$$\begin{array}{ll}\frac{d}{dt}\left[\frac{P\left(t\right)}{L_2}-\frac{L_1}{L_2}\frac{d}{dt}\left(F\left(T\right)-\frac{P\left(t\right)}{R}\right)\right]& =\frac{1}{L_2}\frac{dP\left(t\right)}{dt}-\frac{L_1}{L_2}\frac{d^{2}F\left(t\right)}{d{t}^2}+\frac{L_1}{R{L}_2}\frac{d^{2}P\left(t\right)}{d{t}^2}\\ & =\frac{140\pi }{3L_2}\cos \left(\frac{7\pi t}{3}\right)+\left(1-\frac{2}{2.2}\right)\frac{490{\pi }^2{L}_1}{9L_2}\sin \left(\frac{7\pi t}{3}\right)\\ & =\sqrt{{\left(\frac{140\pi }{3L_2}\right)}^2+{\left(\left(1-\frac{2}{2.2}\right)\frac{490{\pi }^2{L}_1}{9L_2}\right)}^2}\sin \left(\frac{7\pi t}{3}\right)+\phi \end{array}$$

where the initial phase angle $\phi$ is determined only by R and L₁; i.e., the specific feature of mother aneurysm.

$$\tan \phi =\frac{\frac{140\pi }{3L_2}}{\left(1-\frac{2}{2.2}\right)\frac{490{\pi }^2{L}_1}{9L_2}}=\frac{6}{7\pi \left(1-\frac{2}{R}\right) L_1}$$

In this case, as seen in the second term of the left-hand side of Equation (2), decisive factor of oscillation described by (2) is whether

$$C_2{L}_2$$

is positive or not (here it is always assumed to be positive). Oscillation appears for $C_2{L}_2$ > 0.

Another factor is

$$\frac{\sqrt{{\left(\frac{140\pi }{3L_2}\right)}^2+{\left(\left(1-\frac{2}{2.2}\right)\frac{490{\pi }^2{L}_1}{9L_2}\right)}^2}}{{\left(\frac{1}{C_2{L}_2}\right)}^2-{\left(\frac{7\pi }{3}\right)}^2}$$

which means the amplitude of a particular solution of Equation (2). That is, the resonance appears if the denominator

$${\left(\frac{1}{C_2{L}_2}\right)}^2-{\left(\frac{7\pi }{3}\right)}^2$$

is equal to zero. It corresponds to the violent rupture whose oscillation amplitude diverges rapidly. It is actually a tragic situation in which aneurysm rupture takes place even when no significant increase of local blood pressure is noticed. Consequently, the general solution of (2) is given by

$$F_2(\mathrm{t})=A\sin \left(\frac{t}{C_2{L}_2}\right)+B\cos \left(\frac{t}{C_2{L}_2}\right)$$

$$+\frac{\sqrt{{\left(\frac{140\pi }{3L_2}\right)}^2+{\left(\left(1-\frac{2}{2.2}\right)\frac{490{\pi }^2{L}_1}{9L_2}\right)}^2}}{{\left(\frac{1}{C_2{L}_2}\right)}^2-{\left(\frac{7\pi }{3}\right)}^2}\sin \left(\frac{7\pi t}{3}+\phi \right),$$

(3)

where A and B are constants being determined by the initial condition:

$$\begin{gathered} A=-\frac{7\pi }{3}{C}_2{L}_2 \frac{\sqrt{{\left(\frac{140\pi }{3L_2}\right)}^2+{\left(\left(1-\frac{2}{2.2}\right)\frac{490{\pi }^2{L}_1}{9L_2}\right)}^2}}{{\left(\frac{1}{C_2{L}_2}\right)}^2-{\left(\frac{7\pi }{3}\right)}^2}\cos \phi , \\ B=-\frac{\sqrt{{\left(\frac{140\pi }{3L_2}\right)}^2+{\left(\left(1-\frac{2}{2.2}\right)\frac{490{\pi }^2{L}_1}{9L_2}\right)}^2}}{{\left(\frac{1}{C_2{L}_2}\right)}^2-{\left(\frac{7\pi }{3}\right)}^2}\sin \phi . \end{gathered}$$

We have the same denominator for the three terms consisting of the right-hand side of Equation (3).

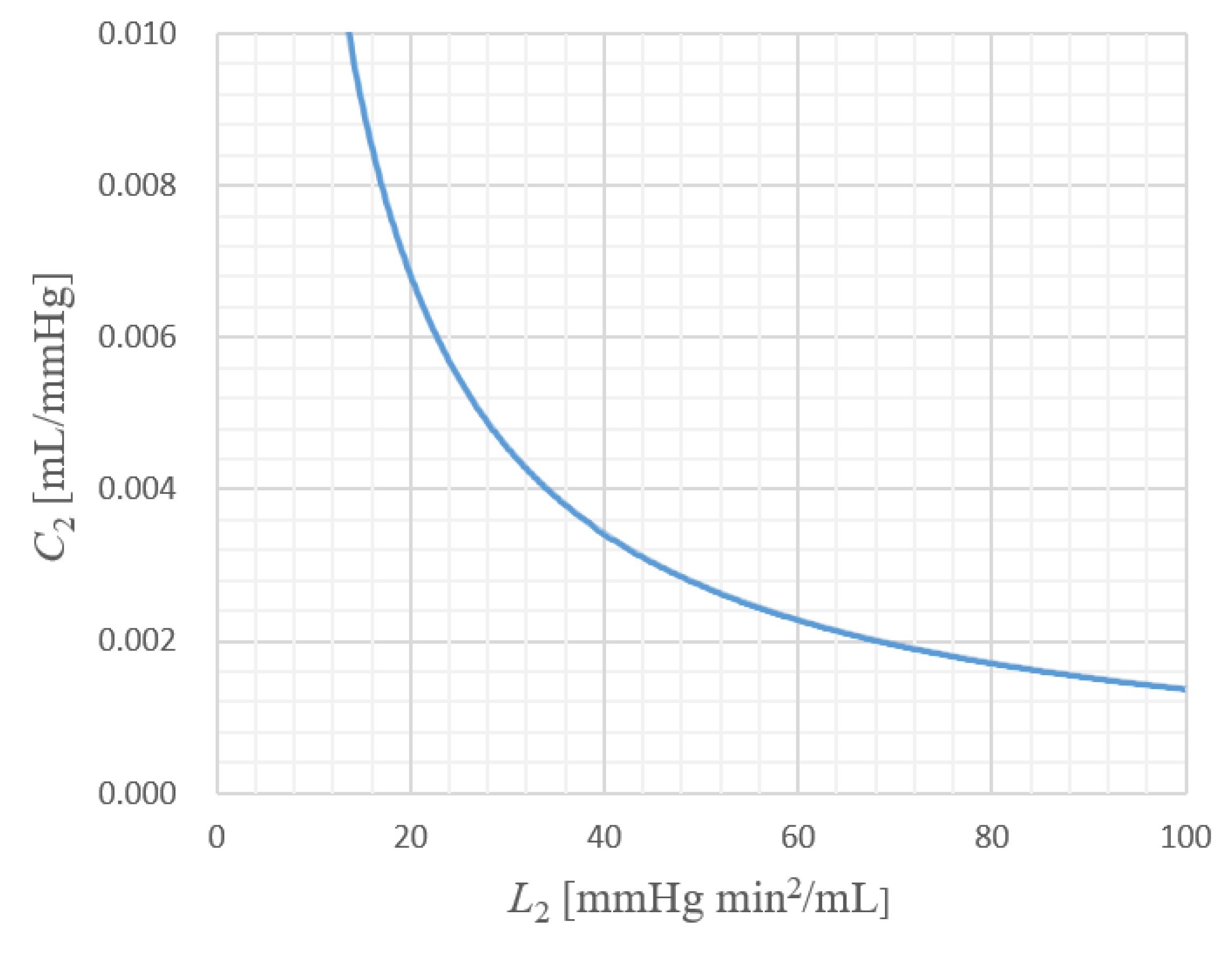
3.2. Condition for the Resonance

The condition for the appearance of resonance is represented by

$$C_2{L}_2=\frac{3}{7\pi }$$

(4)

in the present settings (Figure 3). It arises from equality for the denominator seen in Equation (3): ${\left(1/C_2{L}_2\right)}^2-{\left(7\pi /3\right)}^2=0$. A constant 3/7$\mathsf{\pi }$ in the right-hand side of Equation (4) arises from the heartbeat, and the left-hand side arises from the specific feature of daughter aneurysm. It is worth reminding here the physical meaning of $C_2$ and $L_2$ at this point. $C_2$ is the compliance of daughter aneurysm, and $L_2$ is the inductance of daughter aneurysm. More concretely, $C_2$ is the volume of daughter aneurysm, and $L_2$ is the thinness of the blood wall of daughter aneurysm. Most importantly, it is readily seen from the condition (4) that the appearance of resonance is dependent only on the property of daughter aneurysm. In other words, formation of the daughter aneurysm (i.e., two-story aneurysm) possibly brings about unexpected and sudden aneurysmal rupture.

According to Figure 4 of Ref. [1], values of $L_2$ range from 30 to 100 [mmHg min²/mL]. The condition tells us the value of $C_2$ at the resonance ranges from 0.002 to 0.004 [mL/mmHg]. Since the standard value of $C_1$ (=standard size of mother aneurysm) is taken equal to 0.10 [mL/mmHg] in Ref. [1], in terms of adjusting the possible increase of local pressure inside aneurysms, daughter aneurysms with their volumes 2% or 3% of mother aneurysm, are exposed to the resonance. The size of the daughter aneurysm is not so large in this situation, and therefore the rupture takes place unexpectedly.

4. Conclusions

Based on the solitonic Windkessel model, the condition for unexpected and sudden aneurysmal rupture is obtained by Equation (4). It arises from the flow-mediated resonance. There are several discoveries to be noted for interpreting this type of rupture:

• the sudden rupture appears only when the two-story intracranial aneurysm (so called, dumbbell-shaped aneurysm) is formed;
• the sudden rupture appears even when the daughter aneurysm is not so growing up well, where rupture condition reported in the preceding work [1] claims that rupture appears if the size of daughter aneurysm is comparable to that of mother aneurysm;
• the sudden rupture does not arise from the increase of local/global blood pressure, but rather from the sudden increase of the local blood flow inside daughter aneurysm, where rupture condition reported in the preceding work [1] takes into account ruptures only caused from the increase of local pressure.

According to the second point of the above, the mechanisms in the preceding work, such as the soliton propagation and resonating beat effect, are less useful to explain unexpected aneurysmal ruptures. On the other hand, most of the aneurysmal ruptures appear unexpectedly even without a significant increase of blood pressure. The rupture of resonance type will play a role in explaining such unexpected ruptures.