Vapor Composition and Vaporization Thermodynamics of 1-Ethyl-3-methylimidazolium Hexafluorophosphate Ionic Liquid
Abstract
1. Introduction
2. Materials and Methods
3. Results and Discussion
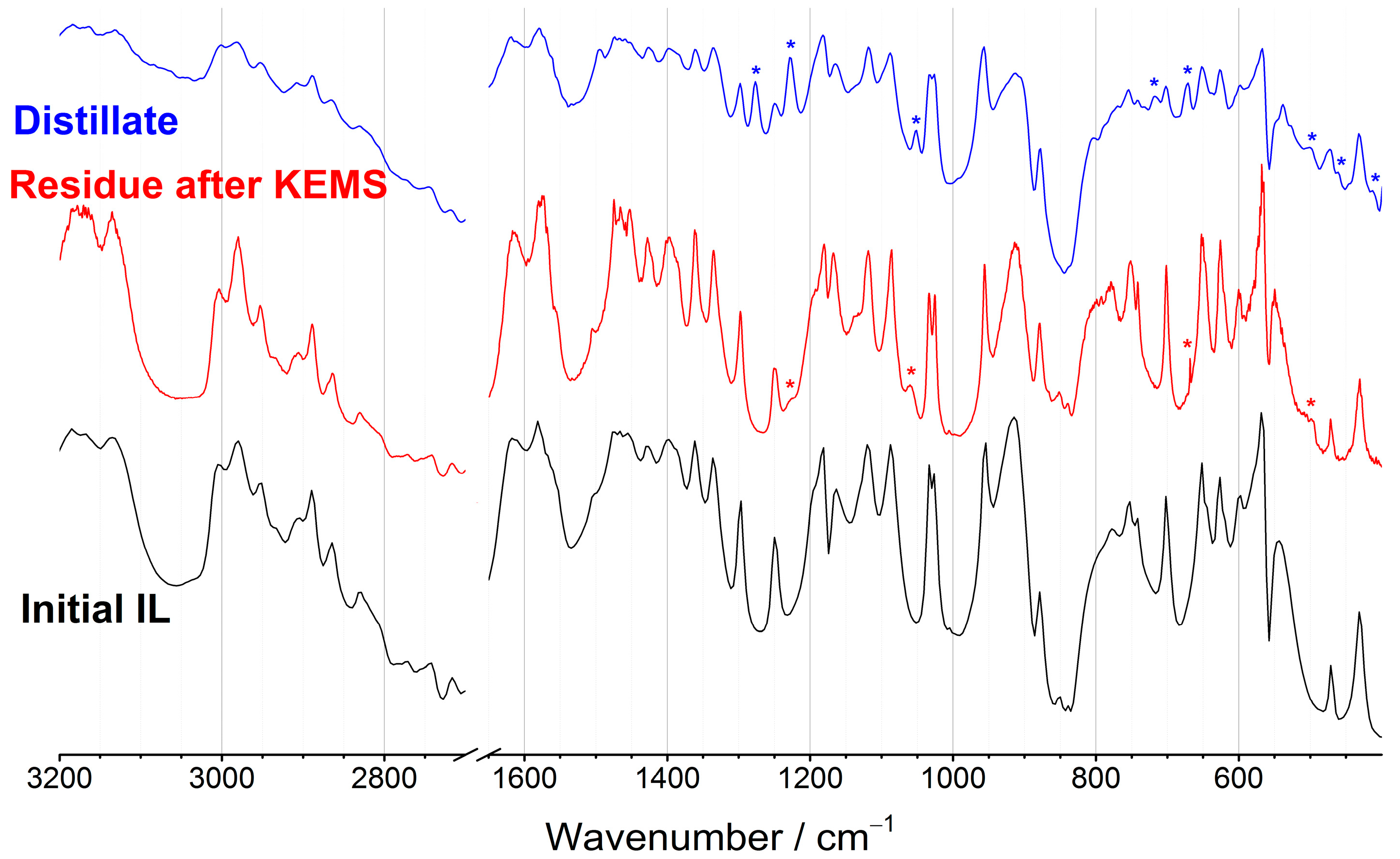
3.1. IR Spectroscopy
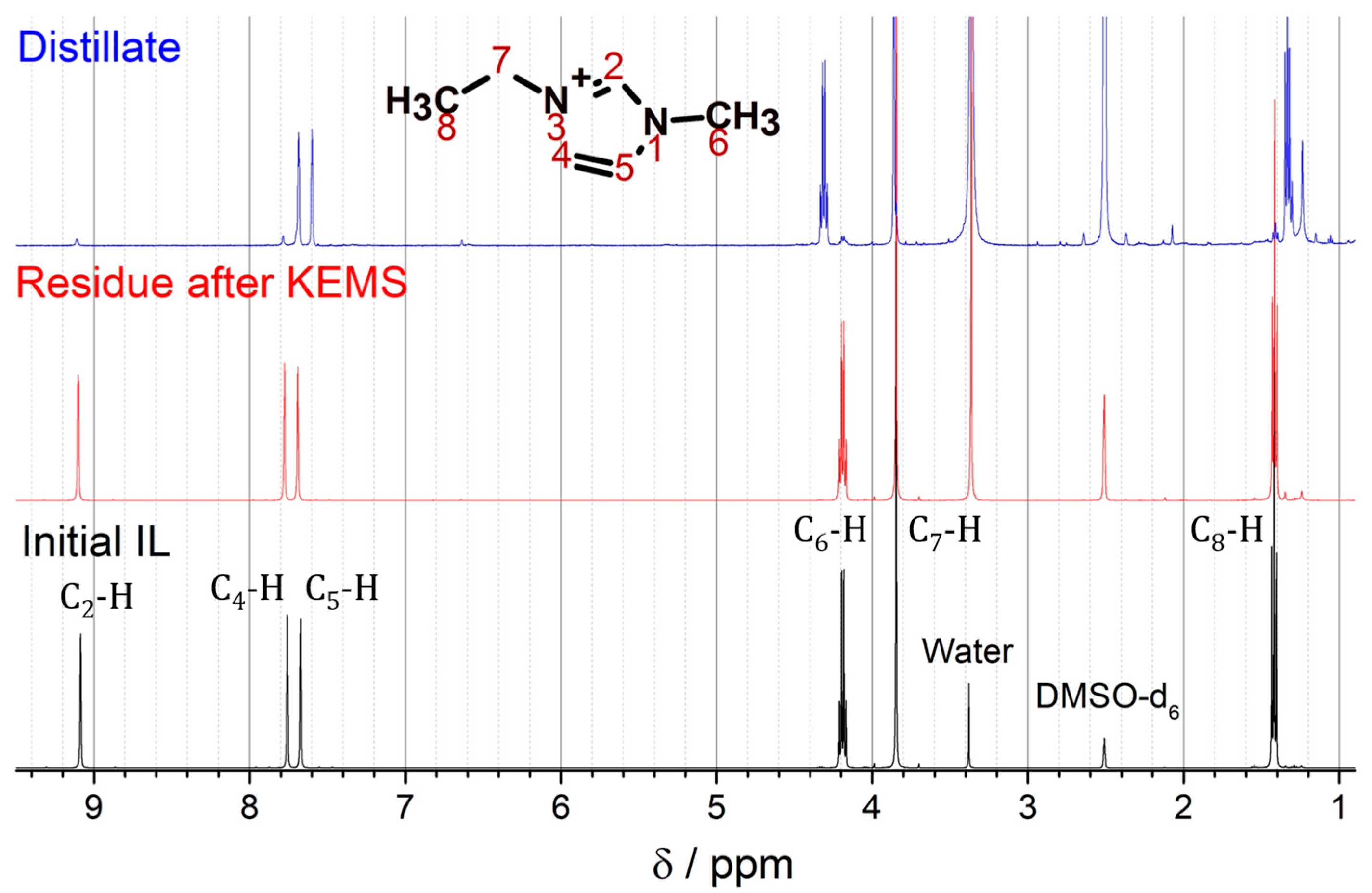
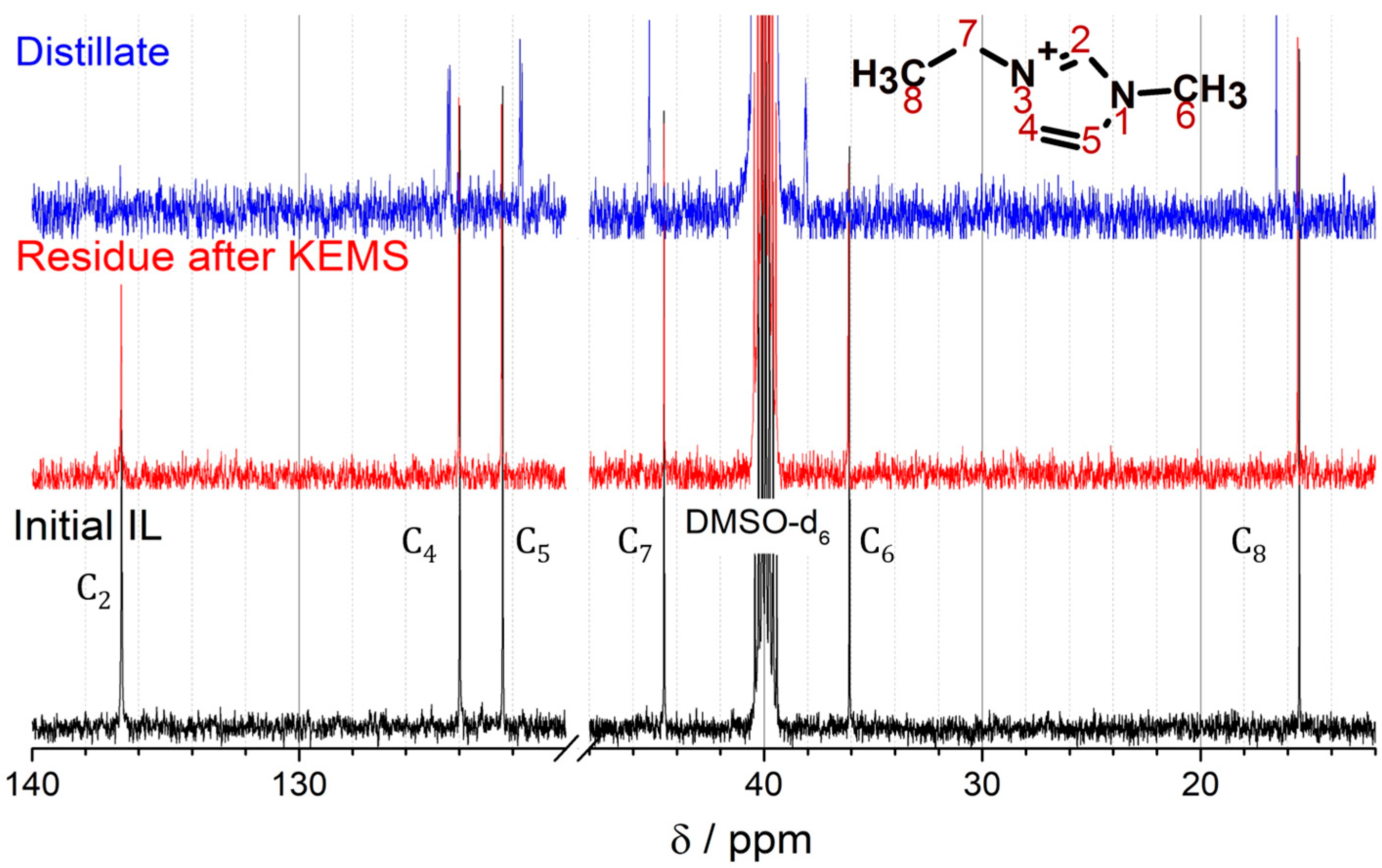
3.2. NMR-Analysis
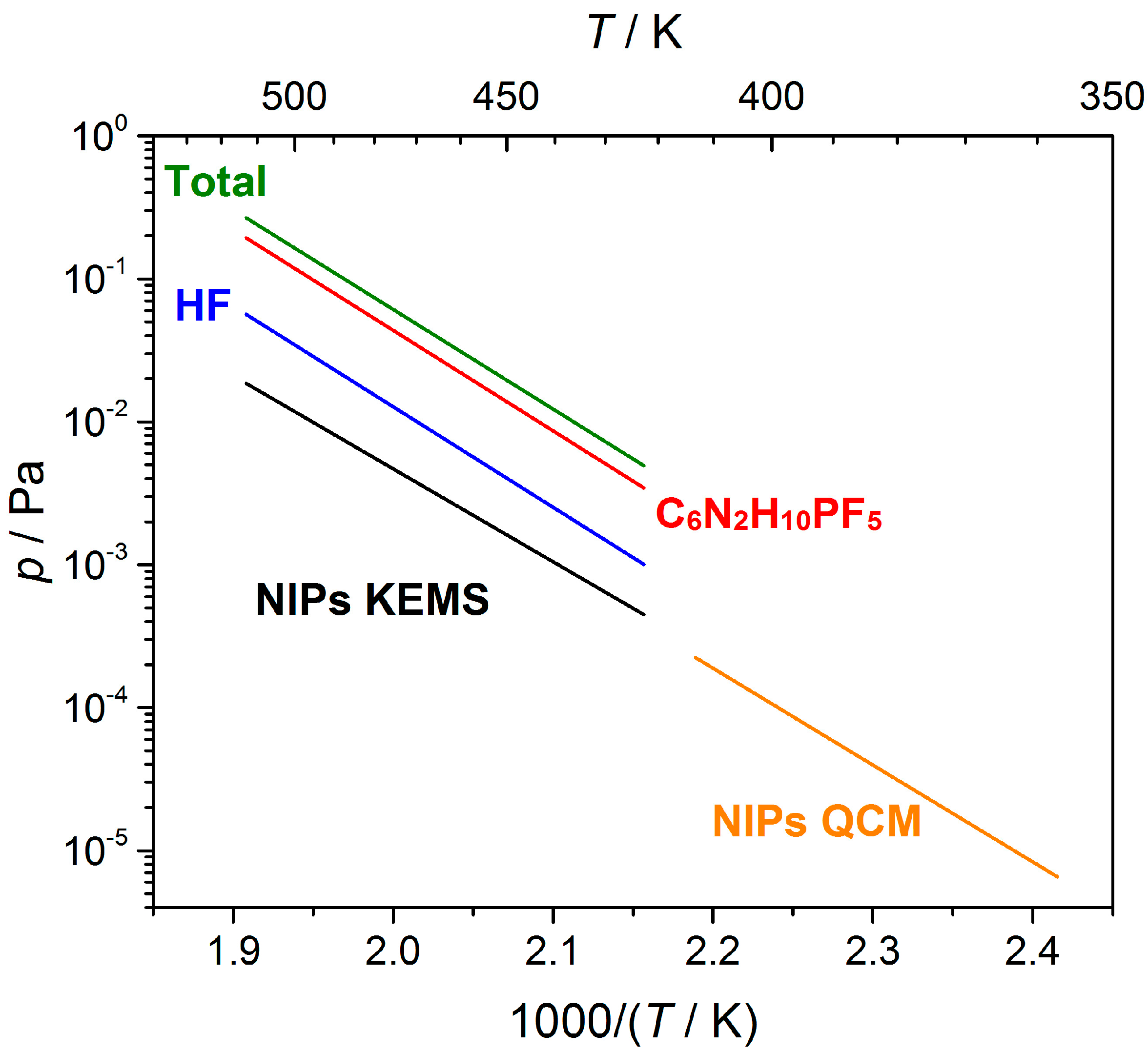
3.3. KEMS
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Taylor, A.W.; Lovelock, K.R.J.; Jones, R.G.; Licence, P. Borane-substituted imidazol-2-ylidenes: Syntheses in vacuo. Dalton Trans. 2011, 40, 1463–1470. [Google Scholar] [CrossRef] [PubMed]
- Dunaev, A.M.; Motalov, V.B.; Kudin, L.S. The Composition of Saturated Vapor Over 1-Butyl-3-Methylimidazolium Tetrafluoroborate Ionic Liquid: A Multi-Technique Study of the Vaporization Process. Entropy 2021, 23, 1478. [Google Scholar] [CrossRef] [PubMed]
- Dunaev, A.M.; Motalov, V.B.; Kudin, L.S.; Zhabanov, Y.A.; Aleksandriiskii, V.V.; Govorov, D.N. Evaporation Thermodynamics of 1-Butyl-3-methylimidazolium Tetrafluoroborate Ionic Liquid. J. Mol. Liq. 2023, 380, 121626. [Google Scholar] [CrossRef]
- Clarke, C.J.; Puttick, S.; Sanderson, T.J.; Taylor, A.W.; Bourne, R.A.; Lovelock, K.R.J.; Licence, P. Thermal stability of dialkylimidazolium tetrafluoroborate and hexafluorophosphate ionic liquids: Ex situ bulk heating to complement in situ mass spectrometry. Phys. Chem. Chem. Phys. 2018, 20, 16786–16800. [Google Scholar] [CrossRef] [PubMed]
- Chambreau, S.D.; Schenk, A.C.; Sheppard, A.J.; Yandek, G.R.; Vaghjiani, G.L.; Maciejewski, J.; Koh, C.J.; Golan, A.; Leone, S.R. Thermal Decomposition Mechanisms of Alkylimidazolium Ionic Liquids with Cyano-Functionalized Anions. J. Phys. Chem. A 2014, 118, 11119–11132. [Google Scholar] [CrossRef]
- Volpe, V.; Brunetti, B.; Gigli, G.; Lapi, A.; Vecchio Ciprioti, S.; Ciccioli, A. Toward the Elucidation of the Competing Role of Evaporation and Thermal Decomposition in Ionic Liquids: A Multitechnique Study of the Vaporization Behavior of 1-Butyl-3-methylimidazolium Hexafluorophosphate under Effusion Conditions. J. Phys. Chem. B 2017, 121, 10382–10393. [Google Scholar] [CrossRef]
- Zaitsau, D.H.; Yermalayeu, A.V.; Emel’yanenko, V.N.; Butler, S.; Schubert, T.; Verevkin, S.P. Thermodynamics of Imidazolium-Based Ionic Liquids Containing PF6 Anions. J. Phys. Chem. B. 2016, 120, 7949–7957. [Google Scholar] [CrossRef]
- Vila, J.; Fernandez-Castro, B.; Rilo, E.; Carrete, J.; Dominguez-Perez, M.; Rodriguez, J.R.; Garcia, M.; Varela, L.M.; Cabeza, O. Liquid-solid-liquid phase transition hysteresis loops in the ionic conductivity of ten imidazolium-based ionic liquids. Fluid Phase Equilib. 2012, 320, 1–10. [Google Scholar] [CrossRef]
- Dunaev, A.M.; Kryuchkov, A.S.; Kudin, L.S.; Butman, M.F. Automatic complex for high temperature investigation on basis of mass spectrometer MI1201. Izv. Vyssh. Uchebn. Zaved. Khim. Khim. Tekhnol 2011, 54, 73–77. (In Russian) [Google Scholar]
- Sergeev, D.N.; Dunaev, A.M.; Ivanov, D.A.; Golovkina, Y.A.; Gusev, G.I. Automatization of mass spectrometer for the obtaining of ionization efficiency functions. Prib. I Tekhnika Eksperimenta. 2014, 1, 139–140. (In Russian) [Google Scholar] [CrossRef]
- Dunaev, A.M.; Motalov, V.B.; Kudin, L.S. A high-temperature mass-spectrometric method for determination of the electron work function of ionic crystals: Lanthanum, cerium, and praseodymium triiodides. Russ. J. Gen. Chem. 2017, 87, 632–638. [Google Scholar] [CrossRef]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian 16, Revision C.01. In Gaussian; Gaussian Inc.: Wallingford, CT, USA, 2016. [Google Scholar]
- Curtiss, L.A.; Redfern, P.C.; Raghavachari, K. Gaussian-4 theory. J. Chem. Phys. 2007, 126, 084108. [Google Scholar] [CrossRef]
- Govorov, D.N.; Dunaev, A.M.; Motalov, V.B.; Kudin, L.S. Quantum chemical calculations of formation enthalpies of cations and anions of ionic liquids. J. Mol. Liq. 2022, 364, 119996. [Google Scholar] [CrossRef]
- Dunaev, A.M.; Kudin, L.S. Statthermo®–New software for calculation of thermodynamic functions. Izv. Vyssh. Uchebn. Zaved. Khim. Khim. Tekhnol. 2017, 60, 40–46. [Google Scholar] [CrossRef]
- Chao, J.; Hall, K.R.; Marsh, K.N.; Wilhoit, R.C. Thermodynamic properties of key organic oxygen compounds in the carbon range C1 to C4. Part 2. Ideal gas properties. J. Phys. Chem. Ref. Data. 1986, 15, 1369–1436. [Google Scholar] [CrossRef]
- Katsyuba, S.A.; Zvereva, E.E.; Vidiš, A.; Dyson, P.J. Application of Density Functional Theory and Vibrational Spectroscopy Toward the Rational Design of Ionic Liquids. J. Phys. Chem. A 2007, 111, 352–370. [Google Scholar] [CrossRef]
- Deyko, A.; Lovelock, K.R.J.; Licence, P.; Jones, R.G. The vapour of imidazolium-based ionic liquids: A mass spectrometry study. Phys. Chem. Chem. Phys. 2011, 13, 16841–16850. [Google Scholar] [CrossRef]
- Dunaev, A.M.; Motalov, V.B.; Govorov, D.N.; Kudin, L.S. Dimer neutral ion pairs and associative ions in saturated vapor of 1-ethyl-3-methylimidazolium trifluoromethanesulfonate ionic liquid. Calphad 2019, 65, 127–131. [Google Scholar] [CrossRef]
- Lias, S.G.; Bartmess, J.E.; Liebman, J.F.; Holmes, J.L.; Levin, R.D.; Mallard, W.G. Ion Energetics Data. In NIST Chemistry WebBook, NIST Standard Reference Database Number 69; Linstrom, P.J., Mallard, W.G., Eds.; National Institute of Standards and Technology: Gaithersburg, MD, USA, 2022; p. 20899. Available online: https://webbook.nist.gov/chemistry/ (accessed on 25 May 2023). [CrossRef]
- Motalov, V.B.; Korobov, M.A.; Dunaev, A.M.; Dunaeva, V.V.; Kudin, L.S. Vapor pressure and thermodynamics of L-tryptophan sublimation. Russ. J. Gen. Chem. 2021, 91, 1938–1945. [Google Scholar] [CrossRef]
- Serra, P.B.P. Thermal Behaviour and Heat Capacity of Ionic Liquids: Benzilimidazolium and Alkylimidazolium Derivatives. Master’s Thesis, Universidade do Porto, Porto, Portugal, 2013. [Google Scholar]
- Serra, P.B.P.; Ribeiro, F.M.S.; Rocha, M.A.A.; Fulem, M.; Růžička, K.; Coutinho, J.A.P.; Santos, L.M.N.B.F. Solid-liquid equilibrium and heat capacity trend in the alkylimidazolium PF6 series. J. Mol. Liq. 2017, 248, 678–687. [Google Scholar] [CrossRef]
- Holbrey, J.D.; Reichert, W.M.; Reddy, R.G.; Rogers, R.D. Heat Capacities of Ionic Liquids and Their Applications as Thermal Fluids. In Ionic Liquids as Green Solvents; American Chemical Society: Washington, DC, USA, 2003; Volume 856, pp. 121–133. [Google Scholar] [CrossRef]
- Glasser, L.; Jenkins, H.D.B. Predictive thermodynamics for ionic solids and liquids. Phys. Chem. Chem. Phys. 2016, 18, 21226. [Google Scholar] [CrossRef] [PubMed]
- Fuller, J.; Carlin, R.T.; De Long, H.C.; Haworth, D. Structure of 1-ethyl-3-methylimidazolium hexafluorophosphate: Model for room temperature molten salts. J. Chem. Soc. Chem. Commun. 1994, 3, 299–300. [Google Scholar] [CrossRef]
- Belov, G.V.; Iorish, V.S.; Yungman, V.S. IVTANTHERMO for Windows–Database on thermodynamic properties and related software CALPHAD. Comput. Coupling Phase Diagrams Thermochem. 1999, 23, 173–180. [Google Scholar] [CrossRef]
- Lemmon, E.W.; McLinden, M.O.; Friend, D.G. NIST Chemistry WebBook, NIST Standard Reference Database, 2017. Available online: https://webbook.nist.gov/chemistry/ (accessed on 25 May 2023). [CrossRef]
- Acree, W., Jr.; Chickos, J.S. Phase Transition Enthalpy Measurements of Organic and Organometallic Compounds. Sublimation, Vaporization and Fusion Enthalpies From 1880 to 2015. Part 1. C1–C10. J. Phys. Chem. Ref. Data. 2016, 45, 033101. [Google Scholar] [CrossRef]
- Zaitsau, D.H.; Kabo, G.J.; Strechan, A.A.; Paulechka, Y.U.; Tschersich, A.; Verevkin, S.P.; Heintz, A. Experimental Vapor Pressures of 1-Alkyl-3-methylimidazolium Bis(trifluoromethylsulfonyl)imides and a Correlation Scheme for Estimation of Vaporization Enthalpies of Ionic Liquids. J. Phys. Chem. A. 2006, 110, 7303–7306. [Google Scholar] [CrossRef]
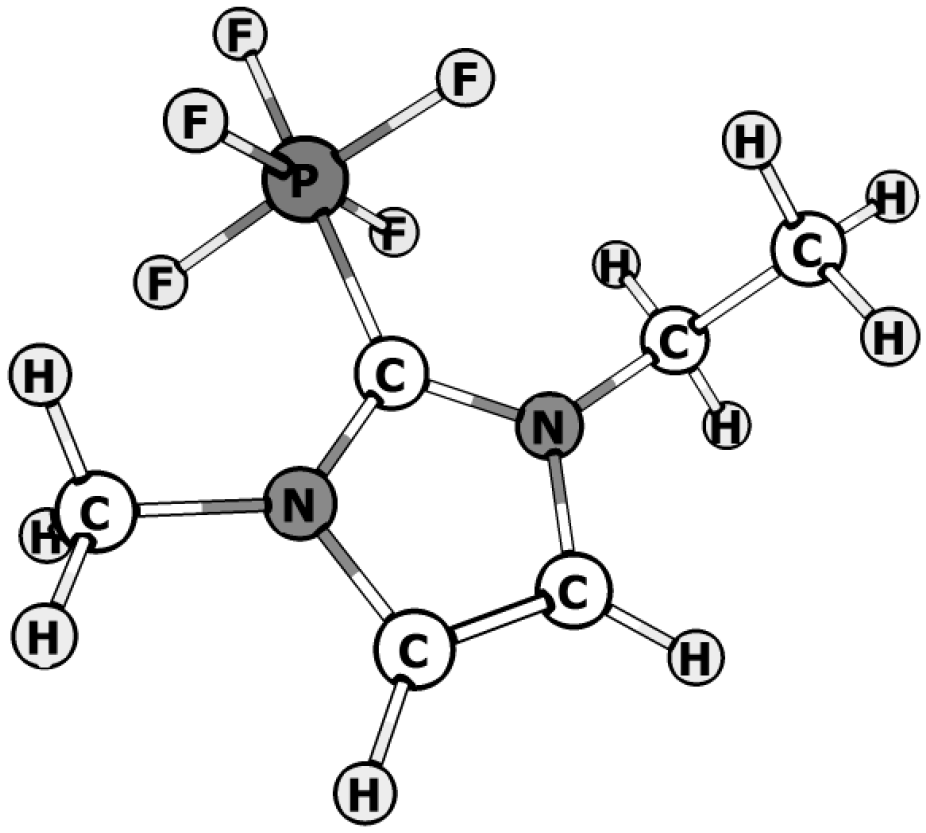


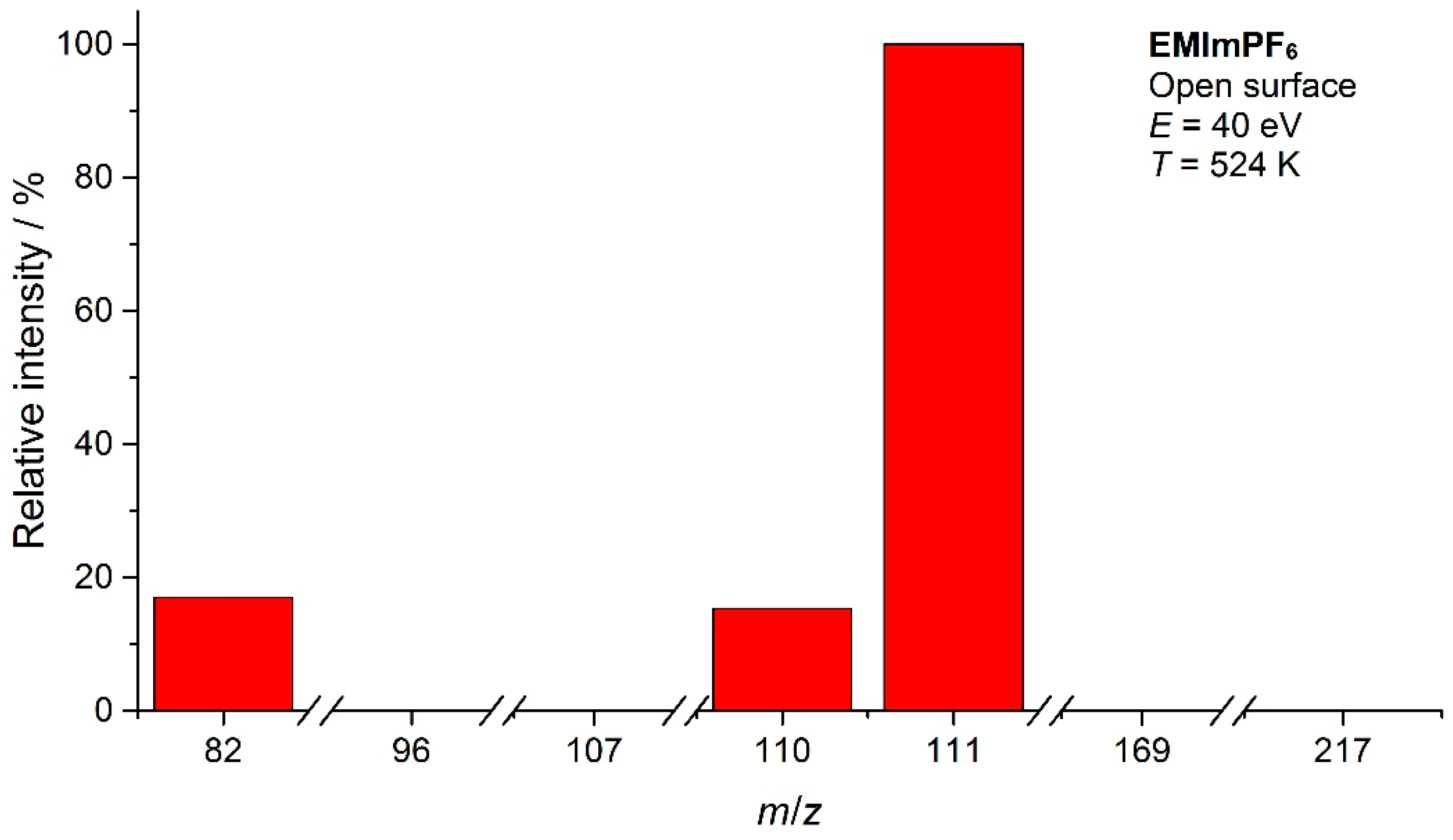
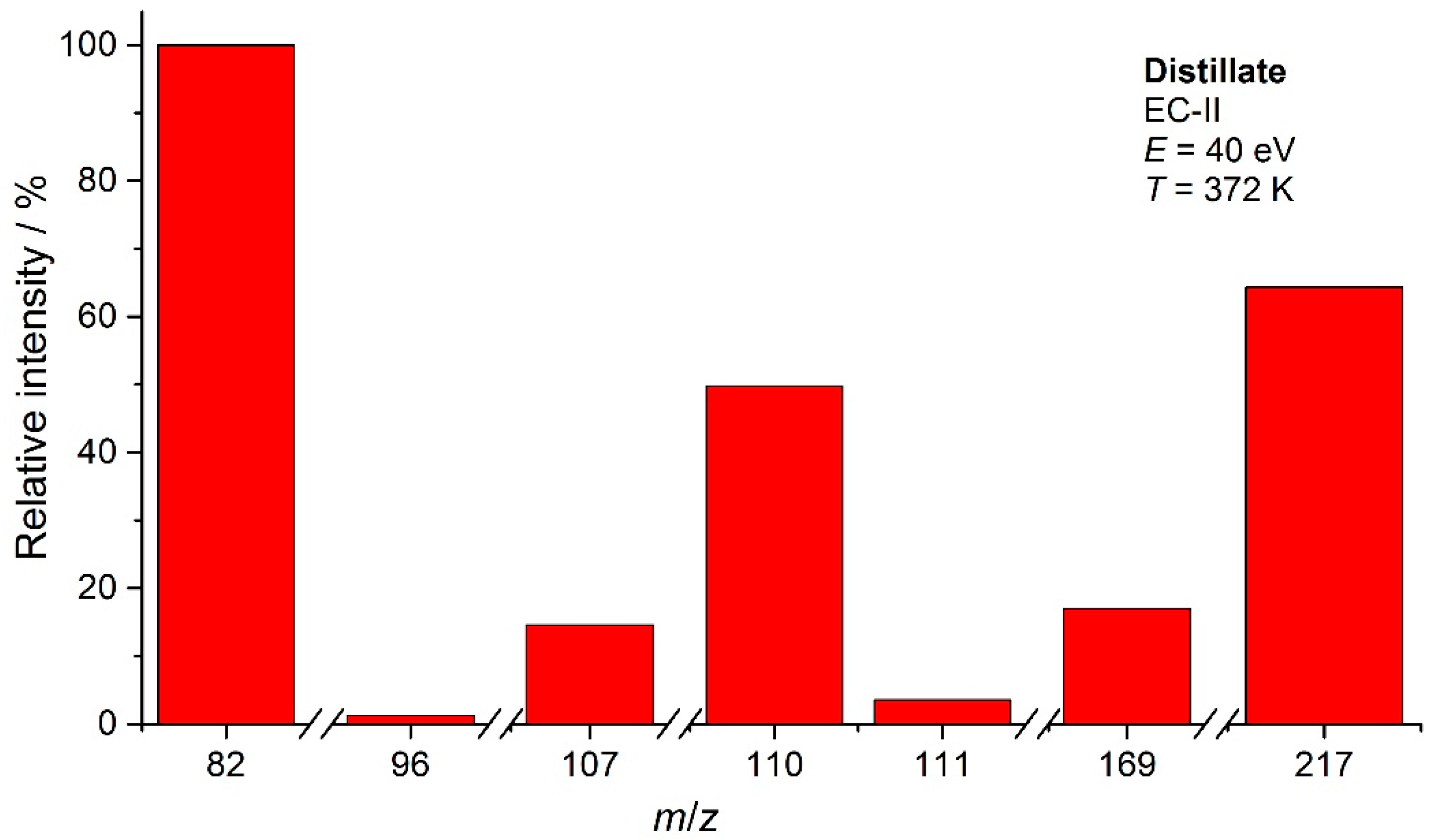
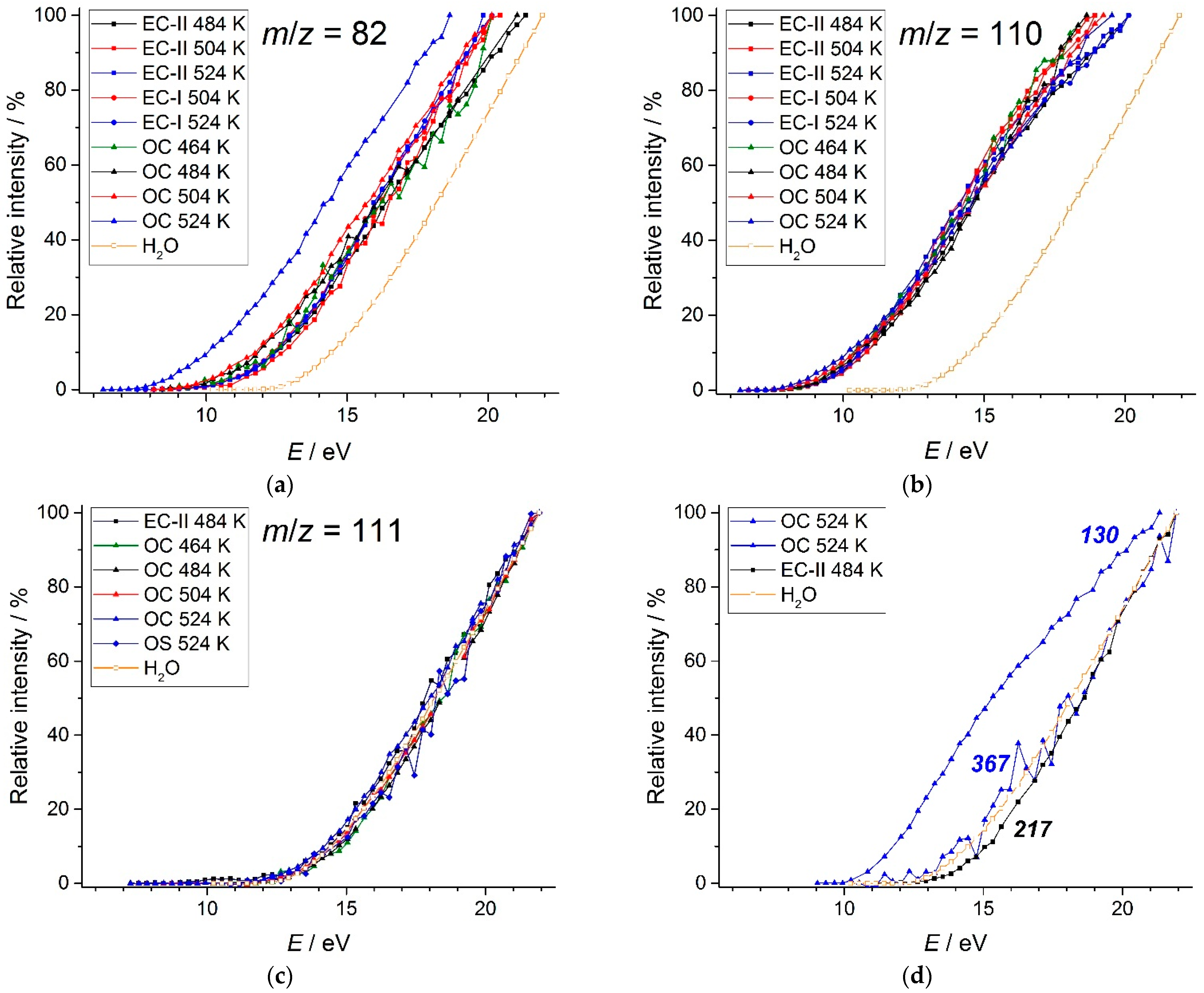
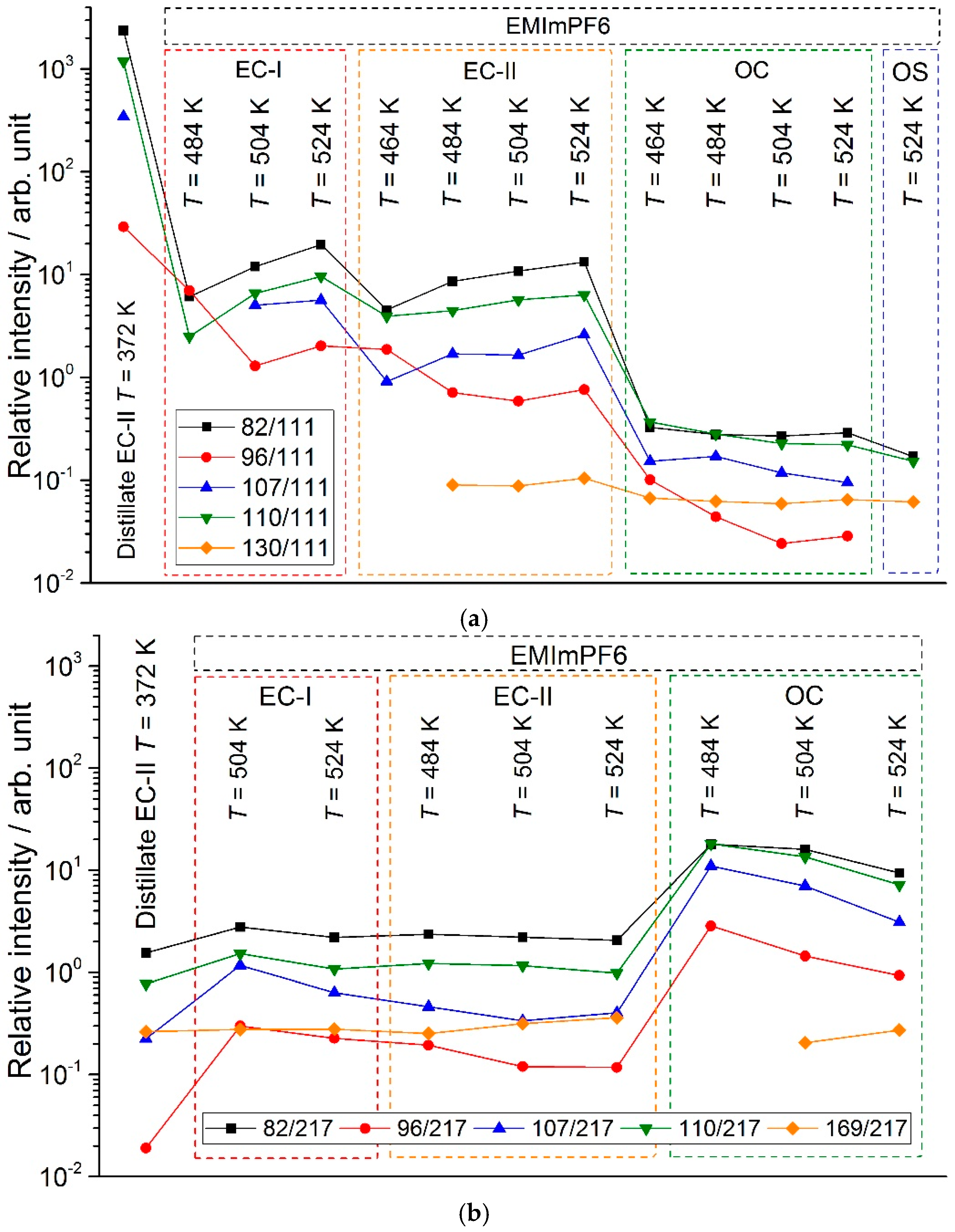
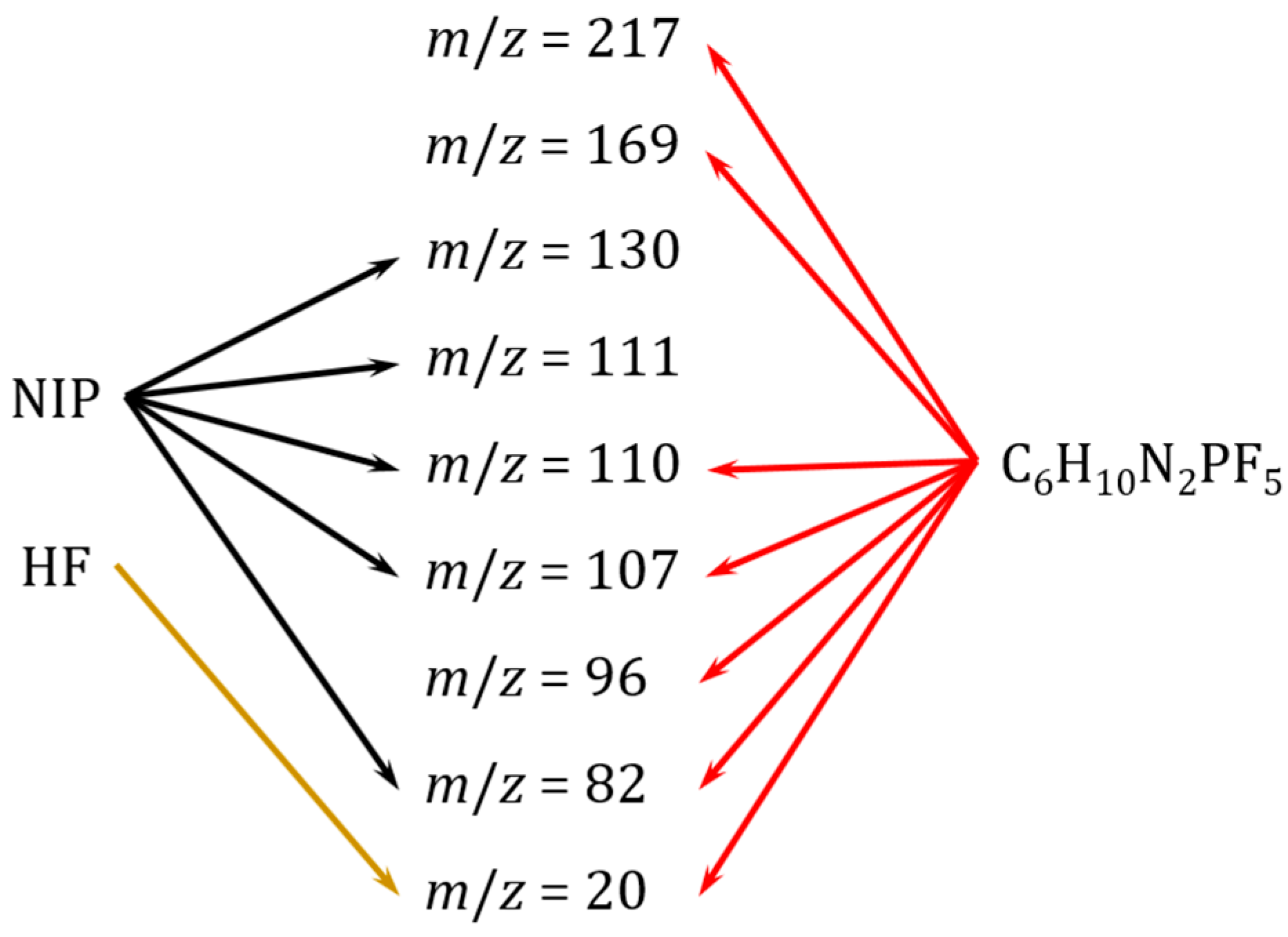
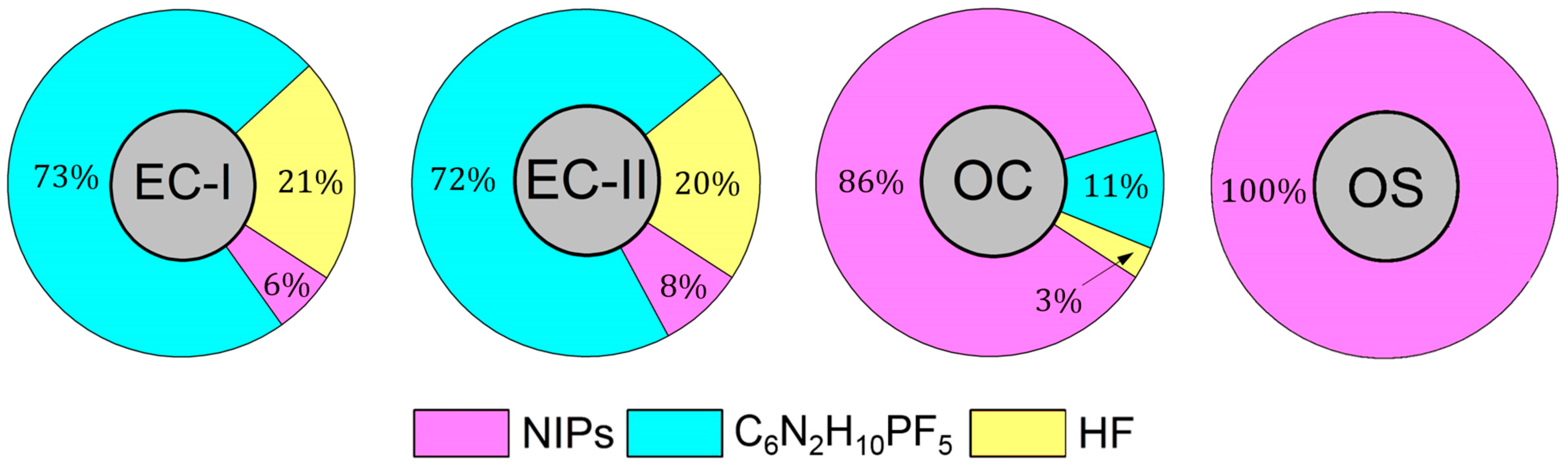

| Conditions | T/K | m/z | ||
|---|---|---|---|---|
| 82 | 110 | 111 | ||
| IL | ||||
| EC-I | 504 | 10.0 | 8.5 | |
| 524 | 10.0 | 8.5 | ||
| EC-II | 484 | 9.8 | 8.8 | 12.1 |
| 504 | 10.2 | 8.8 | ||
| 524 | 10.1 | 8.6 | ||
| OC | 464 | 9.5 | 8.6 | 12.4 |
| 484 | 9.5 | 8.6 | 12.4 | |
| 504 | 9.4 | 8.3 | 12.3 | |
| 524 | 7.9 | 8.0 | 12.0 | |
| OS | 524 | 11.9 | ||
| Distillate | ||||
| EC-II | 374 | 11.4 | 9.6 | |
| Conditions | EC-I | EC-II | OC |
|---|---|---|---|
| ΔT/K | 474–511 | 463–523 | 454–525 |
| m/z | |||
| 82 | −16.457 ± 0.763 | −17.349 ± 0.498 | −15.240 ± 0.379 |
| 110 | −15.636 ± 0.544 | −14.732 ± 0.375 | −14.702 ± 0.232 |
| 111 | −14.049 ± 0.227 | −14.910 ± 0.209 | −14.634 ± 0.245 |
| 130 | −14.242 ± 0.234 | ||
| 217 | −16.839 ± 1.097 | −16.248 ± 0.943 |
| T/K | NIPs | C6N2H10PF5 | HF |
|---|---|---|---|
| 524 | 16.0 | 157 | 45.6 |
| 514 | 10.3 | 117 | 34.0 |
| 505 | 6.60 | 79.0 | 23.0 |
| 494 | 3.51 | 30.9 | 8.99 |
| 484 | 1.73 | 16.1 | 4.70 |
| 474 | 0.93 | 6.93 | 2.02 |
| 464 | 0.37 | 4.84 | 1.41 |
| 469 | 0.63 | 3.92 | 1.14 |
| 479 | 1.30 | 8.01 | 2.33 |
| 488 | 2.51 | 17.3 | 5.04 |
| 499 | 4.44 | 41.6 | 12.1 |
| Vapor Species | a | b |
|---|---|---|
| Ionic liquid (464–524 K) | ||
| NIPs | −14.977 ± 0.380 | 24.592 ± 0.776 |
| C6N2H10PF5 | −16.184 ± 0.867 | 29.239 ± 1.772 |
| HF | −16.184 ± 0.867 | 28.005 ± 1.772 |
| Distillate (332–374 K) | ||
| C6N2H10PF5 | −10.384 ± 0.166 | 25.892 ± 0.475 |
| Compound | ΔT/K | a | b, ·10−3 | c | d, ·103 | e, ·104 | f, ·104 |
|---|---|---|---|---|---|---|---|
| EMImPF6, s | 298.15–334 | 1998 | −1035 | 170.5 | 154.2 | −240.3 | 1340 |
| EMImPF6, s | 334–600 | 9.847 | −8.787 | 5.077 | 8.156 | −2.304 | −5.235 |
| EMImPF6, g | 298.15–600 | −74.95 | 7.229 | 2.427 | 7.009 | −2.240 | 4.391 |
| C6N2H10PF5, g | 298.15–600 | −63.76 | 5.581 | 2.336 | 6.232 | −1.911 | 3.562 |
| ΔT | T | N | ΔrH°(T) | ΔrH°(298.15 K) | ΔrS°(T) | Method 3 | Conditions | Ref. | ||
|---|---|---|---|---|---|---|---|---|---|---|
| II Law 1 | III Law 2 | II Law 1 | III Law 2 | |||||||
| EMImPF6, s,l = EMImPF6, g | ||||||||||
| 464–524 | 490 | 11 | 125 ± 3 | 156 ± 4 | 152 ± 14 | 109 ± 4 | 102 ± 18 | KEMS | EC-II | this work |
| 414–457 | 436 | 18 | 130 ± 1 | 158 ± 2 | 153 ± 14 | 118 ± 1 | 108 ± 18 | QCM | OS | [7] |
| EMImPF6, g = C6N2H10PF5, g + HF, g | ||||||||||
| 464–524 | 490 | 11 | 145 ± 8 | 144 ± 9 | 112 ± 14 | 176 ± 24 | 110 ± 18 | KEMS | EC-II | this work |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dunaev, A.M.; Motalov, V.B.; Korobov, M.A.; Govorov, D.; Aleksandriiskii, V.V.; Kudin, L.S. Vapor Composition and Vaporization Thermodynamics of 1-Ethyl-3-methylimidazolium Hexafluorophosphate Ionic Liquid. AppliedChem 2023, 3, 303-319. https://doi.org/10.3390/appliedchem3020019
Dunaev AM, Motalov VB, Korobov MA, Govorov D, Aleksandriiskii VV, Kudin LS. Vapor Composition and Vaporization Thermodynamics of 1-Ethyl-3-methylimidazolium Hexafluorophosphate Ionic Liquid. AppliedChem. 2023; 3(2):303-319. https://doi.org/10.3390/appliedchem3020019
Chicago/Turabian StyleDunaev, Anatoliy M., Vladimir B. Motalov, Mikhail A. Korobov, Dmitrii Govorov, Victor V. Aleksandriiskii, and Lev S. Kudin. 2023. "Vapor Composition and Vaporization Thermodynamics of 1-Ethyl-3-methylimidazolium Hexafluorophosphate Ionic Liquid" AppliedChem 3, no. 2: 303-319. https://doi.org/10.3390/appliedchem3020019
APA StyleDunaev, A. M., Motalov, V. B., Korobov, M. A., Govorov, D., Aleksandriiskii, V. V., & Kudin, L. S. (2023). Vapor Composition and Vaporization Thermodynamics of 1-Ethyl-3-methylimidazolium Hexafluorophosphate Ionic Liquid. AppliedChem, 3(2), 303-319. https://doi.org/10.3390/appliedchem3020019








