Simulations of Temperature-Dependent Magnetization in FexGd100−x (20 ≤ x ≤ 80) Alloys
Abstract
1. Introduction
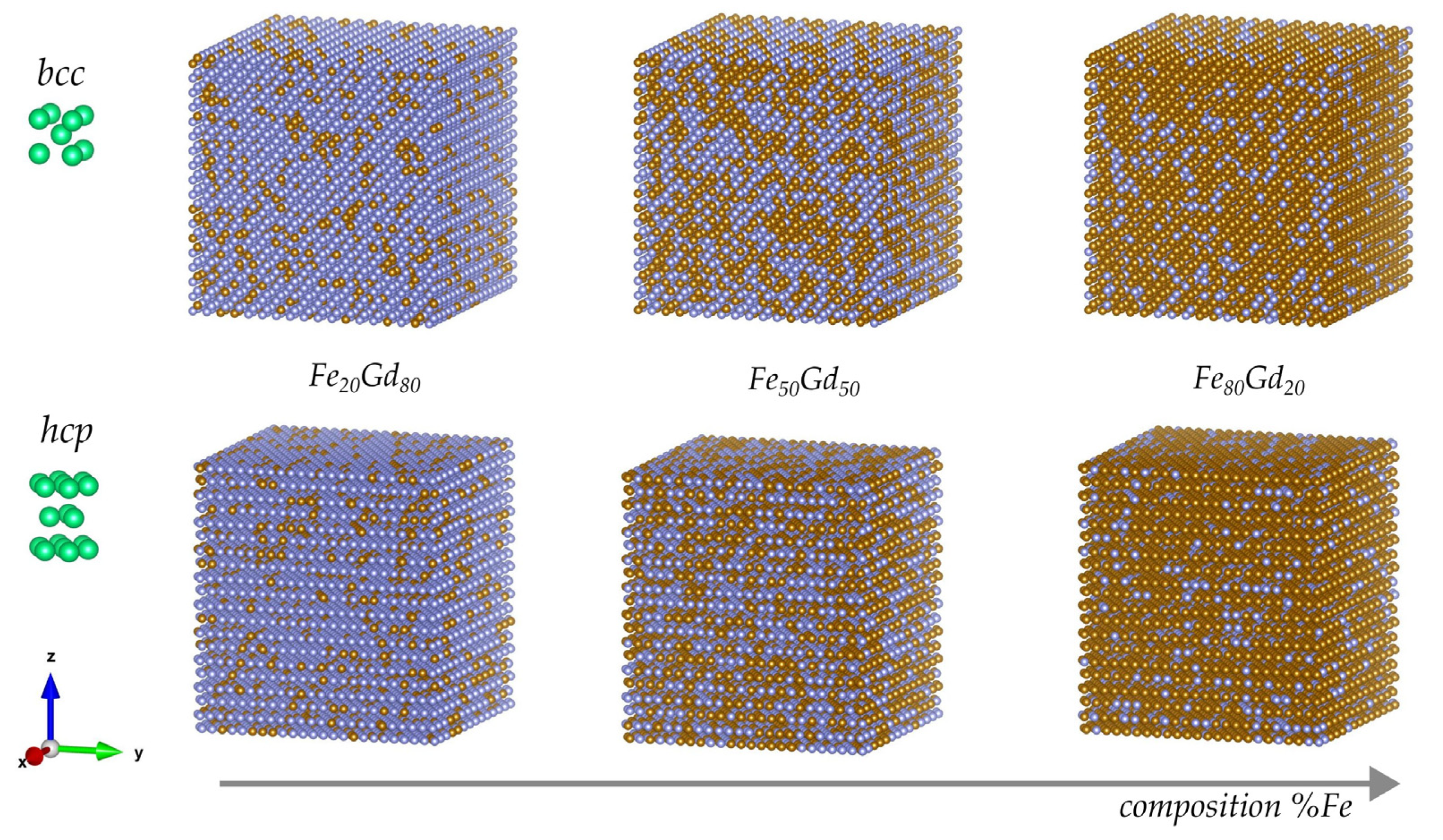
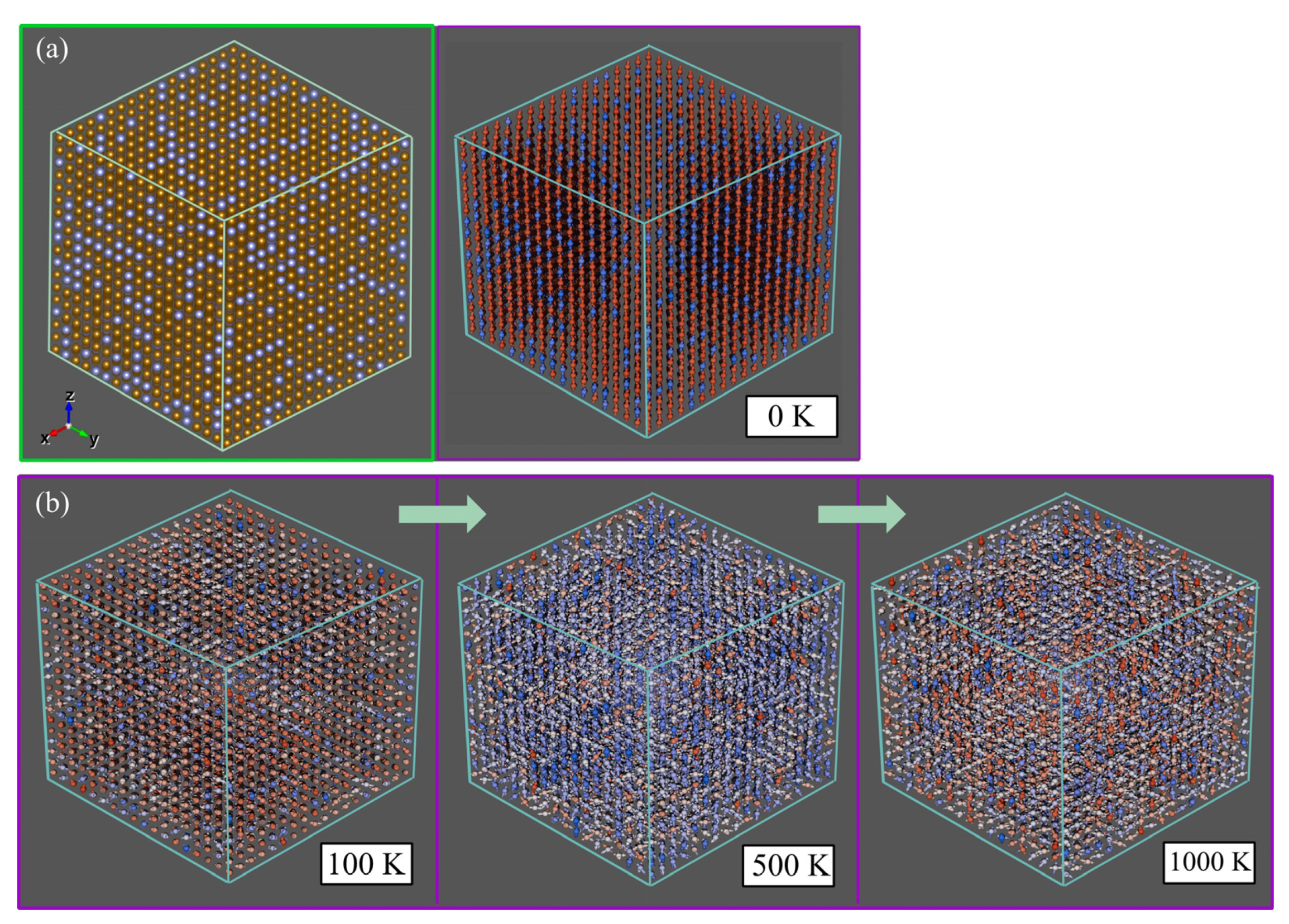
2. Materials and Methods
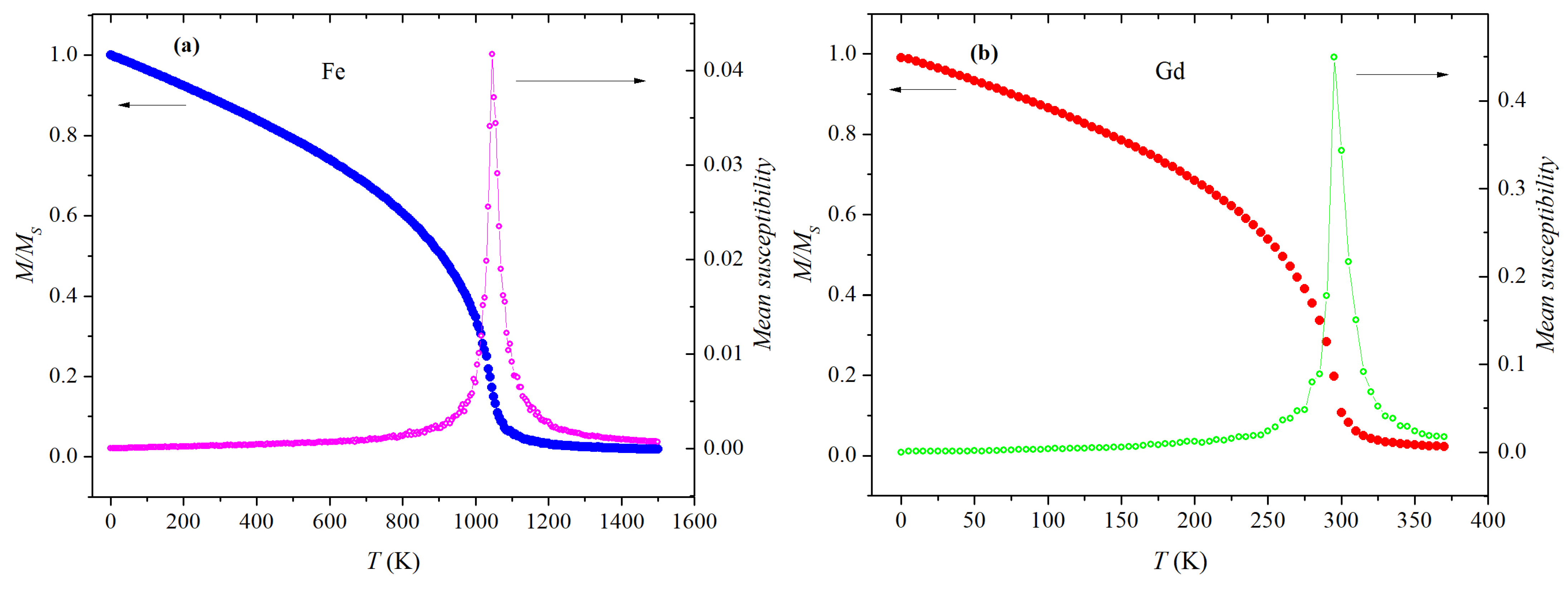
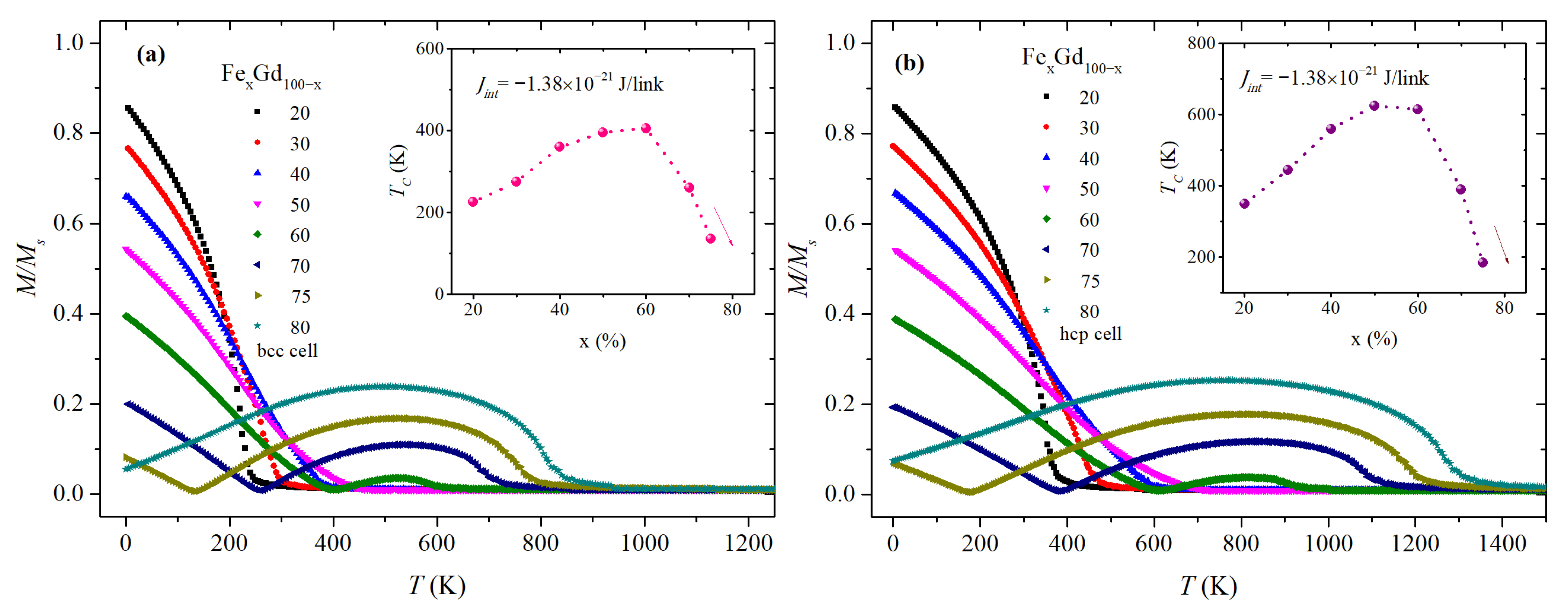
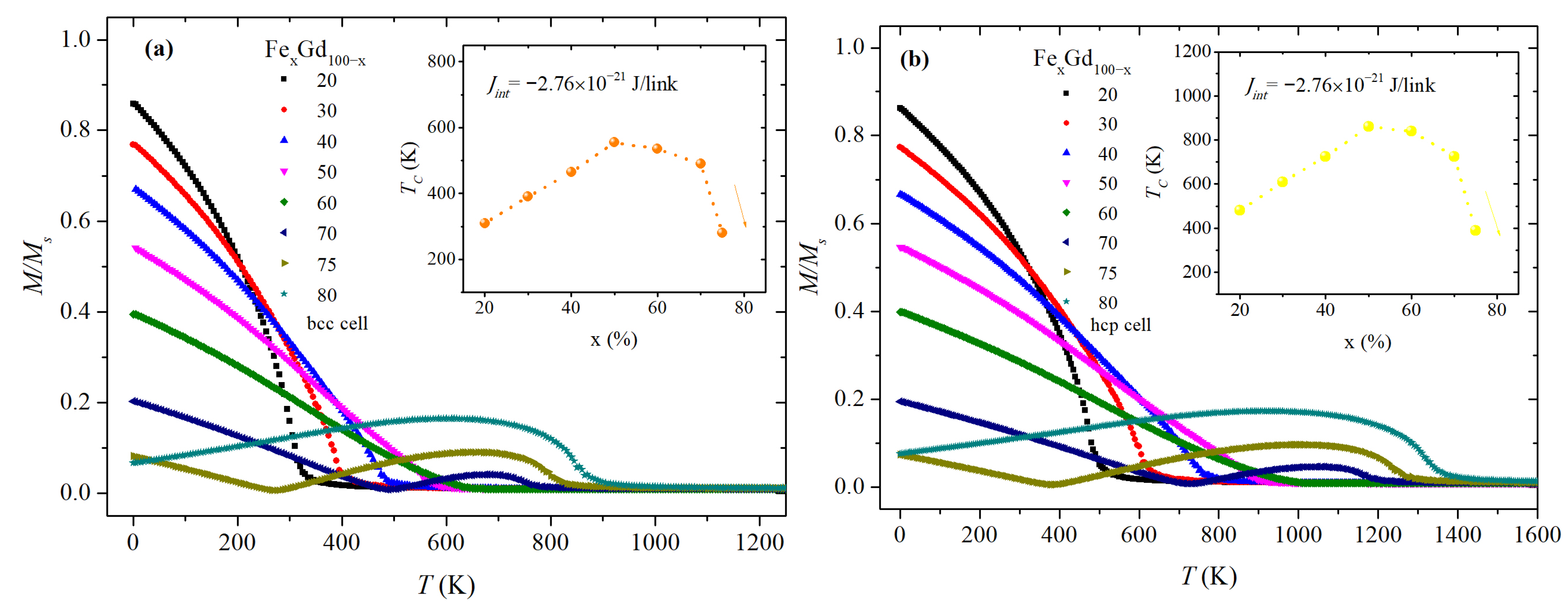
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Nesbitt, E.A.; Wernick, J.H.; Corenzwit, E. Magnetic Moments of Alloys and Compounds of Iron and Cobalt with Rare Earth Metal Additions. J. Appl. Phys. 1959, 30, 365–367. [Google Scholar] [CrossRef]
- Hubbard, W.M.; Adams, E.; Gilfrich, J.V. Magnetic Moments of Alloys of Gadolinium with Some of the Transition Elements. J. Appl. Phys. 1960, 31, S368–S369. [Google Scholar] [CrossRef]
- Vickery, R.C.; Sexton, W.C.; Novy, V.; Kleber, E.V. Magneto Structural Studies on Gadolinium-Iron Alloys. J. Appl. Phys. 1960, 31, S366–S367. [Google Scholar] [CrossRef]
- Nesbitt, E.A.; Williams, H.J.; Wernick, J.H.; Sherwood, R.C. Magnetic Moments of Intermetallic Compounds of Transition and Rare-Earth Elements. J. Appl. Phys. 1962, 33, 1674–1678. [Google Scholar] [CrossRef]
- Campbell, I.A. Indirect Exchange for Rare Earths in Metals. J. Phys. F Met. Phys. 1972, 2, L47–L50. [Google Scholar] [CrossRef]
- Buschow, K.H.J. Intermetallic Compounds of Rare-Earth and 3d Transition Metals. Rep. Prog. Phys. 1977, 40, 1179–1256. [Google Scholar] [CrossRef]
- Kirchmayr, H.R.; Poldy, C.A. Magnetism in Rare Earth—3d Intermetallics. J. Magn. Magn. Mater. 1978, 8, 1–42. [Google Scholar] [CrossRef]
- Baczewski, L.T.; Givord, D.; Alameda, J.M.; Dieny, B.; Nozieres, J.P.; Rebouillat, J.P.; Prejean, J.J. Magnetism in Rare-Earth-Transition Metal Systems. Magnetization Reversal and Ultra-High Susceptibility in Sandwiched Thin Films Based on Rare-Earth and Cobalt Alloys. Acta Phys. Pol. A 1993, 83, 629–641. [Google Scholar] [CrossRef]
- González, J.A.; Andrés, J.P.; López Antón, R. Applied Trends in Magnetic Rare Earth/Transition Metal Alloys and Multilayers. Sensors 2021, 21, 5615. [Google Scholar] [CrossRef]
- Hansen, P.; Clausen, C.; Much, G.; Rosenkranz, M.; Witter, K. Magnetic and Magneto-optical Properties of Rare-earth Transition-metal Alloys Containing Gd, Tb, Fe, Co. J. Appl. Phys. 1989, 66, 756–767. [Google Scholar] [CrossRef]
- Binder, M.; Weber, A.; Mosendz, O.; Woltersdorf, G.; Izquierdo, M.; Neudecker, I.; Dahn, J.R.; Hatchard, T.D.; Thiele, J.-U.; Back, C.H.; et al. Magnetization Dynamics of the Ferrimagnet CoGd near the Compensation of Magnetization and Angular Momentum. Phys. Rev. B 2006, 74, 134404. [Google Scholar] [CrossRef]
- Dong, Q.Y.; Shen, B.G.; Chen, J.; Shen, J.; Wang, F.; Zhang, H.W.; Sun, J.R. Large Magnetic Refrigerant Capacity in Gd71Fe3Al26 and Gd65Fe20Al15 Amorphous Alloys. J. Appl. Phys. 2009, 105, 053908. [Google Scholar] [CrossRef]
- Zheng, Z.; Zhong, X.; Su, K.; Yu, H.; Liu, Z.; Zeng, D. Magnetic Properties and Large Magnetocaloric Effects in Amorphous Gd-Al-Fe Alloys for Magnetic Refrigeration. Sci. China Phys. Mech. Astron. 2011, 54, 1267–1270. [Google Scholar] [CrossRef]
- Jiang, X.; Gao, L.; Sun, J.Z.; Parkin, S.S.P. Temperature Dependence of Current-Induced Magnetization Switching in Spin Valves with a Ferrimagnetic CoGd Free Layer. Phys. Rev. Lett. 2006, 97, 217202. [Google Scholar] [CrossRef] [PubMed]
- Yang, D.Z.; You, B.; Zhang, X.X.; Gao, T.R.; Zhou, S.M.; Du, J. Inverse Giant Magnetoresistance in Fe/Cu/Gd 1 − x Co x Spin-Valves. Phys. Rev. B 2006, 74, 024411. [Google Scholar] [CrossRef]
- Roschewsky, N.; Matsumura, T.; Cheema, S.; Hellman, F.; Kato, T.; Iwata, S.; Salahuddin, S. Spin-Orbit Torques in Ferrimagnetic GdFeCo Alloys. Appl. Phys. Lett. 2016, 109, 112403. [Google Scholar] [CrossRef]
- Szpunar, B.; Kozarzewski, K. The Application of CPA to Calculations of the Mean Magnetic Moment in the Gd1-x-Nix, Gd1-x-Fex, Gd1-x-Cox, and Y1-x-Cox Intermetallic Compounds. Phys. Stat. Sol. B 1977, 82, 205–211. [Google Scholar] [CrossRef]
- Buschow, K.H.J. Crystallization and Magnetic Properties of Amorphous Gd-Fe and Er-Fe Alloys. J. Less Common Met. 1979, 66, 89–97. [Google Scholar] [CrossRef]
- Lee, S.R.; Miller, A.E. Crystallization Behavior of Evaporated Gd-Fe Alloy Films. J. Appl. Phys. 1984, 55, 3465–3470. [Google Scholar] [CrossRef]
- Sajieddine, M.; Bauer, P.H.; Cherifi, K.; Dufour, C.; Marchal, G.; Camley, R.E. Experimental and Theoretical Spin Configurations in Fe/Gd Multilayers. Phys. Rev. B 1994, 49, 8815–8820. [Google Scholar] [CrossRef]
- Ostler, T.A.; Evans, R.F.L.; Chantrell, R.W.; Atxitia, U.; Chubykalo-Fesenko, O.; Radu, I.; Abrudan, R.; Radu, F.; Tsukamoto, A.; Itoh, A.; et al. Crystallographically Amorphous Ferrimagnetic Alloys: Comparing a Localized Atomistic Spin Model with Experiments. Phys. Rev. B 2011, 84, 024407. [Google Scholar] [CrossRef]
- Tang, K.-Q.; Zhong, K.-H.; Cheng, Y.-M.; Huang, Z.-G. Effect of Gd Doping on the Magnetism and Work Function of Fe1—x Gdx/Fe (001). Chin. Phys. B 2014, 23, 056301. [Google Scholar] [CrossRef]
- Chimata, R.; Isaeva, L.; Kádas, K.; Bergman, A.; Sanyal, B.; Mentink, J.H.; Katsnelson, M.I.; Rasing, T.; Kirilyuk, A.; Kimel, A.; et al. All-Thermal Switching of Amorphous Gd-Fe Alloys: Analysis of Structural Properties and Magnetization Dynamics. Phys. Rev. B 2015, 92, 094411. [Google Scholar] [CrossRef]
- Anderson, N.R.; Camley, R.E. Temperature-Dependent Magnetization in Bimagnetic Nanoparticles with Antiferromagnetic Interfacial Exchange. Phys. Rev. B 2016, 94, 134432. [Google Scholar] [CrossRef]
- Kita, E.; Hata, Y.; Yano, K.; Suzuki, H.; Kido, G. Experimental Determination of Inter-Sublattice Exchange Constants in Amorphous FeGd Alloys. J. Appl. Phys. 2004, 95, 6834–6836. [Google Scholar] [CrossRef]
- Radu, I.; Vahaplar, K.; Stamm, C.; Kachel, T.; Pontius, N.; Dürr, H.A.; Ostler, T.A.; Barker, J.; Evans, R.F.L.; Chantrell, R.W.; et al. Transient Ferromagnetic-like State Mediating Ultrafast Reversal of Antiferromagnetically Coupled Spins. Nature 2011, 472, 205–208. [Google Scholar] [CrossRef] [PubMed]
- Imamura, N.; Ota, C. Experimental Study on Magneto-Optical Disk Exerciser with the Laser Diode and Amorphous Magnetic Thin Films. Jpn. J. Appl. Phys. 1980, 19, L731–L734. [Google Scholar] [CrossRef]
- Mangin, S.; Marchal, G.; Bellouard, C.; Wernsdorfer, W.; Barbara, B. Magnetic Behavior and Resistivity of the Domain-Wall Junction GdFe(1000 Å)/TbFe/GdFe(500 Å). Phys. Rev. B 1998, 58, 2748–2757. [Google Scholar] [CrossRef]
- Aoshima, K.; Machida, K.; Kato, D.; Mishina, T.; Wada, K.; Cai, Y.; Kinjo, H.; Kuga, K.; Kikuchi, H.; Ishibashi, T.; et al. A Magneto-Optical Spatial Light Modulator Driven by Spin Transfer Switching for 3D Holography Applications. J. Disp. Technol. 2015, 11, 129–135. [Google Scholar] [CrossRef]
- Luborsky, F.E. Amorphous Metallic Alloys; Butterworths monographs materials; Butterworths: London, UK, 1983; ISBN 978-0-408-11030-3. [Google Scholar]
- Evans, R.F.L.; Fan, W.J.; Chureemart, P.; Ostler, T.A.; Ellis, M.O.A.; Chantrell, R.W. Atomistic Spin Model Simulations of Magnetic Nanomaterials. J. Phys. Condens. Matter 2014, 26, 103202. [Google Scholar] [CrossRef]
- Buschow, K.H.J.; Brouha, M.; Biesterbos, J.W.M.; Dirks, A.G. Crystalline and Amorphous Rare-Earth Transition Metal Alloys. Phys. B+C 1977, 91, 261–270. [Google Scholar] [CrossRef]
- Hinzke, D.; Nowak, U. Monte Carlo Simulation of Magnetization Switching in a Heisenberg Model for Small Ferromagnetic Particles. Comput. Phys. Commun. 1999, 121–122, 334–337. [Google Scholar] [CrossRef]
- Ramos-Guivar, J.A.; Tamanaha-Vegas, C.A.; Litterst, F.J.; Passamani, E.C. Magnetic Simulations of Core–Shell Ferromagnetic Bi-Magnetic Nanoparticles: The Influence of Antiferromagnetic Interfacial Exchange. Nanomaterials 2021, 11, 1381. [Google Scholar] [CrossRef]
- Polcarová, M.; Kadečková, S.; Bradler, J.; Godwod, K.; Bak-misiuk, J. Lattice parameters of FeSi alloy single crystals. Phys. Stat. Sol. 1988, 106, 17–23. [Google Scholar] [CrossRef]
- Eyring, L.; Gschneidner, K.A. Handbook on the Physics and Chemistry of Rare Earths; Elsevier: Amsterdam, The Netherlands, 2000; ISBN 978-0-444-50346-6. [Google Scholar]
- Evans, R.F.L.; Atxitia, U.; Chantrell, R.W. Quantitative Simulation of Temperature-Dependent Magnetization Dynamics and Equilibrium Properties of Elemental Ferromagnets. Phys. Rev. B 2015, 91, 144425. [Google Scholar] [CrossRef]
- Yano, K. Molecular Field Analysis for Melt-Spun Amorphous Fe100−xGdx Alloys (18 ≦ X ≦ 60). J. Magn. Magn. Mater. 2000, 208, 207–216. [Google Scholar] [CrossRef]
- Persistence of Vision Pty. Ltd. Version 3.6; [Computer Software] 2004. Available online: https://www.povray.org (accessed on 10 October 2022).
- Yano, K.; Tokumitsu, K.; Kita, E.; Ino, H.; Tasaki, A. Crystallization Behavior and Curie Temperature for Melt-Spun Amorphous Fe100- X GdX (18 ≤ X ≤ 70) Alloys. Jpn. J. Appl. Phys. 1991, 30, L482–L485. [Google Scholar] [CrossRef]
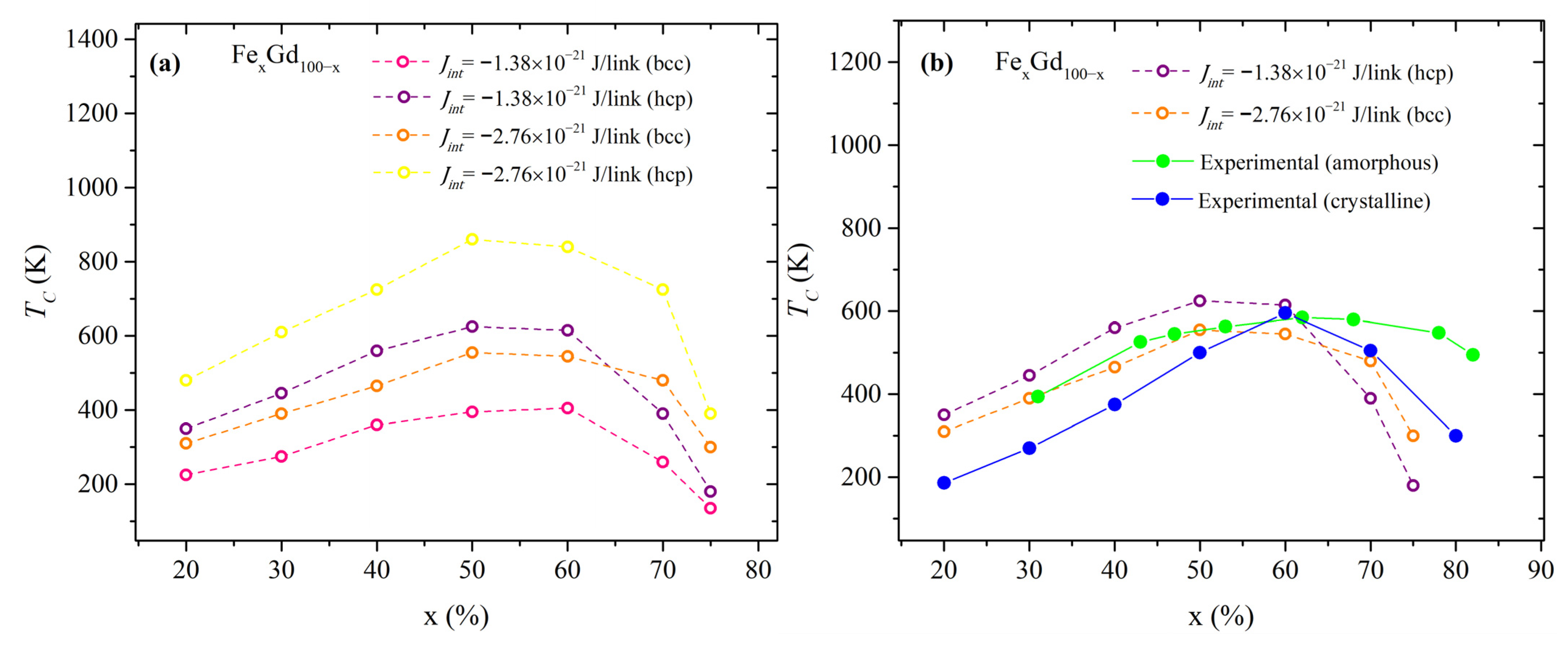
| x (%) | Tc (K) | |||||
|---|---|---|---|---|---|---|
| Exp. Amorphous | Exp. Crystal | |||||
| 20 | 225(5) | 350(5) | 310(5) | 480(5) | — | ~186 |
| 30 | 275(5) | 445(5) | 390(5) | 610(5) | ~382 | ~270 |
| 40 | 360(5) | 560(5) | 465(5) | 725(5) | ~495 | ~375 |
| 50 | 395(5) | 625(5) | 555(5) | 860(5) | ~553 | ~500 |
| 60 | 405(5) | 615(5) | 545(5) | 840(5) | ~575 | ~596 |
| 70 | 260(5) | 390(5) | 480(5) | 725(5) | ~570 | ~505 |
| 75 | 135(5) | 180(5) | 305(5) | 390(5) | ~557 | ~392 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pastukh, O.; Kuźma, D.; Pastukh, S. Simulations of Temperature-Dependent Magnetization in FexGd100−x (20 ≤ x ≤ 80) Alloys. Magnetism 2023, 3, 34-44. https://doi.org/10.3390/magnetism3010004
Pastukh O, Kuźma D, Pastukh S. Simulations of Temperature-Dependent Magnetization in FexGd100−x (20 ≤ x ≤ 80) Alloys. Magnetism. 2023; 3(1):34-44. https://doi.org/10.3390/magnetism3010004
Chicago/Turabian StylePastukh, Oleksandr, Dominika Kuźma, and Svitlana Pastukh. 2023. "Simulations of Temperature-Dependent Magnetization in FexGd100−x (20 ≤ x ≤ 80) Alloys" Magnetism 3, no. 1: 34-44. https://doi.org/10.3390/magnetism3010004
APA StylePastukh, O., Kuźma, D., & Pastukh, S. (2023). Simulations of Temperature-Dependent Magnetization in FexGd100−x (20 ≤ x ≤ 80) Alloys. Magnetism, 3(1), 34-44. https://doi.org/10.3390/magnetism3010004








