Biomechanical Upper Limb Model for Postural Tremor Absorber Design
Abstract
:1. Introduction
2. Methods
2.1. Biodynamic Hand Model
2.2. Patient Tremor Measurements
2.2.1. Acceleration and Angular Velocity
2.2.2. Electromyography
2.3. Design of TMD
2.4. Optimization of TMD
- (1)
- The FFT of the ECR muscle signal is used as an input for the Laplace transform of the equation of motion (8);
- (2)
- For random initial parameters , the responses of the system with and without TMD(s) are obtained;
- (3)
- The inverse fast Fourier transforms (IFFT) of the responses in the frequency domain are used to obtain the corresponding time signals;
- (4)
- The PSD of the time signals, with and without TMD(s), are computed;
- (5)
- The reduction in the amplitude of the peak for the global system due to is then calculated;
- (6)
- The procedure is repeated to obtain the maximum possible reduction in the amplitude while the parameters of the TMD converge.
3. Results
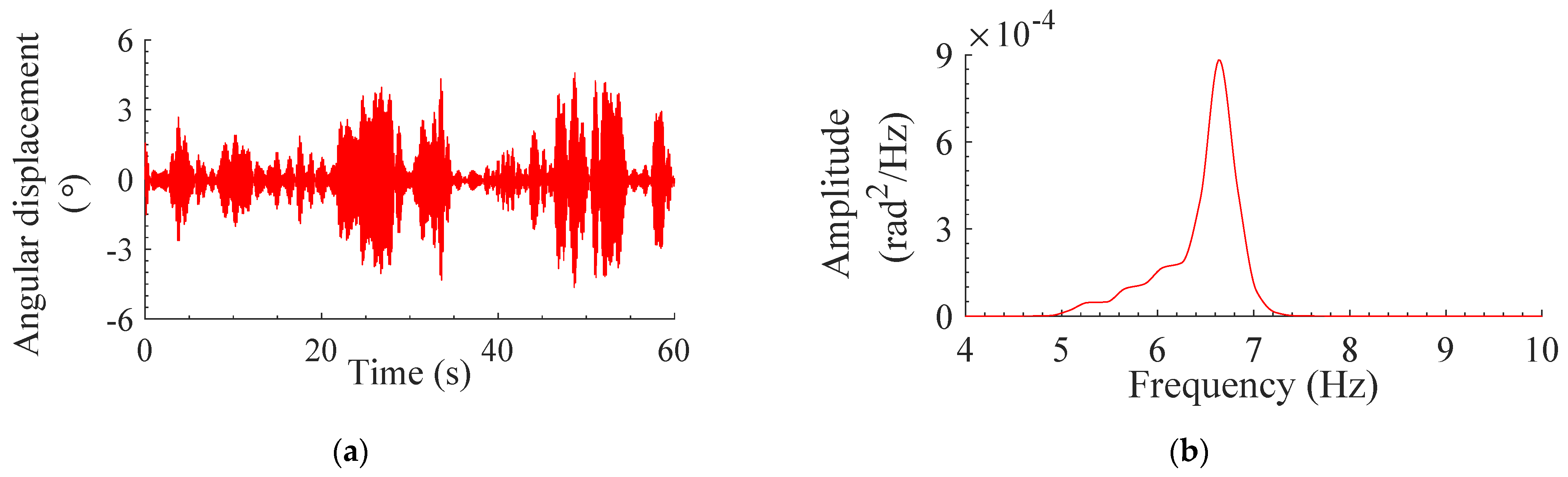
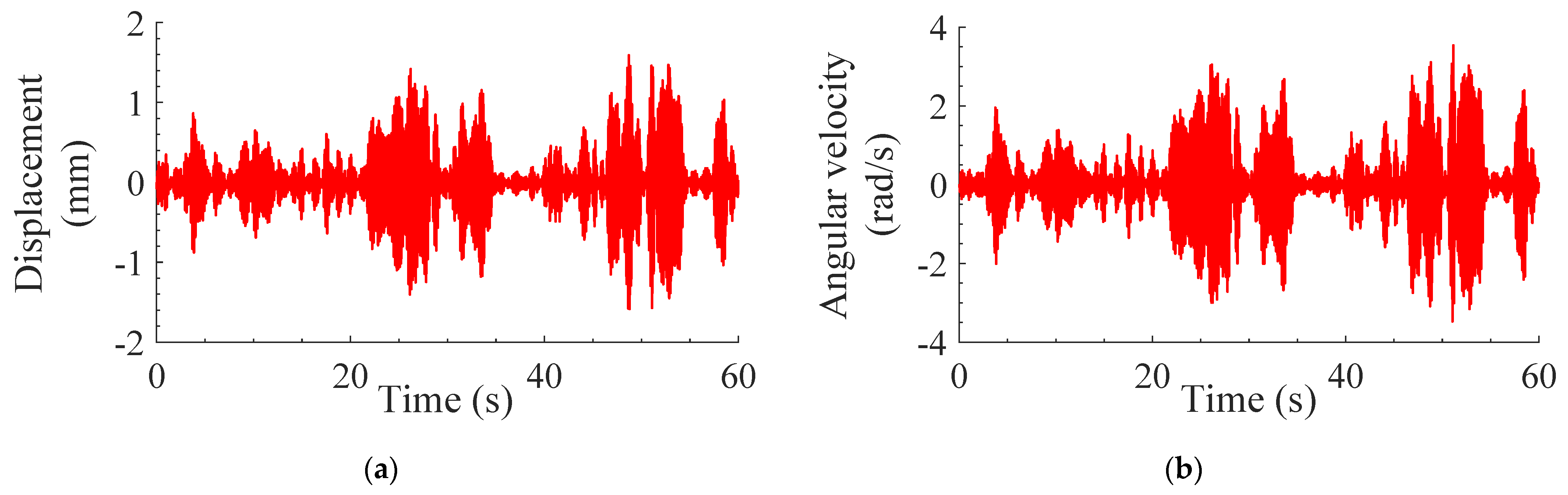
3.1. Measured Signals
3.2. Response of Modeled System
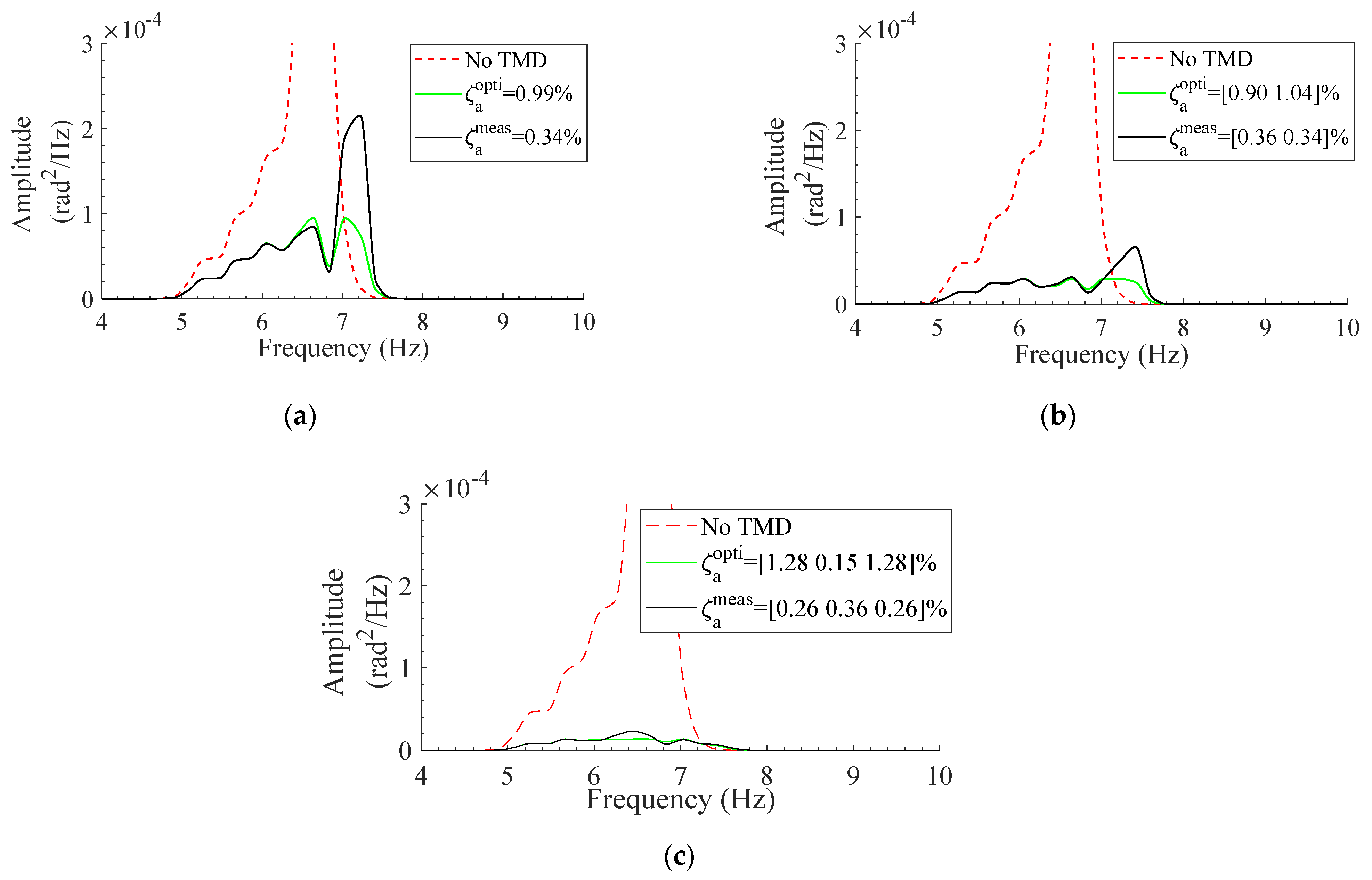
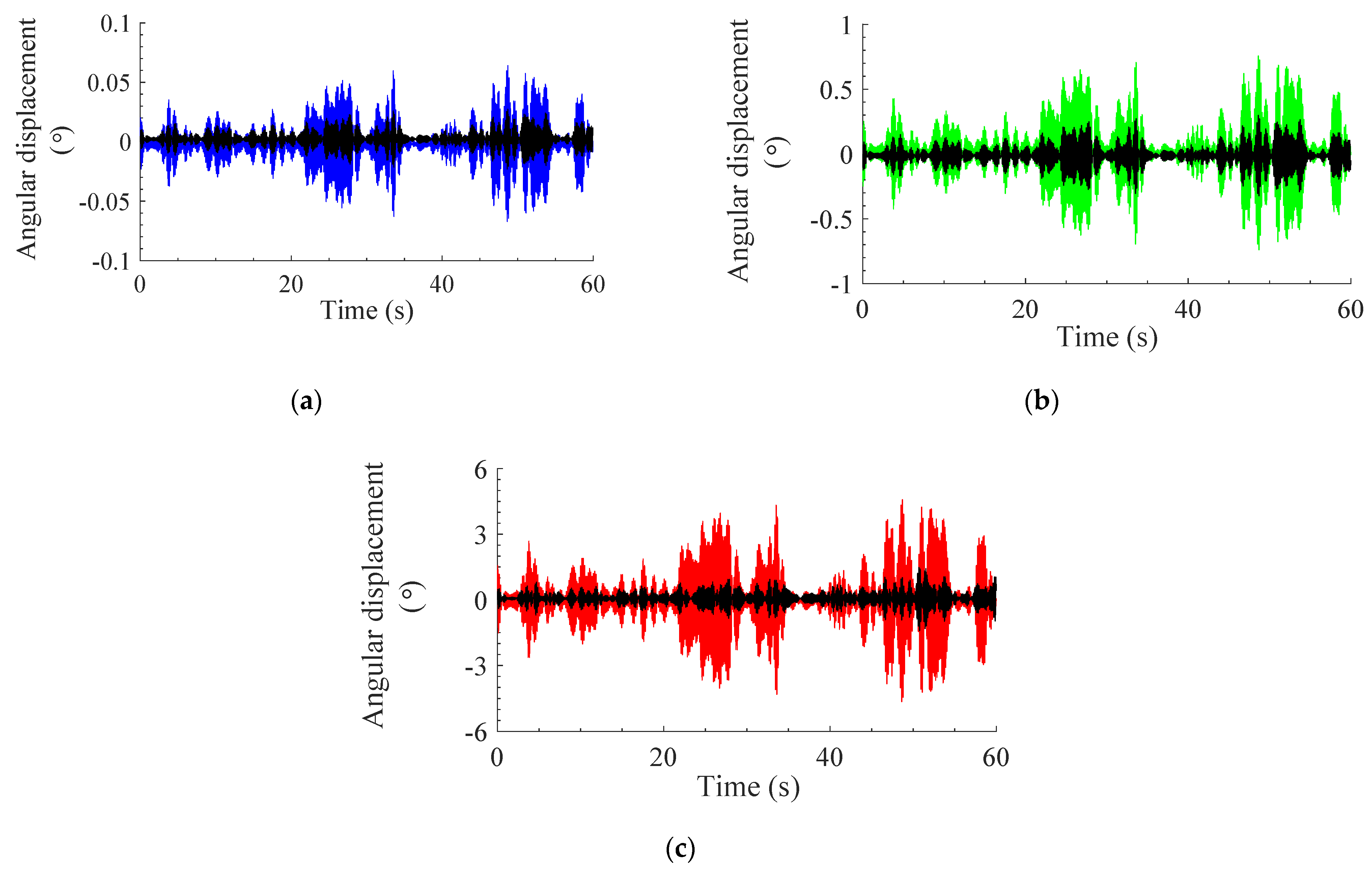
3.3. Effect of Optimized TMD
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Blache, Y.; Begon, M. Influence of shoulder kinematic estimate on joint and muscle mechanics predicted by musculoskeletal model. IEEE Trans. Biomed. Eng. 2017, 65, 715–722. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Blache, Y.; Michaud, B.; Rogowski, I.; Monteil, K.; Begon, M. Sensitivity of shoulder musculoskeletal model predictions to muscle–tendon properties. IEEE Trans. Biomed. Eng. 2018, 66, 1309–1317. [Google Scholar] [CrossRef] [PubMed]
- Bolsterlee, B.; Veeger, D.H.; Chadwick, E.K. Clinical applications of musculoskeletal modelling for the shoulder and upper limb. Med. Biol. Eng. Comput. 2013, 51, 953–963. [Google Scholar] [CrossRef] [PubMed]
- Bolsterlee, B.; Veeger, H.E.J.; van der Helm, F.C. Modelling clavicular and scapular kinematics: From measurement to simulation. Med. Biol. Eng. Comput. 2014, 52, 283–291. [Google Scholar] [CrossRef] [PubMed]
- Louis, E.D.; Ferreira, J.J. How common is the most common adult movement disorder? Update on the worldwide prevalence of essential tremor. Mov. Disord. 2010, 25, 534–541. [Google Scholar] [CrossRef]
- Park, K.; Chang, P.H.; Kang, S.H. In vivo estimation of human forearm and wrist dynamic properties. IEEE Trans. Neural. Syst. Rehabil. Eng. 2017, 25, 436–446. [Google Scholar] [CrossRef]
- Chan, P.Y.; Ripin, Z.M.; Abdul Halim, S.; Tharakan, J.; Muzaimi, M.; Ng, K.S.; Kamarudin, M.I.; Eow, G.B.; Hor, J.Y.; Tan, K.; et al. An in–laboratory validity and reliability tested system for quantifying hand–arm tremor in motions. IEEE Trans. Neural. Syst. Rehabil. Eng. 2018, 26, 460–467. [Google Scholar] [CrossRef] [PubMed]
- Abbasi, M.; Afsharfard, A.; Arasteh, R.; Safaie, J. Design of a noninvasive and smart hand tremor attenuation system with active control: A simulation Study. Med. Biol. Eng. Comput. 2018, 56, 1315–1324. [Google Scholar] [CrossRef]
- Sagi-Dolev, A.M.; Prutchi, D.; Nathan, R.H. Three-dimensional current density distribution under surface stimulation electrodes. Med. Biol. Eng. Comput. 1995, 33, 403–408. [Google Scholar] [CrossRef]
- Triolo, R.J.; Liu, M.Q.; Kobetic, R.; Uhlir, J.P. Selectivity of intramuscular stimulating electrodes in the lower limbs. J. Rehabil. Res. Develop. 2001, 38, 533–544. [Google Scholar]
- Hashemi, S.M.; Golnaraghi, M.F.; Patla, A.E. Tuned vibration absorber for suppression of rest tremor in Parkinson’s disease. Med. Biol. Eng. Comput. 2004, 43, 61–70. [Google Scholar] [CrossRef]
- Buki, E.; Katz, R.; Zacksenhouse, M.; Schlesinger, I. Vib-bracelet: A passive absorber for attenuating forearm tremor. Med. Biol. Eng. Comput. 2018, 56, 923–930. [Google Scholar] [CrossRef]
- Bucolo, M.; Buscarino, A.; Fortuna, L.; Frasca, M. Multiple hysteresis jump resonance in a class of forced nonlinear circuits and systems. Int. J. Bifurc. Chaos 2020, 30, 2050258. [Google Scholar] [CrossRef]
- Gebai, S.; Savadkoohi, A.T.; Lamarque, C.H. Involuntary Tremor Control Using Passive Non-Smooth Absorber. In Proceedings of the 15ème Colloque National en Calcul des Structures, Giens, France, 16–20 May 2022; pp. 1–7. [Google Scholar]
- Gebai, S.; Savadkoohi, A.T.; Lamarque, C.H. Passive Nonlinear Energy Sink for Pathological Tremor Reduction. In Proceedings of the the 10th European Nonlinear Dynamics Conference, Lyon, France, 17–22 July 2022. [Google Scholar]
- Jackson, K.M.; Joseph, J.T.; Wyard, S.J. A mathematical model of arm swing during human locomotion. J. Biomech. 1978, 11, 277–289. [Google Scholar] [CrossRef]
- Hirashima, M. Induced Acceleration Analysis of Three-Dimensional Multi-Joint Movements and Its Application to Sports Movements. In Theoretical Biomechanics; Klika, V., Ed.; IntechOpen: London, UK, 2011. [Google Scholar]
- Harless, E. The static moments of human limbs. Treatsises Math.-Phys Cl. R. Acad. Sci. Bavaria 1860, 8, 69–96. [Google Scholar]
- Contini, R. Body segment parameters, Part II. Artif. Limbs 1972, 16, 1–19. [Google Scholar]
- Clauser, C.E.; Mc Conville, J.T.; Young, J.W. Weight, Volume, and Center of Mass of Segments of the Human Body; Wright-Patterson Air Force Base; (NTIS NO. AD-710 622); Yellow Springs, OH, USA; Antioch Coll: Yellow Springs, OH, USA, 1969. [Google Scholar]
- Velandia, C.; Celedón, H.; Tibaduiza, D.A.; Torres-Pinzón, C.; Vitola, J. Design and control of an exoskeleton in rehabilitation tasks for lower limb. In Proceedings of the 2016 XXI Symposium on Signal Processing, Images and Artificial Vision (STSIVA), Bucaramanga, CO, USA, 31 August–2 September 2016; IEEE: Piscataway, NJ, USA, 2016; pp. 1–6. [Google Scholar]
- Pandis, P. Musculoskeletal Biomechanics of the Shoulder in Functional Activities. Ph.D. Thesis, Imperial College London, London, UK, 2013. [Google Scholar]
- Goddard, R.; Dowson, D.; Longfield, M.D.; Wright, V. The measurement of stiffness in human joints. Rheologica. Acta 1969, 8, 229–234. [Google Scholar] [CrossRef]
- Zernicke, R.F.; Schneider, K.; Buford, J.A. Intersegmental dynamics during gait: Implications for control. Adv. Psychol. 1991, 78, 187–202. [Google Scholar]
- Carmichael, M.G.; Liu, D. Estimating physical assistance need using a musculoskeletal model. IEEE Trans. Biomed. Eng. 2013, 60, 1912–1919. [Google Scholar] [CrossRef]
- Babiarz, A.; Bieda, R.; Jaskot, K.; Klamka, J. The dynamics of the human arm with an observer for the capture of body motion parameters. Bull. Pol. Acad Sci.-Tech. 2013, 61, 955–971. [Google Scholar] [CrossRef]
- Clancy, E.A.; Liu, L.; Liu, P.; Moyer, D.V.Z. Identification of constant-posture EMG–torque relationship about the elbow using nonlinear dynamic models. IEEE Trans. Biomed. Eng. 2012, 59, 205–212. [Google Scholar] [CrossRef]
- Gebai, S.; Cumunel, G.; Hammoud, M.; Foret, G.; Apartis, E.; Flamand-Roze, E.; Hainque, E. S Analysis of Pathological Tremor Behavior: Application to Design a Passive Tremor Attenuator. In Proceedings of the 12th International Workshop on Structural Health Monitoring, Stanford, CA, USA, 10–12 September 2019; pp. 619–626. [Google Scholar]
- Chadwick, E.K.; Blana, D.; van den Bogert, A.J.; Kirsch, R.F. A real-time, 3-D musculoskeletal model for dynamic simulation of arm movements. IEEE Trans. Biomed. Eng. 2008, 56, 941–948. [Google Scholar] [CrossRef] [Green Version]
- Mohammed, G.A.; Hou, M. Optimization of active muscle force–length models using least squares curve fitting. IEEE Trans. Biomed. Eng. 2016, 63, 630–635. [Google Scholar] [CrossRef]
- Wu, B. A correction of the half-power bandwidth method for estimating damping. Arch. Appl. Mech. 2014, 85, 315–320. [Google Scholar] [CrossRef]
- Atzori, B. Dunkerley’s formula for finding the lowest frequency of vibration of elastic systems. J. Sound Vib. 1974, 36, 563–564. [Google Scholar] [CrossRef]
- Inman, D.J. Engineering Vibrations; Virginia Tech: Blacksburg, VA, USA; Prentice Hall: Upper Saddle River, NJ, USA, 2008. [Google Scholar]
- Morrison, S.; Kerr, G.; Silburn, P. Bilateral tremor relations in Parkinson’s disease: Effects of mechanical coupling and medication. Parkinsonism Rel. Disord. 2008, 14, 298–308. [Google Scholar] [CrossRef]
- Argoul, P.; Le, T.P. Instantaneous indicators of structural behaviour based on the continuous Cauchy wavelet analysis. Mech. Syst. Signal Proses. 2003, 17, 243–250. [Google Scholar] [CrossRef]
- Gebai, S.; Cumunel, G.; Hammoud, M.; Foret, G.; Roze, E.; Hainque, E. Upper Limb Involuntary Tremor Reduction Using Cantilever Beam TMDs. J. Vib. Control. 2022. [CrossRef]
- Timmer, J.; Häußler, S.; Lauk, M.; Lücking, C.H. Pathological tremors: Deterministic chaos or nonlinear stochastic oscillators? Chaos Interdiscip. J. Nonlinear Sci. 2000, 10, 278–288. [Google Scholar]
- Gao, J.B. Analysis of amplitude and frequency variations of essential and Parkinsonian tremors. Med. Biol. Eng. Comput. 2004, 42, 345–349. [Google Scholar] [CrossRef]
- Huang, T.Y.; Pan, L.L.H.; Yang, W.W.; Huang, L.Y.; Sun, P.C.; Chen, C.S. Biomechanical Evaluation of Three-Dimensional Printed Dynamic Hand Device for Patients with Chronic Stroke. IEEE Trans. Neural Syst. Rehabil. Eng. 2019, 27, 1246–1252. [Google Scholar] [CrossRef] [PubMed]


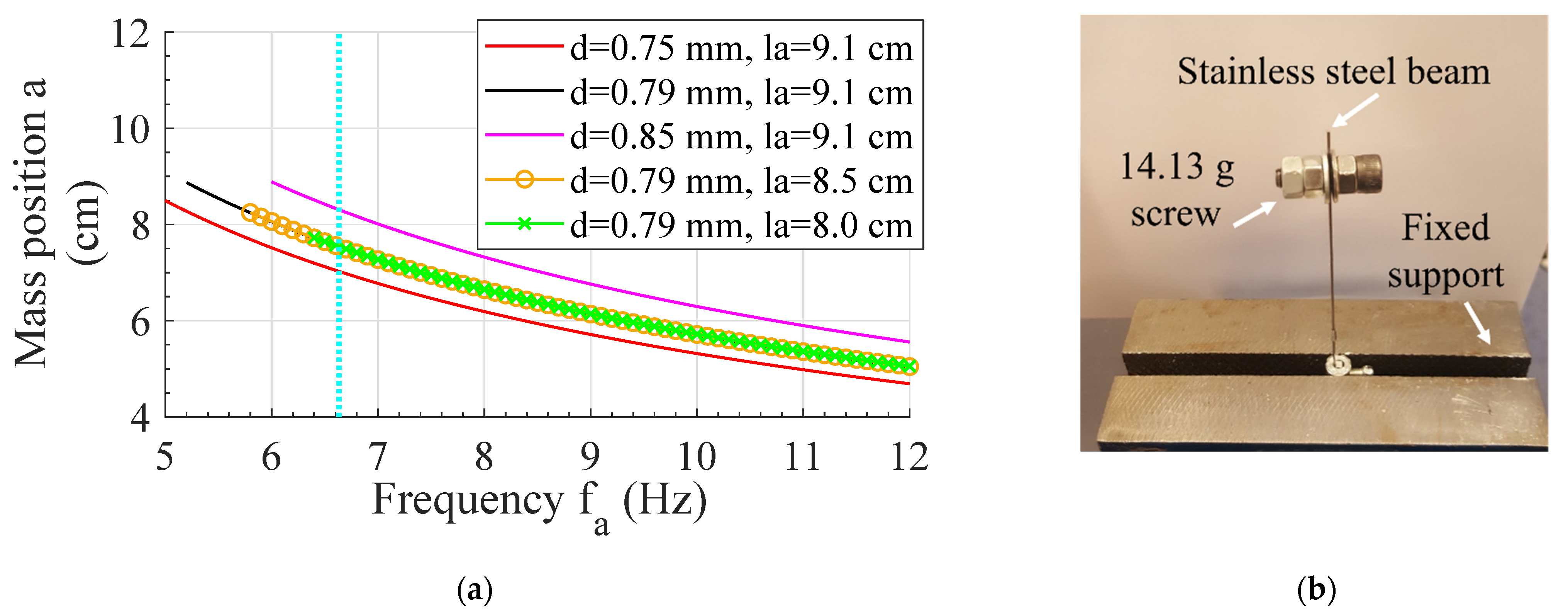
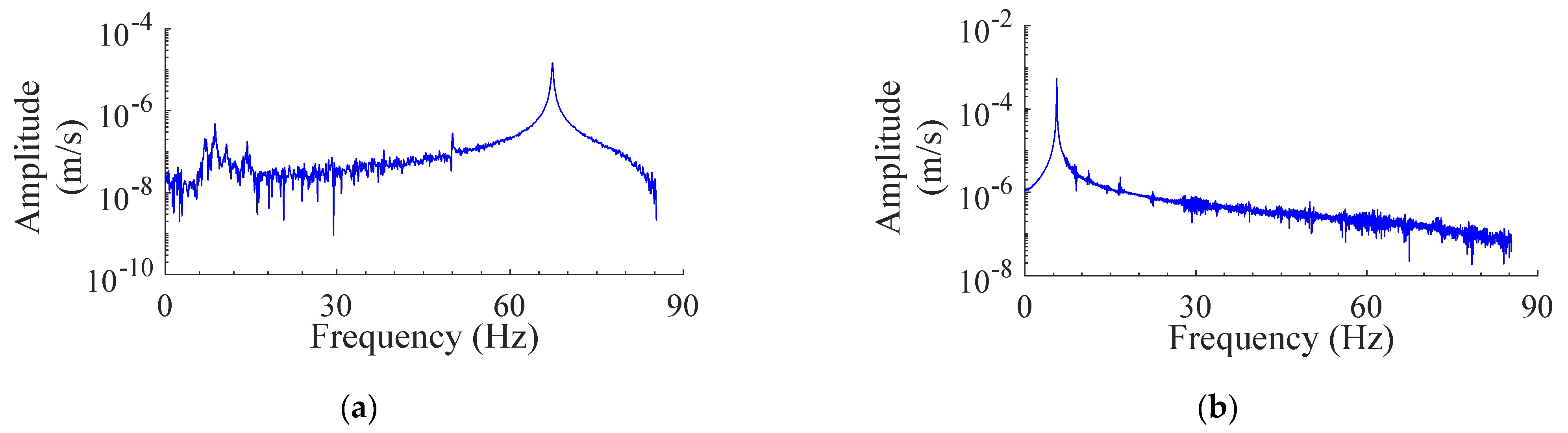


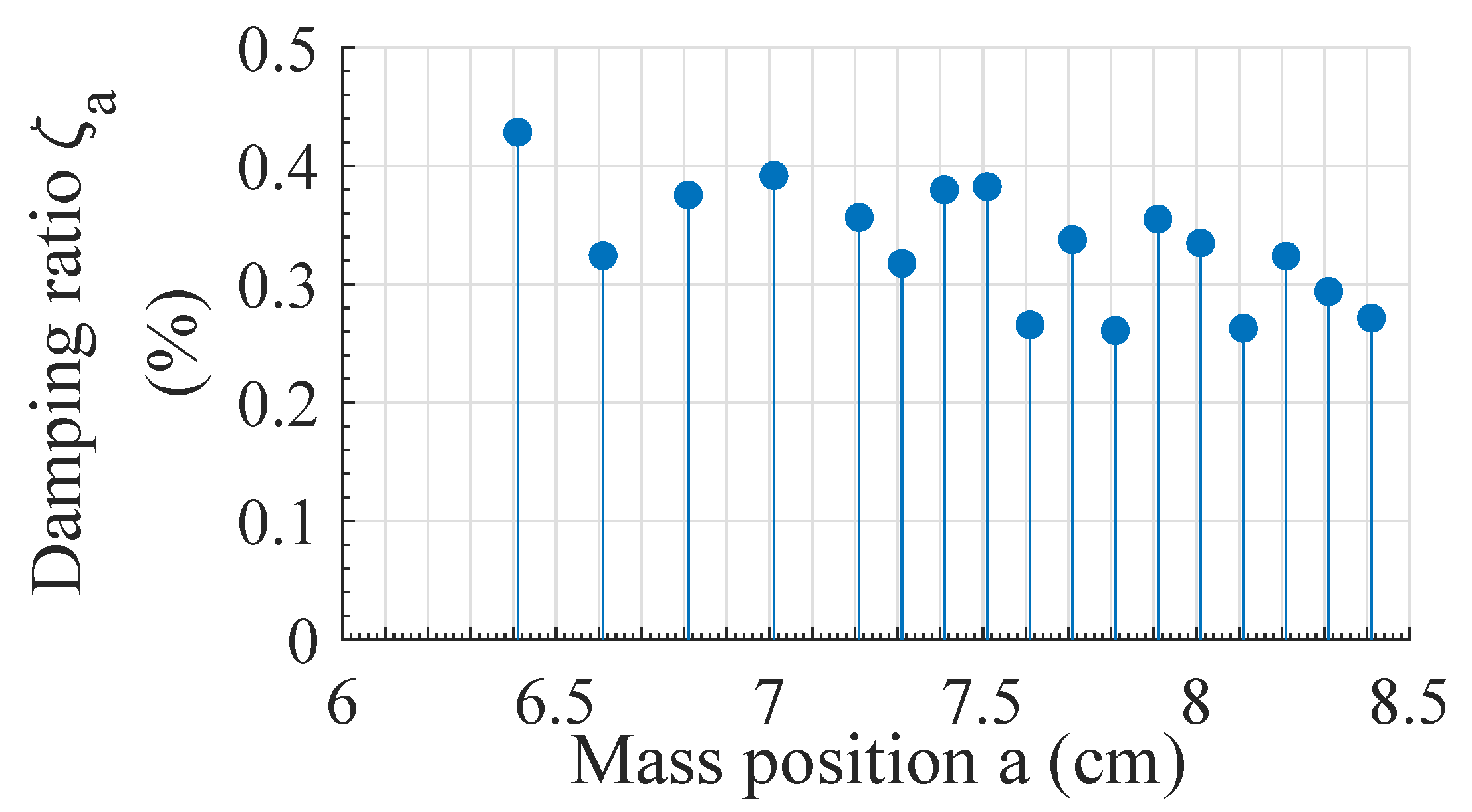

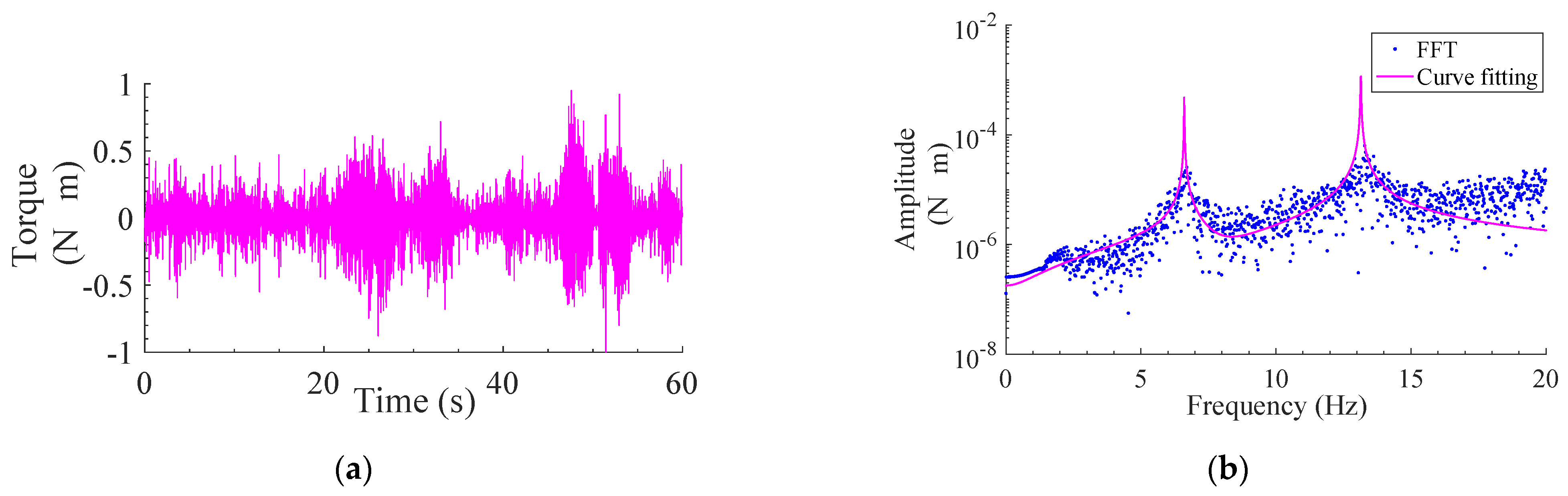

| Length (cm) | Centroid (m) | Mass (kg) | ||||
|---|---|---|---|---|---|---|
| Upper arm | 36.4 | 2.07 | ||||
| Forearm | 29.9 | 1.16 | ||||
| Hand | 20.3 | 0.54 | ||||
| Three TMD System | TMD#1 | TMD#2 | TMD#3 |
|---|---|---|---|
| (cm) | 7.9 | 7.7 | 7.6 |
| (Hz) | 6.44 | 6.71 | 6.85 |
| (%) | 1.27 | 0.15 | 1.28 |
| (%) | 0.33 | 0.38 | 0.37 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gebai, S.; Cumunel, G.; Hammoud, M.; Foret, G.; Roze, E.; Hainque, E. Biomechanical Upper Limb Model for Postural Tremor Absorber Design. Dynamics 2022, 2, 234-251. https://doi.org/10.3390/dynamics2030012
Gebai S, Cumunel G, Hammoud M, Foret G, Roze E, Hainque E. Biomechanical Upper Limb Model for Postural Tremor Absorber Design. Dynamics. 2022; 2(3):234-251. https://doi.org/10.3390/dynamics2030012
Chicago/Turabian StyleGebai, Sarah, Gwendal Cumunel, Mohammad Hammoud, Gilles Foret, Emmanuel Roze, and Elodie Hainque. 2022. "Biomechanical Upper Limb Model for Postural Tremor Absorber Design" Dynamics 2, no. 3: 234-251. https://doi.org/10.3390/dynamics2030012
APA StyleGebai, S., Cumunel, G., Hammoud, M., Foret, G., Roze, E., & Hainque, E. (2022). Biomechanical Upper Limb Model for Postural Tremor Absorber Design. Dynamics, 2(3), 234-251. https://doi.org/10.3390/dynamics2030012






