Robust Porcine GFR Measurement with Radiotracers and Only Late Blood Samples
Abstract
:1. Introduction
2. Material and Methods
2.1. Animals
2.2. Reference GFR: Plasma Clearance Based on Full-Curve Data (Clref)
2.3. One-Pool Approximation Cl1 Based on Late-Sample Data
2.4. Porcine GFR Correction Formula
3. Results
4. Discussion
4.1. Choice of Tracer
4.2. Previous Studies
4.2.1. Frennby Studies
4.2.2. Palnæs Hansen Study
4.2.3. Luis-Lima Study
4.2.4. Van Gelder Study
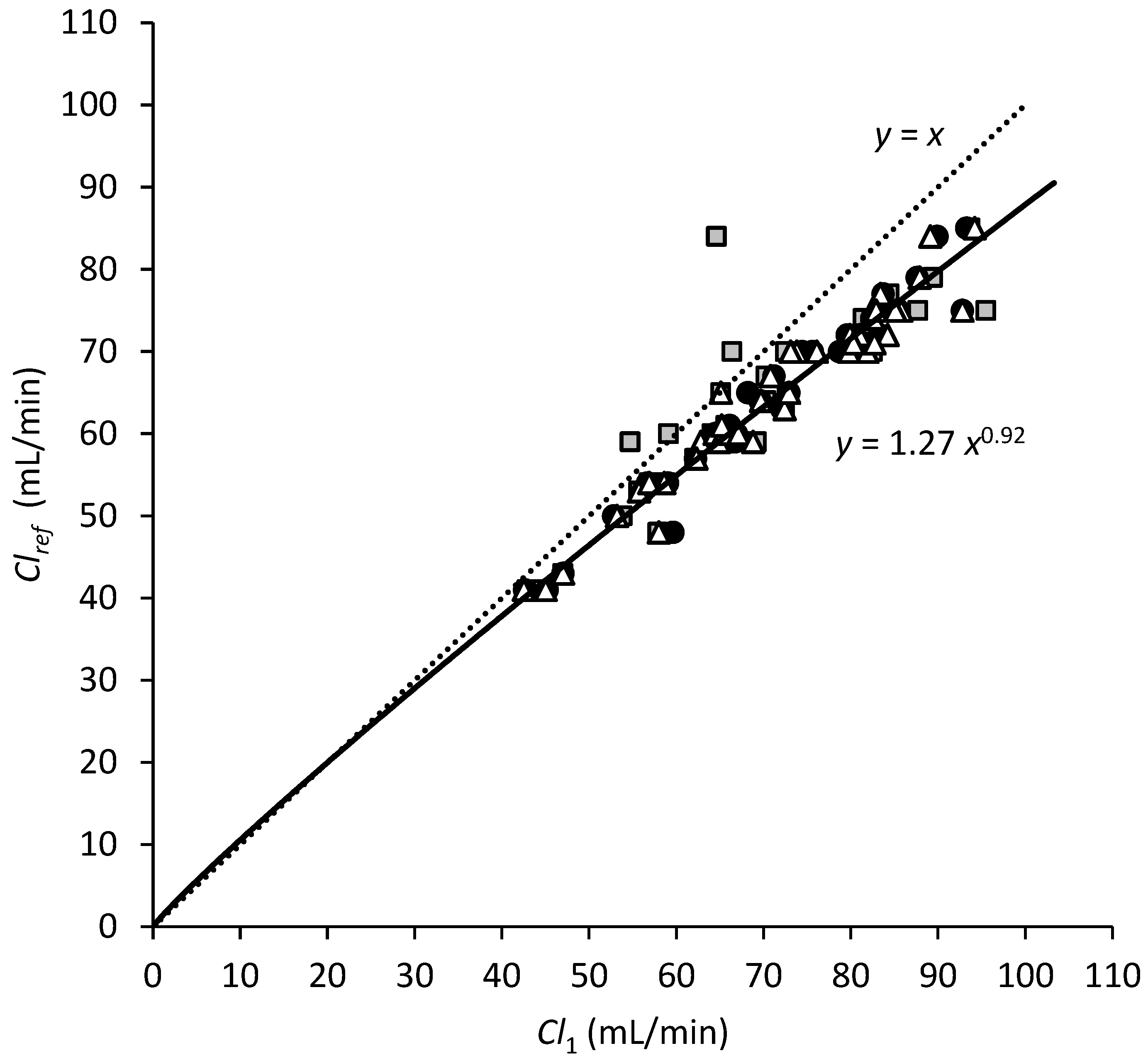
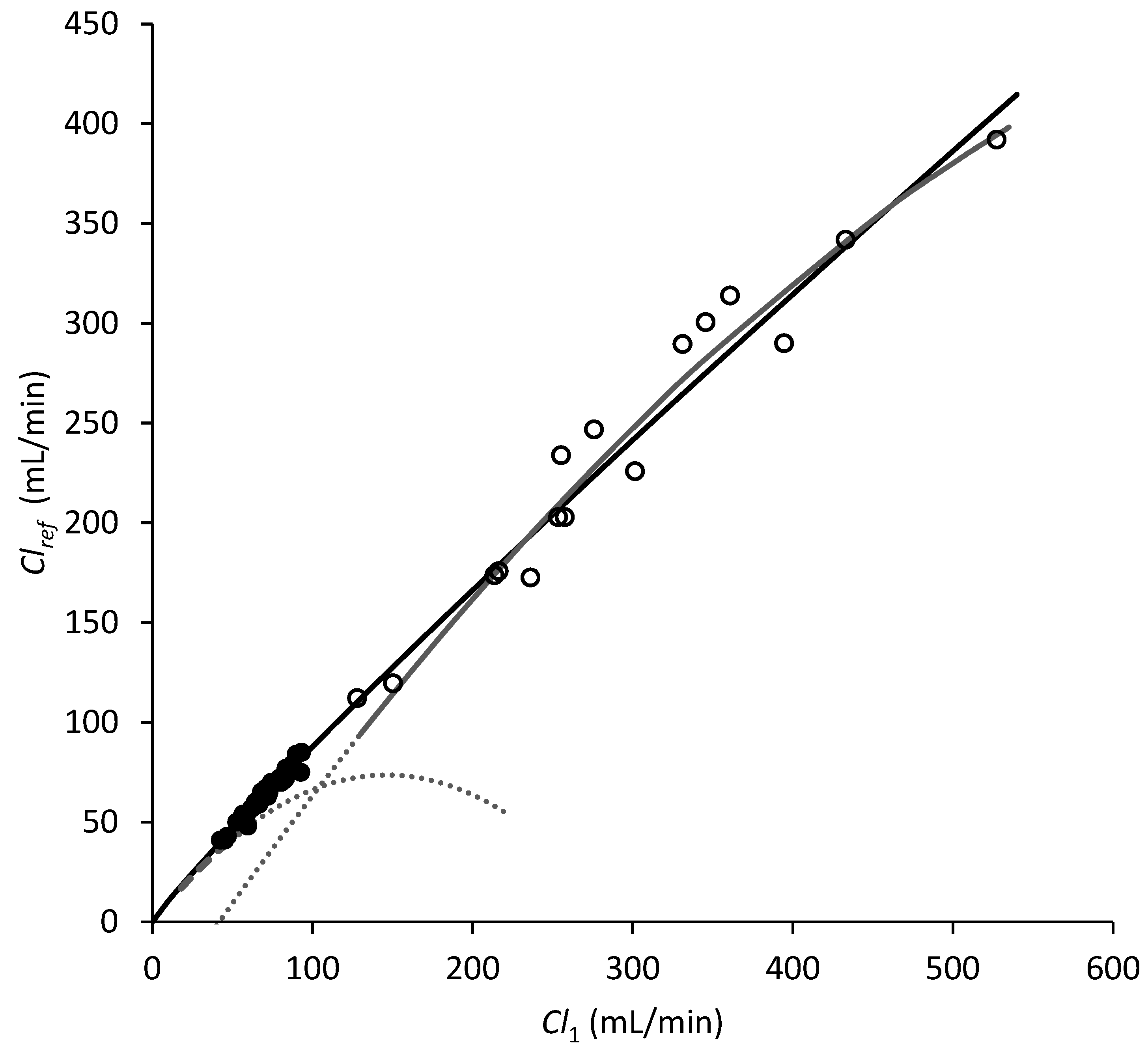
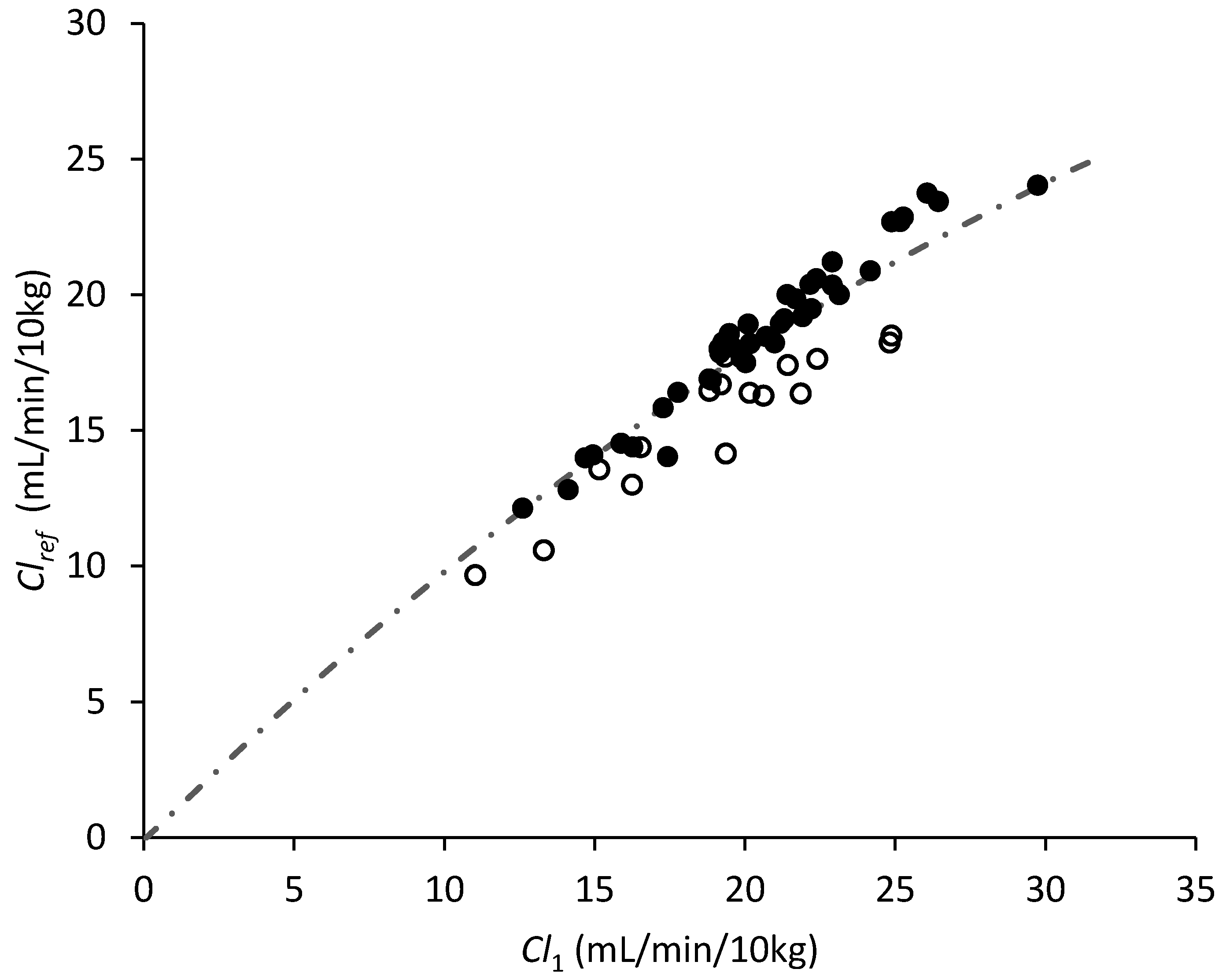
4.3. Comparison of Corrections
4.4. Time Range for Late Samples
4.5. Strengths and Limitations
4.6. Perspectives
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A. Theory of Plasma Clearance Measurements
References
- Sachs, D.H. The Pig as a Potential Xenograft Donor. Vet. Immunol. Immunopathol. 1994, 43, 185–191. [Google Scholar] [CrossRef] [PubMed]
- Luis-Lima, S.; García-Contreras, C.; Vázquez-Gómez, M.; Astiz, S.; Carrara, F.; Gaspari, F.; Negrín-Mena, N.; Jiménez-Sosa, A.; Jiménez-Hernández, H.; González-Bulnes, A.; et al. A Simple Method to Measure Renal Function in Swine by the Plasma Clearance of Iohexol. Int. J. Mol. Sci. 2018, 19, 232. [Google Scholar] [CrossRef] [PubMed]
- Chade, A.R.; Williams, M.L.; Engel, J.; Guise, E.; Harvey, T.W. A Translational Model of Chronic Kidney Disease in Swine. Am. J. Physiol. Ren. Physiol. 2018, 315, F364–F373. [Google Scholar] [CrossRef] [PubMed]
- Brøchner-Mortensen, J. A Simple Method for the Determination of Glomerular Filtration Rate. Scand. J. Clin. Lab. Investig. 1972, 30, 271–274. [Google Scholar] [CrossRef] [PubMed]
- Jødal, L.; Brøchner-Mortensen, J. Reassessment of a Classical Single Injection 51Cr-EDTA Clearance Method for Determination of Renal Function in Children and Adults. Part I: Analytically Correct Relationship between Total and One-pool Clearance. Scand. J. Clin. Lab. Investig. 2009, 69, 305–313. [Google Scholar] [CrossRef] [PubMed]
- Frennby, B.; Sterner, G.; Almén, T.; Chai, C.-M.; Jönsson, B.A.; Mansson, S. Clearance of Iohexol, 51Cr-EDTA and Endogenous Creatinine for Determination of Glomerular Filtration Rate in Pigs with Reduced Renal Function: A Comparison between Different Clearance Techniques. Scand. J. Clin. Lab. Investig. 1997, 57, 241–252. [Google Scholar] [CrossRef] [PubMed]
- van Gelder, M.K.; Stevens, J.; Pieters, T.T.; Vaessen, K.R.D.; Joles, J.A.; Verhaar, M.C.; Gerritsen, K.G.F. Simplified Iohexol-Based Method for Measurement of Glomerular Filtration Rate in Goats and Pigs. Biology 2021, 10, 461. [Google Scholar] [CrossRef] [PubMed]
- Palnæs Hansen, C.; Bie, P.; Stadil, F. Assessment of Renal Function by 51Cr-EDTA and Endogenous Creatinine Clearances in the Pig. Acta Physiol. Scand 1997, 161, 253–260. [Google Scholar] [CrossRef] [PubMed]
- Brignone, J.; Jensen, M.; Jensen, B.L.; Assersen, K.B.; Goetze, J.P.; Jødal, L.; Andersen, T.B.; Magnusdottir, S.O.; Kloster, B.; Jønler, M.; et al. Protective Effect of Sacubitril/Valsartan (Entresto) on Kidney Function and Filtration Barrier Injury in a Porcine Model of Partial Nephrectomy. Nephrol. Dial. Transplant. 2023, 38, 80–92. [Google Scholar] [CrossRef] [PubMed]
- Fleming, J.S.; Zivanovic, M.A.; Blake, G.M.; Burniston, M.; Cosgriff, P.S. Guidelines for the Measurement of Glomerular Filtration Rate Using Plasma Sampling. Nucl. Med. Commun. 2004, 25, 759–769. [Google Scholar] [CrossRef] [PubMed]
- Burniston, M. Clinical Guideline for the Measurement of Glomerular Filtration Rate (GFR) Using Plasma Sampling—Report 2018; British Nuclear Medicine Society: London, UK, 2018; p. 23. [Google Scholar]
- Andersen, T.B.; Jødal, L.; Nielsen, N.S.; Petersen, L.J. Comparison of Simultaneous Plasma Clearance of 99mTc-DTPA and 51Cr-EDTA: Can One Tracer Replace the Other? Scand. J. Clin. Lab. Investig. 2019, 79, 463–467. [Google Scholar] [CrossRef] [PubMed]
- Rehling, M.; Møller, M.L.; Thamdrup, B.; Lund, J.O.; Trap-Jensen, J. Simultaneous Measurement of Renal Clearance and Plasma Clearance of 99mTc-Labelled Diethylenetriaminepenta-Acetate, 51Cr-Labelled Ethylenediaminetetra-Acetate and Inulin in Man. Clin. Sci. 1984, 66, 613–619. [Google Scholar] [CrossRef] [PubMed]
- Simonsen, J.A.; Thilsing-Hansen, K.; Høilund-Carlsen, P.F.; Gerke, O.; Andersen, T.L. Glomerular Filtration Rate: Comparison of Simultaneous Plasma Clearance of 99mTc-DTPA and 51Cr-EDTA Revisited. Scand. J. Clin. Lab. Investig. 2020, 80, 408–411. [Google Scholar] [CrossRef] [PubMed]
- Frennby, B.; Sterner, G.; Almén, T.; Chai, C.-M.; Anders Jönsson, B.; Månsson, S. Clearance of Iohexol, Chromium-51-Ethylenediaminetetraacetic Acid, and Creatinine for Determining the Glomerular Filtration Rate in Pigs with Normal Renal Function: Comparison of Different Clearance Techniques. Acad. Radiol. 1996, 3, 651–659. [Google Scholar] [CrossRef] [PubMed]

| No. of Samples (Time Interval) | Mean Deviation ± SD | |
|---|---|---|
| Absolute | Relative | |
| five (120–240 min) | 0.0 ± 2.3 mL/min | 0.1% ± 3.6% |
| four (150–240 min) | −0.2 ± 2.6 mL/min | −0.2% ± 3.9% |
| three (180–240 min) | −1.1 ± 5.2 mL/min | −1.5% ± 7.0% |
| Luis-Lima data * | −2.2 ± 17.7 mL/min | −0.2% ± 7.2% |
| Study | Pigs | No. of GFR Measurements | GFR * | Correction Equation | |
|---|---|---|---|---|---|
| Weights | Sexes | ||||
| Present study | 26–44 kg | ♀ | 40 in 20 pigs | 41–85 mL/min | (1) |
| Frennby et al. (normal) [15] | 21–27 kg | (not stated) | 19 | 16.5–26.3 mL/min/10 kg | (2) |
| Frennby et al. (reduced) [6] | 21–26 kg | ♀♂ | 21 | 5.3–11.3 mL/min/10 kg | |
| Palnæs Hansen et al. [8] | 30–40 kg | ♀ | 12 | ~45–85 mL/min | (3) |
| Luis-Lima et al. [2] | 101–212 kg | ♀ | 16 | 112–392 mL/min | (4) |
| van Gelder et al. [7] | 34–80 kg | (not stated) | 23 in 5 pigs | ~17–44 mL/min | (5) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jødal, L.; Brignone, J.I.; Ladefoged, P.-K.C.; Lund, L.; Andersen, T.B. Robust Porcine GFR Measurement with Radiotracers and Only Late Blood Samples. Biologics 2023, 3, 296-307. https://doi.org/10.3390/biologics3040015
Jødal L, Brignone JI, Ladefoged P-KC, Lund L, Andersen TB. Robust Porcine GFR Measurement with Radiotracers and Only Late Blood Samples. Biologics. 2023; 3(4):296-307. https://doi.org/10.3390/biologics3040015
Chicago/Turabian StyleJødal, Lars, Juan Ignacio Brignone, Pui-Ki Chan Ladefoged, Lars Lund, and Trine Borup Andersen. 2023. "Robust Porcine GFR Measurement with Radiotracers and Only Late Blood Samples" Biologics 3, no. 4: 296-307. https://doi.org/10.3390/biologics3040015
APA StyleJødal, L., Brignone, J. I., Ladefoged, P.-K. C., Lund, L., & Andersen, T. B. (2023). Robust Porcine GFR Measurement with Radiotracers and Only Late Blood Samples. Biologics, 3(4), 296-307. https://doi.org/10.3390/biologics3040015






